AKSHITA MAPARI

Hi, I’m Akshita Mapari. I have done M.Sc. in Physics. I have worked on projects like Numerical modeling of winds and waves during cyclone, Physics of toys and mechanized thrill machines in amusement park based on Classical Mechanics. I have pursued a course on Arduino and have accomplished some mini projects on Arduino UNO. I always like to explore new zones in the field of science. I personally believe that learning is more enthusiastic when learnt with creativity. Apart from this, I like to read, travel, strumming on guitar, identifying rocks and strata, photography and playing chess.

Tension vs Compression:Comparative Tabular Analysis And Facts

January 21, 2024February 10, 2022 by AKSHITA MAPARI

In this article, we are going to discuss the dissimilarity between tension vs compression briefly and with detailed facts.

The following is a table differentiating between tension vs compression:-

Tension	Compression
Tensional force stretches the object tightly but the length of the object remains unchanged.	Compression is a force applied to reduce the volume or size of the object.
Tension is applied all across the string, rope, or spring due to pulling.	A string or rope can’t be compressed whereas the spring can be compressed.
The dimension of the object is unvaried.	The dimension of the object reduces on compression.
Stress on tension is called tensile stress that is responsible to pull the object away from each other.	Stress on compression is called compressive stress reduces the volume of the object.
The tension in one object makes action-reaction pairs in the opposite direction to each other.	The action-reaction pair due to the compression forces acts towards each other in one axis.
Tension on the string depends upon the mass and acceleration of the object it is attached to, and the net force acting on the object.	Compression depends upon the length, volume, area, density, and force applied to the object.
For an elastic object, the length of the object is increased on tension.	The length of the elastic object decreases on compression.
The force is transmitted through the object	The force is imposed on the object
The density of the object slightly reduces or remains unvaried	The density of the object increases
It is applicable only in 1 dimension	It is applicable in all the dimensions
Examples of tension forces are pendulums, rope bridges, hot air balloons, parachutes, rubber bands, elevators, kites, objects hanging on a hook, etc.	Examples of compression are squeezing a lemon, sponge, compressing spring, pumping, rolling a chapatti, concrete, etc.
Tension separates the objects away from each other.	Compression brings the objects closer to each other.
This is applicable only for string, springs, or ropes.	This is applicable to all materials except rope or strings.
Tension in the objects results in the deformation of the object	Compression results in contraction of the object
Tension is always positive.	The compression is a negative tension.

Tension and Compression Forces

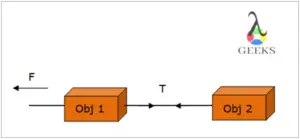
Basically, tension is created due to the action of pulling. The stretching of the rope or string does not change the length of the string, and the displacement between the objects connected by a string remains the same throughout that is if we observe object 2 from the frame of reference of object 1 then object 2 will appear to be stationary with respect to object 1.

tension vs compression — **Tension and Displacement**

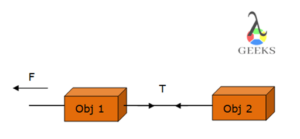
On contrary, the compression is a result of pressure exerted on the object from more than one direction, which consequences in the reduction of the volume and dimensions of the object. Due to a reduction in volume, the molecules per unit volume in the body of the object increases, and hence the density on compression increases. Well, this is not the case for the object undergoing tension.

Unlike tension, this is not exerted across the string or rope, the force due to tension is transmitted. Compression is also called a pushed force whereas tension occurs due to pulling. A tension is felt across the length between the two ends of the object whereas the compression takes place where the pressure on the area is imposed.

Tension and Compression in Bridges

Bridges undergo compression and tension at the same time. The tension comes into the act at the ends of the bridge and the tower of the bridge which supports the load of the bridge undergoes compression. Due to compression on one end of the pole, the tensional force is experienced on the other end of the pole.

When the heavy objects are carried from the bridge, the bridge is compressed due to a load, and the tension is felt underneath the base area of the bridge across the length between two poles of the bridge that support to withstand the load.

If the poles of the bridge are standing in the water bodies, then the water sagging on the walls of the poles also applies compressive force. The compressive force of the bridge is felt on the adjoining end of the poles. Tension is created across the length of the bridge between these two poles acting towards the poles. The tension forming in the bridge helps to withstand it with the compressive force exerting on it.

If you consider a suspension bridge, the cables are anchored on the bridge and are stretched and tightened to the pole. These cables go under tension when driven by the load across them, to sustain their position and provide enough tension to prevent it from collapsing and can prolong for a longer duration.

Compression and Tension Similarities

Both tension and compression are the main forces involved to determine any structure or construction. The presence of both gives better flexibility and durability for any object.

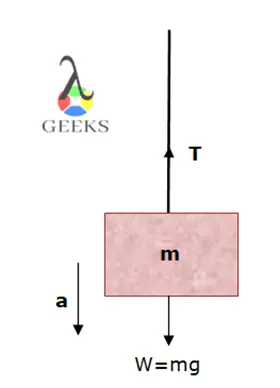
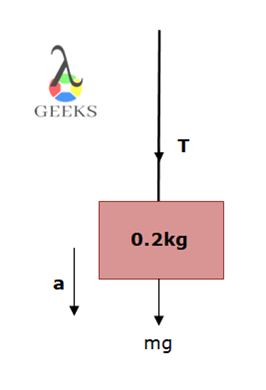
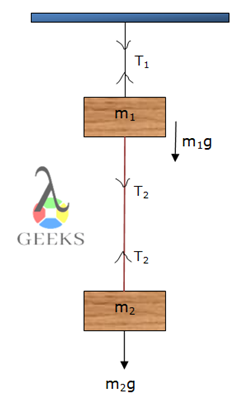
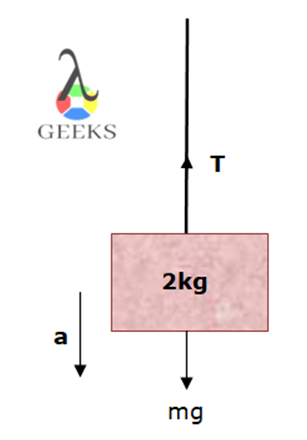
The compression and tension both are measured in the Newton. The tension in the rope due to the weight of mass ‘m’ attached to it in the below diagram is T=m(a+g).

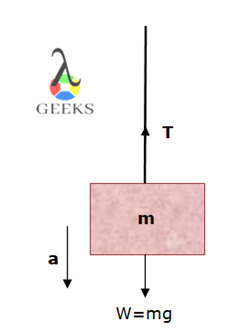
Because the force on the object is F=T-mg and since acceleration is in the negative y-axis direction, we have negative acceleration.

-ma=T-mg

If the acceleration of the object was zero then the tension in the string was just equal to the weight attached to it. That is,

T=mg

SI unit for tension is

T=kg.m/s²=Newton

Compression is also measured in Newton because it’s a force applied on the area and is formulated as

F(c)=ma

Hence, unit for compression is also F(c)=kg.m/s²=Newton.

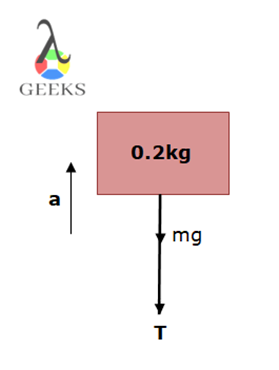
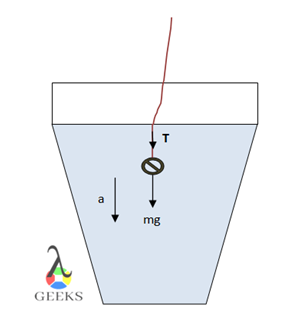
A spring or any elastic object undergoes both tension and compression. A tension is applied that results in the elongation of the object. On compression, the tension is acting downward, although changing the dimensions of the object.

The force due to compressing the elastic object is

F=-T-mg

-ma=-T-mg

As the object accelerates downward in the negative y-axis direction then the acceleration will be taken as negative and hence the negative sign.

Therefore the tension in object is

T=m(a-g)

In this case, the tension will be negative as a<g. This indicates that the negative tension in the object implies compression.

Read more on 15 List of Examples of Tension Force.

What is Better Tension or Compression?

Both, compression as well as tension led to the deformation of the objects. So, we cannot precisely say what is better among each.

If the object is undergoing both compression and tension then it will be better for an object. Because tension acts across the length of the object and acts outwards from the ends well this tensional force is canceled by the compression and hence the object is secured from getting deformed.

There are some materials that can resist the tensional force acting across them, and some materials can withstand compression.

Is Tension a Compressive Force?

Tension is not a compressive force, it is a tensile force.

Tension is opposite to the compression force, as it results in the elongation of the object whereas compression results in the contraction of the object.

Read more on Compression.

Frequently Asked Questions

What is a tension in string tied to the object of mass 5kg accelerating at a speed of 3 m/s²?

Given: m=5kg

a=3 m/s²

The tension in the string is

T=ma=5*3=15N

What will happen if there was no tension in the bridge?

Bridges are made such that they will withstand the heavy load on compression and resist the tension.

The heavy vehicle traveling on a bridge exerts a compression force on the bridge, the bridge would have bent sharply on the application of load on it.

Also Read:

Negative Tension:What,Why,When,Examples,How To Find

January 21, 2024February 9, 2022 by AKSHITA MAPARI

In this article, we will discuss what is negative tension, when it comes into the picture, and how to find it along with examples.

The negative tension comes in the act if the tensional force across the string is less than the weight of the mass attached to it. This is also true, that the tension is acting across the string, making action-reaction pair, so if we consider positive tension in the positive axis then the tension in the opposite direction has to be a negative tension.

What is Negative Tension?

The tension is exerted all across the spring, rope, or strings, and varies depending upon the mass, position, and types of forces experienced on the object attached to it.

If the influence of the tension of the string on the object is less as compared to the weight of the object the string is attached to, that is W>T, then the tension on the string is negative.

Let us understand a valid condition for tension to be negative and how it is different from the other examples. Positive tension is act exactly opposite to the weight of the object attached to it if the object is fixed at a point.

Consider an object of mass ‘m’ attached to a string. A force due to the weight of the mass is acting downward and hence the tension on the string is acting upward across the string.

The net force on the object is

F=T-mg

A force due to weight is acting in a negative y-direction, hence the negative sign.

Now, the acceleration of the object is also in the negative y-direction as the object is accelerating downward.

Hence, we have,

-ma=T-mg

Therefore, the tension in the string is

T=mg-ma

T=m(g-a)

From the above equation, we can say that the tension is negative if a>g. But, this is not a valid case. Let us see further in this article, in which situations we can find negative tension.

When Tension is Negative?

The tension is imposed on the string in the direction opposite to the force acting due to the weight of the object.

The negative tension can be considered as the acceleration of the object due to compression force, a condition where the weight of the object and the tension, both are exerted in the same direction.

The tension will be negative in the following three cases that we are going to discuss below.

Case 1: When a body is accelerating down

Consider an object of mass ‘m’ attached to a string accelerating downward. The tension on the string is also acting in the negative y-axis direction.

negative tension — **Free-body diagram**

The equation of force for the above diagram is

F=-T-mg

-ma=-T-mg

T=m(a-g)

If a<g or a=0,

Then T=-ve or T=-mg

Case 2: When a body is accelerating upward

Consider an object of mass ‘m’ accelerating upward. The tension on the string is also acting downward.

The equation of force for the above diagram is

F=-T-mg

ma=-T-mg

T=-m(a+g)

Here, in this case, the tension is clearly negative.

Case 3: An object in a vertical axis with zero acceleration

Consider an object accelerating in the vertical axis with the help of a rope. The object will experience centripetal force. Let us draw a free-body diagram for it.

The force experienced on the object in a centripetal motion is F=mv²/r which is equal to the tension on the rope if force due to weight is absent.

At a certain point while turning the object is felt heavier, during that time the tension in a rope is equal to the sum of centripetal force acting on the object and the weight of the object. At some point the object feels lighter that is when the tension acts outward, hence the equation of force becomes

F=T+mgSinθ

mv²/r=T+mgSinθ

Hence, the equation for tension becomes,

T=mv²/r-mgSinθ

A tension is negative if v=0 and θ=90⁰, that is the accelerating object stops at 90 degree angle.

Can Tension be Negative?

A tension is positive when the force is applied to pull the object with the help of a string, or rope.

If instead of pulling, a compressive force is applied, then the tension on the string can be negative. This could also be a case when the strength of the tension on the string is less compared to the weight attached to it.

This is also categorized as the compression force on the string. But string or rope can’t be compressed; only the spring can be compressed. Hence, on the application of the compressive force, the tension on the spring is negative.

How to Find Negative Tension?

Negative tension is simply a compression force and acts always in the direction of the weight of the object.

The negative tension in the spring can be calculated by measuring the net force imposed on the object and then finding the acceleration of the object due to force.

Let us understand how to calculate the negative tension by solving the problem given below.

Problem: An object of mass 200 grams attached to a spring is compressed due to which the acceleration of the object is found to be 1m/s. Find the tension in the string.

Given: m=200 grams = 0.2kg

a=1m/s

g=9.8m/s²

Let us first draw a free-body diagram for the same.

Now, write the equation of force.

F=-T-mg

The acceleration of the object is in the negative y-axis plane, hence

-ma=-T-mg

Therefore, the tension on the spring is equal to

T=ma-mg

T=m(a-g)

Now, substitute the given values

T=0.2kg*(1-9.8)m/s²

T=0.2kg*(-8.8)m/s²

T=-1.76N

The tension on the sprig is -1.76N.

Negative Tension Examples

There are various examples of the negative tension that we often come across. Let us ponder upon some examples.

Drowning of Bolt in the Water

Consider a bolt tied to a tread dropped in a glass of water. The molecular density of the bolt is more than the water, the bolt will immerse in the water accelerating down to the bottom of the glass.

If we write the equation of force for tension, then we have

F=-T-mg

T=-F-mg

The acceleration of the bolt is downward, hence,

T=-(-ma)-mg

T=m(a-g)

If the mass of the bolt is 4 grams, and the acceleration is 0.03m/s², then tension on the tread is

T=4*10^-3kg* (0.03-9.8)m/s²

T=4*10^-3kg* (-9.77)m/s²

T=-0.039N

The tension on the tread is -0.039 Newton.

Lantern Hanging on the Hook with String Suddenly Detach and Falls Down

When the string detached from the lantern, the weight of the lantern is larger than the tension across the string, and hence the lantern accelerates downward.

Spring Shoes or Jumping Shoes

These shoes come with a spring attached beneath the shoes or a bouncer. When the body weight falls on the shoe it compresses. This time the body accelerates little downward, and the tension applied on the spring is also acting downward. Well, due to the potential energy accumulation in the spring and because of its elastic nature it regains its shape. That is why the spring is used in shoes to jump higher.

A Ladder on the Helicopter

Imagine that there is no person standing on the latter and the helicopter is accelerating in the upward direction. The tension may be applicable to the latter due to the air resistance. Well, the acceleration is upward and the tension is in the negative direction.

The mass m=0, hence the equation of force will be

F=-T

That is T=-ma.

Skipping

Tension on a massless rope is always zero. Because the tension on one end of the rope is canceled out by the equal and opposite tension from the other end. While skipping, the tension is exerted across the rope, but it is not positive because no pulling force is applied on the rope. The rope undergoes air resistance and the tension is created in the rope due to centrifugal force.

Loosening a Guitar strings

The tension on the strings on the guitar is created to generate the sound by bending the string or strumming it. If we loosen the string, then the tension on the string will be negative.

Balloon Floating in the Air

A balloon is filled with helium gas which is very light and hence freely floats to rise above the air. The density of helium is very less compared to the air molecules.

The balloon experiences a buoy force that carries it in the upward direction. The net force on the balloon is given by the equation

F_buoy=ρ vg=-T-mg

Hence, tension is equal to

T=-ρvg-mg

T=-(ρ v+m)g

The tension on the tread of the balloon is negative.

Read more on Tension.

Frequently Asked Questions

Is stress a negative tension?

Stress is applied in such a way that it results in pulling two objects apart from each other, and it is called tensional stress.

Tensional stress is exerted in two opposite directions, separating or pulling the objects away from each other. If the tension is acting on the x-axis, then the tension in the left hand side is a negative tension.

Why compression is a negative tension?

The force applied to reduce the volume or size of the object is called compression.

On compression the tension is acting in the negative y-direction along with the weight of the object, then acceleration could be in positive or negative axis, the tension is always negative.

Also Read:

Tension Between Two Blocks: 5 Problem Examples

January 21, 2024February 7, 2022 by AKSHITA MAPARI

Dive into our example-rich guide on Tension Between Two Blocks, simplifying this key concept in physics for easy understanding

The tensional force acting on the string is not the same for all objects; it depends upon the mass and the acceleration of the object, and the force. Let us see how to find the tension between two blocks.

How to Find Tension between Two Blocks?

It is a force defined for strings, rope, or springs; tread like objects which experience tension on stretching.

The tension between two blocks can be found by knowing the net forces acting on the two blocks attached to the string, we can calculate the tension exerted on the string due to the two blocks.

Read more on 15 List of Examples of Tension Force.

Problem: Consider two blocks of masses ‘m₁’ and ‘m₂’ attached to a rope and suspended freely in the air. Calculate the tension imposed on the rope due to two blocks.

Solution: The tension felt on the rope is due to the blocks hanging on it, and relies upon the masses of the blocks.

Step1: Draw a free-body diagram for any problem

To calculate the tension on the rope, first draw the free body diagram understanding the problem, explaining the net forces acting on the blocks. Here, is a free body diagram of the two blocks for the above problem.

The diagram above gives us a rough idea of the tension generated in the rope due to two blocks. The tension T₁ is due to the mass ‘m₁’ and tension T₂ is exerted due to the mass ‘m₂’. The tension is felt across the length of the rope and in both the direction, in the positive y-axis and in the negative y-axis direction. The force due to gravity is acting downward due to both the weights is clearly indicated in the free-body diagram.

Step 2: Write the equation for net forces acting on each block.

The net force acting on the mass ‘m₂’ is the tensional force and the force due to gravity acting downward in the negative y-direction. So, we have the equation as below,

F=T₂-m₂g

m₂a=T₂-m₂g —-(1)

The net force acting on the mass ‘m₁’ is the tensional force and weight acting downward in the negative y-direction. So, we can write the equation as,

F=T₁ – T₂ – m₁g

m₁a=T₁ – T₂ – m₁g —(2)

Step 3: Frame the equation to find the net acceleration of the blocks.

The mass ‘m₂’ is fixed and is not accelerating, hence a=0. Therefore we can write Eqn(1) as,

T₂ – m₂g=0—(3)

The mass ‘m₁’ is also fixed at a point and is not accelerating, hence a=0. Therefore from Eqn(2), we have,

T₁-T₂-m₂g=0 —(4)

It doesn’t mean that if there is no acceleration in the rope then there is no tension in the rope, this is evident from the above equation that there is a tension exerting in the rope due to each block. Let us see further how to find this tension in the rope.

Step 4: Calculate the total tension on the rope

From the Eqn(3), we have

T₂= m₂g

The tension T₂ is applicable due to mass ‘m₂’ and the acceleration due to gravity, which is equal to the weight of block 2.

From eqn (4) we have,

T₁ = T₂ + m₂g

Substituting the value to T₂, we now have,

T₁ = m₁g + m₂g

So, T₁=g(m₂+m₁)

The tension T₁ is due to the total mass attached to the string, as the rope exerting tension T₁ is exerting weight of both the blocks.

Step 5: Find the net tension experiencing on the rope

The net tension is the sum of all the tensions exerted on the rope. Hence, net tension T is equal to the addition of T₁ and T₂,

T=T₁+T₂

T=g(m₂+m₁)+m₂g

T=m₁g+2m₂g

T=(m₁+2m₂)g

This is a net tension in the rope due to two blocks suspended vertically above the ground.

Tension between Two Blocks on an Incline

Now, that we know how to calculate the tension between two blocks in a vertical direction, let us also ponder upon how to measure tension between the two blocks on an inclined slope.

Problem: Consider two blocks of masses 30kg and 45kg inclined on the slope attached to string on a pulley. The inclination angle of the slope on which mass of block ‘m₁’ lies is at angle 30⁰, and the slope on which mass ‘m₂’ relies is inclined at an angle 45⁰. Calculate the tension exerted on a string.

Solution: First, let us draw a free-body diagram of the two blocks inclined on the plane of different angles.

Now, write the equation for forces exerting on the blocks. The net force exerted on each body is the additional forces due to weight, gravity, the normal force which acts opposite to the weight of the body, and the tensional force exerted across the string.

The forces acting on mass m₁ are in 2 directions, in the x-direction is, m₁a=-m₁gSin30⁰+T, minus sign is because the force is acting in the negative x-axis; and in the y-direction is m₁a=-m₁gCos300+N.

The forces acting on mass m₂ are, in the x-direction is, m₂a=m₂gSin45⁰-T

The tension is exerted in the negative x-axis.

And, in the y-direction is m₂a=-m₂gcos45⁰+ N

The tension comes into existence in the x-direction; hence we will consider 2 equations,

m₁a=-m₁gSin30°+T

m₂a=m₂gSin45°-T

Adding these two equations, we have,

m₁a + m₂a=m₂gSin45°-T – m₁gSin30°+T

Let us calculate acceleration of the blocks, so let’s substitute the value given.

(30+45)a=9.8* (45*1/√2)-30* (1/2)

75a=9.8* (22.5√2-15)

75a=9.8*(31.82-15)

a=9.8*16.82/75

a=2.2 m/s²

Now, we know the acceleration of the blocks. Substituting this in any of the above equations we can find the tension in the rope due to two blocks.

Consider the equation, m₁a=-m₁gSin30°+T

T=m₁a+m₁gSin30°

T=m₁(a+gSin30°)

=30* (2.2+9.8*1/2)

=30* (2.2+4.9)

=30*7.1=213N

Hence, the tension in the rope is 213N.

Find Tension between Two Blocks on Horizontal Surface

The tension between the blocks placed on a horizontal surface comes into the picture when the pulling force is applied to one of the blocks. On pushing the object closer to each other or away from the other, the tension will be absent in the string joining the both.

Read more on Tension Force.

Problem: Consider two-block kept on a frictionless plane having mass m₁=3kg and m₂= 5kg. Both these masses are tied with a string, and a mass of 5kg is pulled in the positive x-direction applying a force of 230N. Measure the tension exerted on the string.

Solution: Let us draw a free body diagram considering the above situation,

The tension acting on block 1 is equal to the force due to acceleration, given by the equation,

T=m₁a=3a

The force applied on the block 2 is

F=T+m₂a

Tension due to block 2 is

T=F-m₂a

If we know the acceleration of the block, then it is easy to calculate the tensional force.

The net force applied on the blocks is

F= (m₁+m₂)a

Hence,a=F/m₁+m₂

From this, we can write the first equation as

T=3F/m₁+m₂

Hence, the tension exerted on the string is

T=3* 230/3+5

=3*230/8=86.25N

Tension of 86.25N is applied across the string.

Frequently Asked Questions

A body of mass 2kg is attached to the string and is accelerating downward at a rate of 3m/s. What is tension on the string?

Given: m=2kg

a=3m/s

The force acting on the object is

F=T-mg

Since the acceleration of the body is downward F=-ma,

-ma=T-mg

T=m(g-a)

=2* (9.8-3)

=2*6.8=13.6N

The tension on a string is 13.6N.

What is a tension Force?

The force acting on every object is categorized depending upon the shape and size of the object and the direction of the force.

The tensional force is a contact force and acts on pulling the objects. The force acting across the length of the rope, strings, or springs is called the tension force.

Also Read:

Relationship Between Frequency And Energy: Problems, Example And Detailed Facts

January 20, 2024February 5, 2022 by AKSHITA MAPARI

In this article, we are going to discuss the relationship between frequency and energy, along with it we will solve some problems related to the topic with examples.

The frequency of the particle is relative to its energy. If the particle possesses a higher amount of energy, then, the frequency of the particle will be lofting, and hence the speed will be more.

Relationship between Frequency and Energy of Wave

The energy of a wave is characterized by the frequency of the occurrence of particles in a wave. The energy of any body is related to its frequency by the equation

E=hnu

Where h is a Planck’s constant h=6.626 * 10^-34 J.s

nu is a frequency of the wave

The energy and the frequency are directly related to each other. If the energy possessed by the particle oscillating in a wave is more, then the frequency of the particle will be more.

It is also evident that a particle having greater energy will travel at high speed thus the wavelength of the propagating wave is less.

Problem 1: Find the energy of the particle having a frequency of 66PHz.

Given: h=6.626*10^-34J.s

nu =66PHz= 66*10¹⁵Hz

We have,

E=hnu

= 6.626 *10^-34*66*10¹⁵

= 437.3*10^-19Joules

The energy of the particle of frequency 66PHz is 437.3 *10^-19Joules.

Graph of Energy and Frequency of Wave

The particle will travel at high speed if the amount of kinetic energy acquired by the particle is more. The speed of the particle is directly proportional to its wavelength. If the length of the wave of the particle is more, then the frequency of its occurrence will be lessened. The wavelength is inversely related to the energy of the particle.

The following is a graph of the energy v/s frequency of the waves.

The energy of the wave is directly related to the frequency. As the frequency of the wave increases, the energy will simultaneously increase, hence we have a linear slope of the graph.

Relationship between Frequency and Energy of Radiation

The frequency of the wave radiated from the object is more, when the radiations received by the surface of the object are high. The frequency of the wave is reduced at cooler temperatures as the wavelength of the radiated wave increases. At cooler temperatures, the emission of radiations is less, and radiations are emitted at the greater wavelengths.

Based on the wavelength and the frequencies of the wave, the waves are classified as follows:-

Name	Radio Waves	Microwaves	Infrared	Visible	Ultra Violet	X-rays	Gamma rays
Wavelength	>1m	1mm-1m	700nm-1mm	400nm-700nm	10nm-380nm	0.01nm-10nm	<0.01nm
Frequency	<300MHz	300MHz-300GHz	300GHz-430THz	430THz-750THz	750THz-30PHz	30PHz-30EHz	>30Ehz

From the above table, it is clearly indicating that as the wavelength of the radiations decreases the frequency of the wave increases. As the frequency of the waves increases, it implies that the energy of the waves also increases because the energy is linearly proportional to the frequency of the radiations.

The emissivity of the radiations is dependent on the dimensions of the object, the composition, and the color. The power of the emitted radiation is directly dependent upon the fourth power of the temperature the surface of the system is subjected to. The energy of the emitted radiations is given by the equation

U=ɛΣT^4A

Where U is a radiated energy

ɛ is the emissivity of radiation from the object

Σ is the Stefan-Boltzmann constant and is equal to Σ=5.67*10^-8W/m²K⁴

T is an absolute temperature

A is the area of the object

The black-colored objects are said to have an emissivity 1 because no radiations received by the black body are emitted by the object. All the radiations are completely absorbed by the black body. Whereas the white-colored object has the emissivity 0, as all the radiations that fall on the white objects are reflected back hence no radiations are absorbed by the objects.

As the temperature of the system increases, the frequency of the radiations is also increased parallelly.

The above graph shows that as the temperature of the system increases, the energy of the emitted radiations increases with temperature. At low temperatures, the energy associated with the emitted radiations from the surface will be less; hence the radiations of higher wavelengths are emitted. Due to this, the frequency of the waves is decreased.

But, during the higher temperatures of the surrounding, the system will receive more amount of energy from the incident photons, hence the waves of fewer wavelengths will be given out, thus the frequency of the waves will increase. Well, the frequency linearly increases with increasing energy.

Read more on Radiation.

Problem 2: An object having a surface area of 28 sq.m is subjected to the temperature of 1120 Kelvin. The emissivity of the surface is 0.3. Calculate the rate of energy radiated from the object.

Given: ɛ =0.3

Σ=5.67*10^-8W/m²K⁴

T=1120 K

A= 28 sq.m

The energy radiated by the box is

U=ɛΣT^4A

=0.3*5.67*10^-8*112⁰⁴*²⁸

= 2.67*10⁴ Watts

= 26.7 KW

The power of the emitted radiations is 26.7KW.

Relationship between Frequency and Energy of the Photon

The photon is a quantum particle of light that has zero rest mass. The energy of the photon in the electromagnetic radiation is directly proportional to the frequency of the photon and is given by the relation,

E=hnu

Where ‘h’ is a Planck’s constant h=6.626 * 10^-34J.s

nu is a frequency of the photon

The frequency of the photon is correlated to the speed and wavelength of the electromagnetic wave.

nu =v/λ

Since a photon is massless, the velocity of the photon is equal to the speed of light. Hence, the frequency of the photon is,

nu =c/λ

Therefore, the energy of the photon is related to the wavelength by the equation,

E=hc/λ

h=6.626 * 10^-34J.s

c is a velocity of light c=3*10⁸m/s and

λ is a wavelength of the photon

If we know the wavelength of the particular light, then we can calculate the frequency and thereof the energy of the photons emitted by the source of light.

Problem 3: The wavelength of the sodium light beam is 588 nm. Calculate the frequency of the photon emitted from the sodium beam. Also, calculate the energy of the emitted photons.

Given: λ=588 nm

h=6.626 *10^-34J.s

c=3 *10⁸m/s

The frequency of the emitted photons is

nu =c/λ

=3 *10⁸/ 588 *10^-9

=176.4 THz

The frequency of the photon is 176.4 THz.

Now, the energy of the photon is

E=hnu

= 6.626 *10^-34*1.764 *10¹⁴

= 11.67 *10^-20 Joules

The energy of the photons is 11.67 * 10^-20 Joules

Relation between the Kinetic Energy and the Frequency

The kinetic energy of the particle having a mass ‘m’ and travelling with velocity ‘v’ is given by the formula

K.E=1/2 mv²

The velocity of the particle is directly related to the wavelength and the frequency of the wave of the particle. The frequency of the particle is given by the relation

f=v/λ

Where f is a frequency of the particle

V is a velocity and

λ is a wavelength of the particle

Hence,v=fλ

Using this in the above equation,

E=1/2 mf²λ²

2E = mf²λ²

2E/m = f²λ²

f²=2E/mλ²

f=√(2E/mλ²)

f=1/λ √(2E/mλ)

The above equation gives the relation between the energy and the frequency of the particle.

Example: Calculate the frequency of the electron possessing energy of 0.511 MeV. The transiting electrons are traveling with the wavelength of 530 nm.

Given: E = 0.511 MeV

E=0.511*10⁶*1.6*10^-19=0.817*10^-13Joules

λ =530nm

m = 9.1 × 10^-31 kg

We have,

f=1/λ √2ME

=1/(530 * 10^-9) √2(0.18*10¹⁸)

=0.42*10⁹/530*10^-9

=0.79*10¹⁵

=0.79 PHz

The frequency of the electron is 0.79 PHz.

Frequently Asked Questions

What is the frequency of the photon having the energy of 16 × 10^-10 Joules?

Given: E = 16 × 10^-10 Joules

h=6.626 * 10^-34.Js

We have,

E=hnu

nu =E/h

=16*10^-10/6.626 *10^-34

=2.41*10²⁴Joules

The energy of the photon is 2.41*10²⁴ Joules.

What is the wavelength of the particle of mass 1.67 × 10^-27 kg traveling at the speed of 2.5 × 10⁸ m/s?

Given: v = 2.5 × 10⁸ m/s

h=6.626 *10^-34J.s

c=3 *10⁸m/s

The kinetic energy of the photon is

K.E=1/2 mv²

=1/2 * 1.67*10^-27 ( 2.5*10⁸ )²

= 5.22*10^-11Joules

Using de Broglie’s equation,

λ=h/p

The momentum of the particle,

p=√2ME

Hence,

λ =h/ √2ME

=6.626 * 10^-34/√(2*1.67* 10^-27*5.22*10^-11

=6.626* 10^-34/√17.43*10^-38

=1.58 * 10^-15m

The wavelength of the particle moving with velocity 2.5 × 10⁸ m/s is 1.58 × 10^-15 m.

What is a speed of the particle having a wavelength 2.68 pm and energy of 0.45MeV?

Given: E=0.45MeV=0.45*10⁶* 1.6*10^-19=0.72*10^-13Joules

h=6.626 *10^-34 J.s

c=3 *10⁸m/s

We have E=hnu

nu =E/h

=0.72*10^-13/6.626 *10^-34

=0.108*10²¹=108EHz

Now, frequency is related to the speed by the relation

nu =v/λ

v=nuλ

v=108* 10¹⁸*2.68*10^-12

=2.89*10⁸ m/s

The speed of the particle is 2.89*10⁸m/s.

How does the frequency affect the energy of the particle?

The energy is directly related to the frequency of the particle.

As the frequency of the wave increases, the energy of the particle propagating in the wave also increases.

Does the speed of the wave vary with the energy?

The speed of the particle increases on gaining the higher energy.

The frequency of the particle depends upon the energy which is directly proportional to the speed of the particle.

How does the frequency affect the power of the radiations?

The power of the radiations depends upon the emissivity of the objects and the temperature at which they are exposed.

At higher frequencies, the power of radiations is the maximum and at lower frequencies of the radiating photons, the power of the radiations is the minimum.

Also Read:

Effect Of Wavelength On Refraction:

January 20, 2024February 5, 2022 by AKSHITA MAPARI

$Effect Of Wavelength On Refraction: 23$

In this article, we will discuss the effect of wavelength on refraction, how does the refraction of the waves affect by the wavelength with detailed facts.

The wavelength governs the speed of the propagating wave in the medium. Depending upon the speed of the wave and the refractive index of the medium, it bends in the medium and gets refracted.

Does Wavelength Affect Refraction?

The wavelength of the propagating wave is directly correlated to the velocity of the light or a particle traveling in a wave.

The incident wave on the medium having a refractive index ‘n₁’ traveling at a speed ‘v’ and wavelength λ will refract according to the variations of the wavelength while propagating in the medium of a refractive index ‘n₂’.

The refractive index of the medium is related to the speed of the light by the equation

n=c/v

Where n is a refractive index of the medium

C is a speed of light, c=3*10⁸m/s

And v is velocity of the light on refraction

If the wavelength of the beam of particles increases, then the frequency, and thereof the energy of the particles decrease. It is evident that the wavelength of the light on refraction does not change, that is the wavelength of the light before and after the refraction of the same light is the same.

But, the speed of the propagation of the wave depends upon the wavelength of the light. The relation between the speed and the wavelength of the light is formulated as

f=v/λ

Where f is a frequency of light

V is a velocity and

λ is a wavelength of the light

The energy of the particle is directly proportional to the frequency of the oscillating particle, and is given by the equation,

E=hf

Where ‘h’ is a Planck’s Constant, h=6.626*10^-34J.s

The longer the wavelength, the less will be the energy associated with the particle, and thus the speed of the particle will be less. The particle traveling with a smaller wavelength will have higher energy pertaining to the particle and hence will travel with higher velocities.

Example: A photon of energy 0.58 MeV is incident on the medium having refractive index 1.33. Find the wavelength of the incident photons.

Given: n=1.33

E=0.58MeV=0.58*10⁶*1.6*10^-19=0.93*10^-13Joules

h=6.626*10^-34 J.s

The energy of the photon is equal to

E=hf

Hence, the frequency of the photon is

f=E/h

=0.93*10^-13/6.626*10^-34

=0.14*10²¹

=140*10¹⁸

=140EHz

The frequency of the photon is 140EHz.

The refractive index of the medium is the ratio of change in the speed of light.

n=c/v

Hence, the velocity of the light is

v=c/n

=3*10⁸/1.33

=2.25*10⁸m/s

The speed of the photon is 2.25*10⁸m/s

Therefore, the wavelength of the photon is

v=fλ

λ=v/f

=2.25*10⁸/140*10¹⁸

=0.0161*10^-10

=1.61*10^-12=1.61pm

Hence, the wavelength of the photon in the medium of refractive index 1.33 is 1.16 pm.

How does Wavelength affect Refraction?

The propagation of the wave in any medium is defined by the length of the wave, its time period, and the frequency of the particle in a wave.

Though the wavelength does not change drastically on refraction, the speed of the particle relies upon the wavelength. If the wavelength is more, the speed will be less; and the speed acquired by the particle is high if the wavelength is very less.

If the light of the greater wavelength is incident, then the refracted beams of particles will possess less energy, and hence the speed of light will be reduced and refract at smaller angles.

effect of wavelength on refraction — **Refraction of light**

If the beam of the particle of a smaller wavelength is incident, then the particle will possess higher energy, and hence on refraction, the beam of the particle will have a sufficient speed to travel at a certain speed and refract at greater angles.

Read more on Types Of Refraction: Comparative Analysis.

How does Wavelength affect Angle of Refraction?

The wavelength of the light affects the speed and the frequency of the wave.

If the wavelength is bigger, then the speed of the light will be smaller and the light will reflect at a smaller angle; and if the wavelength is small then the light will reflect at the greater angle.

Consider a light incident from the medium 1 of refractive index ‘n₁’ on the surface of the object having a refractive index ‘n₂’. The refractive index is the ratio of change in the speed of light while propagating from medium 1 to medium 2.

n₁₂=v₂/v₁

Where n₁₂ is the ratio of the refractive index of the medium 1 to medium 2,

v₁ is a speed of light in medium 1

v₂ is a speed of light in medium 2

The velocity of the light is related to the wavelength by the relation

v=fλ

At a constant frequency of light, if λ₁ and λ₂ are the wavelength of the light traveling from medium 1 to medium 2 respectively, then the refractive index of the medium is related to the wavelength as,

n₂/n₁=λ₂/λ₁

By Snell’s Law,

n₁sin θ_i = n₂ Sin θ_r

n₁/n₂=sin θ_i/sin θ_r

Hence, relating to the above equation,

λ₂/λ₁ =sin θ_i/sin θ_r

sin θ_r=λ₂λ₁/sin θ_i

θ_r=Sin^-1 λ₂λ₁/sin θ_i

The refractive angle depends upon the variations in the wavelength of the light and the incident angle of the beam as per the above equation.

Read more on Refraction.

Frequently Asked Questions

What is the angle of refraction if the light beam of a wavelength of 450nm is incident on the medium of refractive index 1.33 at an angle of 45 degrees?

Given: n₁=1

n₂=1.33

λ₁ =450nm

We know that,

n₁/n₂=λ₂/λ₁

1/1.33=λ₂/ 450nm

λ₂=450nm/1.33

λ₂=338.34nm

Hence, the wavelength of the light in the medium decreases to 338.34.

The refractive angle of the light is

θ_r=Sin^-1 λ₂λ₁/sin θ_i

θ_r=Sin^-1 338.34*450/sin 45

θ_r=Sin^-1 338.34*450/ (1/√2)

θ_r=Sin (-1)/ (0.53)

θ_r=35.56

The light refracts at 35.56 degree angle.

How does the speed of the wave change on refraction?

As the light enters the denser medium, the speed of the wave decreases.

The frequency of the light decreases on entering the denser mediums and hence the energy reduces reducing the speed of light.

Does the angle of refraction depend upon the speed of the light?

If the wavelength of the particle is small, then the particle possesses high speed.

The greater the speed of the wave, the light will bend at a bigger refractive angle.

Also Read:

Transverse Wave vs Longitudinal Wave: Detailed Explanations

January 21, 2024February 4, 2022 by AKSHITA MAPARI

In this article, we will elaborately discuss the differentiation between transverse wave vs longitudinal waves with a detailed explanation.

Depending upon the propagation of the waves with the vibrational motions of the particle, the waves are differentiated into two; the longitudinal waves and the transverse waves.

Transverse Wave	Longitudinal Wave
The wave has crest and trough	The wave has compression and rarefaction
The propagation of a wave is parallel to the direction of motion of the vibrating molecules	The propagation of the wave is perpendicular to the direction of motion of the vibrating molecules
A transverse wave cannot travel through fluid and propagates from solid mediums and over the liquid surfaces	The longitudinal waves can travel through any medium whether it is solid, liquid, or gas
The propagation of the transverse waves depends upon the displacement of the vibrating molecules along with the distance	The propagation of the longitudinal wave depends upon the density of the medium
The graph for transverse wave will be displacement v/s distance graph	The graph for longitudinal wave will be density v/s distance graph
The density and pressure does not vary in this case	The pressure and the density of the waves is maximum in the region of compression and varies periodically
Examples of transverse waves are ripples on water, sunlight, electromagnetic waves, the vibration of string, oceanic waves, etc.	Examples of longitudinal waves are sound waves, drumming, thundering, earthquakes, tsunami, ultrasound waves, etc.
They are also called shear waves or S-waves	They are also called pressure waves, compression waves, primary waves or p-waves
The frequency and wavelength is constant throughout the propagation of a wave	The frequency of the wave is maximum at the region of compression
This wave acts in two dimensions, the propagation of the wave in one axis and the motion of particles in another	These waves act only in one dimension as the direction of wave and motion of the particle is in the same plane
A transverse wave can be polarized	Longitudinal waves can’t be polarized

What is Transverse Wave?

A transverse wave penetrates in a direction making 90 degrees with the path due to the oscillations of particles.

Transverse waves are formed due to the vibration of particles. If the motion of particles vibrating is in the y-axis then the wave will propagate in the x-axis.

The transverse waves are also called the shear wave as these waves can lead to deformation of the object upon which these waves are traversed. They are sustained for the shortest distance and are not able to penetrate from fluid mediums although they travel over the liquids. They can travel only from solid state.

What is a Longitudinal Wave?

The motion of the longitudinal waves is along the path traversed by the vibrating particles.

The energy of the vibrating molecules is transmitted to the subsequent molecules in the path, and hence the wave propagates along with it.

The longitudinal waves can travel through any medium and hence travels at a longer distance. Unlike the transverse wave, the longitudinal wave consists of regions of compression and rarefaction instead of trough and crest respectively. The density of waves is highest at the compression than that of rarefaction.

Difference between frequency and wavelength of a transverse wave and longitudinal wave

The wavelength of a transverse wave is a length between two subsequent crests or troughs and is consistent with time throughout the propagation. The amplitude of the wave may decrease at the time of vanishing but the wavelength is constant. The frequency of the transverse wave remains constant through the propagation of the wave.

The frequency of the longitudinal wave is highest in the compression region than that of rarefaction. The density of the wave is less in the region of rarefaction and hence the pressure is the minimum, whereas, the pressure is more where the density of the waves is more. Due to the pressure difference the heat is generated, hence constant temperature conditions are required which is essential for the propagation of waves at a longer distance.

What are seismic waves?

Seismic waves are of two types, s-wave, and p-waves. The s-wave is a transverse wave and the p-wave is a longitudinal wave.

Seismic waves are generated due to the plate tectonic activities like an eruption of magma, plate movement causing convergent or divergent plates, earthquakes, landslides, explosives, etc.

These are low frequency waves that are mostly not even felt by human beings. Seismometers are used to trace the wave to get alerts of activities beneath the Earth’s crust. Well, a p-wave called a primary wave is the first to trace in the seismometer which is a longitudinal wave. As the molten magma rises upward the movement of the particles produces vibrational waves. The longitudinal waves are capable to pass any medium, thus crossing the asthenosphere, and are traced on seismometers that give the evidential alert of volcanic activities before the myth hit the surface crust.

Whereas, the shear waves are not able to penetrate the liquid state asthenosphere which is rich in tetrahydrate molten magma. Unable to traverse through, the s-wave also called secondary waves can travel only through the solid rocks. It gives an idea of only the activities happening on the Earth’s crust.

Direction of Propagation of Transverse Waves and Longitudinal Waves

If the oscillation of the particles is on the y-axis then the transverse wave will propagate on the x-axis. The transverse wave always travels at an angle of 90 degrees to the motion of the vibrating particles.

If the oscillation of the particle is in the x-axis then the longitudinal wave will travel on the x-axis. The longitudinal wave travels in a plane making an angle of 180 degrees to the direction of motion of vibrating molecules.

Polarization of Transverse and Longitudinal Wave

Polarization is a method to avoid the vibrations of a wave by restricting it to one direction of propagating.

The source is said to the polarized if the vibrations from the incident source are confined only in one direction on polarization.

The transverse waves when passed from one vertical slit and one horizontal slit, the waves will traverse unpolarized from the first slit and on proceeding to the horizontal slit; no vibrations of the particle will pass through giving zero amplitude of a wave. Hence, the transverse waves can be polarized.

laser g9c7076995 640 — **Polarized light;**
**Image Credit: Pixabay**

When the longitudinal waves are made incident on the slits with the same position instead of transverse waves, the wave passes through both the slits without getting polarized.

Graph of a Transverse Wave and Longitudinal Wave

The graph of transverse wave can be plotted as a displacement of the wave due to the vibrational pattern of the particles v/s the distance traveled by the wave.

transverse wave vs longitudinal wave — **Graph of Displacement v/s Distance for Transverse Waves**

The graph shows the variations of the wave along with the distance. The transverse wave can also be plotted in a graph of displacement v/s time to show the displacement of the wave over time.

As the density of the waves varies in the longitudinal waves, the variations of the density due to the compression and rarefaction of the wave are observed by plotting a graph of density v/s distance as shown below for longitudinal waves.

The above graph shows the variations in the density of the waves due to compression and rarefaction of the wave along with the distance.

Frequently Asked Questions

Q1. A brass wire of length 100 cm is plucked applying a tension of the string of 250N. The mass of the string is 0.25 grams. Calculate the speed of the transverse wave generated on the wire.

Given: m=1.25 grams

T=250N

L=100cm=1m

The mass per unit length is m/l=1.25/1=1.25 grams

The speed of the transverse wave is given by the equation

v=√T/m

v=√(250N/1.25g)

v=200m/s

The speed of the transverse wave is 200 m/s.

What is the wavelength of the transverse wave?

A wave is made up of crest and the trough.

The wavelength of the transverse wave is the displacement of the particle to complete one oscillation. On a graph, it is the path length between crests or troughs.

What are compression and rarefaction?

The compression and rarefaction of a wave are formed due to the pressure difference experienced in the wave propagation.

This is due to the fact that the density of the waves in the compression region is more where the pressure felt is more as compared to the region of rarefaction.

If the propagation of the transverse wave is in the y-direction, then in which direction do the particles move?

The propagation of the wave makes 90 degrees angle with the path traced by the vibrating particles.

Hence, the movement of the particle has to be in the y-z plane, as both the planes are perpendicular to the x-directional axis.

Which waves are produced during a tsunami?

The longitudinal, as well as the transverse waves, are produced due to tsunami.

The transverse waves are generated in the oceanic floor due to the secondary waves produced from the oceanic floor. These transverse waves are then converted into longitudinal waves as they approach the seashore.

Why transverse waves can propagate only from solid mediums and not from gaseous mediums?

A transverse wave propagates through solids and on the surface of liquids.

A transverse wave is generated through the deformation of the solid as the solid has a shear modulus and therefore they undergo stress, liquid or gases do not possess this as they don’t have a definite shape.

Also Read:

Energy And Wavelength Relationship: Problems, Example And Detailed Facts

January 20, 2024February 4, 2022 by AKSHITA MAPARI

In this article, we are going to ponder upon the energy and wavelength relationship along with examples and solve some problems to illustrate the same.

Energy is directly dependent on the frequency of electromagnetic radiations. If the length of the wave increases, this implies that the recurrence of the wave will reduce that directly affecting the energy of the particle in a wave.

Energy and Wavelength Relationship Formula

The energy of the particle can be related to its speed during propagation. The speed of the particle gives the idea of the frequency and the length of the wave. If the wavelength is minute then the frequency and hence the energy of the particle will increase.

If the oscillations of the particle is more in a path trajectory, then the recurrence of the particle in a wave is more and wavelength is small, this implies that the energy possessed by the particle is more.

The energy of any body is related to its wavelength by the equation

E=hc/λ

Where ‘h’ is a Planck’s constant h=6.626 *10^-34\ J.s

C is a velocity of light c=3 *10⁸ m/s and

λ is a wavelength of the light

The energy is inversely proportional to the wavelength of the light. The lessened the wavelength, the more is the energy of the particle in a wave.

Problem 1: Calculate the energy of the photons emitting red light. Consider the wavelength of the beam of red light to be 698 nm. What will be the energy if the wavelength decreases to 500 nm that is if the source emits green light?

Given:λ₁=698nm

λ₂=500 nm

h=6.626 *10^-34 J.s

c=3 * 10⁸ m/s

We have,

E=hc/λ₁

E=6.626*10^-34 J.s* 3 *10⁸ m/s/698* 10^-9m

=0.028* 10^-17=28* 10^-20Joules

The energy of the red wavelength is 28* 10^-20Joules.

If the wavelength λ₂=500 nm

Then the energy associated with the green light is

E=hc/λ₂

E=6.626*10^-34 J.s* 3 *10⁸ m/s / 500* 10^-9m

=0.03910^-17=39* 10^-20Joules

We can see that, the energy has increased to 39*10^-20Joules as the wavelength is reduced.

Energy and Wavelength Relationship Graph

As the wavelength increases, the frequency of the wave falls off thus declining the energy possessed by the wave. If we plot a graph of Energy v/s Wavelength of the emerging particle, then the graph will look like as shown below

The above graph clearly indicated that, as the wavelength increases, the energy associated with the particle decreases exponentially.

Kinetic Energy and Wavelength Relationship

If the speed of the particle is greater, it is evident that the kinetic energy of the particle is high. The kinetic energy is given by the equation

K.E=1/2mv²

Where m is a mass of the object or particle

V is a velocity of the mass

We can write the above equation as

2E=mv²

Multiplying ‘m’ on both sides of the equation

2mE=(mv)²

The momentum of the object is given as the product of object mass and the velocity at which it is moving.

p=mv

Hence, the above equation becomes

P²=2mE

P=√2mE

According to De Broglie,

λ =h/p

Substituting the above equation, we have

λ =h/ √2mE

The above equation gives the relation between the energy and the wavelength of the particle.

Problem 2: Calculate the kinetic energy of a particle of mass 9.1 × 10^-31kg having a wavelength 293nm. Also, find the velocity of the particle.

Given: λ =293 nm

m = 9.1 × 10^-31kg

h=6.626 *10^-34J.s

c=3 *10⁸ m/s

We have,

λ =h/ √2mE

λ²=h²/ 2mE

E= h²/ 2mλ²

=(6.626 * 10^-34 J.s)²/2* 9.1* 10^-31* (293*10^-9) ²

=0.28*10^-23

The kinetic energy associated with the particle is 0.28* 10^-23 Joules.

Now, to calculate the velocity of the particle, let us derive a formula for velocity from the kinetic energy,

K.E=1/2 mv²

2E= mv²

v=√(2E/m)

= √(2(0.28*10^-23)/(9.8*10^-31))

=0.24*10⁴=2400m/s

The velocity of the particle having wavelength 298 nm is 2400 m/s.

Electron Energy and Wavelength Relationship

The energy of the electron is given by the simple equation as

E=h\nu

Where ‘h’ is a Planck’s constant and

nu is a frequency of occurrence of the electron

The frequency of the electron is given as

nu =v/λ

Where v is a velocity of the electron and

λ is a wavelength of the electron wave

Hence, the energy is related to the wavelength of the electron as

E=hv/λ

This is a relation to find the energy associated with the single electron propagating having a specific wavelength, speed, and frequency. The energy is inversely proportional to the wavelength. If the wavelength of the electron is lessened, the energy of the wave has to be greater.

physics gb0c0fbf26 640 — **Electromagnetic Waves;**
**Image Credit: Pixabay**

Upon receiving the energy in some form, the electron gets excited from the lower energy state to the higher energy state. For the transition of the electrons from one state to another, the energy of the electron is given by the equation

E=R_E(1/n_f– 1/n_i)

Where R_E=-2.18* 10^-18m^-1 is a Rydberg Constant

n_f is a final state of the electron

n_i is the initial state of the electron

We can further rewrite the above equation as

h\nu =R_E(1/n_f– 1/n_i)

hc/λ =R_E(1/n_f– 1/n_i)

1/λ =R_Ehc(1/n_f– 1/n_i)

1/λ =R(1/n_f– 1/n_i)

Where,

R=R_Ehc=1.097* 10⁷

As the electron gains the energy, the electron transit and jumps in the higher state of energy level and releases the energy to the electrons present in that state and either gets stable or releases the amount of energy and returns down to the lower energy states.

Problem 3: If the electron transit from state n_i=1 to state n_f=2, then calculate the wavelength of the electron.

Given:

n_i=1

n_f=2

1/λ =R_E(1/n_f– 1/n_i)

1/λ=-1.097*10⁷ * ( 1/2-1/1 )

1/λ=0.5485* 10⁷

Hence,

λ =1/0.5485* 10⁷

λ =1.823*10^-7

λ =182.3*10^-9=182.3nm

The wavelength of the light emitted during the transition of electron from one energy level to the other is 182.3 nm.

Radiant Energy and Wavelength Relationship

Every object absorbs light rays during daylight depending upon its shape, size, and its composition. If the temperature of the surface of the object reaches above the absolute zero temperature, the object will emit the radiations in the form of waves.

This emitted radiation is proportional to the fourth power of the absolute temperature of the object and is given by the equation

U=ɛΣ T^4A

Where U is a radiated energy

ɛ is the emissivity of radiation from the object

Σ is the Stefan-Boltzmann constant and is equal to Σ=5.67*10^-8W/m²K⁴

T is an absolute temperature

A is the area of the object

The object at high temperature emits radiation of short wavelengths, and the cooler surfaces emit waves of large wavelength. Based on the emission of radiation, and the wavelength of the emitted radiations, the waves are classified as given in the below chart.

Name	Radio Waves	Microwaves	Infrared	Visible	Ultra Violet	X-rays	Gamma rays
Wavelength	>1m	1mm-1m	700nm-1mm	400nm-700nm	10nm-380nm	0.01nm-10nm	<0.01nm
Frequency	<300MHz	300MHz-300GHz	300GHz-430THz	430THz-750THz	750THz-30PHz	30PHz-30EHz	>30Ehz

As the wavelength of the radiations decreases, the frequency of the wave escalates. Wavelength is directly related to the temperature, hence if the frequency of the emitted radiation is more this implies that the energy of the object is high.

The gamma rays, x-rays, and UV rays have a very short wavelength, hence the energy of these waves is very high compared to visible, infrared, microwaves, or radio waves. Also, the higher is the radiations received by the object, the more it will emit out depending upon the emissivity factor of the object.

Below is a graph of energy v/s wavelength plotted for different temperatures. The graph shows that, as the temperature of the system rises, the energy of the emitted radiations also increases with temperature.

**Graph of Energy v/s Wavelength for Emission of Radiations**

For, the wavelength in the visible region, the emission of radiation is the maximum. This is because the Sun emits UV rays along with infrared rays and visible rays and the rays are the electromagnetic waves for long range. The ozone layer of the Earth shields the earth’s atmosphere from this harmful radiation and gets either reflected back or trapped in the clouds.

More of the radiations are emitted in the visible region during day time as more and more radiations are received during day time from the Sun and fewer IR rays are given out compared to the visible spectrum. At night, the temperature goes down, the wavelength of the radiation increases, and more of the IR rays are emitted by the object.

Problem 4: A box length 11cm, width 2cm, and breath 7cm is heated at 1200 Kelvin temperature. If the emissivity of the box is 0.5, then calculate the rate of radiation of energy from the box.

Given:l=11cm

h=2cm

b=7cm

ɛ =0.5

Σ=5.67* 10^-8W/m²K⁴

T=1200 K

The total surface area of the box is

A=2(lb+bh+hl)

=2(11*7+7*s 2+2*11)

=2 (77+14+22)

=0.0226 sq.m

The energy radiated by the box is

U=ɛ Σ T^4A

=0.5* 5.67* 10^-8* 1200⁴* 0.0226

=1328.6 Watts

Energy Frequency and Wavelength Relationship

If the frequency of the wave is more, the energy associated with the particle is more. The energy is related to the frequency of the wave as

E=h/nu

Where ‘h’ is a Planck’s Constant

nu is a frequency of the wave

The frequency of the wave is defined as the speed of the wave in the medium and the wavelength of the wave.

nu =v/λ

Where v is a velocity of the wave

λ is a wavelength

Hence,

λ=v/nu

This gives the relation between the frequency and wavelength of the wave. It says that as the wavelength and frequency both are inversely correlated with each other. If the wavelength increases, the frequency f the wave will decrease.

Problem 5: The speed of a beam of light emitted from the source is 1.9 × 10⁸m/s. The frequency of occurrence of the emitted wave is 450THz. Find the wavelength of the emitted radiation.

Given: v=1.9*10⁸ m/s

F=450THz=450*10¹²Hz

The wavelength of the beam of light is

λ =v/f

=1.9* 10⁸/ 450* 10¹²

=0.004222*10^-4

=422.2* 10^-9=422.2nm

The beam of light is of wavelength 422.2 nm.

Energy of Photon and Wavelength Relationship

The energy possessed by a photon is termed as photon energy, and is inversely proportional to the electromagnetic wave of the photon, by the relation

E=hc/λ

Where ‘h’ is a Planck’s constant

C is a speed of light

λ is a wavelength of the photon

The frequency of the photon is given by the equation

f=c/λ

Where f is a frequency

Hence, the photon with a large wavelength possesses a small unit of energy whereas the photon with a smaller wavelength gives a large amount of energy.

Problem 6: Calculate the energy of the photon propagating in an electromagnetic wave having a wavelength of 620 nm.

Given: Wavelengthλ =620 nm

h=6.626 *10^-34 js

c=3 *10⁸ m/s

We have,

E=hc/λ

E=6.626 * 10^-34 J.s*3 * 10⁸m/s/620* 10^-9m

=0.032*10^-17=32*10^-20Joules

The energy associated with the photon is 32* 10^-20Joules.

Frequently Asked Questions

Q1. Calculate the wavelength of the electron traveling at a speed of 6.35 × 10⁶m/s

Given: v=6.35*10⁶m/s

m=9.1*10^-31kg

h=6.62* 10^-34J.s

The kinetic energy of the electron is

K.E=1/2 mv²

=1/2 * 9.1*10^-31* (6.35* 10⁶)²

=1.83* 10^-17Joules

The momentum of the electron is

P=√2mE

=√2* 9.1* 10^-31*1.83* 10^-17

=5.7*10^-24kg.m/s

Now, the wavelength of the electron is

λ =h/√2mE

=6.62*10^-34/5.7*10^-24

=4.8*10^-10m

=48nm

The wavelength of the electron moving with velocity 6.35*10⁶m/s is 48 nm.

Q2. A black object having a surface area of 180 sq.m is kept at a temperature of 550K. What is the rate of radiation of energy from the object?

Given: A=180 sq.m

T=550K

Since the object is black in color, the emissivity is 1.

ɛ =1

We have,

U=ɛΣT^4A

=1*s 5.67* 10^-8*550⁴*180

=0.93*10⁶Watts

The power radiated from the emission of radiation from the object is 0.93*10⁶Watts.

What is the absolute temperature of the system?

It is a non-variable and a perfect value of the temperature of a system.

The absolute temperature of the system is measured on the scale of degree Celsius, Fahrenheit, or Kelvin which measure zero as absolute zero degrees.

How does the wavelength of the photon be dependent on the temperature?

The temperature of the system specifies the agility of the particles of the system.

The more radiations received by the system at higher temperatures, the more emission will be given out from the system. At higher temperatures, shorter wavelength radiations are omitted and at lower temperatures, longer wavelengths are radiated.

Also Read:

11 Transverse Wave Example: Detailed Explanations

January 20, 2024February 1, 2022 by AKSHITA MAPARI

In this article, we are going to discuss various transverse wave examples with detailed information and facts.

The transverse wave is a short-range propagation that comes into existence due to the vibrational motion of the particles. The following is the list of the transverse wave examples:-

Ripples on water

A ripple produced on the water is an example of a transverse wave traveling on the layer of water.

The disturbance generated in the water produces transverse waves on the water in the form of ripples that vanishes to a certain extent. The water molecules move up and down from the place perpendicularly to the wave direction.

Shear waves due to earthquake

The geo-tectonic activities on the Earth give out shear waves, that travel through the solid surface of the Earth that is on the crust as it can’t penetrate through the asthenosphere because it is in liquid form.

The converging or diverging of the plates is responsible for the earthquakes. These propagating shear waves move the particles present in the crust or rocks up and down the motion perpendicular to the direction of propagation of the shear waves.

transverse wave example — **Ripples on water;**
**Image Credit: Pixabay**

Coherent Sources of Light

When a source emits light of constant wavelength and frequency, the source is said to be a coherent source of light. The light waves travel in the direction perpendicular to the direction of the particle, hence is a transverse wave example.

If the two transverse waves of light superimpose on each other then we get either constructive interference or destructive interference of light. The constructive interference is when the crest of waves falls on each other giving the bright fringes of light and when the crest of one wave falls on the trough of the other wave, that cancels out the amplitude of the superimposing waves giving dark fringes as the intensity of light at this phase is zero.

Pendulum

The pendulum in a simple harmonic motion oscillates continuously while the oscillations are maintained by the string it is attached to which is fixed at one point.

pulse gbf63b2516 640 — **Pendulum; Image Credit: Pixabay**

The oscillation of the pendulum is periodically at a mean position of a bob, and perpendicular to the direction of movement of a bob. Hence, this is also a type of transverse wave.

If you plot a graph of position v/s time of an oscillating pendulum considering the initial position of the bob at rest at the origin of a graph when time T=0, then you will find a sinusoidal wave on the x-axis with continuous decreases in the amplitude of the waves. This is because the energy of the pendulum dissipates due to the damped simple harmonic motion of the pendulum.

Sunlight

The vibration of a quantum of light is in a direction perpendicular to the wave. It is also called the electromagnetic wave because of its characteristics.

forest g70412d243 640 — **Sunlight; Image Credit: Pixabay**

The light wave is received on the crystal of the eye and the visible spectrum is visible to the human eyes.

Concentric waves on the surface of the water on tapping the stone

On throwing the stone in the water bodies, the circular wavy pattern is generated that travels for a certain distance apart and disappears.

drop ge5fa38c9a 640 — **A concentric pattern of waves;**
**Image Credit: Pixabay**

Due to the waves developed on the layer of water, the water moves up and down, the position of the particle remains the same but because of the transverse wave the water appears to be in motion, well, it is not so.

Electromagnetic Waves

The ray of light that shows both electric and magnetic characteristics is called electromagnetic light. Sunlight is also electromagnetic in nature. The direction of the electric field applied and the magnetic field produced both lie perpendicular to each other.

X-rays used to scan the inner parts of the body is also an example of electromagnetic wave. Since the field lines produced are perpendicular to the motion of particles and the electric field, this is also an example of transverse waves.

Oceanic Waves

The waves formed in the oceanic water are transverse waves. They come from the mid-ocean, approach the shore, and vanish.

aquatic gf119919c1 640 — **Oceanic Waves; Image Credit: Pixabay**

The water molecules on the surface of the water oscillate with the wave while the transverse wave travels perpendicular to the direction of the molecules.

The oceanic wave is associated with huge energy. The potential energy of the wave is transported to shore by converting it into kinetic energy and the wave returns back to the shore converting kinetic energy back to the potential energy.

Waves from on the string of wire

If you tie one end of the rope and wave a rope up and down position, we get a transverse wave. The wave started at one end will travel across the rope and will vanish at another end of the rope. Only the wave will propagate away from you while the particle on the rope will remain at the same locality.

Plucking a string on a guitar

We plug strings of the guitar to play the notes all the time. On plucking the string, the transverse waves are set onto the string due to which the string starts vibrating.

guitar gf3dd38335 640 — **Plucking string on guitar; Image Credit: Pixabay**

The sound produced by the guitar is a longitudinal wave that travels parallelly from the sound hole of the box guitar. The speed of the wave relies upon the tension applied to the string while plucking the note and the density of the string. It is given by the relation

v=√(T/mu)

Where v is a speed of a wave

T is a tension applied to the string

mu is a linear density of a string

Slinky

Slinky can be an example of both transverse and longitudinal waves. If you hold one end of the slinky and give it a wave by moving a hand up and down position, then the transverse wave will travel from one end to another and vanish.

colors g1bee92198 640 — **Slinky;**
**Image Credit: Pixabay**

If you suspend a slinky freely in the air, then you will notice that the wave is set into motion in a slinky that will move up and down the slinky moving coils. These are also transverse waves.

Tsunami

The ocean waves formed due to tsunami shows both types of waves.

wave gc7e7beb8a 640 — **Tsunami wave; Image Credit: Pixabay**

In the place where the volcano erupts on the oceanic floor, the transverse waves are seen in the ocean that gradually turns into a longitudinal as the wave approaches the shore.

What are transverse waves?

Transverse waves are called shear waves, or s-waves that travel through the crust of the Earth due to plate tectonic activities.

The vibrations produced in the particle give a form of waves due to oscillations of the particles up and down. These waves are called transverse waves. These are short-ranged waves and do not penetrate through the fluid mediums.

The transverse waves are stimulated by some disturbances and vibrations produced. This vibration sets the molecules in the object to oscillate up and down motion that creates the wavy pattern.

How to Calculate the Speed of the Transverse Wave?

A complete one oscillation of a particle in a time period ‘T’ is called the wavelength of that particle.

The ratio of wavelength and the time required for one wavelength gives the speed of the wave. Hence, the speed of the wave is measured as

v=λ/T

The frequency of the transverse wave is the total number of waves passing through in a unit interval of time.

f=1/T

Therefore the velocity of the transverse wave is the product of the frequency of the wave and its wavelength.

v=λf

Characteristics of a Transverse Wave

The propagation of a wave is in the direction perpendicular to the motion of the vibrating particle.
The time taken to complete one oscillation by the particle, that is a time required to travel on wavelength is defined as a time period of a transverse wave.
The speed of a transverse wave is equal to the product of its wavelength and the frequency of occurrence of a transverse wave in a unit of time.
The transverse wave is also called a shear wave as the vibrational motion produced by the particle may result in the deformation of the object.
The transverse wave does not travel through the liquid state, they travel only in the direction perpendicular to the motion of the particles in the fluid.
The transverse wave propagates at lower velocity and only in a solid and gaseous states.
The velocity of the transverse wave in the liquid is zero.
If the velocity of the transverse wave is more, then the energy associated with the transverse wave is more.

Frequently Asked Questions

What is the energy associated with the transverse wave?

If the number of oscillations due to vibrations of a particle is more in a given time period, then the energy of the particles is more.

The energy of a wave is directly dependent on the frequency of occurrence of waves in a unit of time. The total number of oscillations completed by the particle in a unit of time is the frequency of the particle.

Why transverse wave does not travel through fluids?

The transverse wave is generated due to the vibrations of the particle and travels perpendicular to it.

Transverse wave does not travel through fluids as no motion is driven in the direction perpendicular to the propagation of a wave.

Does the transverse wave get reflected?

A transverse wave is reflected when it is not able to transmit through the medium.

The reflected transverse wave will show the phase of π/2 from the incident wave, that is the crest of an incident wave will become a trough of a reflected wave.

On what factor does the speed of a transverse wave depend upon in the medium?

If the refractive index of the medium is more, then the speed of a wave will be very less through the medium.

The speed of the transverse wave depends upon the density of the medium of its propagation. It also relies upon the tension generated that results in the vibrational motion of a particle.

9 Causes Of Interference Of Light:Detailed Facts

January 21, 2024January 31, 2022 by AKSHITA MAPARI

In this article, we are going to discuss various causes of interference of light with detailed facts.

When two rays of light superimposed on each other disperse their energies to one another are called the interference of light. Lets us see the following causes of interference of light in detail:-

Waves of Light are in the Same Plane

Whether in phase or out of phase, the two waves will interfere if they propagate in the same plane.

If the waves are traveling in a different plane such that no two waves intersect or move parallel to each other, then there will be no chances of getting the interference pattern.

Superimposition of Waves

The two waves are said to be superimposed if the two waves running in the same plane overlap while propagating in the same direction.

If the crest and trough of one wave overlap exactly on the crest and trough of another wave respectively, then it enhances bright fringes and is called constructive interference. If the crest and trough of the first wave fall on the trough and crest of a second wave, then it will produce dark fringes. This type of interference that cancels out the crest and trough scraping the wave propagation is called destructive interference.

Coherent Sources of light

If the phase difference between the two waves emitted from different sources having similar frequency and wavelength remains the same, then the two sources are said to be coherent sources.

This is an essential condition that causes interference of light. It helps to produce stationary waves by keeping fixed phase differences. Light waves must have constant wavelength and phase, the frequency of the light sources must be equal or similar to each other.

causes of interference of light — **Candle Light;**
**Image Credit: Pixabay**

It would be inadequate to use the sources of two different frequencies to get the interference pattern, if so; we would have seen abrupt changes in the phase difference. Due to this, the intensity of the light will change unexpectedly and no interference pattern will be observed.

Hence, the sources must be coherent to get stationary waves having constant phase differences and to sustain the constant intensity of the light.

Wavelength of Light Equals the Dimensions of the Object

A light wave diffracting from the object at different angles interferes giving vibrant colors of light.

You must have observed the light reflected from bubbles, thin film of oil, oily surfaces, pools, etc. The light ray incident on the thin layer reflects a part of the light from the top surface of the thin film, and the remaining is refracted through and gets reflect from the bottom layer of the thin film while a part of the light may be transmitted.

These reflected rays of light, reflect at different angles because on refraction the angle of refraction in the medium of thin-film differs due to the refractive index. Hence, the rays of light bend at different reflected angles due to which the rays of light interfere. On interfering with the light rays colorful patterns of light are formed on the objects.

Energy of the Photons is Conserved

The conservation of energy by the photon of light is also an important factor for the interference of light.

If the energy of the wave is not conserved then the wave would have vanished after traveling to a certain distance and no interference pattern would be seen. The energy associated with the photon is given by the equation,

E=hθ

Where θ is a frequency of the light wave.

Since the energy is conserved, the above equation implies that the frequency of the light has to be conserved. If the light wave from the same source coincides with each other then we get the interference pattern of the light.

Narrow Sources of Light

If the sources of light are broad then the light rays emitting from different points would interfere among themselves would result in the overlapping of the interference of fringes and hinder the effect.

waves g0045b4e6e 640 — **Light transmitting from narrow slit;**
**Image Credit: Pixabay**

Hence, the monochromatic sources must be narrow.

Monochromatic Light

A monochromatic light source emits a light wave producing a unique wavelength and frequency. This will produce constructive interference of light.

If two different wavelengths of light are used then the destructive interference will occur at a certain point in between interference of waves giving dark fringes.

The Intensity of the two Monochromatic Lights is the Same

Monochromatic light means the source of the light that produces waves of light of constant wavelength. Two waves of similar frequencies and wavelengths interfere to give the interference pattern.

replacement lamp g4c94acde0 640 — **Sodium Light;**
**Image Credit: Pixabay**

Since the wavelength and frequency of the wave are constant, this implies that the amplitude of the wave that is directly proportional to the intensity of the light wave is constant.

The light waves interfering with each other are of the same amplitude hence the resultant fringes produced on the screen due to interference of the two waves are of equal intensities.

Distance from Source and Screen is Large

The width of the fringes formed due to interference is directly dependent on the distance between the source and the screen where the two beams of light show interference patterns. If the distance is large then the fringes are broadly spaced.

If the distance is shortened between the source and the screen, then we may not get that broad view of the interference pattern that we can get at a bigger distance. If we held the screen near few centimeters away from the source then it is evident that we shall not get any interference of light.

Distance between Coherent Sources is Small

The fringe width is inversely proportional to the distance of separation of two coherent sources of light. It is given by the equation

x=λD/d

Where x is a fringe width

D is a distance between source and screen

d is a distance between two sources

λ is a wavelength of a monochromatic light

If the distance between the two monochromatic lights is smaller then, they would easily interfere with one another. The fringes thus formed are broadly spaced are nicely visible.

Frequently Asked Questions

What is thin-film interference?

The wavelength of light penetrating through a thin film is equal to the dimension of the thin layer for the interference of light to occur.

The reflection of light from two layers of a thin film of solid or liquid interfere with each other to give a colorful pattern of light hence it is called thin-film interference.

How does interference of light take place?

For light waves to interfere, two or more waves of light has to be superimposed on each other.

When two monochromatic light waves of stable phase, and constant wavelength and the frequency overlap with each other, the interference of light takes place.

Define the term quantum interference?

The word quanta describe the discrete quantity of charged particle associated with mass and energy.

Quantum interference is the interference of the wave functions of the particle present in two different situations at a time in a wave.

What is constructive interference of light?

Two light waves of equal phase and equal frequency and amplitude interfere with each other; we get constructive interference of light.

The crest and trough of both the waves overlap on each other amplifying the effect such that the resultant wave produced gives the bright fringes as the amplitude of the wave increases.

What is destructive interference of light?

The phase difference between the two waves giving destructive interference is π/2.

The overlapping of waves having a phase difference cancels out with each other, giving no amplitude of the wave, resulting in zero intensity of light.

Also Read:

3 Types Of Interference Of Light:Detailed Facts

January 21, 2024January 31, 2022 by AKSHITA MAPARI

In this topic, we are going to discuss different types of interference of light that is observed in nature and will exhaustively talk about each type of interference with detailed facts.

Based on how the light rays interfere with each, we can classify the interference of light as follows:-

Constructive Interference

When two different waves interfere in such a way that, the crest of one way is superimposed on the crest of another wave then it is called constructive interference.

The below figure shows, how two waves superimpose to form a constructive interference pattern

types of interference of light — **Constructive interference of the waves**

The brightest fringes are formed due to constructive interference. The resultant wave derives the amplitude which is the resultant amplitudes of the two superimposed waves. Since both the crests of the wave overlap with each other, the resultant amplitude is greater than both the waves.

The crest of one wave falls exactly on the crest of another wave thus giving the phase difference zero, and hence are said to be in phase. Also, the displacement of the waves in a fixed time period is equal. Therefore the bright fringes are obtained on the screen due to crest to crest overlapping of the waves.

Destructive Interference

If the crest of one wave is imposed on the trough of another wave when two waves are superimposed on each other, then the interference of the two waves is called destructive interference.

The below figure clarifies how two waves superimpose to form a constructive interference pattern

The crest of wave 1 overlaps with a trough of the second wave. Hence, the phase difference of both the wave is 180 degrees, and hence the waves are said to be out of phase. The displacement of the wave is not unique in a given time interval.

The waves on interfering, vanish together, giving zero amplitude and no intensity. As there is no intensity of light given, the dark fringes are produced due to destructive interference.

Partial Interference

When two waves of the same wavelength and frequency are superimposed in such a way that the crest and trough of the wave don’t overlap on each other. This is the summing of both constructive and destructive interference.

The two waves imposing on each other are shown in the below diagrams.

The waves are partially imposed, hence called partially interfered. If the two sound waves show partial interference then the resultant sound wave produced will be heard partially muted frequently.

Partial interference is of two types, partial constructive interference, and partial destructive interference. The partial constructive interference is when the crest of two waves are not exactly superimposed on each other or the phase of each wave is not the same. And if the crest of one wave does not exactly superimpose on the trough of the second wave then it is called partial destructive interference.

Thin-Film Interference

When a ray of light reflects from two surfaces of the very thin layer of solid or liquid, the reflected light rays from the top and the bottom surfaces interfere and give colorful patterns of light.

soap bubbles gb916e727a 640 — **Soup Bubbles;**
**Image Credit: Pixabay**

During this type of interference, a part of the light is reflected and a part of the light is transmitted. Examples of thin-film interferences are light reflected from soap bubbles, the reflection of light from the pool, a thin film of oil on water, a thin layer of liquid on road, etc.

What is interference?

Two or more beams of light trespassing each other is called interference of light.

Two waves superimpose to produce a resulting wave having the same amplitude or either increase or decrease the amplitude of the emerging wave.

The interference of light gives the dark fringes which are called minima where the intensity of the light is zero and the bright fringes called the maxima where the intensity of the light rays is maximum.

How interference is different from Diffraction?

Interference is the overlapping of two or more waves whereas diffraction is the bending of light waves.

The fringes formed due to interference of light are equally spaced and not like the one observed in the diffraction pattern of light where the spacing between the fringes goes on decreasing from the center.

The intensity of the light is highest at the center if we see the diffraction pattern, but in the case of the interference pattern, the intensity of all the maxima has the same intensity. Dislike the diffraction, the fringes are equally spaces in the interference pattern. The minima observed in the diffraction of light are not perfectly dark whereas the dark fringes seen in the interference pattern are perfectly dark.

What is Quantum Interference?

From the word quantum, it is understood that it resembles the quanta of particles.

The addition of a wave function of a particle based on the probability of finding the particle at two different positions in a wave interfering among itself is called quantum interference.

It can be denoted by the equation below,

Ψ (x,t)=Ψ_A(x,t)+Ψ_B(x,t)

Where Ψ (x,t) is a linear superposition of two waves

Ψ_A(x,t) is a wave function of a particle in condition A

Ψ_B(x,t) is a wave function of a particle in condition B

The probability of finding the particle at certain position ‘x’ is the square of the wave function of the particle.

Hence,

P=Ψ(x,t)²=(Ψ_A(x,t)+Ψ_B(x,t) )²

P=(Ψ_A(x,t)²+Ψ_B(x,t)²+Ψ_A^(x,t)+Ψ_B(x,t)+Ψ_A(x,t)+Ψ_B^(x,t)

The terms Ψ_A(x,t)/Ψ_B(x,t) and Ψ_A(x,t)+Ψ_B^(x,t) represent the quantum interference. Whereas, the term Ψ_A²(x,t) gives the exact probability of a particle in condition A and the term Ψ_B²(x,t)gives the exact probability of a particle in condition B.

What is Resonance?

Resonance occurs when the frequency matches the natural frequency of the object.

The repetitive constructive interference results in resonance as the frequency of the wave matches the natural frequency of vibration of any object.

If you hammer a tuning fork and held it near the hollow vessel, the vibrations produced due to the tuning fork will travel across the vessel; and once the frequency of the vibrating waves matches the natural frequency of the hollow vessel, a resonating sound will be produced through a vessel.

What are Beats?

It is an interference of two sound waves of similar frequencies and a constant phase difference.

The addition of two waves having similar frequencies will overlap in a way making nodes and antinodes; the distance between two antinodes is called the beat.

The superimposition of the wave and the resultant wave produced will look like as shown below

This is used to tune any musical instrument. Suppose you want to tune string 1 on guitar, so lift a tuning fork ringing “E” note and ring a note while you plug your string 1 which is slightly out of tune, then both the notes will appear to be in synchronization initially and then will go out of tune.

Frequently Asked Questions

What is phase of a wave?

A phase of a wave is calculated on the axis of propagation of a wave.

A complete wavelength λ of one wave, that is the addition of sine and cosine function, gives a complete phase of 360 degrees. Half of the wavelength will give 180 degrees.

What is a phase difference between the two waves?

The phase difference is the degree to which one wave is lagging behind the phase of the other wave.

If the two waves overlap exactly on crest-to-crest of each other then the phase difference is zero. For partial constructive or partial destructive interference, the phase difference is greater than 0 and less than 90 degrees.

Also Read:

Tension and Compression Forces

Tension and Compression in Bridges

Compression and Tension Similarities

What is Better Tension or Compression?

Is Tension a Compressive Force?

Frequently Asked Questions

What is a tension in string tied to the object of mass 5kg accelerating at a speed of 3 m/s2?

What will happen if there was no tension in the bridge?

What is Negative Tension?

When Tension is Negative?

Case 1: When a body is accelerating down

Case 2: When a body is accelerating upward

Case 3: An object in a vertical axis with zero acceleration

Can Tension be Negative?

How to Find Negative Tension?

Problem: An object of mass 200 grams attached to a spring is compressed due to which the acceleration of the object is found to be 1m/s. Find the tension in the string.

Negative Tension Examples

Drowning of Bolt in the Water

Lantern Hanging on the Hook with String Suddenly Detach and Falls Down

Spring Shoes or Jumping Shoes

A Ladder on the Helicopter

Skipping

Loosening a Guitar strings

Balloon Floating in the Air

Frequently Asked Questions

Is stress a negative tension?

Why compression is a negative tension?

How to Find Tension between Two Blocks?

Problem: Consider two blocks of masses ‘m1’ and ‘m2’ attached to a rope and suspended freely in the air. Calculate the tension imposed on the rope due to two blocks.

Step1: Draw a free-body diagram for any problem

Step 2: Write the equation for net forces acting on each block.

Step 3: Frame the equation to find the net acceleration of the blocks.

Step 4: Calculate the total tension on the rope

Step 5: Find the net tension experiencing on the rope

Tension between Two Blocks on an Incline

Find Tension between Two Blocks on Horizontal Surface

Problem: Consider two-block kept on a frictionless plane having mass m1=3kg and m2= 5kg. Both these masses are tied with a string, and a mass of 5kg is pulled in the positive x-direction applying a force of 230N. Measure the tension exerted on the string.

Frequently Asked Questions

A body of mass 2kg is attached to the string and is accelerating downward at a rate of 3m/s. What is tension on the string?

What is a tension Force?

Relationship between Frequency and Energy of Wave

Problem 1: Find the energy of the particle having a frequency of 66PHz.

Graph of Energy and Frequency of Wave

Relationship between Frequency and Energy of Radiation

Problem 2: An object having a surface area of 28 sq.m is subjected to the temperature of 1120 Kelvin. The emissivity of the surface is 0.3. Calculate the rate of energy radiated from the object.

Relationship between Frequency and Energy of the Photon

Problem 3: The wavelength of the sodium light beam is 588 nm. Calculate the frequency of the photon emitted from the sodium beam. Also, calculate the energy of the emitted photons.

Relation between the Kinetic Energy and the Frequency

Example: Calculate the frequency of the electron possessing energy of 0.511 MeV. The transiting electrons are traveling with the wavelength of 530 nm.

Frequently Asked Questions

What is the frequency of the photon having the energy of 16 × 10-10 Joules?

What is the wavelength of the particle of mass 1.67 × 10-27 kg traveling at the speed of 2.5 × 108 m/s?

What is a speed of the particle having a wavelength 2.68 pm and energy of 0.45MeV?

How does the frequency affect the energy of the particle?

Does the speed of the wave vary with the energy?

How does the frequency affect the power of the radiations?

Does Wavelength Affect Refraction?

Example: A photon of energy 0.58 MeV is incident on the medium having refractive index 1.33. Find the wavelength of the incident photons.

How does Wavelength affect Refraction?

How does Wavelength affect Angle of Refraction?

Frequently Asked Questions

What is the angle of refraction if the light beam of a wavelength of 450nm is incident on the medium of refractive index 1.33 at an angle of 45 degrees?

How does the speed of the wave change on refraction?

Does the angle of refraction depend upon the speed of the light?

What is Transverse Wave?

What is a Longitudinal Wave?

Difference between frequency and wavelength of a transverse wave and longitudinal wave

What are seismic waves?

Direction of Propagation of Transverse Waves and Longitudinal Waves

Polarization of Transverse and Longitudinal Wave

Graph of a Transverse Wave and Longitudinal Wave

Frequently Asked Questions

Q1. A brass wire of length 100 cm is plucked applying a tension of the string of 250N. The mass of the string is 0.25 grams. Calculate the speed of the transverse wave generated on the wire.

What is the wavelength of the transverse wave?

What are compression and rarefaction?

If the propagation of the transverse wave is in the y-direction, then in which direction do the particles move?

Which waves are produced during a tsunami?

Why transverse waves can propagate only from solid mediums and not from gaseous mediums?

Energy and Wavelength Relationship Formula

Problem 1: Calculate the energy of the photons emitting red light. Consider the wavelength of the beam of red light to be 698 nm. What will be the energy if the wavelength decreases to 500 nm that is if the source emits green light?

What is a tension in string tied to the object of mass 5kg accelerating at a speed of 3 m/s²?

Problem: Consider two blocks of masses ‘m₁’ and ‘m₂’ attached to a rope and suspended freely in the air. Calculate the tension imposed on the rope due to two blocks.

Problem: Consider two-block kept on a frictionless plane having mass m₁=3kg and m₂= 5kg. Both these masses are tied with a string, and a mass of 5kg is pulled in the positive x-direction applying a force of 230N. Measure the tension exerted on the string.

What is the frequency of the photon having the energy of 16 × 10^-10 Joules?

What is the wavelength of the particle of mass 1.67 × 10^-27 kg traveling at the speed of 2.5 × 10⁸ m/s?

Problem 2: Calculate the kinetic energy of a particle of mass 9.1 × 10^-31kg having a wavelength 293nm. Also, find the velocity of the particle.

Problem 3: If the electron transit from state n_i=1 to state n_f=2, then calculate the wavelength of the electron.

Problem 5: The speed of a beam of light emitted from the source is 1.9 × 10⁸m/s. The frequency of occurrence of the emitted wave is 450THz. Find the wavelength of the emitted radiation.

Q1. Calculate the wavelength of the electron traveling at a speed of 6.35 × 10⁶m/s