Manish Naik - lambdageeks.com

Quick Guide On Net Force Formulas,Examples: Read This First!

October 2, 2024April 16, 2023 by Manish Naik

The example of net force describes an object’s acceleration when different forces act. The article discusses about the various example of the net force listed below:

Read more about Net Force Vs Force

Pushing Cupboard

It is challenging for one person to push the heavy cupboard forward. Suppose you and your friends A and B are together pushing the cupboard from the same direction. Force applied by you is 5N, and your friend A and B apply the force of 6N and 5N, respectively.

Adding all the forces 5 + 6 + 5 = 16N, displaying that the net force of 16N accelerates the heavy cupboard forward.

Different Forces acting in Same Direction — **Net Force includes Forces**
**in Same Direction**

Falling Ball

Suppose when we toss a ball in the sky, we apply the muscular force of 20N to it, which accelerates the ball upward with increasing velocity. When the air drag or air resistance force of -25N overcomes the muscular force, the ball reverses its direction and moves downward with different acceleration.

To determine the falling balls acceleration and direction, adding both forces, 20 + (-25) = -5N, displaying that the net force of -5N accelerates the ball downwards towards the ground.

Read more about Types of Forces

Stationary Rock

An object having mass stays at rest unless any applied force acts upon it. So when we are not pushing or pulling the stable rock, is there no force acting? Or is the net force on stationary rock zero?

Whether an object is in motion or at rest or moving in the air or the ground, one force always acts on it. i.e., gravity force. When any object moves or rests on the horizontal surface, the surface exerts the normal force upward on an object.

Suppose the rock is at rest on the ground or hill surface; the normal force of 15N acts upward on the rock opposite to the gravity force of -20N. Adding up all the pair of vertical forces 15 + (-20) = -5N, displaying the net force of -5N trying to accelerate the rock down on the ground.

Read more about Gravity Force.

Pushing Toy Car

Pushing is one type of applied force. Suppose a child drives the toy car on the horizontal floor by applying a push force of 10N and the floor surface also exerts the sliding friction force of -6N, which resists the car’s motion.

The gravity force of -5N acts downward on the toy car. The normal force of 5N exerted upward by the floor surface on the car is opposite to the gravity. Since the pair of vertical forces such as gravity and normal force acting on the toy have equal magnitude and opposite directions, both cancel each other.

Adding up a pair of horizontal forces to a car, such as applied and friction force, 10 + (-6) = 4N, displays that the net force of 4N accelerates the toy car forward.

Read more about Sliding Friction

Walking

We apply push force on the ground surface while walking or running. Suppose for push force of 8N; the ground surface exerts sliding friction force of -5N, which prevents us from slipping on the ground while walking.

There are vertical forces such as gravity force of -6N, and normal force of 5N always acts on us while walking. Adding up all the horizontal and vertical forces (8 + (-5) + 5 + (-6) = 3-1= 2N, displaying that the net force of 2N accelerates us forward when we walk.

Playing Golf

Suppose when you strike the golf ball with a golf club or stick, you apply the muscular force of 12N to it. The strike ball first drives in the air rapidly where an air resistance force of -6N lowers its motion. Once its velocity decreases, it falls on the ground.

Since the ball additionally slides across the ground after falling, it employs the rolling friction force of -2N parallel to the ball, which annihilates its rolling motion. A pair of vertical forces, such as gravity force of -2N and normal force of 2N, act on the golf ball, canceling each other.

**Net Force includes Four Forces**
(credit: shutterstock)

Hence, adding up all the remaining forces, 12 + (-6) + (-2) = 4N, displays that the net force of 4N accelerates the golf ball forward.

Read more about Rolling Friction.

Tug of War

The tug of war game is based on who will apply more force than others. Suppose team A applies a muscular force of 20 N on the rope, whereas team B is applying a muscular force of 15N.

The rope also exerts a tension force of -10N to both ends to prevent it from breaking.

Adding up all the force acting on the rope, [(20+ (-10)) + (15+(-10)] = 15N, displaying that net force 15N accelerating the rope towards the team A.

Read more about Tension Force.

Bungee Jumping

The attached cord during bungee jumping prevents us from casualty and delivers us an exhilarating experience as it bounces back.

Suppose you jump from a height. So the gravity force of -10N acts downward to you. Then just a certain distance before the ground, the rope pulls you upward by applying an elastic force of 8N. After pulling upward to a specific height, the air resistance force of -8N again takes you downwards along with the rope.

Adding up all these vertical forces, (-10) + 8 + (-8) = -10N, displays that the net force of -10N accelerates you downwards towards the ground.

Read more about Elastic Force.

Swimming

When you began swimming, have you wondered how many forces act on you, accelerating you forward in the water instead of drowning? Suppose we apply the muscular force of 10N as a thrust on the water to move forward.

The fluid layers of water exert the fluid friction force of -5N as a reaction force to resist our motion in water. But these two forces are not enough for us to swim. A pair of vertical forces also act on us while swimming. The gravity force of -10N acts downward, whereas fluid layers exert the upthrust or buyout force of 8N, because of which we can swim on or inside the water.

**Net Force while Swimming**
(credit: Biomechanics Tutorial)

Adding up all the forces, 10 + (-5) + 8 + (-10) = 5 + -2 = 3N, displaying that the net of 3N accelerates us forward during the swimming.

Airplane

Like swimming, four different forces act on the airplane from four different directions, accelerating it to move safely in the air. Suppose the airplane employs a thrust of 50N to fly forward in the air, whereas the air employs the air resistance force of -30N to the airplane.

A pair of vertical forces involves the gravity force of -40N downward and a lift force, a mechanical aerodynamic force of 35N exerted by the airplane’s motion through the air, which counterpart the gravity force.

**Net Force on the Airplane**
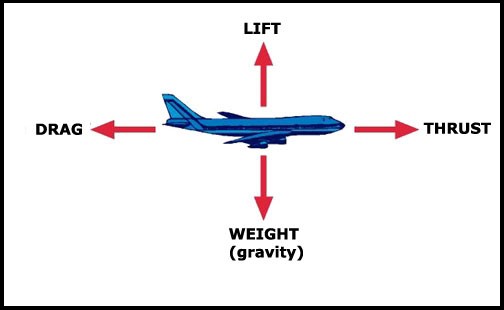
(credit: UScentennial)

Adding up all four forces, 50 + (-30) + 35 + (-40) = 20 -5 = 15N, displaying that the net of 15N accelerates the plane to move forward.

Spring

When we compress or stretch the spring from its equilibrium position, it regains its initial position once we release it. Suppose we apply a force of 20N on the spring by stretching it by the attached ball.

In reaction, the spring exerts the opposite force of -22N, restoring its original position. Adding up both action and reaction forces, 20 + (-22) = -2N, displaying that the net force of -2N accelerates the spring backward.

Long Jump

To complete the long jump activities, you demand several forces first to accelerate and then deaccelerate yourself. Before the jump, you need to run a specific distance to get momentum. Suppose you apply the muscular push force of 8N on the ground surface, whereas the ground exerts the sliding friction of -2N to you.

At the jump point, you again apply a more muscular force of 10N upward on the ground. After taking a jump, the air resistance force of -5N and the gravity force of -6N are exerted on you, accelerating you downward. After finishing the jump, you again apply a muscular push force of 5N to slide on the floor when you reach the ground. That is when the ground exerts more sliding friction 10N, which stops your motion gradually.

**Net Force during Long Jump**
(credit: shutterstock)

Modified Statement:

To calculate the total net force exerted during a complete long jump activity, we sum the forces of each phase of the jump: ([8 + (-2)] + [10 + (-5) + (-6)] + [5 + (-6)] = 6 – 1 – 1 = 4N). This calculation shows that a net force of 4N acts to accelerate us forward during the jump.

Carrying Bag

When you walk on the floor along with carrying your heavy bag on your back, the bag and your body experience different net forces.

Suppose you are applying a push force of 10N on the ground floor on which the friction force of -5N is exerted. Since you are walking on the floor, the exerted gravity force of -5N and the normal force of 5N cancel each other. Hence, adding up a pair of horizontal forces, 15 + (-5) = 10N, indicates that the net force of 10N accelerates you to move forward.

To carry the heavy bag, you balance the gravity force of -5N to the bag by applying a muscular force of 10N. Hence, the net force of 5N acts on the bag that accelerates it forward along with you.

When we talk about net force acting on you who is carrying the heavy bag, we add net force on us and the bag as, 10N + 5 = 15N. We learned that it needed a big net force to walk with bearing any weight than the net force needed to walk.

Charged Balloon

Suppose we charged the balloon and plastic stick by rubbing it with animal fur. If we tossed the charged balloon in the air and held the charged plastic stick beneath it, the balloon would not move away from the stick or hover at a certain distance.

Suppose the electric force of 10N between the balloon and stick counterpart to the balloon’s gravity force of -10N.

**Net Force includes Electrostatic Force**

Since the electric force and gravity force cancel each other, the net force on a charged balloon is zero. That’s because the balloon ties to the stick and does not drive away from it.

Rolling Car on Hill

Suppose the frictionless road is built on the inclined hill. So what will be the force acting on the car of mass 1 kg accelerating downward on such a hill road that is inclined at 30°? When an object moves on a horizontal surface, the object’s gravity force is mg. But when an object moves in the frictionless inclined plane, the gravity force is split into two components.

One gravity force component of mgcosθ¸ perpendicular to moving car cancels the normal force. Therefore, another gravity force component of mgsinθ¸ parallel to the car is the only net force (mgsinθ¸ = 1 x 9.8 x sing30° = 4.9N) that accelerates the car on the frictionless inclined road.

Read More about Inclined Plane

Running Train

It is difficult for the train driver to find different forces acting on the train. But they must comprehend the trainâ€™s mass and acceleration rate.

Suppose the train of mass 500 kg is running at 20 m/s, then as per Newton’s second law of motion, the net force acting on the running train F_net = ma = 500 x 20 = 1000 N.

Also Read:

9 Example of Potential Energy to Chemical Energy: Detailed Explanations

January 21, 2024March 9, 2023 by Manish Naik

The conversion from potential to chemical energy depicts how stationery molecules form chemical bonds. The article discusses such an example of potential energy to chemical energy, which is listed below:

Battery

It operates on the electrochemical principle where energy is converted from electric to chemical and vice versa. The battery holds the electric potential energy in a chemical state within its stationary particles during charging. In contrast, the chemical reaction delivers electric energy during the discharging.

That means chemical energy has both forms. i.e., potential energy and kinetic energy. When the energy is held in the chemical bonds of stationary particles, it is chemical potential energy.

The energy is released after particle breaking bonds when it reacts with other particles; it is transformed into chemical kinetic energy, which causes work through particle movement.

**Example of Potential Energy to Chemical Energy**

Electrolyte Cell

It encloses cathode and anode as electrodes dissolved by a chemical solution termed electrolyte. On incoming electrical energy, an electrolysis process decays the chemical compounds at electrodes which induces a non-spontaneous chemical redox reaction, delivering the chemical energy in the cell.

The conversion from electrical to chemical energy occurs due to oxidation and reduction of chemical reactions at both electrodes. Unlike surfaces, a chemical reaction happens between the two electrodes. That’s why the unlike substances have chemical energy because of the electrostatic attraction between their chemical bonds.

Chemical Energy Example of Electrolyte Cell — **Chemical Energy in Electrolyte Cell** (credit: shutterstock)

Coal and Wood

The combustion process breaks the bonds between molecules that store the chemical potential energy within the surface. The broken bonds release the energy so that stationary particles react to surrounding air molecules to construct another chemical element.

When heat energy is provided to the coal or wood that contains Methane CH₄, a natural gas, it reacts to oxygen O₂, discharging chemical energy to create light and heat energy as a fire; and then assembling new element as carbon dioxide CO₂, water H₂O and ashes ΔH.

Like coal or wood, each chemical compound has potential energy held into its chemical bonds. When any contact or non-contact force functions on such compounds, it damages their chemical bonds. The chemical energy is radiated in heat energy, i.e., either exothermic or endothermic.

Candle Wax

It is a Paraffin Wax C₂₅H₅₂ that contains the chemical potential energy. When we ignite the candle, it supplies heat energy to wax from its wick. The paraffin wax melted to expose to the air molecule O₂ and create carbon dioxide CO₂ and water molecule H₂0.

The reactions due to burning provide heat energy and light energy through a wick.

Read about Physical Change

Air Bag

Have you known the airbag that protects the driver during an accident is activated when chemical reactions happen within the bag? When a car unexpectedly deaccelerated, the sensor in the system turned on the electrical panel, which ignited the Sodium Azide NaN₃ inside the bag.

The chemical reaction yields the nitrogen gas, filling the bag at a highly rapid rate.

Chemical Energy of Air Bag — **Chemical Energy in Air Bag**
(credit: shutterstock)

Petroleum

It comprises both crude oil and natural gas with molecules of hydrogen and carbon that keep the potential energy chemically into any vehicle’s tank. The combustion of petroleum or gasoline creates chemical energy, composing different chemical elements.

The newly formed Isooctane C₈H₁₈ and oxygen O₂ are subjected to the engine, burning steadily to do the tasks.

Some of its transformed chemical kinetic energy enables the vehicle to move on the road.
Some of the potential chemical energy is transformed into heat energy as the vehicle’s engine becomes hot.

Chemical Energy of Petroleum — **Chemical Energy in Fuels**
(credit: shutterstock)

Dynamite or Explosives

It contains Nitro-glycerine C₃H₅N₃O₉, a various unstable chemical element that shows it has the potential energy to explode. It is exposed to the air O₂ molecule when the dynamite ignites The reactions provoke the dynamite to blast with the release of immense amounts of nitrogen and tremendous heat and sound energy.

Chemical Energy Example of Explosion — **Chemical Energy in Dynamite**
(credit : shutterstock)

Firecrackers also operate on an equivalent principle to convert potential energy to chemical energy.

Food

Eating food provides energy to the body. That indicates that the food contains its energy, energizing the human body. When we consume it, our body organ combines the food with enzymes and acids in the stomach.

The chemical reaction remakes the potential energy in carbohydrates into Glucose C₆H₁₂O₆, which is then discharged into the bloodstream, energizing our body.

Chemical Energy Example of food — **Chemical Energy in Food**
(credit: shutterstock)

Cellular Respiration

It utilizes the energy to survive, reproduce, react to stimuli, and drive biological processes. Within the human body, the atoms and molecules were structured together into chemical bonds to hold the chemical potential energy in the form of glucose.

The stored chemical energy into molecules is transformed into chemical kinetic energy, allowing the human body to move and do the work. The process of spreading the energy into your body cell is called ‘Cellular Respiration’.

Chemical Energy Example of Cellular Respiration — **Chemical Energy in Cellular Respiration**
(credit: shutterstock)

Photosynthesis

When the sun lights on the plants, it changes solar energy to chemical energy. The process is called ‘photosynthesis’ which involves the chemical reaction between carbon dioxide and water molecules in solar energy to create glucose and oxygen.

That’s why the plant soaks carbon dioxide and emits oxygen during photosynthesis. In comparison, photosynthesis discharges more chemical energy than the energy needed to start the chemical reactions.

Biomass

Using chemical agents, one can convert the biomass materials into biodiesel as a liquid fuel. It involves the chemical reaction between glucose and the air molecule O2. The chemical reaction transforms the stored potential energy into heat energy by comprising carbon dioxide and a water molecule.

Also Read:

The Intricate Relationship between Dew Point and Altitude

June 25, 2024April 20, 2022 by Manish Naik

Dew point and altitude are intricately linked through the concept of density altitude, which is a crucial parameter in aviation and meteorology. Density altitude is a measure of air density that takes into account temperature and pressure variations with altitude, and it can be significantly affected by the dew point, which is a measure of the amount of moisture in the air.

Understanding Density Altitude

Density altitude is the altitude at which the air density is equal to the air density at a given location, temperature, and pressure. It is calculated by taking the pressure altitude and correcting it for non-standard temperature and humidity conditions. The formula for calculating density altitude is:

Density Altitude = Pressure Altitude + [(288 – 0.00198 × Temperature in °C) / 288] × 1000

This formula shows that density altitude is primarily a function of temperature and pressure, but it can also be influenced by humidity, as represented by the dew point.

The Effect of Dew Point on Density Altitude

According to a study by Guinn and Barry (2016), density altitude is a function only of dew-point temperature for a given pressure altitude. They found that the absolute errors between density altitude calculations that incorporate humidity and those that do not are significant, and they created a simple rule of thumb for diagnosing the impact of humidity on density altitude.

The rule of thumb is that the correction (in feet) is simply twenty times the dew-point temperature in Celsius, or colloquially, “double the dew point and add a zero.” This rule of thumb is shown to limit the percent error in density-altitude calculations to within 5% for the range of dew-point temperatures between 5°C to 30°C and elevations below 6,000 feet, compared to over 20% for the same conditions using the dry case alone.

For example, if the dew point is 15°C, the correction to the density altitude would be:

Dew point correction = 20 × 15°C = 300 feet

This correction would then be added to the density altitude calculated using the standard formula.

The Effect of Altitude on Dew Point

The relationship between dew point and altitude is not a simple one, as it can be influenced by various factors, such as temperature, pressure, and humidity. In general, as altitude increases, the dew point tends to decrease due to the lower air pressure and temperature.

However, the rate of decrease in dew point with altitude can vary depending on the specific atmospheric conditions. For example, in humid environments, the dew point may not decrease as rapidly with altitude as in drier environments.

According to a study by the National Oceanic and Atmospheric Administration (NOAA), the dew point at the summit of Mount Washington (elevation 6,288 feet) was found to be consistently lower than the dew point at the base of the mountain, with an average difference of around 5°C.

Practical Applications of Dew Point and Altitude

The relationship between dew point and altitude has important practical applications, particularly in the field of aviation. Pilots need to be aware of the effects of humidity on density altitude, as it can significantly impact aircraft performance and safety.

For example, if an aircraft is taking off from a high-altitude airport with a high dew point, the density altitude may be much higher than the actual altitude, leading to reduced engine power and lift. This can make it more difficult for the aircraft to take off and climb, potentially increasing the risk of accidents.

Similarly, in the case of landing, a high density altitude due to a high dew point can make it more challenging for the aircraft to slow down and stop, as the reduced air density can affect the effectiveness of the brakes and other systems.

Numerical Examples

To illustrate the impact of dew point on density altitude, let’s consider a few numerical examples:

Scenario 1: Pressure Altitude = 5,000 feet, Temperature = 20°C, Dew Point = 10°C
Density Altitude (without dew point correction) = 5,000 feet
Dew point correction = 20 × 10°C = 200 feet
Corrected Density Altitude = 5,000 feet + 200 feet = 5,200 feet
Scenario 2: Pressure Altitude = 8,000 feet, Temperature = 15°C, Dew Point = 5°C
Density Altitude (without dew point correction) = 8,000 feet
Dew point correction = 20 × 5°C = 100 feet
Corrected Density Altitude = 8,000 feet + 100 feet = 8,100 feet
Scenario 3: Pressure Altitude = 3,000 feet, Temperature = 25°C, Dew Point = 20°C
Density Altitude (without dew point correction) = 3,000 feet
Dew point correction = 20 × 20°C = 400 feet
Corrected Density Altitude = 3,000 feet + 400 feet = 3,400 feet

These examples demonstrate how the dew point can significantly impact the density altitude, and how the simple rule of thumb can be used to quickly estimate the correction.

Conclusion

In summary, dew point and altitude are closely related through the concept of density altitude, which is a crucial parameter in aviation and meteorology. The dew point can significantly affect the density altitude, and the simple rule of thumb of “double the dew point and add a zero” can be used to quickly estimate the correction. Understanding the relationship between dew point and altitude is essential for pilots, meteorologists, and anyone working in fields where air density and its effects are important.

References

Guinn, T. A., & Barry, R. J. (2016). Quantifying the Effects of Humidity on Density Altitude Calculations for Professional Aviation Education. International Journal of Aviation, Aeronautics, and Aerospace, 3(3), 1-10. doi:10.15394/ijaaa.2016.1124
Vaisala. (2019). What is dew point and how to measure it? Retrieved from https://www.vaisala.com/en/blog/2019-09/what-dew-point-and-how-measure-it
NOAA. (2007). Dewpoint and Humidity Measurements and Trends at the Summit of Mount Washington. Retrieved from https://journals.ametsoc.org/view/journals/clim/20/22/2007jcli1604.1.xml
Aviation Stack Exchange. (2017). Does the dew point affect density altitude? Retrieved from https://aviation.stackexchange.com/questions/45095/does-the-dew-point-affect-density-altitude
Embry-Riddle Aeronautical University. (2016). Quantifying the Effects of Humidity on Density Altitude Calculations. Retrieved from https://commons.erau.edu/ijaaa/vol3/iss3/2/

Relative Velocity and the Speed of Light: A Comprehensive Guide

June 25, 2024April 15, 2022 by Manish Naik

The speed of light in a vacuum is a fundamental constant in physics, with a value of approximately 299,792 kilometers per second. According to the theory of special relativity, this speed is the same for all observers, regardless of their relative motion or the motion of the light source. Understanding the relationship between relative velocity and the speed of light is crucial for comprehending various phenomena in the realm of modern physics.

Reference Frames and Relative Velocity

When measuring the relative velocity between two objects, it is essential to specify the reference frame. A reference frame is a point of view from which events are observed. For example, if a train is moving at 40 m/s relative to the train track, the same train is stationary relative to a passenger on the train.

The relative velocity between two objects is the difference in their velocities as measured from a specific reference frame. This relative velocity can be calculated using the formula:

$v_{rel} = v_2 – v_1$

where $v_{rel}$ is the relative velocity, $v_2$ is the velocity of the second object, and $v_1$ is the velocity of the first object.

The Speed of Light Limit

In the context of the speed of light, the relative velocity between two objects cannot exceed the speed of light. This is a fundamental principle of special relativity, known as the speed of light limit. However, it is possible for the relative velocity to appear to exceed the speed of light from a particular observer’s perspective. This apparent violation of the speed of light limit is a result of two key concepts in special relativity: time dilation and length contraction.

Time Dilation

Time dilation refers to the phenomenon where a moving observer’s clock will appear to tick slower than a stationary observer’s clock. This effect is described by the following equation:

$t’ = \gamma t$

where $t’$ is the time measured by the moving observer, $t$ is the time measured by the stationary observer, and $\gamma = \frac{1}{\sqrt{1 – \frac{v^2}{c^2}}}$ is the Lorentz factor.

Length Contraction

Length contraction, on the other hand, means that a moving object will appear shorter in the direction of motion to a stationary observer. The equation for length contraction is:

$L’ = \frac{L}{\gamma}$

where $L’$ is the length measured by the moving observer, $L$ is the length measured by the stationary observer, and $\gamma$ is the Lorentz factor.

Apparent Violation of the Speed of Light Limit

When combining the effects of time dilation and length contraction, the relative velocity between two objects can appear to exceed the speed of light from a particular observer’s perspective. This is known as the “apparent” violation of the speed of light limit.

For example, consider two objects moving towards each other at 55% the speed of light. From the perspective of a stationary observer, the closing velocity between the two objects would be 110% of the speed of light. However, this does not actually violate the speed of light limit, as it is a result of the way that time and space are perceived by the moving observers.

Numerical Problems

Spaceship Traveling Away from Earth
A spaceship is traveling away from Earth at 99% the speed of light.
An astronaut on the spaceship sends a signal back to Earth.
Question: How much time will pass on Earth between the moment the signal is sent and the moment it is received?

To solve this problem, we can use the time dilation equation:
$t’ = \gamma t$
where $t’$ is the time measured by the moving observer (the astronaut on the spaceship), $t$ is the time measured by the stationary observer (on Earth), and $\gamma = \frac{1}{\sqrt{1 – \frac{v^2}{c^2}}}$ is the Lorentz factor.

Given:
– Velocity of the spaceship: $v = 0.99c$
– Speed of light: $c = 299,792 \text{ km/s}$

Calculating the Lorentz factor:
$\gamma = \frac{1}{\sqrt{1 – \frac{(0.99c)^2}{c^2}}} = \frac{1}{\sqrt{1 – 0.9801}} = 7.089$

Therefore, the time that passes on Earth between the moment the signal is sent and the moment it is received is:
$t = \frac{t’}{7.089}$

This means that for every 7.089 seconds that pass on the spaceship, only 1 second will pass on Earth.

Figures, Data Points, and Measurements

Speed of light in a vacuum: approximately 299,792 kilometers per second
Time dilation: affects the perception of time for moving observers
Length contraction: affects the perception of length for moving observers

Reference Links

This comprehensive guide on relative velocity and the speed of light covers the fundamental concepts, technical specifications, physics formulas, examples, and numerical problems. By understanding the principles of reference frames, time dilation, and length contraction, you can gain a deeper appreciation for the complex relationship between these two essential aspects of modern physics.

Torque and Moment of Inertia: A Comprehensive Guide for Physics Students

June 25, 2024April 9, 2022 by Manish Naik

Torque and moment of inertia are fundamental concepts in the study of rotational motion, which is a crucial aspect of classical mechanics. Understanding these two quantities and their relationship is essential for solving a wide range of physics problems, from the motion of celestial bodies to the design of machinery and engineering systems.

Understanding Torque

Torque is a measure of the force that can cause an object to rotate about an axis. It is calculated as the cross product of the force and the distance from the axis to the point of application of the force. The unit of torque is the newton-meter (Nm).

The formula for calculating torque is:

τ = r × F

where:
– τ is the torque
– r is the distance from the axis of rotation to the point of application of the force
– F is the applied force

Torque can be thought of as the “twisting” or “turning” effect of a force on an object. The magnitude of the torque depends on the magnitude of the force, the distance from the axis of rotation, and the angle between the force and the line connecting the axis to the point of application.

Theorem: Torque and Angular Acceleration

The relationship between torque and angular acceleration is given by the equation:

τ = Iα

where:
– τ is the torque
– I is the moment of inertia
– α is the angular acceleration

This equation is known as the rotational analog of Newton’s second law of motion. It states that the torque acting on an object is equal to the product of its moment of inertia and its angular acceleration.

Examples of Torque Calculations

Rotating a door: Consider a door with a mass of 10 kg and a length of 2 meters. If a force of 20 N is applied at a distance of 1 meter from the hinge, the torque acting on the door is:

τ = r × F = (1 m) × (20 N) = 20 Nm

Tightening a nut with a wrench: Suppose you are using a wrench with a length of 0.5 meters to tighten a nut. If you apply a force of 50 N perpendicular to the wrench, the torque applied to the nut is:

τ = r × F = (0.5 m) × (50 N) = 25 Nm

Rotating a pulley: A pulley with a radius of 0.2 meters is connected to a weight of 5 kg. If the weight is pulled with a force of 10 N, the torque acting on the pulley is:

τ = r × F = (0.2 m) × (10 N) = 2 Nm

These examples illustrate how the magnitude and direction of the applied force, as well as the distance from the axis of rotation, determine the torque acting on an object.

Understanding Moment of Inertia

Moment of inertia, on the other hand, is a measure of an object’s resistance to rotational motion. It is calculated as the sum of the products of the mass of each particle in the object and the square of its distance from the axis of rotation. The unit of moment of inertia is kilogram-meter squared (kgm^2).

The formula for calculating the moment of inertia of a system of particles is:

I = Σ m_i r_i^2

where:
– I is the moment of inertia
– m_i is the mass of the i-th particle
– r_i is the distance of the i-th particle from the axis of rotation

Theorem: Moment of Inertia for Common Shapes

The moment of inertia for some common geometric shapes can be calculated using the following formulas:

Solid cylinder about its central axis:
I = (1/2) m r^2
Solid sphere about its central axis:
I = (2/5) m r^2
Solid rod about an axis perpendicular to the rod and passing through its center:
I = (1/12) m L^2
Solid rectangular plate about an axis perpendicular to the plate and passing through its center:
I = (1/12) m (L^2 + W^2)

where:
– m is the mass of the object
– r is the radius of the cylinder or sphere
– L is the length of the rod or plate
– W is the width of the rectangular plate

These formulas provide a convenient way to calculate the moment of inertia for common geometric shapes, which can be useful in a variety of physics problems.

Examples of Moment of Inertia Calculations

Rotating a solid cylinder: Consider a solid cylinder with a mass of 5 kg and a radius of 0.3 meters. The moment of inertia of the cylinder about its central axis is:

I = (1/2) m r^2 = (1/2) × (5 kg) × (0.3 m)^2 = 0.225 kgm^2

Rotating a solid sphere: Suppose you have a solid sphere with a mass of 2 kg and a radius of 0.1 meters. The moment of inertia of the sphere about its central axis is:

I = (2/5) m r^2 = (2/5) × (2 kg) × (0.1 m)^2 = 0.004 kgm^2

Rotating a solid rod: Consider a solid rod with a mass of 1 kg and a length of 0.5 meters. The moment of inertia of the rod about an axis perpendicular to the rod and passing through its center is:

I = (1/12) m L^2 = (1/12) × (1 kg) × (0.5 m)^2 = 0.0208 kgm^2

These examples demonstrate how the moment of inertia of an object depends on its mass and the distribution of that mass relative to the axis of rotation.

Relationship between Torque and Moment of Inertia

The relationship between torque and moment of inertia is given by the equation:

τ = Iα

where:
– τ is the torque
– I is the moment of inertia
– α is the angular acceleration

This equation is the rotational analog of Newton’s second law of motion and is a fundamental principle in the study of rotational dynamics.

Physics Formulas Involving Torque and Moment of Inertia

Rotational Kinetic Energy:
Rotational kinetic energy = (1/2) I ω^2
where ω is the angular velocity of the object.
Torque Required for Acceleration:
Torque required to accelerate an object from rest to an angular velocity ω in time t:
τ = (1/2) I ω^2 / t
Torque Required for Deceleration:
Torque required to stop an object rotating at an angular velocity ω in time t:
τ = (1/2) I ω^2 / t
Moment of Inertia of a System of Particles:
I = Σ m_i r_i^2
where m_i is the mass of the i-th particle and r_i is its distance from the axis of rotation.

These formulas demonstrate the important role that both torque and moment of inertia play in the analysis of rotational motion and the design of mechanical systems.

Numerical Problems and Exercises

Rotating a Flywheel: A flywheel with a moment of inertia of 0.5 kgm^2 is initially at rest. If a constant torque of 10 Nm is applied to the flywheel, calculate:
a. The angular acceleration of the flywheel.
b. The time it takes for the flywheel to reach an angular velocity of 50 rad/s.
Stopping a Rotating Wheel: A wheel with a moment of inertia of 0.2 kgm^2 is rotating at an angular velocity of 30 rad/s. If a constant torque of 5 Nm is applied to stop the wheel, calculate:
a. The time it takes for the wheel to come to a complete stop.
b. The angular displacement of the wheel during the stopping process.
Lifting a Load with a Pulley: A pulley with a radius of 0.1 meters and a moment of inertia of 0.01 kgm^2 is used to lift a load of 20 kg. If a force of 50 N is applied to the pulley, calculate:
a. The torque acting on the pulley.
b. The angular acceleration of the pulley.
c. The time it takes for the load to be lifted 2 meters.
Rotating a Solid Cylinder: A solid cylinder with a mass of 10 kg and a radius of 0.2 meters is rotating about its central axis. If the cylinder is subjected to a constant torque of 5 Nm, calculate:
a. The moment of inertia of the cylinder.
b. The angular acceleration of the cylinder.
c. The time it takes for the cylinder to reach an angular velocity of 20 rad/s.
Moment of Inertia of a Compound Object: A system consists of a solid cylinder with a mass of 5 kg and a radius of 0.1 meters, and a solid sphere with a mass of 3 kg and a radius of 0.05 meters. The objects are connected by a massless rod of length 0.2 meters. Calculate the moment of inertia of the system about an axis passing through the center of the cylinder and perpendicular to the rod.

These problems cover a range of applications of torque and moment of inertia in rotational motion, allowing you to practice and reinforce your understanding of these important concepts.

Conclusion

Torque and moment of inertia are fundamental quantities in the study of rotational motion, and a deep understanding of these concepts is essential for solving a wide range of physics problems. By mastering the formulas, theorems, and examples presented in this guide, you will be well-equipped to tackle complex problems involving the dynamics of rotating systems.

References

OpenStax, University Physics Volume 1. https://openstax.org/books/university-physics-volume-1/pages/10-4-moment-of-inertia-and-rotational-kinetic-energy
Raptor Scientific, Why Is Moment of Inertia Important? https://raptor-scientific.com/news/why-moi-is-important/
Hoffner Physics, Moment of Inertia Lab. http://hoffnerphysics.weebly.com/moment-of-inertia-lab.html
Serway, R. A., & Jewett, J. W. (2018). Physics for Scientists and Engineers with Modern Physics (10th ed.). Cengage Learning.
Halliday, D., Resnick, R., & Walker, J. (2013). Fundamentals of Physics (10th ed.). Wiley.

Torque and Angular Momentum: A Comprehensive Guide for Physics Students

June 25, 2024March 31, 2022 by Manish Naik

Torque and angular momentum are fundamental concepts in classical mechanics that describe the rotational motion of objects around an axis. Torque is a measure of the force that can cause an object to rotate, while angular momentum is a measure of the amount of rotation an object has. Understanding these concepts is crucial for analyzing the dynamics of various systems, from simple rigid bodies to complex celestial bodies.

Torque: The Rotational Equivalent of Force

Torque is a vector quantity that represents the rotational force acting on an object. It is defined as the product of the magnitude of the force and the perpendicular distance between the axis of rotation and the line of action of the force. Mathematically, torque can be expressed as:

τ = r × F

where:
– τ is the torque (in Newton-meters, N·m)
– r is the position vector from the axis of rotation to the point of force application (in meters, m)
– F is the force vector (in Newtons, N)
– × represents the cross product operation

The direction of the torque vector is determined by the right-hand rule, which states that if the fingers of the right hand are curled in the direction of rotation, the thumb will point in the direction of the torque vector.

Factors Affecting Torque

The magnitude of the torque depends on the following factors:

Force Magnitude: The greater the force applied, the greater the torque.
Distance from Axis: The greater the distance from the axis of rotation to the line of action of the force, the greater the torque.
Angle between Force and Distance: The torque is maximized when the force is perpendicular to the distance vector (i.e., the angle between them is 90 degrees).

These factors can be used to calculate the torque using the formula:

τ = r × F = r · F · sin(θ)

where θ is the angle between the force vector and the distance vector.

Torque Examples and Applications

Opening a Jar Lid: When you apply a force to the lid of a jar, you create a torque that causes the lid to rotate and open.
Tightening a Nut with a Wrench: The longer the wrench, the greater the torque applied to the nut, making it easier to tighten.
Balancing a Seesaw: The torque created by the weight of a person on one side of the seesaw must be balanced by the torque created by the weight of another person on the other side.
Rotating a Door: The torque created by pushing or pulling on a door handle causes the door to rotate around its hinges.

Angular Momentum: The Rotational Equivalent of Linear Momentum

Angular momentum is a vector quantity that describes the rotational motion of an object. It is defined as the product of the object’s moment of inertia and its angular velocity. Mathematically, angular momentum can be expressed as:

L = Iω

where:
– L is the angular momentum (in kilogram-square meters per second, kg·m²/s)
– I is the moment of inertia (in kilogram-square meters, kg·m²)
– ω is the angular velocity (in radians per second, rad/s)

The moment of inertia is a measure of an object’s resistance to rotational motion and depends on the distribution of the object’s mass around the axis of rotation. For a point mass, the moment of inertia is simply the product of the mass and the square of the distance from the axis of rotation. For more complex objects, the moment of inertia can be calculated using integration or measured experimentally.

Factors Affecting Angular Momentum

The angular momentum of an object depends on the following factors:

Mass Distribution: The more the mass is distributed away from the axis of rotation, the greater the moment of inertia and the angular momentum.
Angular Velocity: The greater the angular velocity, the greater the angular momentum.

These factors can be used to calculate the angular momentum using the formula:

L = Iω

Angular Momentum Examples and Applications

Spinning Top: A spinning top has a high angular momentum, which allows it to remain upright and stable.
Gyroscope: Gyroscopes use the conservation of angular momentum to maintain a stable orientation, which is useful in navigation and stabilization systems.
Satellite Stabilization: Satellites use onboard gyroscopes to maintain their orientation and stability in space, where there are no external forces to keep them stable.
Skating and Gymnastics: Skaters and gymnasts use their angular momentum to perform complex rotations and twists, taking advantage of the conservation of angular momentum.

Advanced Concepts in Torque and Angular Momentum

Conservation of Angular Momentum: In a closed system, the total angular momentum is conserved, meaning that the angular momentum of the system remains constant unless an external torque is applied.
Relationship between Torque and Angular Acceleration: The torque acting on an object is proportional to the object’s angular acceleration, as described by the equation:

τ = Iα

where α is the angular acceleration (in radians per second squared, rad/s²).

Angular Momentum of a System of Particles: The total angular momentum of a system of particles is the vector sum of the angular momenta of the individual particles.
Rotational Kinetic Energy: The rotational kinetic energy of an object is given by the formula:

KE_rot = (1/2) Iω²

where KE_rot is the rotational kinetic energy (in joules, J).

Precession and Nutation: Gyroscopes and other rotating objects can exhibit precession and nutation, which are complex rotational motions that involve the interaction between torque and angular momentum.

Conclusion

Torque and angular momentum are fundamental concepts in classical mechanics that are essential for understanding the rotational motion of objects. By understanding the factors that affect these quantities and the relationships between them, students can develop a deeper understanding of the dynamics of various physical systems, from simple rigid bodies to complex celestial bodies.

References:

The Inverse Relationship Between Torque and Speed: A Comprehensive Guide

June 25, 2024March 29, 2022 by Manish Naik

The relationship between torque and speed is an essential concept in physics and mechanical engineering. As the speed of a rotating body increases, the torque it can produce decreases, and vice versa. This inverse relationship is crucial in understanding the behavior of various mechanical systems and designing efficient power transmission mechanisms.

Understanding the Torque-Speed Relationship

The relationship between torque and speed can be expressed mathematically as:

Torque = Power / Speed or τ = P / ω

Where:
– P is the power (work done per unit time)
– τ is the torque (rotational ability of a body)
– ω is the angular speed/velocity (rate of change of angular displacement)

This formula can be rearranged to compute the angular velocity required to achieve a given torque and power:

Angular Velocity (ω) = Power (P) / Torque (τ)

The inverse relationship between torque and speed can be visualized in the following graph:

As the graph shows, as the speed increases, the torque decreases, and vice versa. This relationship is crucial in the design and operation of various mechanical systems, such as electric motors, gearboxes, and power transmission systems.

Theoretical Foundations

The inverse relationship between torque and speed is rooted in the fundamental principles of physics, specifically the conservation of energy and the concept of power.

Conservation of Energy

The conservation of energy states that energy cannot be created or destroyed, but it can be transformed from one form to another. In the case of a rotating body, the total energy is the sum of the kinetic energy (due to the body’s motion) and the potential energy (due to the body’s position).

As the speed of the rotating body increases, its kinetic energy increases, but its potential energy (in the form of torque) decreases. This inverse relationship between kinetic and potential energy is the basis for the inverse relationship between torque and speed.

Power and Torque

Power is defined as the rate of work done, or the amount of energy transferred per unit time. In the context of a rotating body, power can be expressed as the product of torque and angular velocity:

Power (P) = Torque (τ) × Angular Velocity (ω)

Rearranging this equation, we can derive the inverse relationship between torque and speed:

Torque (τ) = Power (P) / Angular Velocity (ω)

This equation clearly shows that as the angular velocity (speed) increases, the torque must decrease to maintain the same power output.

Applications and Examples

The inverse relationship between torque and speed has numerous applications in various fields, including:

Electric Motors

Electric motors are a prime example of the torque-speed relationship in action. Electric motors are designed to produce a specific torque-speed characteristic, which is determined by the motor’s construction and the applied voltage. As the load on the motor increases, the speed decreases, and the torque increases to maintain the same power output.

For instance, a 100-watt electric motor operating at 100 rad/s would have a torque of 1 Nm. If the angular velocity were to increase to 200 rad/s, the torque would decrease to 0.5 Nm, while the power output would remain the same.

Gearboxes

Gearboxes are used to transmit power from one rotating shaft to another, often with a change in speed and torque. The gear ratio of a gearbox is designed to match the torque-speed requirements of the driven load. By adjusting the gear ratio, the gearbox can increase the torque while decreasing the speed, or vice versa, depending on the application.

For example, a gearbox with a gear ratio of 1:5 would increase the torque by a factor of 5 while decreasing the speed by the same factor. This is useful in applications where high torque is required, such as in heavy machinery or industrial equipment.

Power Transmission Systems

The inverse relationship between torque and speed is also crucial in the design of power transmission systems, such as those found in vehicles, industrial machinery, and renewable energy systems. Engineers must carefully consider the torque-speed characteristics of the various components in the system to ensure efficient power transfer and optimal performance.

In a vehicle, for instance, the engine produces a specific torque-speed curve, which must be matched by the transmission and final drive gears to provide the desired acceleration and top speed. The gear ratios are selected to ensure that the engine operates within its optimal torque and speed range, maximizing the vehicle’s efficiency and performance.

Numerical Examples

Let’s consider a few numerical examples to illustrate the inverse relationship between torque and speed:

Example 1: A motor has a power rating of 1 kW and is operating at an angular velocity of 100 rad/s. What is the torque produced by the motor?

Given:
– Power (P) = 1 kW = 1000 W
– Angular Velocity (ω) = 100 rad/s

Torque (τ) = Power (P) / Angular Velocity (ω)
Torque (τ) = 1000 W / 100 rad/s = 10 Nm

Example 2: The same motor from Example 1 is now operating at an angular velocity of 200 rad/s. What is the new torque produced by the motor?

Given:
– Power (P) = 1000 W
– Angular Velocity (ω) = 200 rad/s

Torque (τ) = Power (P) / Angular Velocity (ω)
Torque (τ) = 1000 W / 200 rad/s = 5 Nm

As the angular velocity doubled, the torque decreased by a factor of 2, from 10 Nm to 5 Nm.

Example 3: A gearbox has a gear ratio of 1:10. If the input shaft is rotating at 1000 rpm, what is the output shaft’s torque and speed?

Given:
– Gear Ratio = 1:10
– Input Shaft Speed = 1000 rpm

Output Shaft Speed = Input Shaft Speed / Gear Ratio
Output Shaft Speed = 1000 rpm / 10 = 100 rpm

Output Shaft Torque = Input Shaft Torque × Gear Ratio
Output Shaft Torque = Input Shaft Torque × 10

The gearbox has increased the torque by a factor of 10 while decreasing the speed by the same factor, as expected from the inverse relationship between torque and speed.

These examples demonstrate how the inverse relationship between torque and speed can be used to calculate the various parameters in mechanical systems, which is crucial for their design and optimization.

Conclusion

The inverse relationship between torque and speed is a fundamental concept in physics and mechanical engineering. This relationship is rooted in the principles of conservation of energy and the definition of power, and it has numerous applications in various fields, such as electric motors, gearboxes, and power transmission systems.

By understanding the mathematical relationship between torque and speed, engineers can design and optimize mechanical systems to achieve the desired performance characteristics. The numerical examples provided in this article illustrate how this relationship can be applied to solve real-world problems and ensure the efficient operation of rotating machinery.

References

Vedantu. (n.d.). Relation between Torque and Speed. Retrieved from https://www.vedantu.com/physics/relation-between-torque-and-speed
Byjus. (n.d.). Relation between Torque and Speed. Retrieved from https://byjus.com/physics/relation-between-torque-and-speed/
I Need Motors. (n.d.). What is the Relationship Between Speed and Torque? Retrieved from https://www.ineedmotors.com/news/what-is-the-relationship-between-speed-and-tor-53813295.html

How to Find Torque from Angular Velocity: A Comprehensive Guide

June 25, 2024March 27, 2022 by Manish Naik

Summary

To find the torque from angular velocity, you need to understand the relationship between torque, moment of inertia, and angular acceleration. This comprehensive guide will walk you through the step-by-step process, including the necessary formulas, examples, and problem-solving techniques to help you master this concept in physics.

Understanding Torque and Angular Acceleration

Torque (τ) is a measure of the rotational force applied to an object, which causes it to rotate around a specific axis. The formula for torque is:

τ = I × α

Where:
– τ is the torque (in Newton-meters, N·m)
– I is the moment of inertia of the object (in kilogram-square meters, kg·m²)
– α is the angular acceleration of the object (in radians per second squared, rad/s²)

The moment of inertia (I) is a measure of an object’s resistance to changes in its rotational motion. It depends on the object’s mass and the distribution of that mass around the axis of rotation.

Angular acceleration (α) is the rate of change of an object’s angular velocity (ω) over time. It can be calculated using the formula:

α = Δω / Δt

Where:
– Δω is the change in angular velocity (in radians per second, rad/s)
– Δt is the change in time (in seconds, s)

Calculating Moment of Inertia

The moment of inertia (I) of an object depends on its mass and the distribution of that mass around the axis of rotation. For simple geometric shapes, there are standard formulas for calculating the moment of inertia:

Solid Cylinder or Disk:
I = (1/2) × m × r²
Where:
m is the mass of the object (in kilograms, kg)
r is the radius of the object (in meters, m)
Hollow Cylinder:
I = (1/2) × m × (r₁² + r₂²)
Where:
m is the mass of the object (in kilograms, kg)
r₁ is the inner radius (in meters, m)
r₂ is the outer radius (in meters, m)
Solid Sphere:
I = (2/5) × m × r²
Where:
m is the mass of the object (in kilograms, kg)
r is the radius of the object (in meters, m)
Thin Rod (about the center):
I = (1/12) × m × L²
Where:
m is the mass of the object (in kilograms, kg)
L is the length of the object (in meters, m)

For more complex shapes, you may need to use integration techniques to calculate the moment of inertia.

Solving for Angular Acceleration

Once you have the moment of inertia (I) and the applied torque (τ), you can solve for the angular acceleration (α) using the formula:

α = τ / I

Rearranging the torque formula, we get:

α = τ / I

Where:
– α is the angular acceleration (in radians per second squared, rad/s²)
– τ is the applied torque (in Newton-meters, N·m)
– I is the moment of inertia of the object (in kilogram-square meters, kg·m²)

Calculating Angular Velocity

With the angular acceleration (α) and the initial angular velocity (ω₀), you can calculate the angular velocity (ω) at any given time (t) using the formula:

ω = ω₀ + α × t

Where:
– ω is the final angular velocity (in radians per second, rad/s)
– ω₀ is the initial angular velocity (in radians per second, rad/s)
– α is the angular acceleration (in radians per second squared, rad/s²)
– t is the time elapsed (in seconds, s)

Example Problem

Let’s solve an example problem to demonstrate the process of finding torque from angular velocity.

Problem: A wheel with a mass of 50 kg and a radius of 0.5 m is rotating with an initial angular velocity of 10 rad/s. A constant torque of 50 N·m is applied to the wheel. Find the angular acceleration and the angular velocity after 5 seconds.

Solution:

Calculate the moment of inertia (I) of the wheel:
I = (1/2) × m × r²
I = (1/2) × 50 kg × (0.5 m)²
I = 3.125 kg·m²
Calculate the angular acceleration (α) using the torque formula:
τ = I × α
α = τ / I
α = 50 N·m / 3.125 kg·m²
α = 16 rad/s²
Calculate the final angular velocity (ω) after 5 seconds:
ω = ω₀ + α × t
ω = 10 rad/s + 16 rad/s² × 5 s
ω = 80 rad/s

Therefore, the angular acceleration of the wheel is 16 rad/s², and the angular velocity after 5 seconds is 80 rad/s.

Additional Examples and Practice Problems

Here are some additional examples and practice problems to help you further understand how to find torque from angular velocity:

Example: A solid cylinder with a mass of 20 kg and a radius of 0.3 m is rotating at an angular velocity of 12 rad/s. If a constant torque of 15 N·m is applied, find the angular acceleration and the new angular velocity after 3 seconds.
Practice Problem: A hollow cylinder with an inner radius of 0.2 m and an outer radius of 0.4 m has a mass of 30 kg. The cylinder is initially rotating at 8 rad/s. If a constant torque of 25 N·m is applied, calculate the angular acceleration and the angular velocity after 4 seconds.
Example: A solid sphere with a mass of 10 kg and a radius of 0.1 m is rotating at an angular velocity of 6 rad/s. A constant torque of 2 N·m is applied to the sphere. Find the angular acceleration and the new angular velocity after 2 seconds.
Practice Problem: A thin rod with a mass of 5 kg and a length of 1 m is rotating about its center. The rod is initially rotating at 4 rad/s. If a constant torque of 3 N·m is applied, calculate the angular acceleration and the angular velocity after 1.5 seconds.

Remember to show your work and use the appropriate formulas and equations to solve these problems.

Conclusion

In this comprehensive guide, we have explored the concepts of torque, moment of inertia, and angular acceleration, and how they are related to finding the torque from angular velocity. By understanding the underlying principles and applying the relevant formulas, you can confidently solve a wide range of problems involving the rotational motion of objects.

References

Electrical Energy to Heat Energy: What, How to Convert, Process

January 20, 2024March 23, 2022 by Manish Naik

The article discusses about the electrical energy to heat energy conversion that depicts how electrons are charged to lose their energy.

When moving electrons have electrical energy interact with stationary electrons, their energy is converted into chemical energy or light energy. But if their energy is more than the stationary electron’s capacity, the excess of electrical energy is released in the form of heat energy.

The electrical energy is transformed into chemical energy when stationary electrons soak the kinetic energy. It is the process of kinetic energy to potential energy conversion. If more electrons with kinetic energy interact with the chemically bonded stationary electrons with the stored potential energy, it reverses the energy conversion.

When switching on the electric bulb attached to the battery, the stationary electrons within the battery split their chemical bond. They become free to carry stored potential energy as kinetic energy in the form of electrical energy through the conducting wire. When such electrons contact the tungsten material of the bulb, they interact to generate another energy form.

The external electrical energy from the battery breaks the chemical bonds of the electrons within the bulb so that they begin moving rapidly by absorbing external kinetic energy. The temperature is a physical quantity that briefs us on how fast electrons move. Hence, the rapid motion of electrons radiates heat energy from electrical energy.

That’s the reason when the external electric energy flows through the current-carrying conductor, we sense some heat on its surface when we touch it.

Depending on the external electrical energy, electrons hold quicker movement. The quicker the electrons move, the hotter their surface becomes. An object with a higher temperature delivers its heat to other objects at lower temperatures. Therefore, the fast-moving electrons discharge excess energy in the form of light energy.

When we add bulb’s light energy and heat energy, we discovered that their sum equals the electrical energy, as per the energy conversion law.

Read about Electrical Energy to Heat Energy Examples.

What Process is Electrical Energy to Heat Energy?

The process of electrical energy to heat energy is electric heating.

Electric heating is the process of yielding heat energy from the chemical elements on the external electric energy passes. The electrical device contains a resistor as a heating element that functions on the Joule heating principle to deliver heat energy, later laboriously employed for commercial purposes.

The instant water heater installed in the bathroom is based on the electrical energy to heat energy conversion. Whenever we turn on the heater, we acquire hot water streams. But have you wondered why it takes some time for the water to become hot? The water heater operates on Joule heating or Resistive heating, where heat is created by passing an electric current through the conductor.

Electric heating involves the interactions of moving electrons as a charge carrier with the heating element within the conductor. The electric field developed on the conductor due to potential difference accelerates the stationary electrons. So electrons transmit with kinetic energy towards the direction of the electric field into the conductor.

In most electric heaters, a resistor is employed as the heating element. It is two passive terminal components that regulate the flow of electrical energy within the conductor.

The kinetic energy is then transmitted to the fixed electrons when moving electrons hit within the resistor element. The electrons within the resistor are excited to move rapidly due to absorbing kinetic energy. That is when the resistor dissipates the excess energy of its electrons as the heat energy using the Joule heating principle.

The work done (W) of the moving electron into the conductor is given by W = VIt.

where, V is voltage and I is current passing through conductor.

The amount of heat dissipated by the resistor of the conductor is termed as its Power of heating P.

P = W/t = VI

As per Ohm’s law, V = IR,

Therefore, P = I²R, which analogs to Joule’s first law.

Therefore, the principle of Joule heating is derived from Joule’s first law, which states that

“The power of heating (P) of an electrical conductor is proportional to the product of the square of the electric current passes (I) and its resistance(R)”.

Read about Mechanical Energy to Kinetic Energy Conversion

How to Convert Electrical Energy to Heat Energy?

The electrical energy is converted into heat energy due to resistance.

When some quantity of electric current flows through the conducting material, it gets heated. Every conductor has an inbuilt resistance that disperses heat energy when it acquires electrical energy. The phenomenon prevents the conductor from short-circuiting during high electrical energy.

Every conductor contains some resistance to maintain the flow of current. We can also manage the current flow by adding an external resistor to the conductor. The desired heat energy value can be obtained from the conductor using Joule heating.

When a current passes through any conductors, its surface becomes hotter. The conductor’s resistance maintains the electrical energy by absorbing and then radiating the exact amount of energy as heat energy. Otherwise, we notice short-circuiting when an immense amount of current passes through the resistance free conductor.

Also Read:

Electrical Energy to Chemical Energy: What, How to Convert, Process

January 21, 2024March 20, 2022 by Manish Naik

The article discusses about electrical energy to chemical energy conversion that depicts how molecules form chemical bonds due to electric charge transfer.

The flow of electrons carries kinetic energy in the form of electrical energy. The moving electrons induce a chemical reaction when they hit with other material’s chemically bonded electrons. The chemical reactions construct a new molecule that retains energy in chemical energy form.

The electrical to chemical energy transformation illustrates how kinetic energy is changed into potential energy. The kinetic energy allows a stationary charged particle to be in motion. So when the electrons at rest acquire the energy from an external power source, they are excited to move rapidly.

Such moving electrons collide with the other chemical compounds and annihilate their chemically bonded electrons. Hence, the chemical reaction happens between free electrons of the compound, creating a new chemical compound.

Energy in Chemical Bonding — **Breaking and Forming of Chemical Bonds**
(credit: shutterstock)

When the newly bonded stationary electrons store the kinetic energy of moving electrons, it becomes their potential energy. That’s how the new chemical compound holds an equivalent amount of electrical kinetic energy to form chemical potential energy.

Read about Electrical Energy to Chemical Energy Examples

What Process is Electrical Energy to Chemical Energy?

The electrical energy to chemical energy conversion is the electrolysis process.

Electrolysis is the electrochemical process that begins when the electrical energy is delivered from external sources. The outer electrical energy is then accumulated chemically within the chemical compound, permitting us to acquire the electrical energy back out later.

What Process is Electrical Energy to Chemical Energy — **Electrolysis Process**
(credit: shutterstock)

Every rechargeable electronic device works on an electrolysis process. When we connect the charger to such a device and switch it on, the electrons within the charger are excited. The excited electrons move with electrical energy towards the rechargeable battery through the wire.

The rechargeable battery includes chemical elements such as electrolytic solution, which permit charge transfer within the battery. So when external energy is delivered to the battery, the chemical bonds within its elements are broken. The electrons get free from chemical bonding and then react to construct new chemical elements by various chemical reactions.

The new chemical elements within the battery then have stationary charge electrons, which store the external electrical energy as chemical potential energy for a more extended period.

Except the charging a storage battery, the example that involves the electrolysis process to convert the electrical to chemical energy is

Charging Capacitors
Electroplating
Cyclotrons
RF Inductive Heating
Chip Making
Electric Welding

Whenever we like to utilize such charged devices, reverse chemical reactions occur. It transforms the chemical potential energy stored within the battery into electrical energy and other kinetic energy forms such as light energy or heat energy.

Read about the Potential Energy to Chemical Energy Examples

How to Convert Electrical Energy to Chemical Energy?

The electricity is converted into chemicals within the electrolytic cell when chemical reactions occur.

The electrolytic cell possesses two chemically dissimilar electrodes. When electrical energy is provided to the cell, a couple of non-spontaneous redox reactions arise at both electrodes, transforming the supplied electrical energy into chemical energy.

Redox Reactions — **How to Convert Electrical Energy to Chemical Energy**
(credit: shuttertsock)

The electrochemical electrolytic electrodes are separated by specific distance and dipped into the electrolytic solution, which acts as a solvent. The electrode within a cell connected to the external battery’s positive terminal is termed ‘anode’, whereas the electrode connected to the battery’s negative terminal is termed ‘cathode’.

The positively charged ions, such as cations, get attracted toward the negative electrode. The negatively charged ions, such as anions, get attracted toward the positive electrode.

Suppose both electrodes are immersed into NaCl electrolytic solution and an external power source connected.

When electrical energy is delivered to both the electrodes, moving free electrons are injected into the cathode. Rich in electrons, the cathode attracts the positive ions Na⁺ within the electrolytic solution. The chemical reaction between Na⁺ ions and free electrons e^– creates a new chemical compound Na, deposited into the cathode electrode.

Na⁺ + e^–→ Na

The half-cell reaction that occurs at the negative cathode is the “reduction redox reaction” that transforms the electrical energy (I) into chemical potential energy.

At the same time as the reduction reaction, the positive anode saturates out its electrons which react with the negative ion Cl within the electrolytic solution. The chemical reaction oxidizes the anode, giving rise to the formation of chlorine Cl₂ gas.

2Cl^–→ Cl₂ + 2e^–

The half-cell reaction that occurs at the positive anode is the “oxidation redox reaction” that transforms the electrical energy (I) into chemical potential energy.

After both redox reactions, we found that two ions within the electrolytic solution become new chemical compounds.

Therefore, we understood that the electrolysis process created the chemical energy by decomposing both electrodes on the electrical energy passes.

The amount of chemical substance forming during redox reaction is signified by ‘Faraday’s law of electrolysis’, such as the weight of the chemical substance is directly proportional to the quantity of electrical energy passed through the cell.

The anode becomes negative during the discharging process, and the cathode becomes positive because of a reversible chemical reaction to yield electrical energy from stored potential energy. The electrolytic cell is called a ‘galvanic cell’ as it does not demand the external power source to initiate energy conversations.

**Galvanic Cell Vs. Electrolytic Cell** (credit: shutterstock)

Also Read: