Solenoid produces magnetic field when a conventional current is been instantly passed through the wires of the solenoid. Basically when current is passed through a conducting material it will instantly produce current.
Solenoid is a current conducting material which is basically a coil wound around a straight material. When current is been passed through the wires of the coil, the respective charges present in the coil will produce both electric and magnetic fields.
Solenoid is one of the forms of electromagnet since it has magnetisms in it the minute current is passed through it. When current is passed through it, the coil produces uniform magnetic field in the given space.
When we place any metal core inside the solenoid it will make the magnetic lines of flux surround them. Also the presence of the metal core will increase the induction inside it compared to that of the air core left outside.
An interesting fact about the solenoid is that they are basic coils wound around a metal which will produce controlled magnetic fields which and rather can be used as one of the electromagnet as well.
What is the magnetic field of solenoid?
Normally when a coil simply conducts electricity the electric field and the magnetic field will be not large, but if it is said to be a solenoid the case differs.
Solenoid is a coil which conducts electricity which also produces the magnetic field inside the coil. Solenoid is the one which will produce strong magnetic field when wound around a coil. Solenoid is basically a wire wound around a current conducting coil.
The magnetic field of the coil is the one which when wound around a cylindrical coil. Usually a normal coil will produce electricity which in turn will produce electric field and magnetic field.
Solenoid is considered to be a temporary magnet due to which when it is unwound there will no trace of magnetism present in the system. The main reason for solenoid produces magnetic field is the presence of electric current in the coil.
Now when the coil has a long wire wound around it, it will also produce magnetic field. The wire wound around the coil is basically is the solenoid and this solenoid produces magnetic field. Solenoid produces magnetic field which comparatively gives of a strong magnetic field and also is uniform in nature.
The solenoid is one of the best examples for a strong electromagnet and this magnetism produced in the solenoid is a controlled magnetic field and a uniform magnetic field too.
When does a solenoid produce a magnetic field?
The answer to this question is, when the electric current is passed through the wires of the coil it will in turn produce magnetic field is simple as that.
Solenoid is simply not an individual material which by itself will produces magnetic field and in fact when wound around a coil it will produce magnetic field. Solenoid automatically produces magnetic field when the current from external source is passed through it.
Solenoid is basically a long straight wire which when wound around a current conducting coil it will produces a strong magnetic field which is a uniform magnetic field too.
Let us now take into account that the current carrying coil will for sure produce magnetic field which is very much similar to that of the bar magnet which is regarded to be a permanent magnet.
But the magnetic field produced by coil and the bar magnet is not the same because the bar magnet has magnetic field which is almost straight line and is contrary to that of the magnetic fields of the coil
Now when we consider another coil, it can be a long wire wound around the coil too. The wire is basically in circular form and will produce a magnetic field which does not intersect with the one of the solenoid.
It is how the solenoid produces magnetic field which does not intersect with other magnetic field but when put together as a whole it will produce a large and strong magnetic field.
Where is magnetic field more in solenoid?
When the circuit is closed, the current is passed to the copper wire, and it will conduct electricity which in turn produces a magnetic field.
The magnetic field in a solenoid is more close to the wire of solenoid the reason being, each turns in the wire of a solenoid will produces its own magnetic field compared to that of a straight wire.
Now let us understand this using an example, consider copper wire in circles to be connected through a glass. Place some iron fillings in the set up to know how the magnetic field works in current loops.
The iron fillings will gather mostly close to the wire loops and in the centre it will look spread around.
The main reason for this occurrence is that the magnetic in this step up is closer to the wire than in the centre as the magnetic fields in the centre are almost a straight line.
Hence, magnetic field in a solenoid will more and string in the area closer to the wire rather than in the centre, since each wire in the solenoid has number of turns which produces its own magnetic field.
How to find magnetic field in solenoid?
Magnetic field in a solenoid is a strong uniform one when current is passed into the circuit.
Solenoid produces magnetic field which acts as a temporary magnet and it will lose its magnetism property when current is not passed in the coil.
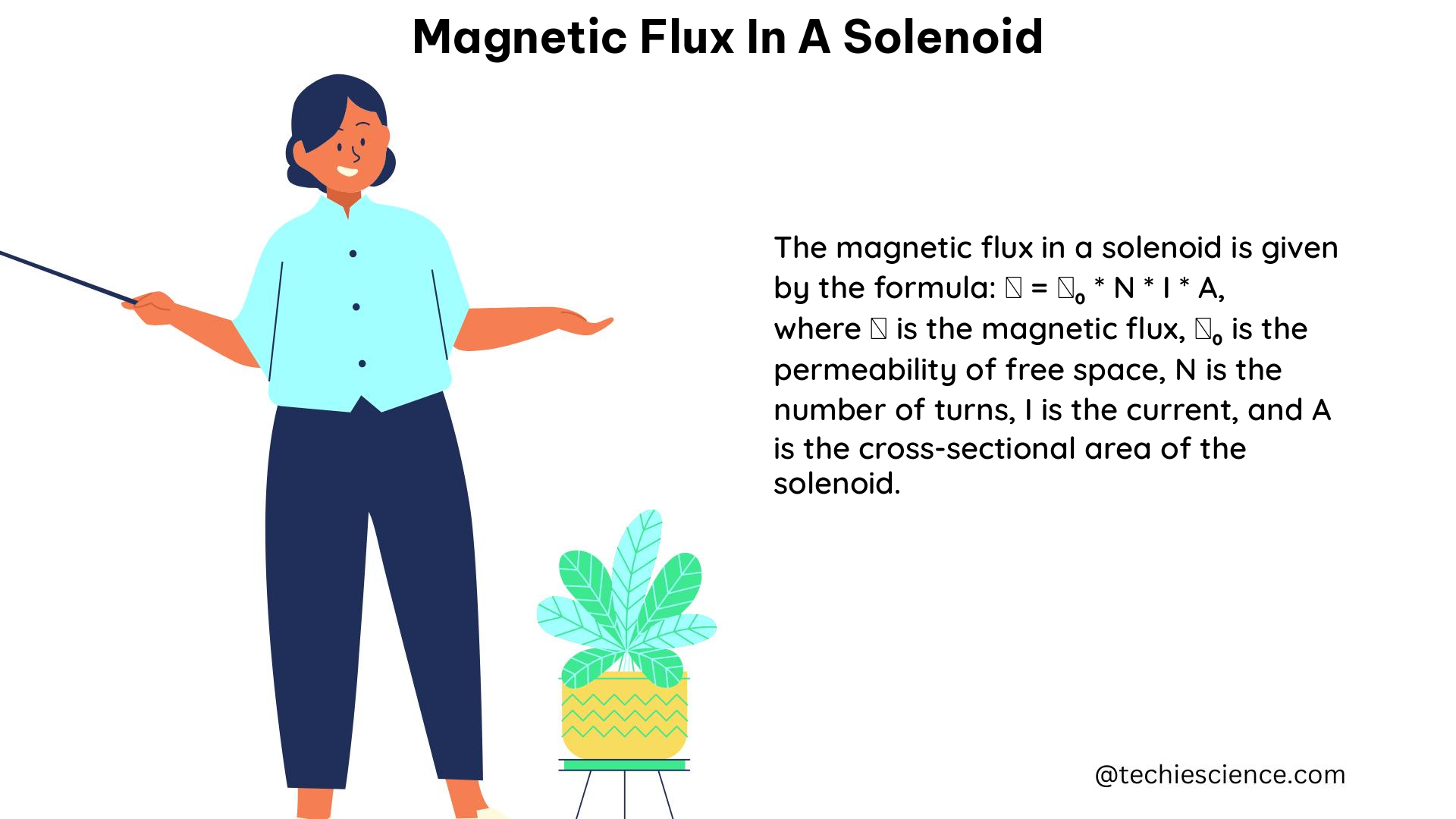
Now using a formula we shall find out how the magnetic field in a solenoid is calculated. There is a formula to calculate the magnetic field in a solenoid, B = μoIN / L. Here, μo=permeability, N= number of turns in the wire, I= amount of current passed in the coil.
Here the number of turns of wire is very much important because of the reason being, each turn of the wire will produce its own magnetic field and also a strong one.
Solenoid is usually used for practical purposes as it is used as a temporary magnet instead of a bar magnet. One main advantage of using solenoid is that they have large and strong magnetic fields.
Is magnetic field inside a solenoid is zero?
In general for a solenoid being a long one, the magnetic fields will be zero outside the solenoid but inside the solenoid there will always be magnetic field present.
In a current carrying coil as long as current is been passed to the wires wound around it there will magnetic fields produced in it.
When there is no current been passed, there will be no production of electric current due to which no electric and magnetic fields will be produced so the magnetic field in solenoid will be zero, which is one of the ways in which the magnetic field could be zero.
It can be proved using the right hand’s thumb rule, where thumb will indicate the direction of the current in the coil and the fingers encircling will indicate the direction of the magnetic field.
So by this way we can determine the magnetic field inside a solenoid, like mentioned before, it either can be due the absence of current and the long solenoid.
Why is magnetic field in solenoid uniform?
The magnetic field in a solenoid is uniform due to the fact that each turn of the wire produces its own magnetic field.
Solenoid is a material which produces a temporary magnetic field and it is a strong one. When each turn of the wire wound around the current carrying coil it produces its own magnetic field.
When the current is passed through the wire each of it will have equal amount of current passing through it. So the current passed will produce the magnetic field which is produced by each turn of the wire.
The individual magnetic field produced by the wire will merge with one another and shall create large magnetic field which results to be a uniform magnetic field too.
How does a solenoid produce a uniform magnetic field?
We must know that the amount of current passed to the solenoid and the magnetic field produced in each point of the it will be the same.
The wires in the solenoid is regarded to be parallel to each other so the magnetic produced by it will be parallel too. The parallel wires will now merge together giving a great deal of magnetic field to the system
The parallel magnetic fields of each wire will not intersect each other but they will merge with one another and create a strong uniform magnetic field.
Considering an experiment of the iron filing spread around the copper wire wound around the current carrying coils shall prove us with essential answers that the magnetic fields are uniform in the whole of the solenoid.
Unlike the straight wire, the wire wound around a coil will be circular in form. So the each circular wire will produce magnetic field which is strong compared to the straight wire.
In each of the turns the magnetic field is produced and also merges with one producing a strong uniform magnetic field.
Why solenoid has no magnetic field outside?
The magnetic field outside the solenoid is weak compared to that of inner magnetic field which is closest to the wire.
Solenoid has no magnetic field outside because of the fact that the number of turns of the wire is very much less as compared to that of lines inside the solenoid.
When we pass current, it usually is passed inside the loops of wire, so the magnetic field is strong in the core rather than outside the core. The magnetic field lines are merged and become a strong uniform one inside the solenoid making the magnetic fields outside the coils as zero.
One of the big impacts of the solenoid is that the number of turns of wire is proportional to the magnetic field produced. Each turns contributes its own magnetic field inside the solenoid and so by which the magnetic field is very much less outside the solenoid.
Problem:
A solenoid has a length of 80 cm having the number of turns of the coil to be 360 and the current passing through the solenoid is 15 A. Calculate the magnetic field produced by the solenoid?
Solution:
N = 280
I = 13 A
μo = 1.26 × 10−6 T/m
L = 0.7m
According to the formula, B = μoIN / L
B = (1.26×10−6 × 13 × 280) / 0.7
B = 6.552 × 10−3 N/Amps m
Conclusion
solenoid produces magnetic field when current is passed through it. the magnetic field produced in a solenoid is much more stronger and uniform too. Solenoid is a material used for practical purposes since it acts as a temporary magnet. solenoid is one of the best examples of electromagnetism. On the whole solenoid is a strong temporary magnet which will produce strong magnetic fields compared to the bar magnet.
Also Read: