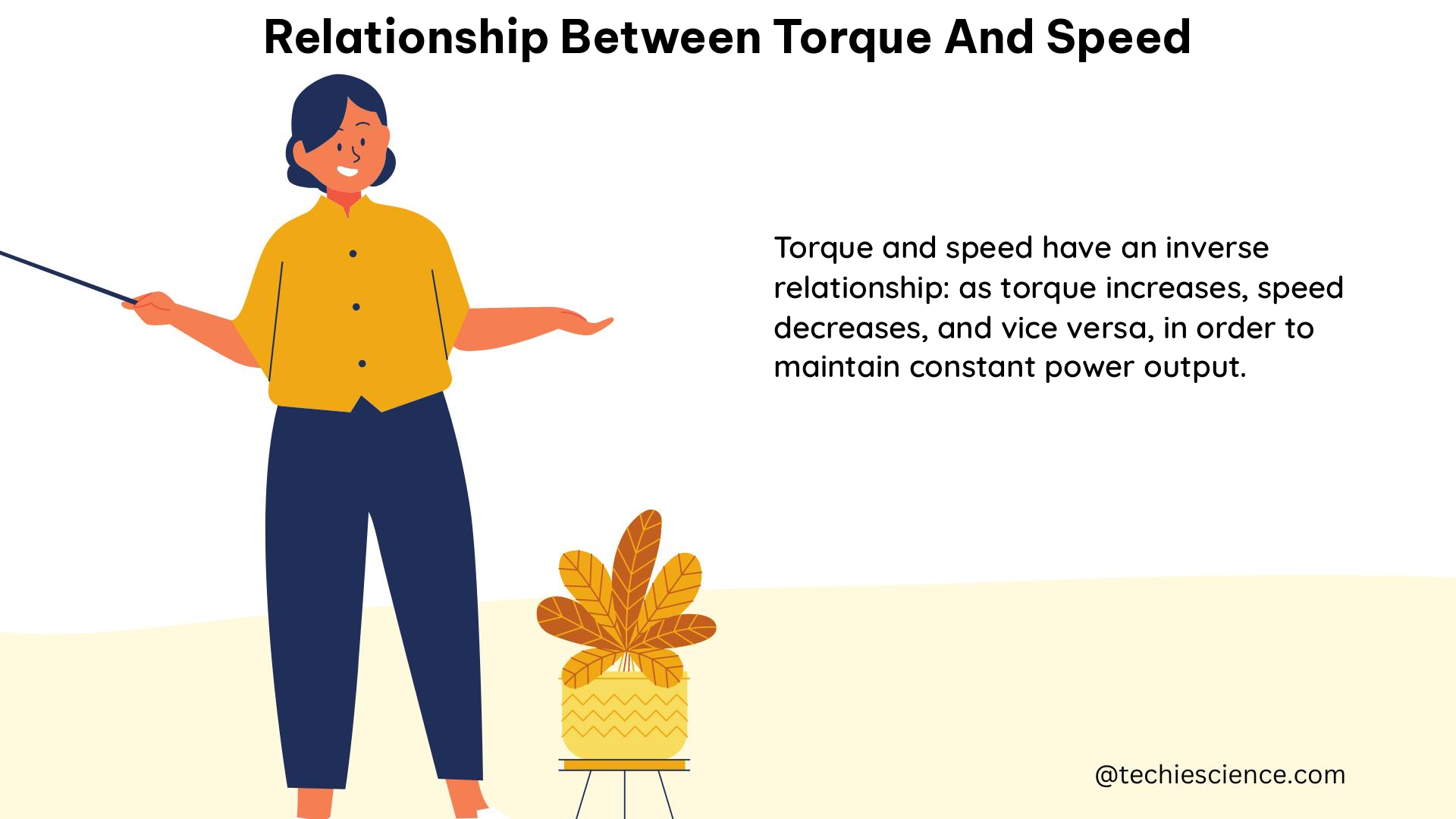
The relationship between torque and speed is an essential concept in physics and mechanical engineering. As the speed of a rotating body increases, the torque it can produce decreases, and vice versa. This inverse relationship is crucial in understanding the behavior of various mechanical systems and designing efficient power transmission mechanisms.
Understanding the Torque-Speed Relationship
The relationship between torque and speed can be expressed mathematically as:
Torque = Power / Speed or τ = P / ω
Where:
– P is the power (work done per unit time)
– τ is the torque (rotational ability of a body)
– ω is the angular speed/velocity (rate of change of angular displacement)
This formula can be rearranged to compute the angular velocity required to achieve a given torque and power:
Angular Velocity (ω) = Power (P) / Torque (τ)
The inverse relationship between torque and speed can be visualized in the following graph:

As the graph shows, as the speed increases, the torque decreases, and vice versa. This relationship is crucial in the design and operation of various mechanical systems, such as electric motors, gearboxes, and power transmission systems.
Theoretical Foundations
The inverse relationship between torque and speed is rooted in the fundamental principles of physics, specifically the conservation of energy and the concept of power.
Conservation of Energy
The conservation of energy states that energy cannot be created or destroyed, but it can be transformed from one form to another. In the case of a rotating body, the total energy is the sum of the kinetic energy (due to the body’s motion) and the potential energy (due to the body’s position).
As the speed of the rotating body increases, its kinetic energy increases, but its potential energy (in the form of torque) decreases. This inverse relationship between kinetic and potential energy is the basis for the inverse relationship between torque and speed.
Power and Torque
Power is defined as the rate of work done, or the amount of energy transferred per unit time. In the context of a rotating body, power can be expressed as the product of torque and angular velocity:
Power (P) = Torque (τ) × Angular Velocity (ω)
Rearranging this equation, we can derive the inverse relationship between torque and speed:
Torque (τ) = Power (P) / Angular Velocity (ω)
This equation clearly shows that as the angular velocity (speed) increases, the torque must decrease to maintain the same power output.
Applications and Examples
The inverse relationship between torque and speed has numerous applications in various fields, including:
Electric Motors
Electric motors are a prime example of the torque-speed relationship in action. Electric motors are designed to produce a specific torque-speed characteristic, which is determined by the motor’s construction and the applied voltage. As the load on the motor increases, the speed decreases, and the torque increases to maintain the same power output.
For instance, a 100-watt electric motor operating at 100 rad/s would have a torque of 1 Nm. If the angular velocity were to increase to 200 rad/s, the torque would decrease to 0.5 Nm, while the power output would remain the same.
Gearboxes
Gearboxes are used to transmit power from one rotating shaft to another, often with a change in speed and torque. The gear ratio of a gearbox is designed to match the torque-speed requirements of the driven load. By adjusting the gear ratio, the gearbox can increase the torque while decreasing the speed, or vice versa, depending on the application.
For example, a gearbox with a gear ratio of 1:5 would increase the torque by a factor of 5 while decreasing the speed by the same factor. This is useful in applications where high torque is required, such as in heavy machinery or industrial equipment.
Power Transmission Systems
The inverse relationship between torque and speed is also crucial in the design of power transmission systems, such as those found in vehicles, industrial machinery, and renewable energy systems. Engineers must carefully consider the torque-speed characteristics of the various components in the system to ensure efficient power transfer and optimal performance.
In a vehicle, for instance, the engine produces a specific torque-speed curve, which must be matched by the transmission and final drive gears to provide the desired acceleration and top speed. The gear ratios are selected to ensure that the engine operates within its optimal torque and speed range, maximizing the vehicle’s efficiency and performance.
Numerical Examples
Let’s consider a few numerical examples to illustrate the inverse relationship between torque and speed:
- Example 1: A motor has a power rating of 1 kW and is operating at an angular velocity of 100 rad/s. What is the torque produced by the motor?
Given:
– Power (P) = 1 kW = 1000 W
– Angular Velocity (ω) = 100 rad/s
Torque (τ) = Power (P) / Angular Velocity (ω)
Torque (τ) = 1000 W / 100 rad/s = 10 Nm
- Example 2: The same motor from Example 1 is now operating at an angular velocity of 200 rad/s. What is the new torque produced by the motor?
Given:
– Power (P) = 1000 W
– Angular Velocity (ω) = 200 rad/s
Torque (τ) = Power (P) / Angular Velocity (ω)
Torque (τ) = 1000 W / 200 rad/s = 5 Nm
As the angular velocity doubled, the torque decreased by a factor of 2, from 10 Nm to 5 Nm.
- Example 3: A gearbox has a gear ratio of 1:10. If the input shaft is rotating at 1000 rpm, what is the output shaft’s torque and speed?
Given:
– Gear Ratio = 1:10
– Input Shaft Speed = 1000 rpm
Output Shaft Speed = Input Shaft Speed / Gear Ratio
Output Shaft Speed = 1000 rpm / 10 = 100 rpm
Output Shaft Torque = Input Shaft Torque × Gear Ratio
Output Shaft Torque = Input Shaft Torque × 10
The gearbox has increased the torque by a factor of 10 while decreasing the speed by the same factor, as expected from the inverse relationship between torque and speed.
These examples demonstrate how the inverse relationship between torque and speed can be used to calculate the various parameters in mechanical systems, which is crucial for their design and optimization.
Conclusion
The inverse relationship between torque and speed is a fundamental concept in physics and mechanical engineering. This relationship is rooted in the principles of conservation of energy and the definition of power, and it has numerous applications in various fields, such as electric motors, gearboxes, and power transmission systems.
By understanding the mathematical relationship between torque and speed, engineers can design and optimize mechanical systems to achieve the desired performance characteristics. The numerical examples provided in this article illustrate how this relationship can be applied to solve real-world problems and ensure the efficient operation of rotating machinery.
References
- Vedantu. (n.d.). Relation between Torque and Speed. Retrieved from https://www.vedantu.com/physics/relation-between-torque-and-speed
- Byjus. (n.d.). Relation between Torque and Speed. Retrieved from https://byjus.com/physics/relation-between-torque-and-speed/
- I Need Motors. (n.d.). What is the Relationship Between Speed and Torque? Retrieved from https://www.ineedmotors.com/news/what-is-the-relationship-between-speed-and-tor-53813295.html