The article discusses about is horizontal speed constant, along with its problem examples.
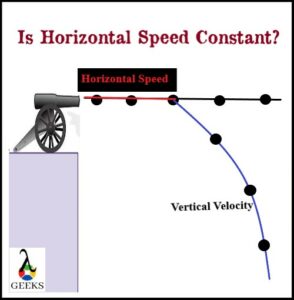
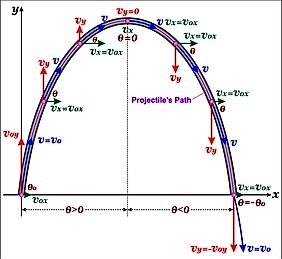
The horizontal speed is constant throughout the path as no force acts on the projectile in the horizontal direction to alter its acceleration. At the same time, the projectile travels downwards due to the gravity force, which defines the projectile motion along with constant horizontal speed.
Read more about Constant Horizontal Velocity.
The projectile travels vertically and horizontally at the exact time. Firstly, it happens because the air resistance force or air drag on the projectile is neglected as it is minimal. Hence, there is no force acting on the projectile in the horizontal direction to change its horizontal component of speed. As a result, the projectile traveled a constant horizontal distance (x) in unit time with constant horizontal speed (vH).
x = vHt …………. (*)
The horizontal speed equals the initial velocity u at the projectile launch. Hence,
vH = u …………… (1)
On the other hand, the gravity force, a non-contact force, always acts on the projectile after it is launched, changing its vertical speed component. That’s why the projectile accelerated at 9.8m/s2 downward, which changes its vertical speed. The vertical acceleration is the change in vertical speed (vV-uV) per unit time.

a =vV – uV/t
If we rearrange, we can obtain the kinematics equation of motion.
vV = uV + at ……………. (2)
Since the vertical component of speed uV is zero initially and acceleration(g) is driven by the gravity force,
vV = gt …………… (3)
If a cannonball fired from the cannon horizontally from the cliff, it takes 5 sec to reach the ground. Also, it falls on the ground at 20m from the base of the cliff. Calculate both the horizontal and vertical speeds of the cannonball after the fire?
Given:
t = 5s
x = 20m
g = 9.8m/s2
To Find:
- vH =?
- vV =?
Formula:
- x = vHt
- vV = uV + gt
Solution:
The horizontal speed is calculated as,
x = vHt
vH = x/t
Substituting all values,
vH = 20 / 5
vH = 4
The horizontal speed of the cannonball is 4m/s
The vertical speed is calculated from the kinematics equation of motion,
vV = uV + gt
Since initial vertical velocity is zero. i.e., uV = 0.
Substituting all values,
vV = 0 + 9.8 x 5
vV = 49
The vertical speed of the cannonball is 49m/s.
Read more about How to Calculate Momentum.
Why is Horizontal Speed Constant?
The horizontal speed is constant in projectile motion due to no forces in the horizontal direction.
The horizontal speed implies that the projectile moves in a straight path without changing its direction. No net forces are acting in a horizontal direction after the projectile is launched. Hence, the projectile’s horizontal speed stays constant throughout the trajectory motion due to zero acceleration.
In the previous article, we comprehended the difference between horizontal speed and horizontal velocity. In projectile motion, the velocity has horizontal and vertical components. The vertical velocity emerges due to gravity force that acts downward on the projectile.
In contrast, no forces act horizontally on the projectile, guiding the projectile to move with constant horizontal velocity. That’s the reason the projectile moves with both vertical and horizontal velocity at the same time after it is launched.
But just after launching the projectile, its vertical velocity is zero initially. Hence, the projectile initially moves with the constant horizontal speed in a linear path. Once the vertical velocity increases, changing the projectile’s direction, its horizontal speed becomes the horizontal velocity.
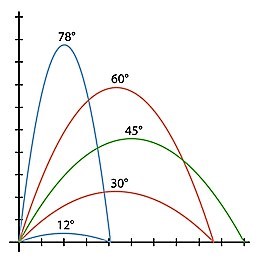
The conversion from horizontal speed to horizontal velocity depends on the launch angle of the projectile. Lesser the launch angle with horizontal, the projectile moves with constant horizontal speed more than horizontal velocity. If the launch angle increases, the horizontal speed becomes the horizontal velocity with an increase in vertical velocity.
Suppose a cannonball is a fire from a cannon located at the cliff’s edge. If we launch the cannonball at an angle of 0°, it moves in a straight direction with constant horizontal speed unless an external force stops it. Suppose we increase the launch angle to 30°, then its horizontal speed becomes horizontal velocity due to an increase in vertical velocity, and the cannonball starts to descend towards the ground.
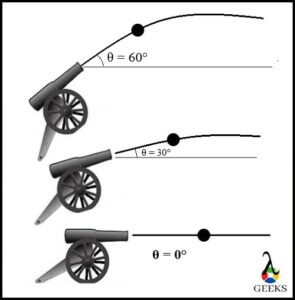
If the launch angle becomes 80°, then the cannonball moves with constant horizontal velocity, and it initially gets vertical velocity immediately after it launches. The cannonball moves upwards with both changing vertical velocity and constant horizontal velocity.
At maximum height, the vertical velocity becomes zero, at which the cannonball moves only the constant horizontal velocity. Below the maximum height, it gains the vertical velocity as it starts to move towards the ground, and hence, its acceleration is in the same direction as the gravity force.
Read more about Gravitational Acceleration.
A football moves 20m along the ground at 10 sec after a player kicks it. When a player kicks the football at 60°, it changes velocity from 5m/s to 10m/s; it reaches the ground in 5 sec after moving in a parabolic path. Calculate the horizontal speed and vertical velocity in both cases.
Given:
Case 1:
x = 20m
t = 10sec
Case 2:
θ = 60°
u = 5m/s
uV = 10m/s
t = 5sec
g = 9.8m/s2
To Find:
- vH =?
- vV =?
Formula:
- x = vHt
- vV = uV + gt
Solution:
1) As the football moves along the ground, the launch angle θ is zero.
Hence, in case 1, the football moves with constant horizontal speed, and the vertical velocity vV is zero.
The horizontal speed of football is calculated as,
x = vHt
vH = x/t
Substituting all values,
vH = 20/10
vH = 2
The football moves along the ground with a constant horizontal speed of 2m/s.
2) As the football launches at 60°, instead of horizontal speed, the football moves with a horizontal velocity equal to the initial velocity of football u = 5m/s.
The vertical velocity of the football is calculated as,
vV = uV + gt
Substituting all values,
vV = 10 + 9.8 x 5
vV = 10 + 49
vV = 59
The vertical velocity of the football launched at 60° is 59m/s.
How is Horizontal Speed Constant?
The horizontal speed is constant only when the object moves in a parabolic path.
The projectile motion in parabolic trajectory defined by horizontal and vertical velocity is only controlled by the downward gravity force, which sets up only vertical acceleration. Since there is no net force to set up the horizontal acceleration, the projectile moves with constant horizontal speed.
Read more about Relative Motion.
Earlier, we discussed the launch angle and horizontal speed relation. We will learn more about the time in which the projectile remains in the air. The best combination for the time in the air when the projectile moves with horizontal speed is launching at 45°, which provides the maximum horizontal distance to the projectile. The basketball and javelin thrower is a projectile motion example that proves this sentence true.
(credit: shutterstock)
We have determined earlier the formula about horizontal distance x. The vertical distance travelled by projectile is calculated using second kinematics equation of motion.
y = uyt + 1/2 gt2
But uV is zero.
y = gt2/2 ……….. (4)
Substituting value of t from equation (*), t = x/vx , we get
y = g(x/vx)2 / 2
y = gx/2vx2 ……………… (5)
The above equation is the parabola equation, which predicts how much the projectile travels vertical and horizontal after launch. It shows the parabolic trajectory of the projectile, which launched parallel to horizontal.
Let’s calculate the magnitude of the resultant velocity of the projectile using the Pythagoras theorem.
v2 =vx2 + vy2
From equation (1) and (4).
v= √(u2+g2t2) ……………. (6)
Now let’s calculate the formula about how the projectile remains in the air from the second kinematics equation of motion for vertical distance traveled (4),
t = √(2y/g) ………… (7)
That means, if we determine the time for a projectile in the air (t), we can also calculate the horizontal distance travelled (x) as the constant horizontal speed (vH) is the same as the initial velocity at which the projectile launched during the trajectory.
Therefore, the horizontal distance (x) by the projectile with constant horizontal speed (vH) is given by,
x = vHt
Using equation (1) and (7), we get
x = u √(2y/g) ………………(8)
(credit: shuttertstock)
Read more about How to Calculate Distance.
Suppose a basketball is thrown at angle 30°, which moves initially at 5m/s and then reaches the basket in 4sec after parabolic trajectory.
Calculate the vertical distance travelled by the basketball.
Calculate the horizontal distance travelled by the basketball.
Calculate the horizontal speed of the basketball.
Calculate the resultant velocity of the basketball.
Given:
θ = 30°
u = 5m/s
t = 4s
g = 9.8m/s2
To Find:
- y =?
- x =?
- vH =?
- v =?
Formula:
- y = gt2/2
- x = u √(2y/g)
- x = vHt
- v = √(u2+g2t2)
Solution:
1)The vertical distance travelled by the basketball is calculated as,
y =gt2/2
Substituting all values,
y = 9.8*42/2
y = 78.4
The vertical distance travelled by basketball, launched at 30°, is 78.4m.
2) The horizontal distance travelled by the basketball is calculated as,
x = u √(2y/g)
Substituting all values,
x = 5 √(2*74.8/9.8)
x = 5√15.26
x = 5×3.9
x = 19.5
The horizontal distance travelled by basketball, launched at 30°, is 19.5m.
3)The horizontal speed of basketball is calculated as,
x = vHt
vH = x/t
Substituting all values,
vH = 19.5/4
vH = 4.87
The basketball moves with a constant horizontal speed of 4.87m/s.
4)The resultant velocity of basketball is calculated as,
v = √(u2+g2t2)
Substituting all values,
v = √(52+9.82*42)
v = √(25+1536.64)
v = √1561.64
v = 39.51
The resultant velocity of a basketball, launched at 30°, is 39.51m/s.
Also Read:
- Friction and speed
- Instantaneous vs average speed
- Examples of speed in everyday life
- High speed camera lenses
- Dual speed focuser in telescopes
- How to calculate speed
- How to find orbital speed
- Instantaneous speed examples
- Constant speed examples
- Relationship between torque and speed

Hello, I’m Manish Naik completed my MSc Physics with Solid-State Electronics as a specialization. I have three years of experience in Article Writing on Physics subject. Writing, which aimed to provide accurate information to all readers, from beginners and experts.
In my leisure time, I love to spend my time in nature or visiting historical places.
Looking forward to connecting you through LinkedIn –