Dive into our example-rich guide on Tension Between Two Blocks, simplifying this key concept in physics for easy understanding
The tensional force acting on the string is not the same for all objects; it depends upon the mass and the acceleration of the object, and the force. Let us see how to find the tension between two blocks.
How to Find Tension between Two Blocks?
It is a force defined for strings, rope, or springs; tread like objects which experience tension on stretching.
The tension between two blocks can be found by knowing the net forces acting on the two blocks attached to the string, we can calculate the tension exerted on the string due to the two blocks.
Read more on 15 List of Examples of Tension Force.
Problem: Consider two blocks of masses ‘m1’ and ‘m2’ attached to a rope and suspended freely in the air. Calculate the tension imposed on the rope due to two blocks.
Solution: The tension felt on the rope is due to the blocks hanging on it, and relies upon the masses of the blocks.
Step1: Draw a free-body diagram for any problem
To calculate the tension on the rope, first draw the free body diagram understanding the problem, explaining the net forces acting on the blocks. Here, is a free body diagram of the two blocks for the above problem.
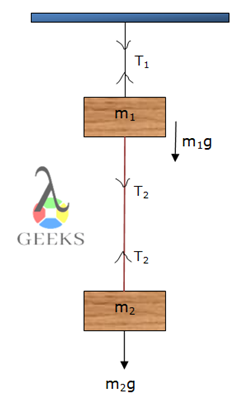
The diagram above gives us a rough idea of the tension generated in the rope due to two blocks. The tension T1 is due to the mass ‘m1’ and tension T2 is exerted due to the mass ‘m2’. The tension is felt across the length of the rope and in both the direction, in the positive y-axis and in the negative y-axis direction. The force due to gravity is acting downward due to both the weights is clearly indicated in the free-body diagram.
Step 2: Write the equation for net forces acting on each block.
The net force acting on the mass ‘m2’ is the tensional force and the force due to gravity acting downward in the negative y-direction. So, we have the equation as below,
F=T2-m2g
m2a=T2-m2g —-(1)
The net force acting on the mass ‘m1’ is the tensional force and weight acting downward in the negative y-direction. So, we can write the equation as,
F=T1 – T2 – m1g
m1a=T1 – T2 – m1g —(2)
Step 3: Frame the equation to find the net acceleration of the blocks.
The mass ‘m2’ is fixed and is not accelerating, hence a=0. Therefore we can write Eqn(1) as,
T2 – m2g=0—(3)
The mass ‘m1’ is also fixed at a point and is not accelerating, hence a=0. Therefore from Eqn(2), we have,
T1-T2-m2g=0 —(4)
It doesn’t mean that if there is no acceleration in the rope then there is no tension in the rope, this is evident from the above equation that there is a tension exerting in the rope due to each block. Let us see further how to find this tension in the rope.
Step 4: Calculate the total tension on the rope
From the Eqn(3), we have
T2 = m2g
The tension T2 is applicable due to mass ‘m2’ and the acceleration due to gravity, which is equal to the weight of block 2.
From eqn (4) we have,
T1 = T2 + m2g
Substituting the value to T2, we now have,
T1 = m1g + m2g
So, T1=g(m2+m1)
The tension T1 is due to the total mass attached to the string, as the rope exerting tension T1 is exerting weight of both the blocks.
Step 5: Find the net tension experiencing on the rope
The net tension is the sum of all the tensions exerted on the rope. Hence, net tension T is equal to the addition of T1 and T2,
T=T1+T2
T=g(m2+m1)+m2g
T=m1g+2m2g
T=(m1+2m2)g
This is a net tension in the rope due to two blocks suspended vertically above the ground.
Tension between Two Blocks on an Incline
Now, that we know how to calculate the tension between two blocks in a vertical direction, let us also ponder upon how to measure tension between the two blocks on an inclined slope.
Read more on How to calculate tension force: Exhaustive Insight.
Problem: Consider two blocks of masses 30kg and 45kg inclined on the slope attached to string on a pulley. The inclination angle of the slope on which mass of block ‘m1’ lies is at angle 300, and the slope on which mass ‘m2’ relies is inclined at an angle 450. Calculate the tension exerted on a string.
Solution: First, let us draw a free-body diagram of the two blocks inclined on the plane of different angles.
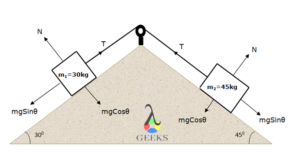
Now, write the equation for forces exerting on the blocks. The net force exerted on each body is the additional forces due to weight, gravity, the normal force which acts opposite to the weight of the body, and the tensional force exerted across the string.
The forces acting on mass m1 are in 2 directions, in the x-direction is, m1a=-m1gSin300+T, minus sign is because the force is acting in the negative x-axis; and in the y-direction is m1a=-m1gCos300+N.
The forces acting on mass m2 are, in the x-direction is, m2a=m2gSin450-T
The tension is exerted in the negative x-axis.
And, in the y-direction is m2a=-m2gcos450+ N
The tension comes into existence in the x-direction; hence we will consider 2 equations,
m1a=-m1gSin30°+T
m2a=m2gSin45°-T
Adding these two equations, we have,
m1a + m2a=m2gSin45°-T – m1gSin30°+T
Let us calculate acceleration of the blocks, so let’s substitute the value given.
(30+45)a=9.8* (45*1/√2)-30* (1/2)
75a=9.8* (22.5√2-15)
75a=9.8*(31.82-15)
a=9.8*16.82/75
a=2.2 m/s2
Now, we know the acceleration of the blocks. Substituting this in any of the above equations we can find the tension in the rope due to two blocks.
Consider the equation, m1a=-m1gSin30°+T
T=m1a+m1gSin30°
T=m1(a+gSin30°)
=30* (2.2+9.8*1/2)
=30* (2.2+4.9)
=30*7.1=213N
Hence, the tension in the rope is 213N.
Read more on How To Calculate Tension In A String:Exhaustive Insights.
Find Tension between Two Blocks on Horizontal Surface
The tension between the blocks placed on a horizontal surface comes into the picture when the pulling force is applied to one of the blocks. On pushing the object closer to each other or away from the other, the tension will be absent in the string joining the both.
Read more on Tension Force.
Problem: Consider two-block kept on a frictionless plane having mass m1=3kg and m2= 5kg. Both these masses are tied with a string, and a mass of 5kg is pulled in the positive x-direction applying a force of 230N. Measure the tension exerted on the string.
Solution: Let us draw a free body diagram considering the above situation,
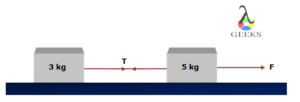
The tension acting on block 1 is equal to the force due to acceleration, given by the equation,
T=m1a=3a
The force applied on the block 2 is
F=T+m2a
Tension due to block 2 is
T=F-m2a
If we know the acceleration of the block, then it is easy to calculate the tensional force.
The net force applied on the blocks is
F= (m1+m2)a
Hence,a=F/m1+m2
From this, we can write the first equation as
T=3F/m1+m2
Hence, the tension exerted on the string is
T=3* 230/3+5
=3*230/8=86.25N
Tension of 86.25N is applied across the string.
Read more on Is tension a conservative force: Exhaustive Insight.
Frequently Asked Questions
A body of mass 2kg is attached to the string and is accelerating downward at a rate of 3m/s. What is tension on the string?
Given: m=2kg
a=3m/s
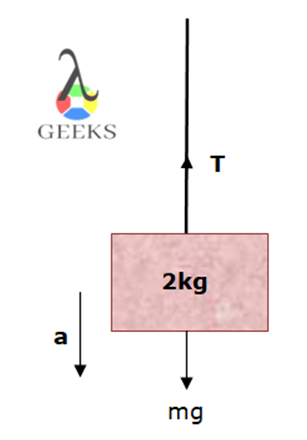
The force acting on the object is
F=T-mg
Since the acceleration of the body is downward F=-ma,
-ma=T-mg
T=m(g-a)
=2* (9.8-3)
=2*6.8=13.6N
The tension on a string is 13.6N.
What is a tension Force?
The force acting on every object is categorized depending upon the shape and size of the object and the direction of the force.
The tensional force is a contact force and acts on pulling the objects. The force acting across the length of the rope, strings, or springs is called the tension force.
Also Read:
Hi, I’m Akshita Mapari. I have done M.Sc. in Physics. I have worked on projects like Numerical modeling of winds and waves during cyclone, Physics of toys and mechanized thrill machines in amusement park based on Classical Mechanics. I have pursued a course on Arduino and have accomplished some mini projects on Arduino UNO. I always like to explore new zones in the field of science. I personally believe that learning is more enthusiastic when learnt with creativity. Apart from this, I like to read, travel, strumming on guitar, identifying rocks and strata, photography and playing chess.