Velocity

Relative velocity is a fundamental concept in physics that describes the velocity of one object with respect to another object. When two objects move in the same direction, the relative velocity is given by the difference in their velocities. This guide will provide you with a comprehensive understanding of this topic, including the underlying principles, mathematical formulas, practical examples, and real-world applications.

Understanding the Concept of Relative Velocity

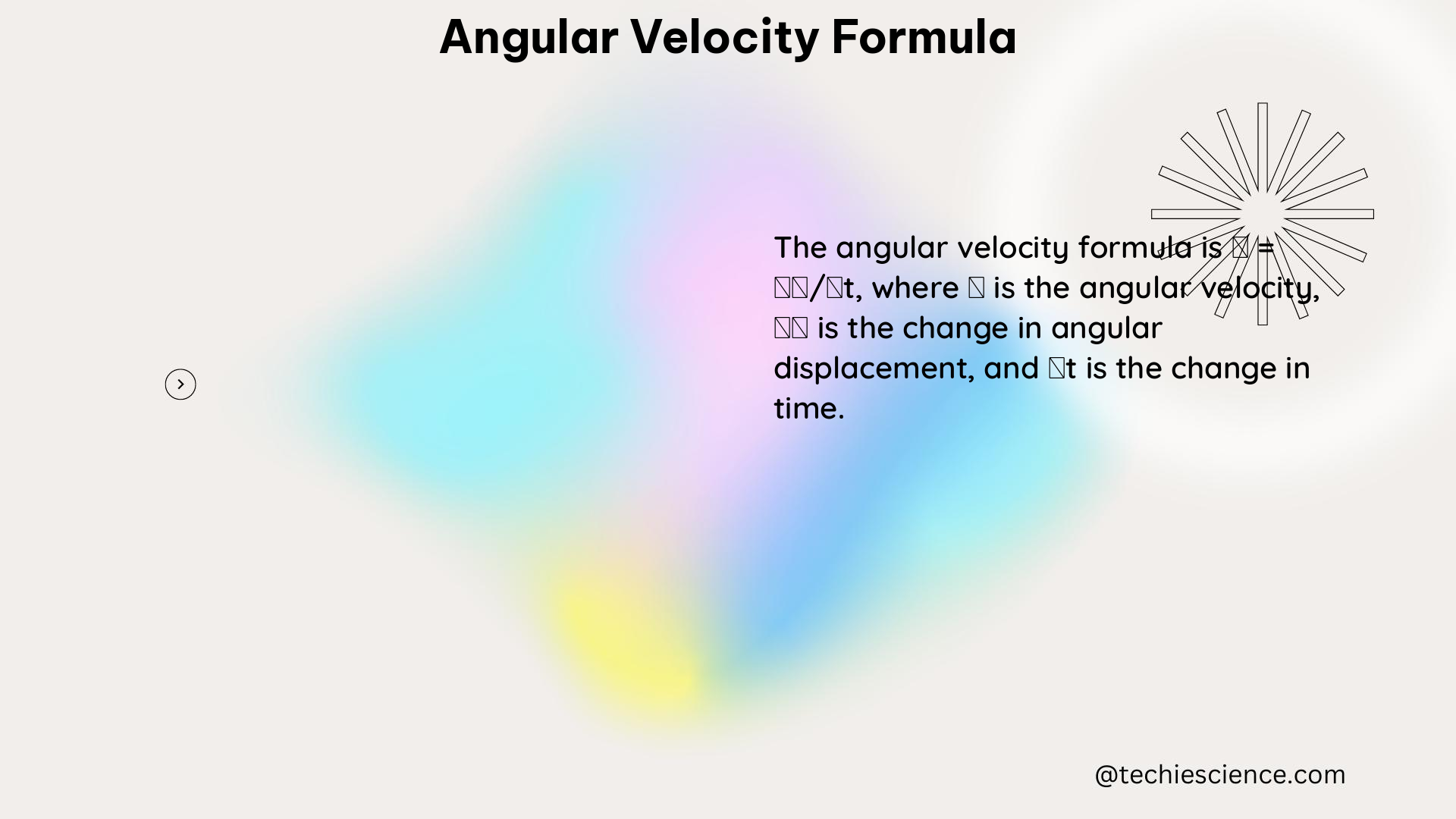
Relative velocity is the velocity of one object with respect to another object. When two objects are moving in the same direction, the relative velocity can be calculated using the following formula:

Relative velocity (Vab) = Velocity of object A (Va) – Velocity of object B (Vb)

The direction of the relative velocity is determined by the direction of the difference between the velocities of the two objects. If the velocity of object A is greater than the velocity of object B, then the relative velocity is in the same direction as the velocity of object A. If the velocity of object B is greater than the velocity of object A, then the relative velocity is in the opposite direction of the velocity of object A.

Theorem: Relative Velocity in the Same Direction

The theorem for relative velocity in the same direction can be stated as follows:

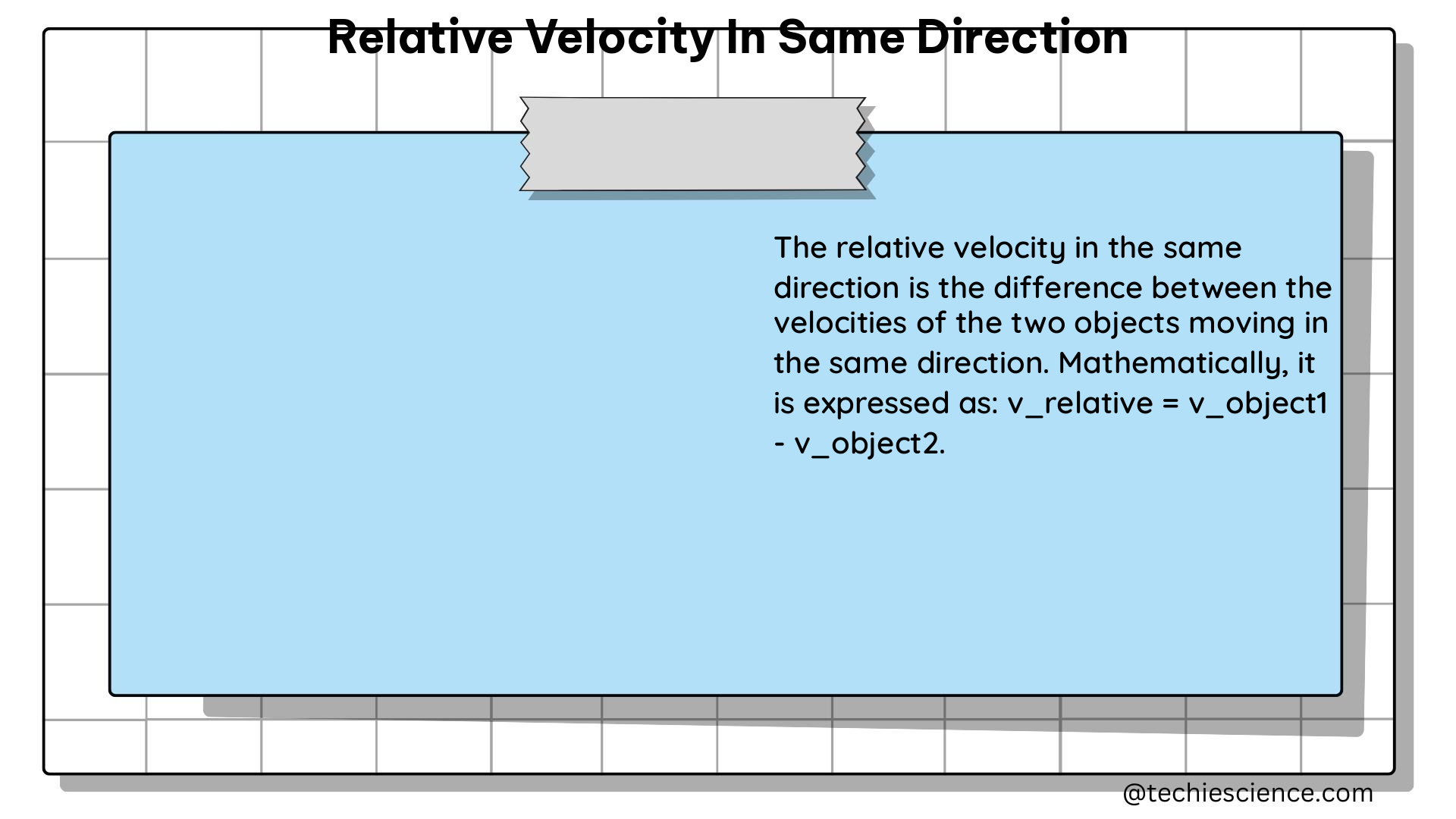
Theorem: When two objects are moving in the same direction, the relative velocity of object A with respect to object B is equal to the difference between the velocity of object A and the velocity of object B.

Mathematically, this can be expressed as:

Vab = Va – Vb

where:
– Vab is the relative velocity of object A with respect to object B
– Va is the velocity of object A
– Vb is the velocity of object B

Example: Relative Velocity of Cars on a Straight Road

Consider two cars, Car A and Car B, moving in the same direction on a straight road. Let’s assume the following velocities:

• Car A’s velocity (Va) = 60 mph
• Car B’s velocity (Vb) = 50 mph

Using the relative velocity formula, we can calculate the relative velocity of Car A with respect to Car B:

Vab = Va – Vb
Vab = 60 mph – 50 mph
Vab = 10 mph

In this case, the relative velocity of Car A with respect to Car B is 10 mph in the same direction as Car A.

Now, let’s consider a different scenario where Car B’s velocity is greater than Car A’s velocity:

• Car A’s velocity (Va) = 60 mph
• Car B’s velocity (Vb) = 70 mph

Applying the relative velocity formula:

Vab = Va – Vb
Vab = 60 mph – 70 mph
Vab = -10 mph

In this case, the relative velocity of Car A with respect to Car B is -10 mph, which means it is in the opposite direction of Car A’s velocity.

Physics Formulas and Equations

The relative velocity in the same direction can be expressed using the following physics formulas and equations:

1. Relative Velocity Formula:
   Vab = Va – Vb

2. Displacement Formula:
   Δs = Vab × Δt

where:
– Vab is the relative velocity of object A with respect to object B
– Va is the velocity of object A
– Vb is the velocity of object B
– Δs is the displacement of object A relative to object B
– Δt is the time interval

These formulas and equations are essential for understanding and applying the concept of relative velocity in the same direction.

Physics Numerical Problems

1. Problem: Two cars, Car A and Car B, are moving in the same direction on a straight road. Car A has a velocity of 80 km/h, and Car B has a velocity of 60 km/h. Calculate the relative velocity of Car A with respect to Car B.

Solution:
Given:
– Velocity of Car A (Va) = 80 km/h
– Velocity of Car B (Vb) = 60 km/h

Using the relative velocity formula:
Vab = Va – Vb
Vab = 80 km/h – 60 km/h
Vab = 20 km/h

Therefore, the relative velocity of Car A with respect to Car B is 20 km/h in the same direction as Car A.

1. Problem: A train is moving at a velocity of 120 km/h, and a car is moving in the same direction at a velocity of 90 km/h. Calculate the relative velocity of the train with respect to the car.

Solution:
Given:
– Velocity of the train (Va) = 120 km/h
– Velocity of the car (Vb) = 90 km/h

Using the relative velocity formula:
Vab = Va – Vb
Vab = 120 km/h – 90 km/h
Vab = 30 km/h

Therefore, the relative velocity of the train with respect to the car is 30 km/h in the same direction as the train.

These numerical problems demonstrate the application of the relative velocity formula in the same direction and provide a deeper understanding of the concept.

Practical Applications of Relative Velocity in the Same Direction

Relative velocity in the same direction has numerous practical applications in various fields, including transportation, space travel, and navigation.

Transportation

In transportation, directional velocity measurements are essential for ensuring safe and timely travel. Pilots and navigators use velocity measurements to determine the speed and direction of their aircraft or ship relative to the ground or the water. This information is crucial for determining the correct flight or navigation path and ensuring that the aircraft or ship reaches its destination on time.

For example, consider a commercial airliner flying at an altitude of 30,000 feet with a ground speed of 500 mph. If there is a strong tailwind blowing at 50 mph in the same direction as the aircraft, the relative velocity of the aircraft with respect to the ground would be 550 mph (500 mph + 50 mph). Knowing this relative velocity is essential for the pilot to accurately calculate the aircraft’s position, fuel consumption, and arrival time.

Space Travel

In space travel, the concept of relative velocity is also crucial for determining the trajectory of spacecraft, planets, and stars. By understanding the relative velocity of these objects, scientists can predict their movement and plan space missions accordingly.

For instance, when a spacecraft is launched into space, its velocity is measured relative to the Earth’s surface. As the spacecraft travels through space, its velocity is constantly changing due to the gravitational pull of other celestial bodies. By calculating the relative velocity of the spacecraft with respect to these bodies, scientists can accurately predict the spacecraft’s trajectory and make necessary adjustments to ensure a successful mission.

Navigation

Relative velocity measurements are also essential for navigation, both on land and at sea. Navigators use velocity measurements to determine the speed and direction of their vehicles relative to their surroundings, which is crucial for planning the most efficient and safe routes.

For example, in marine navigation, the relative velocity of a ship with respect to the water is used to calculate the ship’s speed over ground (SOG) and course over ground (COG). This information is essential for navigating through waterways, avoiding obstacles, and reaching the desired destination.

Conclusion

Relative velocity in the same direction is a fundamental concept in physics with numerous practical applications. By understanding the underlying principles, mathematical formulas, and real-world examples, you can develop a comprehensive understanding of this topic and apply it in various fields, such as transportation, space travel, and navigation.

Remember, the key to mastering relative velocity in the same direction is to practice solving numerical problems, understanding the physics formulas and equations, and applying the concept to real-world scenarios. With this knowledge, you’ll be well-equipped to tackle any challenges related to relative velocity in the same direction.

References

1. FasterCapital. Relative Velocity – FasterCapital. https://fastercapital.com/keyword/relative-velocity.html
2. Physics Stack Exchange. Doubt in negative sign of relative velocities of two objects in same directions. https://physics.stackexchange.com/questions/791019/doubt-in-negative-sign-of-relative-velocities-of-two-objects-in-same-directions
3. GeeksforGeeks. Relative Velocity Formula. https://www.geeksforgeeks.org/relative-velocity-formula/
4. OpenStax. 2.1 Relative Motion, Distance, and Displacement. https://openstax.org/books/physics/pages/2-1-relative-motion-distance-and-displacement
5. YouTube. Relative Velocity of a body moving in the same direction … – YouTube. https://www.youtube.com/watch?v=AFgNpH5RFjE

Relative velocity is defined as “the binary dissimilarity between the velocities of two figures or bodies: the velocity of a body about another considered as existence at rest- analogy corresponding motion. The velocity is determined within the gadget as per the spectator utilizing the relative velocity formula vAB=vA-vB in relativity.This post gives you a detailed explanation of such relative velocity examples.

• The motion of two cars
• A boat crossing a river
• A boat and the spectator
• The line joining two stationary objects
• Two fast-moving trains in opposite directions on the adjacent track
• A ball proceeds to the participant advancing against him
• A swimmer swimming across a river
• Flying the airplane and moving the car
• The pilgrim in a van
• The motion of the desk with a bundle of books
• Bird concerning the airplane
• Commuters on the giant dipper
• Parading
• Two friends strolling simultaneously
• Cyclist driving in the rain
• Snowboarding
• Windsurfing
• Geosynchronous equatorial orbit
• Reposing on a swing
• Gliding in chopper

The motion of two cars

Consider the two cars, A and B, traveling toward each other at disparate speeds. Both drivers since the other car traveling with a velocity or speed identical to the summation of the particular speeds. The two perspectives are from the side of the road, from the car A as if it were at rest, and from the car B as if it were at rest.

A boat crossing a river

A boat in a river travels among the river current, water traveling regarding the spectator on dry land. On such occasions as this, the immensity of the velocity of the traveling gadget regarding the spectator on land will be different from the tachometer reading of the vehicle.

A boat and the spectator

Suppose that the tachometer on the boat maybe 30 meters/hour; still, the boat may be traveling relative to the spectator on the seaside at a speed of 35meter/hour. Motion is correlative to the spectator. The speed of the spectator on land, generally named immobilized spectator, is disparate from that of the spectator.

The line joining two stationary objects

The spectator travels at a constant speed through the line joining the two stationary objects. The spectator will notice that the two objects have the same speed same velocity, and travel under the same supervision because the relative velocity of the two objects is zero.

Two fast-moving trains in opposite directions on the adjacent track

When the train in which the spectator is sitting is traveling with a velocity V1 of 20km/hr, another train is advancing against the first train with a velocity V2 of 40 km/hr. In this case, from the frame of reference of the spectator, it seems that the train advancing against him is traveling faster at a speed of 20+40=60 km/hr.

A ball proceeds to the participant advancing against him

For the participant who proceeds with the ball, the ball’s velocity will be the real velocity. Still, for a capturer advancing the ball, the velocity is higher than the real for a capturer. The relative velocity in his consciousness is the summation of the velocity of a ball and his streaming rapidity against the ball.

A swimmer swimming across a river

Suppose the sea crests are impending the swimmer, the relative velocity of the swimmer would be smaller than his real velocity. For a swimmer, it will emanate that swimmer is traveling quicker, but the rapidity of the crests diminishes the swimmer’s velocity.

Flying the airplane and moving a car

When we see the airplane through our moving car in the reverse direction, if it’s too distant, the airplane appears as it will stand for an observer in the car. The relative velocity of the observer in the car is more circular than his real velocity.

The pilgrim in a van

The pilgrim is deep-seated in a van in an immobilized situation, but the relative velocity of the pilgrim in a van is identical to the velocity of the bus. The pilgrim is traveling through the bus with rearrangement identical to the amount of rearrangement on the bus.

The motion of the desk with a bundle of books

The bundle of books is at ease, so the book’s velocity with the viewpoint on the desk is zero. But for the observers, the velocity of the books is correlative to the rapidity of the table.

Bird concerning the airplane

Suppose the birds fly relative to the airplane, and the passenger arrives to be immobilized by the bird. The bird seems to be immobilized by the passenger in the airplane because the bird has a definite velocity relative to that of the airplane, which is flying too. The bird and airplane fly in an identical coordinate system, called relative motion.

Commuters on the giant dipper

The commuter seated on the giant dipper will anticipate that the other commuters are immobilized and didn’t travel in his viewpoint. The relative velocity of the commuter from the viewpoint of other commuters is null. But the real rapidity of the commuter is relative to the rapidity of the giant dipper.

Parading

When the boy is parading in an association, the rapidity of the other boys in an association in his viewpoint is null due to all the boys traveling at a similar rapidity and therefore seem to be immobilized.

Two friends strolling simultaneously

Suppose the two friends are strolling simultaneously at a similar rapidity, then the relative velocity of the two friends about each other is to be null. Other humans, seeing them from afar, would notice the pragmatic velocity of the two friends.

Cyclist driving in the rain

Suppose the cyclist drives a cycle in the rain at a definite velocity. The cyclist would observe that the rapidity of the rain is higher than the real rapidity of the raindrops; from the cyclist’s viewpoint, the relative velocity of the raindrop is in addition to the cyclist’s velocity. Therefore, the cyclist senses that the rapidity of the rain is heavier than the real.

Snowboarding

The relative velocity of the snowboard in the viewpoint of the BomberBomber is null because the BomberBomber is upstanding upon the snowboard, which is traveling throughout with the BomberBomber. From the other viewpoint, the relative velocity of the BomberBomber is correlative to the snowboard.

Windsurfing

The surfer’s relative rapidity relies on the water current’s rapidity. Suppose the surfer travels under the supervision of the current. The relative rapidity of the surfer will be the summation of the rapidity of water circulate, and the surfer, in contradiction, supposes the surfer is moving in the supervision reverse to the flow of water. The correlative speed of the surfer would diminish.

Geosynchronous equatorial orbit

The geosynchronous equatorial orbits are kept at an elevation of almost 35,800 kilometers linearly about the middle circumference of the earth. Therefore, the rapidity of geosynchronous equatorial orbit from the viewpoint of the earth and equatorial orbit seems to be zero.

Reposing on a swing

The momentum of the person seated upon a swing is in relative locomotion with the fluctuating swing. A person is seated immobilized upon the swing, and their real velocity is zero. But the person is in fluctuation through the swing; the person’s velocity is identical to the velocity of the swing.

Gliding in chopper

The relative velocity of the person seated in a chopper is identical to the rapidity because the person is in an immobilized position.

From studying above mentioned examples, we finally concluded that the relative velocity of two objects is said to be the velocity of object A in conformity with object B or vice versa. Relative velocity is also utilized to determine the object’s velocity along with fluid. i.e., swimming, rowing.

Also Read:

• How to compute velocity in superstring theory
• How to calculate velocity in quantum computing
• How to find delta velocity
• How to find velocity at impact
• How to determine velocity in black hole
• How to calculate velocity in wormholes
• How to determine velocity in uniform circular motion
• Is angular velocity negative
• How to find time when velocity is zero 2
• How does position change velocity

Negative relative velocity is a fundamental concept in physics that describes the motion of one object relative to another, where the sign of the velocity indicates the direction of motion. Understanding this concept is crucial for various applications, from analyzing the motion of celestial bodies to designing efficient transportation systems. In this comprehensive guide, we will delve into the intricacies of negative relative velocity, providing you with a thorough understanding of the underlying principles, mathematical formulations, and practical applications.

Understanding the Basics of Relative Velocity

Relative velocity is the velocity of one object with respect to another. It is calculated by subtracting the velocity of the second object from the velocity of the first object. The sign of the relative velocity indicates the direction of motion of the first object relative to the second object.

If two objects are moving in the same direction, their relative velocity can be either positive or negative, depending on which object is moving faster. If the first object is moving faster than the second, the relative velocity will be positive, indicating that the first object is moving away from the second. Conversely, if the second object is moving faster than the first, the relative velocity will be negative, indicating that the second object is moving away from the first.

On the other hand, if two objects are moving in opposite directions, their relative velocity will always be negative, as the objects are moving away from each other.

Calculating Negative Relative Velocity

The formula for calculating the relative velocity of two objects, A and B, is:

$v_{AB} = v_A – v_B$

where:
– $v_{AB}$ is the relative velocity of object A with respect to object B
– $v_A$ is the velocity of object A
– $v_B$ is the velocity of object B

If the result of this calculation is negative, it indicates that object B is moving faster than object A in the same direction, or that the objects are moving in opposite directions.

Example 1: Negative Relative Velocity in the Same Direction

Consider two cars, A and B, traveling in the same direction on a highway. Car A has a velocity of 80 km/h, and Car B has a velocity of 60 km/h. The relative velocity of Car A with respect to Car B can be calculated as:

$v_{AB} = v_A – v_B = 80 \text{ km/h} – 60 \text{ km/h} = 20 \text{ km/h}$

Since the result is positive, it indicates that Car A is moving faster than Car B in the same direction.

Now, let’s consider the case where Car B is moving faster than Car A:

$v_{AB} = v_A – v_B = 60 \text{ km/h} – 80 \text{ km/h} = -20 \text{ km/h}$

The negative result indicates that Car B is moving faster than Car A in the same direction.

Example 2: Negative Relative Velocity in Opposite Directions

Consider two objects, A and B, moving in opposite directions. Object A has a velocity of 50 mph, and Object B has a velocity of -60 mph (i.e., Object B is moving in the opposite direction with a speed of 60 mph).

The relative velocity of Object A with respect to Object B can be calculated as:

$v_{AB} = v_A – v_B = 50 \text{ mph} – (-60 \text{ mph}) = 110 \text{ mph}$

The negative result indicates that Object A is moving away from Object B with a speed of 110 mph.

Practical Applications of Negative Relative Velocity

Negative relative velocity has numerous practical applications in various fields, including:

1. Astronomy and Astrophysics: Astronomers use the concept of negative relative velocity to study the motion of celestial bodies, such as stars, galaxies, and exoplanets, relative to each other. This information is crucial for understanding the structure and evolution of the universe.

2. Transportation and Navigation: In the field of transportation, negative relative velocity is used to analyze the motion of vehicles, such as cars, trains, and aircraft, relative to each other or to a fixed reference frame. This information is essential for designing efficient transportation systems, traffic management, and navigation.

3. Particle Physics: In particle physics, the concept of negative relative velocity is used to study the motion of subatomic particles, such as electrons and protons, relative to each other or to a fixed reference frame. This information is crucial for understanding the behavior of these particles and the fundamental laws of physics.

4. Robotics and Automation: In the field of robotics and automation, negative relative velocity is used to analyze the motion of robotic systems, such as manipulators and mobile robots, relative to their environment or to other objects. This information is essential for designing efficient and precise robotic systems.

5. Fluid Dynamics: In the study of fluid dynamics, negative relative velocity is used to analyze the motion of fluids, such as air and water, relative to solid objects or other fluids. This information is crucial for designing efficient and effective fluid systems, such as aircraft wings and hydroelectric turbines.

Numerical Problems and Exercises

To further solidify your understanding of negative relative velocity, let’s explore some numerical problems and exercises:

1. Two cars, A and B, are traveling on the same highway. Car A has a velocity of 90 km/h, and Car B has a velocity of 70 km/h. Calculate the relative velocity of Car A with respect to Car B.

2. An airplane is flying at a speed of 500 mph, and a strong wind is blowing in the opposite direction at a speed of 80 mph. Calculate the relative velocity of the airplane with respect to the wind.

3. A spaceship is traveling at a speed of 10,000 km/s, and a nearby asteroid is moving in the opposite direction at a speed of 5,000 km/s. Calculate the relative velocity of the spaceship with respect to the asteroid.

4. A boat is traveling upstream on a river with a velocity of 20 km/h, and the river has a current speed of 5 km/h. Calculate the relative velocity of the boat with respect to the river.

5. Two objects, A and B, are moving in the same direction. Object A has a velocity of 80 m/s, and Object B has a velocity of 100 m/s. Calculate the relative velocity of Object A with respect to Object B.

By working through these problems, you will gain a deeper understanding of the concept of negative relative velocity and its practical applications.

Conclusion

Negative relative velocity is a fundamental concept in physics that has numerous practical applications. In this comprehensive guide, we have explored the underlying principles, mathematical formulations, and practical applications of negative relative velocity. By understanding this concept, you will be better equipped to analyze the motion of various objects, from celestial bodies to transportation systems, and to design efficient and effective systems in a wide range of fields.

References

1. Doubt in negative sign of relative velocities of two objects in same direction, Physics Stack Exchange, https://physics.stackexchange.com/questions/791019/doubt-in-negative-sign-of-relative-velocities-of-two-objects-in-same-directions
2. Lab Report 1 – How to Quantify Motion, Studocu, https://www.studocu.com/en-us/document/university-of-maryland-baltimore/physical-chemistry/lab-report-1-how-to-quantify-motion/56608359
3. How do you acquire a target’s relative speed and distance?, Reddit, https://www.reddit.com/r/Kos/comments/15oo7oe/how_do_you_acquire_a_targets_relative_speed_and/
4. 2.1 Relative Motion, Distance, and Displacement, Physics | OpenStax, https://openstax.org/books/physics/pages/2-1-relative-motion-distance-and-displacement
5. Relative Wear, Altair, https://2022.help.altair.com/2022/EDEM/Creator/Physics/Additional_Models/Relative_Wear.htm

A relative velocity graph is a powerful tool used in physics to analyze and understand the motion of objects in different reference frames. It provides a graphical representation of the relative position and motion of two objects over time, allowing for a deeper understanding of the principles of relative motion. In this comprehensive guide, we will delve into the technical specifications, examples, formulas, and numerical problems associated with relative velocity graphs, equipping physics students with a thorough understanding of this essential concept.

Technical Specifications of Relative Velocity Graphs

1. Position-Time Graph: A relative velocity graph is a type of position-time graph that shows the relative position of two objects over time.
2. Relative Position: The relative position of two objects is the difference between their individual positions, calculated using the formula: Δx = x1 – x2, where Δx is the relative position, x1 is the position of the first object, and x2 is the position of the second object.
3. Slope of the Graph: The slope of the graph represents the relative velocity of the two objects. A steeper slope indicates a higher relative velocity, while a shallower slope indicates a lower relative velocity. The slope is calculated using the formula: m = Δy / Δx, where m is the slope, Δy is the change in position of the second object, and Δx is the change in position of the first object.
4. Intercepts: The intercepts of the graph represent the initial positions of the two objects.
5. Shape of the Graph: The shape of the graph can reveal important information about the motion of the two objects. A straight line indicates constant relative velocity, while a curved line indicates changing relative velocity.

Examples of Relative Velocity Graphs

1. Two Cars Moving in the Same Direction with Equal Velocities: The position-time graph of the two cars is a pair of parallel straight lines, indicating that the distance between the two cars remains constant over time.
2. Two Cars Moving in the Same Direction with Unequal Velocities: The position-time graph of the two cars is a pair of straight lines with different slopes, indicating that the distance between the two cars is changing over time.
3. Two Cars Moving in Opposite Directions: The position-time graph of the two cars is a pair of straight lines with opposite slopes, indicating that the distance between the two cars is increasing over time.

Physics Formulas

1. Relative Position: Δx = x1 – x2
2. Relative Velocity: Δv = v1 – v2
3. Slope of the Graph: m = Δy / Δx

Physics Numerical Problems

1. Problem 1: Two cars are moving in the same direction. Car A is moving at a velocity of 60 mph, and Car B is moving at a velocity of 80 mph. Calculate the relative velocity of the two cars.

2. Solution: Δv = v1 – v2 = 60 mph – 80 mph = -20 mph

3. Problem 2: Two cars are moving in opposite directions. Car A is moving at a velocity of 60 mph, and Car B is moving at a velocity of 80 mph. Calculate the relative velocity of the two cars.

4. Solution: Δv = v1 + v2 = 60 mph + 80 mph = 140 mph

Figures and Data Points

Figure 1: Relative velocity graph of two cars moving in the same direction with equal velocities.
Figure 2: Relative velocity graph of two cars moving in the same direction with unequal velocities.
Figure 3: Relative velocity graph of two cars moving in opposite directions.

Measurements and Quantifiable Details

1. Position: Measured in meters or feet
2. Velocity: Measured in meters per second or miles per hour
3. Time: Measured in seconds or minutes
4. Distance: Measured in meters or feet
5. Slope: Measured in meters per second or miles per hour

Reference Links

1. Relative Velocity Overview, Formulas & Equations – Study.com
2. Relative Motion in One and Two Dimensions – OpenStax
3. Relative Velocity and River Boat Problems – The Physics Classroom

In conclusion, relative velocity graphs are a crucial tool in the study of physics, providing a visual representation of the relative motion of objects in different reference frames. By understanding the technical specifications, examples, formulas, and numerical problems associated with relative velocity graphs, physics students can develop a deeper understanding of the principles of relative motion and apply this knowledge to solve complex problems in various fields of physics.

Relative angular velocity is a fundamental concept in physics that describes the rate of rotation of one object with respect to another. This measure is crucial in understanding the dynamics of rotating systems, from the Earth’s rotation to the motion of car wheels and spinning tops. In this comprehensive guide, we will delve into the intricacies of relative angular velocity, providing a wealth of technical details, formulas, examples, and numerical problems to help you gain a deep understanding of this essential topic.

Understanding Relative Angular Velocity

Relative angular velocity is the rate of change of the angular position of one object with respect to another. It is typically measured in radians per second (rad/s) or degrees per second (deg/s). This measure is crucial in understanding the dynamics of rotating systems, as it allows us to quantify the relative motion between two objects.

The formula for relative angular velocity is:

$\omega_{rel} = \omega_1 – \omega_2$

Where:
– $\omega_{rel}$ is the relative angular velocity
– $\omega_1$ is the angular velocity of the first object
– $\omega_2$ is the angular velocity of the second object

It’s important to note that the relative angular velocity can be positive or negative, depending on the direction of rotation of the two objects.

Measuring Relative Angular Velocity

Measuring relative angular velocity requires the use of specialized sensors and instruments. Here are some common methods and their associated data points:

Angular Velocity of the Earth

• The Earth rotates on its axis once every 24 hours, which means its angular velocity is approximately 0.0000727 rad/s.
• The angular velocity of the Earth relative to the Sun is about 0.0000102 rad/s, as the Earth orbits the Sun once every 365.25 days.

Angular Velocity of a Car Wheel

• The angular velocity of a car wheel depends on the speed of the car and the radius of the wheel.
• For example, if a car is moving at a speed of 60 km/h (16.67 m/s) and the radius of the wheel is 0.3 m, the angular velocity of the wheel is approximately 34.6 rad/s.

Angular Velocity of a Spinning Top

• The angular velocity of a spinning top can be measured using a high-speed camera and image processing software.
• For example, a top spinning at a rate of 10 revolutions per second has an angular velocity of approximately 628 rad/s.

Angular Velocity of a Gyroscope

• The angular velocity of a gyroscope can be measured using a variety of sensors, such as optical encoders, magnetometers, or accelerometers.
• For example, a gyroscope with a sensitivity of 1 degree per second (0.0175 rad/s) can measure angular velocities up to several hundred rad/s.

Calculating Relative Angular Velocity

To calculate the relative angular velocity between two objects, you can use the formula:

$\omega_{rel} = \omega_1 – \omega_2$

Here are some examples:

1. Example 1: A car is moving at a speed of 60 km/h (16.67 m/s) and the radius of the wheel is 0.3 m. The angular velocity of the wheel is 34.6 rad/s. The car is driving on a road that is rotating at an angular velocity of 0.0001 rad/s. Calculate the relative angular velocity between the wheel and the road.

Solution:
– Angular velocity of the wheel: $\omega_1 = 34.6$ rad/s
– Angular velocity of the road: $\omega_2 = 0.0001$ rad/s
– Relative angular velocity: $\omega_{rel} = \omega_1 – \omega_2 = 34.6 – 0.0001 = 34.5999$ rad/s

1. Example 2: A gyroscope has a sensitivity of 1 degree per second (0.0175 rad/s) and can measure angular velocities up to several hundred rad/s. If the gyroscope is measuring an angular velocity of 150 rad/s, what is the relative angular velocity between the gyroscope and the object it is measuring?

Solution:
– Angular velocity of the gyroscope: $\omega_1 = 150$ rad/s
– Angular velocity of the object: $\omega_2 = 0$ rad/s (assuming the object is stationary)
– Relative angular velocity: $\omega_{rel} = \omega_1 – \omega_2 = 150$ rad/s

1. Example 3: The Earth rotates on its axis once every 24 hours, and it orbits the Sun once every 365.25 days. Calculate the relative angular velocity between the Earth’s rotation and its orbit around the Sun.

Solution:
– Angular velocity of the Earth’s rotation: $\omega_1 = 0.0000727$ rad/s
– Angular velocity of the Earth’s orbit around the Sun: $\omega_2 = 0.0000102$ rad/s
– Relative angular velocity: $\omega_{rel} = \omega_1 – \omega_2 = 0.0000727 – 0.0000102 = 0.0000625$ rad/s

Relative Angular Velocity in Practical Applications

Relative angular velocity has numerous practical applications in various fields, including:

1. Robotics and Automation: Relative angular velocity is crucial in the control and navigation of robotic systems, allowing for precise control of rotational motion.
2. Aerospace Engineering: Relative angular velocity is essential in the design and control of aircraft, satellites, and spacecraft, where the accurate measurement of rotational motion is crucial for stability and navigation.
3. Mechanical Engineering: Relative angular velocity is used in the analysis and design of rotating machinery, such as gears, bearings, and turbines, to ensure efficient and reliable operation.
4. Biomechanics: Relative angular velocity is used to study the rotational motion of the human body, such as the movement of joints and limbs, which is essential for understanding and improving human performance and rehabilitation.
5. Geophysics: Relative angular velocity is used to study the Earth’s rotation and its interactions with other celestial bodies, which is crucial for understanding phenomena such as tides, precession, and nutation.

Numerical Problems and Exercises

To further solidify your understanding of relative angular velocity, here are some numerical problems and exercises for you to practice:

1. A car is moving at a speed of 80 km/h (22.22 m/s) and the radius of the wheel is 0.35 m. Calculate the angular velocity of the wheel and the relative angular velocity between the wheel and the road, assuming the road is stationary.

2. A gyroscope is mounted on a platform that is rotating at an angular velocity of 10 deg/s (0.1745 rad/s). The gyroscope has a sensitivity of 0.5 deg/s (0.0087 rad/s) and is measuring an angular velocity of 50 rad/s. Calculate the relative angular velocity between the gyroscope and the platform.

3. The Earth rotates on its axis once every 23 hours and 56 minutes (sidereal day), and it orbits the Sun once every 365.25 days. Calculate the relative angular velocity between the Earth’s rotation and its orbit around the Sun.

4. A spinning top is rotating at a rate of 15 revolutions per second. Calculate the angular velocity of the top and the relative angular velocity between the top and a stationary reference frame.

5. A robot arm has two joints, each with a different angular velocity. The first joint has an angular velocity of 2 rad/s, and the second joint has an angular velocity of 1.5 rad/s. Calculate the relative angular velocity between the two joints.

Remember to show your work and provide the final answers with the appropriate units.

Conclusion

Relative angular velocity is a fundamental concept in physics that is essential for understanding the dynamics of rotating systems. In this comprehensive guide, we have explored the intricacies of relative angular velocity, including its formula, measurement techniques, and practical applications. By working through the examples and numerical problems, you should now have a deeper understanding of this crucial topic and be well-equipped to apply it in various fields of study and real-world scenarios.

References

1. Relative angular velocity – Physics Stack Exchange
2. Moment of Inertia and Rotational Kinetic Energy – OpenStax
3. Angular Velocity – an overview | ScienceDirect Topics
4. Copy of Lady Bug Revolution.docx – Student Directions for…
5. Human Biomechanics – Rotation Angle and Angular Velocity

Relative velocity is a fundamental concept in physics that describes the motion of objects with respect to each other. It is defined as the ratio of the relative velocity of separation to the relative velocity of approach between the two objects. In the context of collisions, relative velocity is an important quantity to consider, especially in elastic collisions where both momentum and kinetic energy are conserved.

Understanding Relative Velocity in Elastic Collisions

In an elastic collision, the relative velocity has certain properties that hold true before and after the collision for any combination of masses. Specifically, the magnitude of the relative velocity is the same before and after the collision, while the relative velocity has opposite signs before and after the collision. This means that if we are sitting on one object moving at a certain velocity, the other object will appear to change direction after the collision, but its speed will remain the same as seen from the first object’s reference frame.

Relative Velocity Theorem

The Relative Velocity Theorem states that in an elastic collision, the relative velocity before the collision is equal in magnitude but opposite in direction to the relative velocity after the collision. Mathematically, this can be expressed as:

$\vec{v}{r,\text{before}} = -\vec{v}{r,\text{after}}$

where $\vec{v}{r,\text{before}}$ is the relative velocity before the collision and $\vec{v}{r,\text{after}}$ is the relative velocity after the collision.

Example: Elastic Collision Between Two Balls

Consider an elastic collision between two balls with masses $m_1$ and $m_2$, moving with initial velocities $\vec{v}_1$ and $\vec{v}_2$, respectively. The relative velocity before the collision is:

$\vec{v}_{r,\text{before}} = \vec{v}_1 – \vec{v}_2$

After the collision, the velocities of the two balls change to $\vec{v}_1’$ and $\vec{v}_2’$, respectively. The relative velocity after the collision is:

$\vec{v}_{r,\text{after}} = \vec{v}_1′ – \vec{v}_2’$

According to the Relative Velocity Theorem, the magnitude of the relative velocity is the same before and after the collision, but the direction is reversed:

$\vec{v}{r,\text{before}} = -\vec{v}{r,\text{after}}$

This means that if the relative velocity before the collision was directed towards the second ball, after the collision, it will be directed away from the second ball.

Calculating Mean Relative Velocity in Gas Collisions

When calculating the mean relative velocity between gas molecules in a collision mean-free path problem, the formula used is:

$\langle |v_r|\rangle =\sqrt2\langle |v|\rangle$

This formula is derived using the Maxwell-Boltzmann distribution of velocities, which describes the distribution of velocities for a large number of particles in a gas. The distribution is given by:

$B(v^2) = 4\pi\left(\frac{m}{2\pi k_BT}\right)^{3/2}v^2e^{-mv^2/2k_BT}$

where $m$ is the mass of the gas molecule, $k_B$ is the Boltzmann constant, and $T$ is the absolute temperature of the gas.

Since the molecules in the gas are independent, the distribution describing them is the product of the two independent distributions $B(v^2, v’^2)$. This leads to the formula for the mean relative velocity between gas molecules.

Example: Calculating Mean Relative Velocity in Argon Gas

Consider a sample of argon gas at a temperature of 300 K. The mass of an argon atom is $6.63 \times 10^{-26}$ kg. Using the formula for the mean relative velocity, we can calculate the value:

$\langle |v_r|\rangle =\sqrt2\langle |v|\rangle$

where $\langle |v|\rangle$ is the mean speed of the argon atoms, which can be calculated using the Maxwell-Boltzmann distribution:

$\langle |v|\rangle = \sqrt{\frac{8k_BT}{\pi m}}$

Plugging in the values, we get:

$\langle |v|\rangle = \sqrt{\frac{8 \times 1.38 \times 10^{-23} \text{ J/K} \times 300 \text{ K}}{\pi \times 6.63 \times 10^{-26} \text{ kg}}} = 402 \text{ m/s}$

Substituting this into the formula for the mean relative velocity, we get:

$\langle |v_r|\rangle =\sqrt2 \times 402 \text{ m/s} = 568 \text{ m/s}$

So, the mean relative velocity between argon gas molecules at 300 K is approximately 568 m/s.

Relative Velocity in Inelastic Collisions

While the Relative Velocity Theorem holds true for elastic collisions, the relationship between the relative velocities before and after the collision is different for inelastic collisions. In an inelastic collision, the relative velocity after the collision is not simply the negative of the relative velocity before the collision.

In an inelastic collision, the relative velocity after the collision is given by:

$\vec{v}_{r,\text{after}} = \frac{m_1\vec{v}_1′ + m_2\vec{v}_2′}{m_1 + m_2} – \frac{m_1\vec{v}_1 + m_2\vec{v}_2}{m_1 + m_2}$

where $\vec{v}_1’$ and $\vec{v}_2’$ are the final velocities of the two objects after the collision, and $\vec{v}_1$ and $\vec{v}_2$ are the initial velocities before the collision.

This formula takes into account the fact that in an inelastic collision, the final velocities of the two objects are not simply the negative of their initial velocities, as in the case of an elastic collision.

Conclusion

Relative velocity is a fundamental concept in physics that describes the motion of objects with respect to each other. In the context of collisions, relative velocity is an important quantity to consider, especially in elastic collisions where both momentum and kinetic energy are conserved.

The Relative Velocity Theorem states that in an elastic collision, the relative velocity before the collision is equal in magnitude but opposite in direction to the relative velocity after the collision. This property holds true for any combination of masses.

When calculating the mean relative velocity between gas molecules in a collision mean-free path problem, the formula used is $\langle |v_r|\rangle =\sqrt2\langle |v|\rangle$, which is derived using the Maxwell-Boltzmann distribution of velocities.

While the Relative Velocity Theorem holds true for elastic collisions, the relationship between the relative velocities before and after the collision is different for inelastic collisions, where the final velocities of the two objects are not simply the negative of their initial velocities.

Understanding the concept of relative velocity and its properties in different types of collisions is crucial for analyzing and predicting the behavior of objects in various physical systems.

Reference Links:

1. How to work out the relation between the “mean relative speed” and the “mean speed” in a gas?
2. Relative Velocity – FasterCapital
3. Elastic Collisions: Bouncing Back with Momentum