Physics

Homeostasis is a fundamental concept in biology, describing the ability of living organisms to maintain a stable internal environment despite changes in external conditions. This concept can be understood through the lens of dynamic equilibrium, which is a state where opposing forces or processes balance each other out, resulting in a relatively constant overall state. In the context of homeostasis, this dynamic equilibrium is achieved through various physiological mechanisms that work to counteract disturbances and maintain the body’s internal parameters within a narrow, optimal range.

Understanding Homeostasis as a Dynamic Equilibrium

Integrative Physiology Perspective

From an integrative physiology standpoint, homeostasis is viewed as a key goal that drives various body processes. The body’s internal environment is constantly subjected to external and internal perturbations, such as changes in temperature, pH, or nutrient levels. To maintain homeostasis, the body employs a complex network of regulatory mechanisms that work together to detect these changes and initiate appropriate responses to restore the desired state.

Systems Biology Perspective

In the systems biology approach, homeostasis is considered an emergent mechanistic fact, where the overall stability of the system arises from the intricate interactions between its various components. These components, which include sensors, controllers, and effectors, work together to form a feedback control system that maintains the dynamic equilibrium of the body’s internal environment.

Evolutionary Biology Perspective

From an evolutionary biology perspective, homeostasis can be understood as a fundamental adaptation that has evolved to ensure the survival and reproduction of living organisms. By maintaining a stable internal environment, organisms are better equipped to withstand environmental stresses and fluctuations, allowing them to thrive and pass on their genetic information to future generations.

Quantifiable Perspective

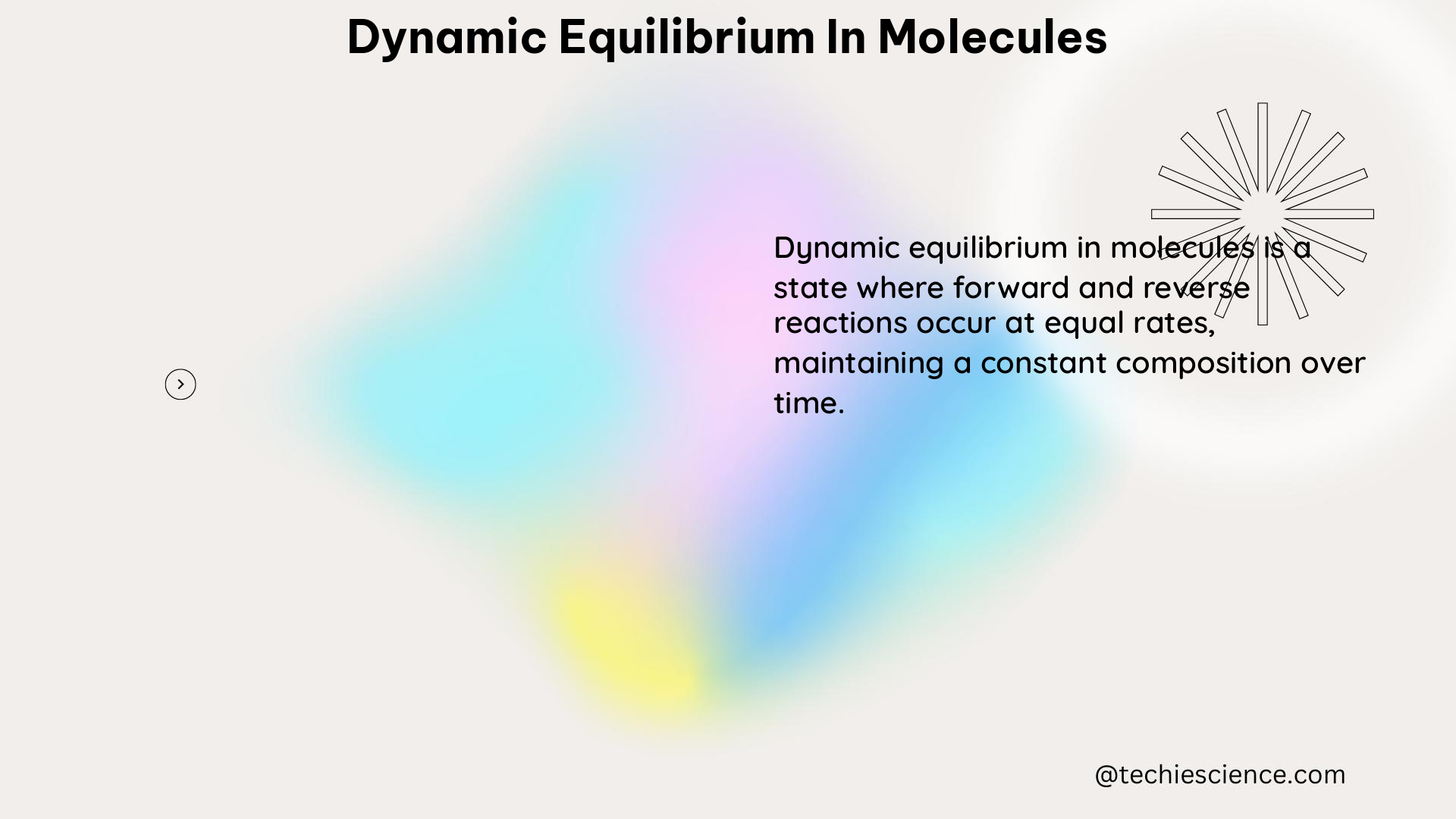
Homeostasis can be quantified and described using the concept of dynamic equilibrium. This equilibrium is achieved through internal control mechanisms that counteract external forces that could disrupt the body’s internal environment. These control mechanisms involve various physiological processes, such as the regulation of blood pressure, pH, and temperature, which work to maintain the desired state.

Mathematical Modeling of Homeostatic Equilibrium

Equations Representing Physiological Variables

Homeostasis can be described using mathematical equations that represent the relationships between various physiological variables. For example, the homeostatic equilibrium of a healthy organism can be studied using oppositely effective physiologic feedback signal-pairs, which can be represented mathematically. These equations can help researchers understand the underlying mechanisms and dynamics of the homeostatic system.

Probability Distribution Functions

In addition to using equations, the probability distribution function of the vectors in the homeostatic system can be used to determine quantities within the system. This approach can provide insights into the statistical properties of the homeostatic system and how it responds to perturbations.

Homeostasis and Feedback Control Systems

Feedback Control Theory

From a physics perspective, homeostasis can be understood through the concept of feedback control systems. These systems are designed to maintain a stable state in the presence of external disturbances. In the context of homeostasis, the body acts as a feedback control system, using sensors, controllers, and effectors to maintain a stable internal environment.

Control Theory and Mathematical Models

Control theory involves the use of mathematical models to predict and control the behavior of a system. In the case of homeostasis, control theory can be applied to describe the body’s regulatory mechanisms and how they work to maintain the dynamic equilibrium of the internal environment.

Practical Applications and Examples

Regulation of Blood Pressure

One example of homeostasis as a dynamic equilibrium is the regulation of blood pressure. The body’s cardiovascular system uses a feedback control system to maintain blood pressure within a narrow, optimal range. This involves sensors that detect changes in blood pressure, controllers that process this information, and effectors (such as the heart and blood vessels) that adjust the flow of blood to restore the desired pressure.

Regulation of Body Temperature

Another example is the regulation of body temperature. The body’s thermoregulatory system uses a feedback control system to maintain a stable core temperature, even in the face of external temperature changes. This involves sensors that detect changes in temperature, controllers that process this information, and effectors (such as the sweat glands and blood vessels) that adjust heat production and dissipation to maintain the desired temperature.

Regulation of Blood pH

The regulation of blood pH is another example of homeostasis as a dynamic equilibrium. The body’s respiratory and renal systems work together to maintain a stable blood pH, even in the face of changes in metabolic processes that can alter the balance of acids and bases in the body.

Conclusion

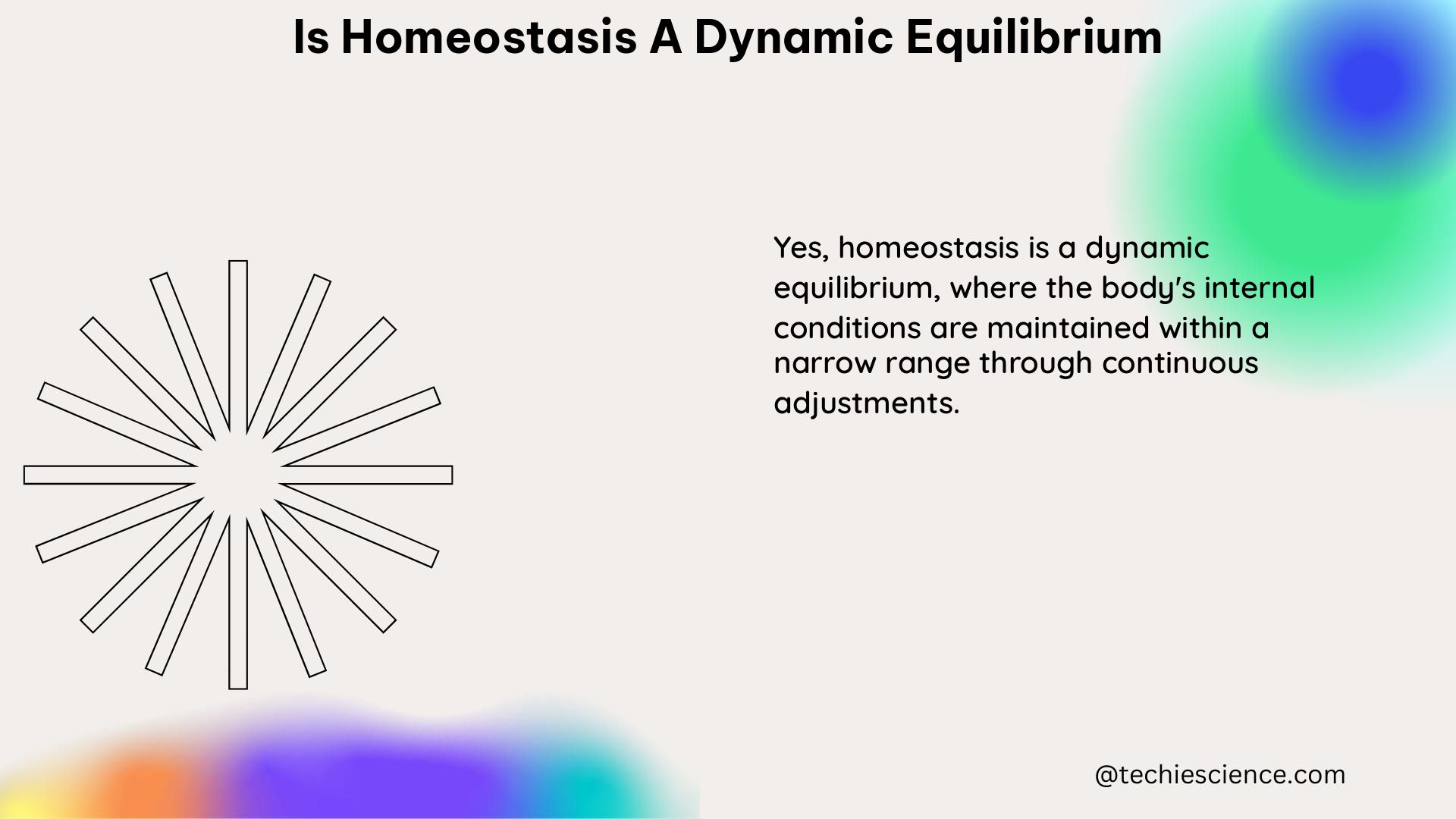
In summary, homeostasis is indeed a dynamic equilibrium, where the body’s internal environment is maintained within a narrow, optimal range through a complex network of regulatory mechanisms. This concept can be understood from various perspectives, including integrative physiology, systems biology, and evolutionary biology, and can be described using mathematical models and the principles of feedback control systems. By understanding the dynamic nature of homeostasis, we can gain deeper insights into the fundamental mechanisms that sustain life and adapt to changing environmental conditions.

References:
1. Goldstein, D. S. (2020). How does homeostasis happen? Integrative physiological, systems biological, and evolutionary perspectives. American Journal of Physiology-Regulatory, Integrative and Comparative Physiology, 318(4), R867-R892.
2. Goldstein, D. S. (2019). On the dynamic equilibrium in homeostasis. Frontiers in Endocrinology, 10, 311.
3. University of California, Santa Barbara. (n.d.). Control Theory and Feedback Mechanisms. Retrieved from https://www.control.engineering.ucsb.edu/
4. Encyclopædia Britannica. (n.d.). Homeostasis. Retrieved from https://www.britannica.com/science/homeostasis
5. Chemistry LibreTexts. (n.d.). Dynamic Equilibrium. Retrieved from https://chem.libretexts.org/Bookshelves/Physical_and_Theoretical_Chemistry_Textbook_Maps/Supplemental_Modules_(Physical_and_Theoretical_Chemistry)/Kinetics/Equilibrium/Dynamic_Equilibrium

Reaction and dynamic equilibrium refer to the state of a chemical system where the forward and reverse reactions occur at equal rates, resulting in no net change in the concentrations of reactants and products over time. This state is characterized by several measurable and quantifiable properties, which are crucial for understanding and predicting the behavior of chemical systems.

Constant Concentrations

At dynamic equilibrium, the concentrations of reactants and products remain constant over time. This means that the rate of the forward reaction is equal to the rate of the reverse reaction, resulting in no net change in the concentration of any species in the system. Consider the following reversible reaction:

[\text{A} + \text{B} \rightleftharpoons \text{C} + \text{D}]

At dynamic equilibrium, the concentrations of A, B, C, and D do not change over time, even though the reactions are still occurring in both directions. This can be expressed mathematically as:

[\frac{d[A]}{dt} = \frac{d[B]}{dt} = \frac{d[C]}{dt} = \frac{d[D]}{dt} = 0]

where [A], [B], [C], and [D] represent the concentrations of the respective species, and t is time.

Reaction Cross Section

The reaction cross section is a measure of the probability that two reactant particles will collide and undergo a reaction. At dynamic equilibrium, the reaction cross section remains constant, indicating that the rate of the forward reaction is equal to the rate of the reverse reaction. The reaction cross section can be expressed as:

[\sigma = \pi r^2]

where σ is the reaction cross section, and r is the effective radius of the reactant particles.

Rate Constants

The rate constants for the forward and reverse reactions are related to the reaction cross section and the concentrations of the reactants and products. At dynamic equilibrium, the rate constants for the forward and reverse reactions are equal, indicating that the rates of the forward and reverse reactions are equal. The rate constants can be expressed using the Arrhenius equation:

[k = A e^{-\frac{E_a}{RT}}]

where k is the rate constant, A is the pre-exponential factor, Ea is the activation energy, R is the universal gas constant, and T is the absolute temperature.

Equilibrium Constant

The equilibrium constant (Keq) is a measure of the ratio of the concentrations of products to reactants at dynamic equilibrium. It is defined as:

[K_{eq}=\dfrac{[C]^x[D]^y}{[A]^m[B]^n}]

where [A], [B], [C], and [D] are the concentrations of the reactants and products, and m, n, x, and y are the stoichiometric coefficients of the reaction. At dynamic equilibrium, the value of Keq remains constant, indicating that the concentrations of the reactants and products are in a fixed ratio.

The equilibrium constant can be calculated from the standard Gibbs free energy change (ΔG°) of the reaction using the following equation:

[K_{eq} = e^{-\frac{\Delta G^{\circ}}{RT}}]

where R is the universal gas constant, and T is the absolute temperature.

Temperature Dependence

The rate constants for the forward and reverse reactions are dependent on temperature, with higher temperatures generally leading to faster reaction rates. This is due to the increased kinetic energy of the reactant particles, which increases the probability of successful collisions.

However, the equilibrium constant (Keq) is independent of temperature, indicating that the ratio of the concentrations of products to reactants remains constant over a range of temperatures. This is because the temperature dependence of the forward and reverse rate constants cancels out in the expression for Keq.

The relationship between the equilibrium constant and temperature is given by the van ‘t Hoff equation:

[\frac{d\ln K_{eq}}{dT} = \frac{\Delta H^{\circ}}{RT^2}]

where ΔH° is the standard enthalpy change of the reaction.

Numerical Examples

1. Reversible Reaction: Consider the following reversible reaction at 25°C:

[\text{2NO} + \text{O}_2 \rightleftharpoons 2\text{NO}_2]

The equilibrium constant (Keq) for this reaction is 4.0 × 10^3. If the initial concentrations of NO and O2 are 0.10 M and 0.050 M, respectively, calculate the concentrations of all species at equilibrium.

Given:
– Keq = 4.0 × 10^3
– [NO]₀ = 0.10 M
– [O₂]₀ = 0.050 M

Using the equilibrium expression:

[K_{eq} = \frac{[NO_2]^2}{[NO]^2[O_2]}]

Let x be the change in concentration of each species at equilibrium. Then, the equilibrium concentrations are:
– [NO] = 0.10 – x
– [O₂] = 0.050 – x/2
– [NO₂] = x

Substituting these values into the equilibrium expression, we get:

[4.0 \times 10^3 = \frac{x^2}{(0.10 – x)^2(0.050 – x/2)}]

Solving this equation numerically, we find that x = 0.098 M. Therefore, the equilibrium concentrations are:
– [NO] = 0.10 – 0.098 = 0.002 M
– [O₂] = 0.050 – 0.098/2 = 0.001 M
– [NO₂] = 0.098 M

1. Temperature Dependence: Consider the following reversible reaction:

[\text{N}_2(g) + 3\text{H}_2(g) \rightleftharpoons 2\text{NH}_3(g)]

The standard enthalpy change (ΔH°) for this reaction is -92.4 kJ/mol. Calculate the change in the equilibrium constant (Keq) when the temperature is increased from 25°C to 50°C.

Given:
– ΔH° = -92.4 kJ/mol
– T₁ = 25°C (298 K)
– T₂ = 50°C (323 K)

Using the van ‘t Hoff equation:

[\frac{d\ln K_{eq}}{dT} = \frac{\Delta H^{\circ}}{RT^2}]

Integrating the equation between the two temperatures, we get:

[\ln\left(\frac{K_{eq,2}}{K_{eq,1}}\right) = \frac{\Delta H^{\circ}}{R}\left(\frac{1}{T_1} – \frac{1}{T_2}\right)]

Substituting the values, we get:

[\ln\left(\frac{K_{eq,2}}{K_{eq,1}}\right) = \frac{-92.4 \times 10^3 \text{ J/mol}}{8.314 \text{ J/(mol·K)}}\left(\frac{1}{298 \text{ K}} – \frac{1}{323 \text{ K}}\right)]

Solving this equation, we find that Keq,2 / Keq,1 = 0.67. This means that the equilibrium constant decreases by a factor of 0.67 when the temperature is increased from 25°C to 50°C.

These examples demonstrate the application of the principles of reaction and dynamic equilibrium to solve practical problems in chemistry. By understanding the relationships between the various properties, such as concentrations, rate constants, and equilibrium constant, chemists can predict and control the behavior of chemical systems at equilibrium.

References:

1. Atkins, P., & de Paula, J. (2014). Atkins’ Physical Chemistry (10th ed.). Oxford University Press.
2. Silbey, R. J., Alberty, R. A., & Bawendi, M. G. (2005). Physical Chemistry (4th ed.). Wiley.
3. Levine, I. N. (2009). Physical Chemistry (6th ed.). McGraw-Hill.
4. Chang, R., & Goldsby, K. A. (2013). Chemistry (11th ed.). McGraw-Hill.

Dynamic equilibrium in molecules is a state where the rate of the forward reaction is equal to the rate of the backward reaction, resulting in no net change in the concentrations of reactants and products. This equilibrium is dynamic, meaning that both reactions are ongoing, and the concentrations of reactants and products remain constant. The equilibrium constant (Keq) is a measure of the equilibrium position of a reaction and is defined as the ratio of the concentrations of products to reactants, raised to their stoichiometric coefficients, at equilibrium.

Understanding Dynamic Equilibrium

Dynamic equilibrium is a fundamental concept in chemistry and is essential for understanding the behavior of chemical systems. In a dynamic equilibrium, the forward and backward reactions are occurring simultaneously, but the net change in the concentrations of reactants and products is zero. This means that the system has reached a state of balance, where the rate of the forward reaction is equal to the rate of the backward reaction.

The concept of dynamic equilibrium can be expressed mathematically using the following equation:

Rate of forward reaction = Rate of backward reaction

At equilibrium, the concentrations of reactants and products remain constant, and the system is said to be in a state of dynamic equilibrium.

Equilibrium Constant (Keq)

The equilibrium constant (Keq) is a measure of the equilibrium position of a reaction and is defined as the ratio of the concentrations of products to reactants, raised to their stoichiometric coefficients, at equilibrium. The value of Keq is constant for a given reaction at a constant temperature and does not depend on the initial concentrations used to reach the point of equilibrium.

The equilibrium constant (Keq) is defined as:

Keq = [C]^c / ([A]^a * [B]^b)

where:
– [C] is the equilibrium concentration of the product(s)
– [A] and [B] are the equilibrium concentrations of the reactant(s)
– a, b, and c are the stoichiometric coefficients of the reactants and products, respectively

The value of Keq can be used to predict the direction of the reaction and the concentrations of reactants and products at equilibrium. If Keq is greater than 1, the reaction will favor the formation of products, and if Keq is less than 1, the reaction will favor the formation of reactants.

Factors Affecting Dynamic Equilibrium

Several factors can affect the dynamic equilibrium of a chemical system, including:

1. Temperature: The value of Keq is temperature-dependent, and changes in temperature can shift the equilibrium position of a reaction.
2. Pressure: Changes in pressure can affect the equilibrium position of a reaction, especially for reactions involving gases.
3. Concentration: Adding or removing reactants or products can shift the equilibrium position of a reaction, as described by Le Chatelier’s principle.
4. Catalysts: The presence of a catalyst can affect the rates of the forward and backward reactions, but it does not change the equilibrium position of the reaction.

Examples of Dynamic Equilibrium

1. Dissociation of Acetic Acid in Water:
2. Reaction: CH3COOH(aq) ⇌ CH3COO⁻(aq) + H⁺(aq)

3. Equilibrium constant: Keq = [CH3COO⁻][H⁺] / [CH3COOH]

4. Ionization of Ammonia in Water:

5. Reaction: NH3(aq) + H2O(l) ⇌ NH4⁺(aq) + OH⁻(aq)

6. Equilibrium constant: Keq = [NH4⁺][OH⁻] / [NH3]

7. Dissociation of Carbonic Acid in Water:

8. Reaction: H2CO3(aq) ⇌ HCO3⁻(aq) + H⁺(aq)

9. Equilibrium constant: Keq = [HCO3⁻][H⁺] / [H2CO3]

10. Dimerization of Nitrogen Dioxide:

11. Reaction: 2NO2(g) ⇌ N2O4(g)
12. Equilibrium constant: Keq = [N2O4] / [NO2]^2

These examples demonstrate the application of the equilibrium constant (Keq) in various chemical systems and how it can be used to predict the concentrations of reactants and products at equilibrium.

Numerical Problems and Calculations

1. Problem: Consider the reaction: 2NO(g) + O2(g) ⇌ 2NO2(g)
2. At equilibrium, the concentrations are: [NO] = 0.40 M, [O2] = 0.20 M, and [NO2] = 0.60 M.
3. Calculate the equilibrium constant (Keq) for this reaction.

Solution:
The equilibrium constant (Keq) is defined as:
Keq = [NO2]^2 / ([NO]^2 * [O2])
Substituting the given values:
Keq = (0.60)^2 / ((0.40)^2 * 0.20)
Keq = 0.36 / 0.032
Keq = 11.25

1. Problem: Consider the reaction: N2(g) + 3H2(g) ⇌ 2NH3(g)
2. At a certain temperature, the equilibrium constant (Keq) is 0.5.
3. If the initial concentrations are [N2] = 0.10 M and [H2] = 0.30 M, calculate the equilibrium concentrations of N2, H2, and NH3.

Solution:
Let’s assume the change in concentrations is x.
Initial concentrations:
[N2] = 0.10 M
[H2] = 0.30 M
[NH3] = 0 M
At equilibrium:
[N2] = 0.10 – x
[H2] = 0.30 – 3x
[NH3] = 2x
Equilibrium constant:
Keq = [NH3]^2 / ([N2] * [H2]^3)
0.5 = (2x)^2 / ((0.10 – x) * (0.30 – 3x)^3)
Solving this equation, we get:
x = 0.0667 M
Equilibrium concentrations:
[N2] = 0.10 – 0.0667 = 0.0333 M
[H2] = 0.30 – 3 * 0.0667 = 0.1 M
[NH3] = 2 * 0.0667 = 0.1333 M

These examples demonstrate the application of the equilibrium constant (Keq) in solving numerical problems related to dynamic equilibrium in chemical systems.

Figures and Data Points

To further illustrate the concept of dynamic equilibrium in molecules, let’s consider the following figure and data points:

This figure represents the dynamic equilibrium of the reaction:

A(g) + B(g) ⇌ C(g) + D(g)

At equilibrium, the following data points are observed:

Parameter	Value
[A]	0.2 M
[B]	0.3 M
[C]	0.4 M
[D]	0.5 M
Keq	2.0

The equilibrium constant (Keq) for this reaction is calculated as:

Keq = [C] * [D] / ([A] * [B])
Keq = (0.4 * 0.5) / (0.2 * 0.3)
Keq = 2.0

This data demonstrates the relationship between the concentrations of reactants and products at equilibrium, as well as the value of the equilibrium constant (Keq) for this specific reaction.

Conclusion

Dynamic equilibrium in molecules is a fundamental concept in chemistry that describes the state where the rate of the forward reaction is equal to the rate of the backward reaction, resulting in no net change in the concentrations of reactants and products. The equilibrium constant (Keq) is a measure of the equilibrium position of a reaction and can be used to predict the direction of the reaction and the concentrations of reactants and products at equilibrium.

Understanding dynamic equilibrium and the factors that affect it is crucial for studying and analyzing various chemical systems, from simple acid-base reactions to complex industrial processes. By mastering the concepts and techniques presented in this comprehensive guide, you can develop a deep understanding of dynamic equilibrium in molecules and apply it to solve a wide range of problems in chemistry.

References

1. Studysmarter. (n.d.). Dynamic Equilibrium. Retrieved from https://www.studysmarter.co.uk/explanations/chemistry/physical-chemistry/dynamic-equilibrium/
2. GeeksforGeeks. (n.d.). Equilibrium in Chemical Processes. Retrieved from https://www.geeksforgeeks.org/equilibrium-in-chemical-processes/
3. LibreTexts. (n.d.). The Idea of Dynamic Chemical Equilibrium. Retrieved from https://chem.libretexts.org/Bookshelves/Introductory_Chemistry/Introductory_Chemistry/15%3A_Chemical_Equilibrium/15.03%3A_The_Idea_of_Dynamic_Chemical_Equilibrium

Dynamic equilibrium is a fundamental concept in chemistry and physics, describing a state where the rate of the forward reaction equals the rate of the backward reaction, resulting in constant concentrations of reactants and products. This intricate balance is governed by a set of principles and mathematical relationships that are crucial for understanding and predicting the behavior of chemical systems. In this comprehensive guide, we will delve into the technical details, formulas, and practical applications of dynamic equilibrium conditions.

Understanding the Principles of Dynamic Equilibrium

At the heart of dynamic equilibrium lies the principle of reversibility. In a reversible reaction, the forward and backward reactions occur simultaneously, with the rates of these processes being equal at equilibrium. This can be represented by the general equation:

A + B ⇌ C + D

where the forward reaction rate is equal to the backward reaction rate, resulting in a constant composition of the system.

The key properties of dynamic equilibrium include:

1. Constant Concentrations: The concentrations of reactants and products remain constant over time, as the forward and backward reaction rates are equal.
2. Constant Measurable Properties: Properties such as concentration, density, color, and pressure remain constant at a given temperature.
3. Reversibility: The reaction is reversible, with the forward and backward reactions occurring simultaneously.
4. Equilibrium Constant (Keq): The equilibrium constant, Keq, is a measure of the equilibrium position and is calculated as the ratio of the concentrations of products to reactants, raised to the power of their stoichiometric coefficients.

Equilibrium Constant (Keq) and Its Significance

The equilibrium constant, Keq, is a crucial parameter in understanding and predicting the behavior of a dynamic equilibrium system. It is defined as the ratio of the concentrations of the products raised to their stoichiometric coefficients, divided by the concentrations of the reactants raised to their stoichiometric coefficients.

For the general reaction:

aA + bB ⇌ cC + dD

The equilibrium constant, Keq, is calculated as:

Keq = [C]^c × [D]^d / ([A]^a × [B]^b)

where [A], [B], [C], and [D] represent the equilibrium concentrations of the respective species, and a, b, c, and d are their stoichiometric coefficients.

The value of Keq provides valuable insights into the equilibrium position of the reaction:

• A large Keq value (>> 1) indicates that the reaction favors the formation of products.
• A small Keq value (< 1) indicates that the reaction favors the formation of reactants.
• A Keq value of 1 indicates that the reaction is at equilibrium, with equal amounts of reactants and products.

The equilibrium constant is a powerful tool for predicting the direction of a reaction and the relative concentrations of reactants and products at equilibrium.

Factors Affecting Dynamic Equilibrium

The position of a dynamic equilibrium can be influenced by various factors, including temperature, pressure, and the addition or removal of reactants or products. These factors can be understood and predicted using the principles of Le Chatelier’s Principle.

1. Temperature: An increase in temperature will shift the equilibrium in the direction that absorbs heat (endothermic reaction), while a decrease in temperature will shift the equilibrium in the direction that releases heat (exothermic reaction).

2. Pressure: An increase in pressure will shift the equilibrium in the direction that reduces the total number of moles of gas, while a decrease in pressure will shift the equilibrium in the direction that increases the total number of moles of gas.

3. Addition or Removal of Reactants or Products: Adding a reactant or removing a product will shift the equilibrium in the direction that counteracts the change, while adding a product or removing a reactant will shift the equilibrium in the direction that counteracts the change.

These principles can be used to predict the direction of the shift in the equilibrium position and the resulting changes in the concentrations of reactants and products.

Practical Applications of Dynamic Equilibrium

Dynamic equilibrium conditions have numerous practical applications in various fields, including:

1. Chemical Processes: Understanding dynamic equilibrium is crucial in the design and optimization of chemical processes, such as the Haber process for the production of ammonia, the Contact process for the production of sulfuric acid, and the Solvay process for the production of sodium carbonate.

2. Biological Systems: Dynamic equilibrium plays a vital role in biological systems, such as the maintenance of pH in the human body, the transport of molecules across cell membranes, and the regulation of enzyme-catalyzed reactions.

3. Environmental Chemistry: Dynamic equilibrium principles are used to understand and predict the behavior of pollutants in the environment, such as the distribution of heavy metals in soil and water, the formation of acid rain, and the fate of organic compounds in aquatic ecosystems.

4. Materials Science: Dynamic equilibrium concepts are applied in the study of phase transitions, the formation of solid solutions, and the understanding of defects in crystalline materials.

5. Atmospheric Chemistry: Dynamic equilibrium is crucial in understanding the composition of the Earth’s atmosphere, including the formation and dissociation of ozone, the transport of greenhouse gases, and the dynamics of atmospheric reactions.

By understanding the principles of dynamic equilibrium, scientists and engineers can design more efficient and sustainable chemical processes, predict the behavior of complex systems, and develop innovative solutions to pressing environmental and technological challenges.

Numerical Examples and Problem-Solving Strategies

To solidify your understanding of dynamic equilibrium conditions, let’s explore some numerical examples and problem-solving strategies.

Example 1: Calculating the Equilibrium Constant (Keq)

Consider the following reversible reaction:

2NO(g) + Cl2(g) ⇌ 2NOCl(g)

At equilibrium, the concentrations of the reactants and products are:
[NO] = 0.10 M, [Cl2] = 0.050 M, and [NOCl] = 0.20 M.

Calculate the equilibrium constant, Keq, for this reaction.

Solution:
The equilibrium constant, Keq, is calculated as:

Keq = [NOCl]^2 / ([NO]^2 × [Cl2])
Keq = (0.20 M)^2 / ((0.10 M)^2 × 0.050 M)
Keq = 0.04 / 0.0005
Keq = 80

Therefore, the equilibrium constant, Keq, for this reaction is 80.

Example 2: Predicting the Direction of Reaction Shift

Consider the following reversible reaction:

N2(g) + 3H2(g) ⇌ 2NH3(g)

The reaction is initially at equilibrium, with the following concentrations:
[N2] = 0.20 M, [H2] = 0.60 M, and [NH3] = 0.10 M.

If the pressure is increased, predict the direction of the shift in the equilibrium position.

Solution:
To predict the direction of the shift, we can use Le Chatelier’s Principle.

The reaction has the following balanced equation:
N2(g) + 3H2(g) ⇌ 2NH3(g)

Increasing the pressure will shift the equilibrium in the direction that reduces the total number of moles of gas. In this case, the forward reaction (formation of NH3) reduces the total number of moles of gas, as 4 moles of reactants (N2 and 3H2) are converted to 2 moles of product (2NH3).

Therefore, increasing the pressure will shift the equilibrium in the forward direction, towards the formation of more NH3.

Example 3: Solving a Dynamic Equilibrium Problem

A mixture of H2(g) and I2(g) is allowed to reach equilibrium at a certain temperature, and the equilibrium concentrations are found to be:
[H2] = 0.10 M, [I2] = 0.10 M, and [HI] = 0.80 M.

Calculate the value of the equilibrium constant, Keq, for the reaction:
H2(g) + I2(g) ⇌ 2HI(g)

Solution:
The equilibrium constant, Keq, is calculated as:

Keq = [HI]^2 / ([H2] × [I2])

Substituting the given values:
Keq = (0.80 M)^2 / (0.10 M × 0.10 M)
Keq = 0.64 / 0.01
Keq = 64

Therefore, the equilibrium constant, Keq, for the given reaction is 64.

These examples demonstrate the application of the principles of dynamic equilibrium, including the calculation of the equilibrium constant and the prediction of the direction of reaction shifts. By mastering these concepts and problem-solving strategies, you can develop a deep understanding of dynamic equilibrium conditions and their practical implications.

Conclusion

Dynamic equilibrium is a fundamental concept in chemistry and physics, describing a state where the forward and backward reaction rates are equal, resulting in constant concentrations of reactants and products. Understanding the principles of dynamic equilibrium, the significance of the equilibrium constant (Keq), and the factors that affect the equilibrium position is crucial for analyzing and predicting the behavior of chemical systems.

By exploring the technical details, formulas, and practical applications of dynamic equilibrium conditions, you can develop a comprehensive understanding of this important topic. The examples and problem-solving strategies provided in this guide will help you apply the principles of dynamic equilibrium to a wide range of scenarios, from chemical processes to biological systems and environmental chemistry.

Mastering dynamic equilibrium conditions is an essential step in becoming a proficient physicist or chemist, as it underpins the understanding of many complex phenomena in the natural world. By continuing to explore and deepen your knowledge in this area, you will be well-equipped to tackle challenging problems, design innovative solutions, and contribute to the advancement of scientific knowledge.

References

1. A framework for quantifying deviations from dynamic equilibrium theory. (2021). Retrieved from https://www.researchgate.net/publication/355159538_A_framework_for_quantifying_deviations_from_dynamic_equilibrium_theory
2. Dynamic Equilibrium. (2013). Retrieved from https://www.unite.it/UniTE/Engine/RAServeFile.php/f/File_Prof/CHIARINI_2014/Equilibrio_Chimico.pdf
3. The Idea of Dynamic Chemical Equilibrium. (2022). Retrieved from https://chem.libretexts.org/Bookshelves/Introductory_Chemistry/Introductory_Chemistry/15:_Chemical_Equilibrium/15.03:_The_Idea_of_Dynamic_Chemical_Equilibrium
4. Identify the common property for a chemical reaction at dynamic equilibrium. (2022). Retrieved from https://byjus.com/question-answer/identify-the-common-property-for-a-chemical-reaction-at-dynamic-equilibrium/
5. Dynamic Equilibrium. (n.d.). Retrieved from https://www.studysmarter.co.uk/explanations/chemistry/physical-chemistry/dynamic-equilibrium/