magnetic

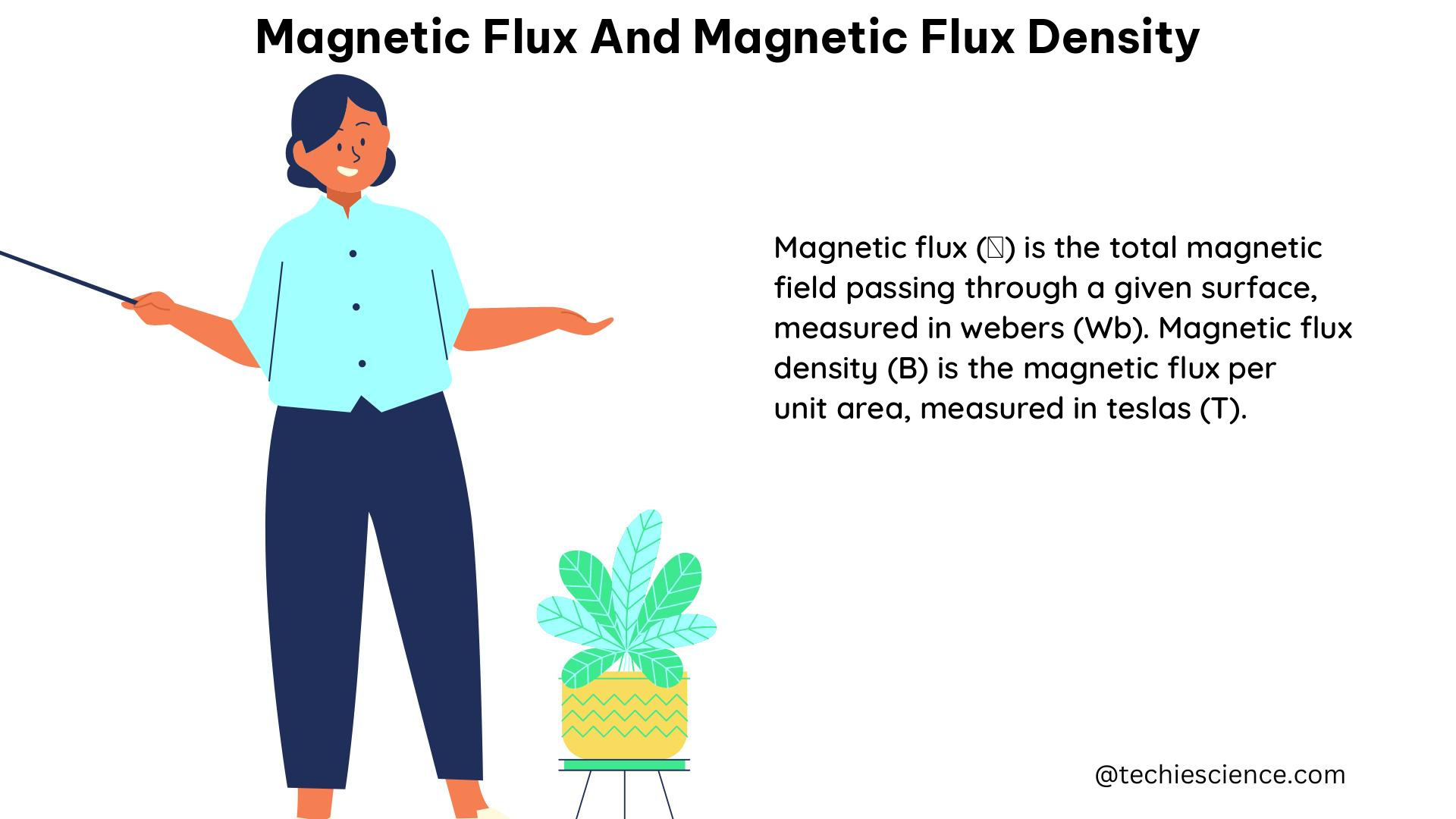
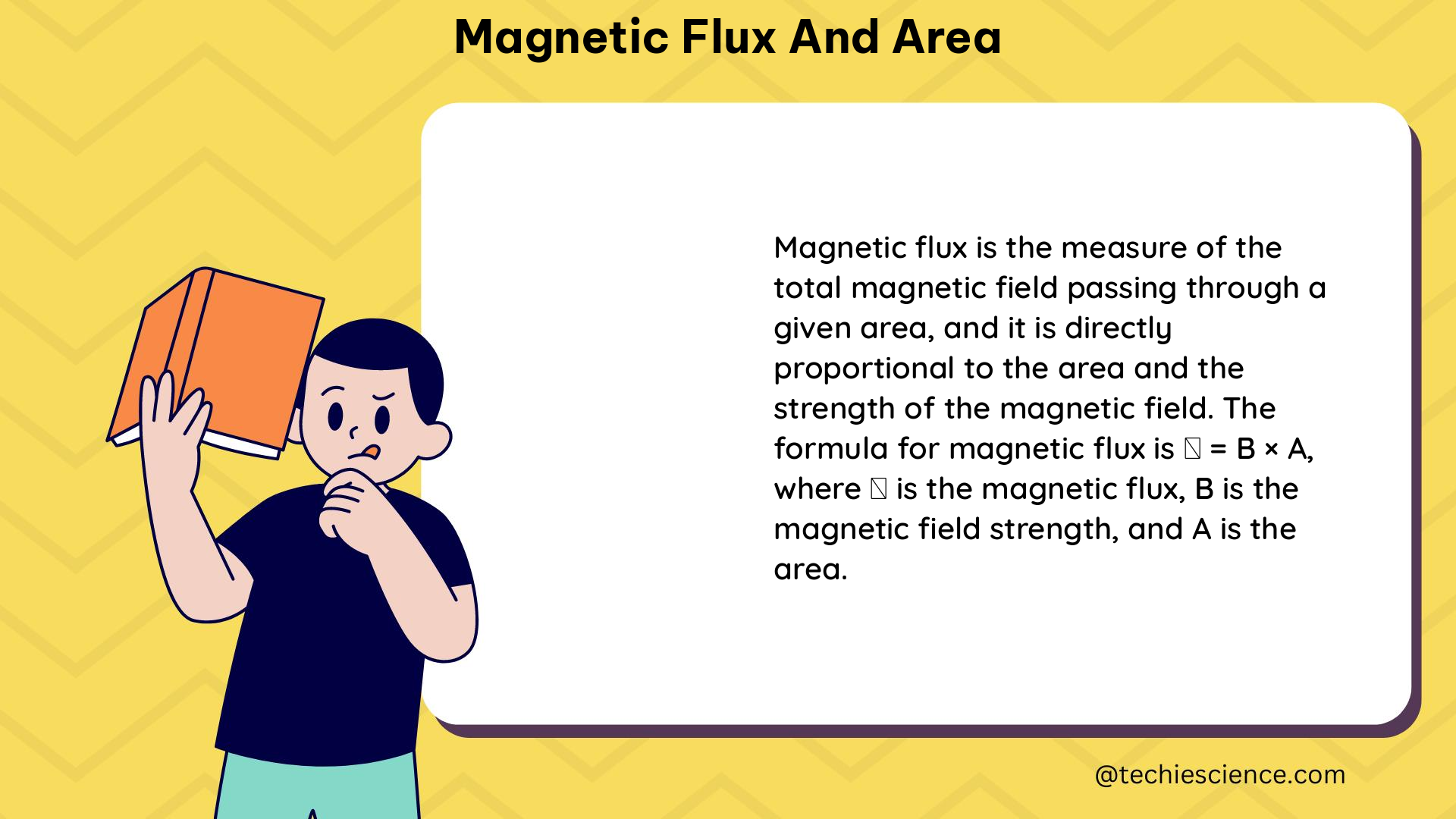
The Earth’s magnetic field is a complex and dynamic phenomenon that is constantly changing due to various factors. Understanding the nature of these changes is crucial for a wide range of applications, from navigation and space weather monitoring to geological investigations. In this comprehensive guide, we will delve into the intricacies of how the magnetic field changes, the methods used to measure it, and the implications of these changes.

Factors Influencing Magnetic Field Changes

The magnetic field is influenced by several factors, including:

1. Geographical Location: The Earth’s magnetic field varies significantly across different regions of the planet. This is due to the uneven distribution of magnetic materials within the Earth’s core and the complex interactions between the Earth’s internal and external magnetic sources.

2. Time: The Earth’s magnetic field is not static and undergoes continuous changes over time. These changes can occur on various timescales, from daily fluctuations to long-term reversals that happen every few hundred thousand years.

3. External Magnetic Sources: The presence of other magnetic fields, such as those generated by the Sun, can interact with the Earth’s magnetic field and cause it to change.

Measuring the Earth’s Magnetic Field

The Earth’s magnetic field is measured using a variety of techniques, including:

1. Magnetometers: These instruments measure the strength and direction of the magnetic field. They can be based on different principles, such as the Hall effect, fluxgate, or proton precession.

2. Magnetic Observatories: Approximately 200 magnetic observatories around the world continuously monitor the Earth’s magnetic field, providing valuable data for research and applications.

3. Satellite Measurements: Satellites equipped with magnetometers can measure the Earth’s magnetic field from space, providing a global perspective and insights into the field’s structure and dynamics.

The Earth’s magnetic field is described by seven parameters:

1. Declination (D): The angle between true north and magnetic north.
2. Inclination (I): The angle between the horizontal plane and the direction of the magnetic field.
3. Horizontal Intensity (H): The strength of the horizontal component of the magnetic field.
4. North (X) and East (Y) Components: The north and east components of the horizontal intensity.
5. Vertical Intensity (Z): The strength of the vertical component of the magnetic field.
6. Total Intensity (F): The overall strength of the magnetic field.

These parameters are used to characterize the Earth’s magnetic field and its changes over time.

Magnetic Field Reversals

The Earth’s magnetic field is not constant and can undergo reversals, where the north and south magnetic poles swap positions. These reversals are relatively rare, occurring every few hundred thousand years on average. During a reversal, the magnetic field intensity can decrease by as much as 90% at the Earth’s surface.

Magnetic field reversals are of great interest to scientists as they provide insights into the dynamics of the Earth’s core and the processes that generate the magnetic field. Understanding these reversals is also crucial for various applications, such as navigation and the study of the Earth’s geological history.

Measuring Magnetic Field Changes

The changes in the Earth’s magnetic field can be measured using a variety of techniques, including:

1. Hall Effect: The Hall effect is a phenomenon where a voltage difference is generated across a material when it is subjected to a magnetic field. By measuring this voltage difference, the strength of the magnetic field can be determined.

2. Fluxgate Magnetometers: Fluxgate magnetometers are sensitive instruments that can measure the strength and direction of the magnetic field. They work by detecting changes in the magnetic flux within a core material.

3. Proton Precession Magnetometers: These magnetometers measure the precession of protons in a magnetic field, which is directly related to the strength of the field.

4. Superconducting Quantum Interference Devices (SQUIDs): SQUIDs are highly sensitive magnetometers that can detect even the smallest changes in the magnetic field. They are often used in research and specialized applications.

Applications of Magnetic Field Measurements

The measurement and understanding of the Earth’s magnetic field changes have numerous applications, including:

1. Navigation: The magnetic field is used for navigation, both on land and at sea, as it provides a reference for determining direction.

2. Space Weather Monitoring: Monitoring the changes in the Earth’s magnetic field is crucial for understanding and predicting space weather, which can affect satellite operations, communication systems, and power grids.

3. Geological Investigations: Studying the Earth’s magnetic field can provide insights into the planet’s geological history, including the formation of continents and the movement of tectonic plates.

4. Mineral Exploration: Magnetic field measurements can be used to detect the presence of magnetic minerals, which can aid in the exploration and extraction of valuable resources.

5. Archeological Surveys: Magnetic field measurements can be used to detect and map buried archaeological features, such as ancient structures and artifacts.

Conclusion

The magnetic field is a dynamic and complex phenomenon that is constantly changing due to various factors. Understanding these changes and the methods used to measure them is crucial for a wide range of applications, from navigation and space weather monitoring to geological investigations and mineral exploration. By delving into the intricacies of magnetic field changes, physics students can gain a deeper understanding of this fundamental aspect of the physical world and its practical implications.

Reference:

1. Measuring Magnetic Fields | Science Project
2. How Do You Measure the Magnetic Field? – WIRED
3. Geomagnetism Frequently Asked Questions
4. Why measure the magnetic field at the Earth’s surface? Wouldn’t satellites be better suited for space-weather studies?
5. Magnetic Method | US EPA

Magnetic flux and magnetic flux density are fundamental concepts in electromagnetism that describe the behavior of magnetic fields. Magnetic flux is a scalar quantity that measures the amount of magnetic field crossing perpendicular to a certain surface, while magnetic flux density is a vector quantity that represents the force per unit of sensitive element.

Understanding Magnetic Flux

Magnetic flux, denoted by Φ or ΦB, is defined as the surface integral of the normal component of the magnetic field B over a given surface. Mathematically, it can be expressed as:

Φ = ∫∫ B⋅dA

Where:
– Φ is the magnetic flux (in webers, Wb)
– B is the magnetic field strength (in teslas, T)
– dA is the infinitesimal surface element (in square meters, m²)

The SI unit of magnetic flux is the weber (Wb), and the CGS unit is the maxwell (Mx). Magnetic flux is usually measured with a fluxmeter, which contains measuring coils, and it calculates the magnetic flux from the change of voltage on the coils.

Factors Affecting Magnetic Flux

The magnetic flux through a surface is influenced by several factors:

1. Magnetic Field Strength: The stronger the magnetic field, the greater the magnetic flux.
2. Surface Area: The larger the surface area, the greater the magnetic flux.
3. Angle of the Surface: The magnetic flux is maximized when the surface is perpendicular to the magnetic field (i.e., the angle between the surface normal and the magnetic field is 90 degrees).

Magnetic Flux Density

Magnetic flux density, also known as magnetic induction or magnetic field density, is a vector quantity that represents the force per unit of sensitive element, which in this case is a current. It is denoted by the symbol B and its SI unit is the tesla (T).

The magnetic flux density through a surface is proportional to the magnetic field strength and the number of turns in the coil, as described by the equation:

B = Φ / A

Where:
– B is the magnetic flux density (in teslas, T)
– Φ is the magnetic flux (in webers, Wb)
– A is the cross-sectional area of the surface (in square meters, m²)

Relationship between Magnetic Flux and Magnetic Flux Density

The relationship between magnetic flux and magnetic flux density is non-linear, history-dependent, and anisotropic. This means that the magnetic field strength and flux density are not always directly proportional, and their relationship can vary depending on the medium and the history of the magnetic field.

The magnetic flux density is a vector quantity, which means it has both magnitude and direction. The direction of the magnetic flux density is determined by the direction of the magnetic field lines, which can be visualized using iron filings or a compass.

Magnetic Flux Linkage

Magnetic flux linkage, denoted by Ψ, is a related concept that describes the total magnetic flux that links a coil or a circuit. It is defined as the product of the magnetic flux and the number of turns in the coil:

Ψ = N × Φ

Where:
– Ψ is the magnetic flux linkage (in weber-turns, Wb-turns)
– N is the number of turns in the coil
– Φ is the magnetic flux (in webers, Wb)

Magnetic flux linkage is an important concept in the analysis of transformers, generators, and other electromagnetic devices.

Practical Applications of Magnetic Flux and Magnetic Flux Density

Magnetic flux and magnetic flux density have numerous practical applications in various fields, including:

1. Electrical Machines: Magnetic flux and flux density are crucial in the design and operation of electrical machines, such as motors, generators, and transformers.
2. Magnetic Resonance Imaging (MRI): MRI technology relies on the manipulation of magnetic fields and the measurement of magnetic flux density to create detailed images of the human body.
3. Magnetic Sensors: Magnetic sensors, such as Hall effect sensors and magnetometers, measure the magnetic flux density to detect the presence, strength, and direction of magnetic fields.
4. Magnetic Recording: Magnetic recording technologies, such as hard disk drives and magnetic tape, use magnetic flux density to store and retrieve digital information.
5. Particle Accelerators: Particle accelerators, such as cyclotrons and synchrotrons, use magnetic fields and flux density to guide and control the motion of charged particles.

Numerical Examples and Problems

1. Example 1: A circular coil with 100 turns has a radius of 10 cm. If the magnetic flux through the coil is 0.5 Wb, calculate the magnetic flux density.

Given:
– Number of turns, N = 100
– Radius of the coil, r = 10 cm = 0.1 m
– Magnetic flux, Φ = 0.5 Wb

Calculation:
– Area of the coil, A = π × r² = π × (0.1 m)² = 0.0314 m²
– Magnetic flux density, B = Φ / A = 0.5 Wb / 0.0314 m² = 15.92 T

1. Example 2: A rectangular coil with 50 turns has dimensions of 20 cm × 30 cm. The coil is placed in a uniform magnetic field of 0.8 T, and the angle between the normal to the coil and the magnetic field is 45 degrees. Calculate the magnetic flux through the coil.

Given:
– Number of turns, N = 50
– Length, l = 20 cm = 0.2 m
– Width, w = 30 cm = 0.3 m
– Magnetic field strength, B = 0.8 T
– Angle between the normal to the coil and the magnetic field, θ = 45 degrees

Calculation:
– Area of the coil, A = l × w = 0.2 m × 0.3 m = 0.06 m²
– Magnetic flux, Φ = B × A × cos(θ) = 0.8 T × 0.06 m² × cos(45°) = 0.0339 Wb

1. Numerical Problem 1: A solenoid with 500 turns and a cross-sectional area of 0.02 m² is placed in a uniform magnetic field of 1.2 T. Calculate the magnetic flux through the solenoid.

2. Numerical Problem 2: A circular coil with 80 turns has a radius of 15 cm. If the magnetic flux density through the coil is 0.5 T, find the magnetic flux through the coil.

3. Numerical Problem 3: A rectangular coil with 100 turns has dimensions of 25 cm × 35 cm. The coil is placed in a uniform magnetic field of 0.6 T, and the angle between the normal to the coil and the magnetic field is 30 degrees. Calculate the magnetic flux linkage of the coil.

Figures and Data Points

1. Figure 1: Illustration of magnetic flux through a surface.
2. Figure 2: Relationship between magnetic flux, magnetic flux density, and the number of turns in a coil.
3. Table 1: Comparison of magnetic flux and magnetic flux density units in the SI and CGS systems.

1. Data Point 1: The Earth’s magnetic field has an average flux density of approximately 25 to 65 microteslas (μT) at the surface.
2. Data Point 2: The magnetic flux density in a typical household magnet is around 0.01 to 0.1 T.
3. Data Point 3: The magnetic flux density in a medical MRI machine can range from 1.5 to 3 T.

Conclusion

Magnetic flux and magnetic flux density are fundamental concepts in electromagnetism that are crucial for understanding the behavior of magnetic fields. By understanding the relationships between these quantities, as well as their practical applications and numerical examples, students and professionals can gain a deeper understanding of the principles of electromagnetism and their real-world applications.

References

1. Magnetic Flux – Wikipedia
2. Magnetic Flux Density – ScienceDirect
3. Magnetic Flux and Magnetic Flux Linkage – StudySmarter
4. Magnetic Flux Density – e-magnetica

Magnetic flux, represented by the symbol Φ, is a fundamental physical quantity that describes the amount of magnetic field passing through a given surface. The magnetic flux quantum, Φ₀, is a constant that represents the smallest possible change in magnetic flux, and it plays a crucial role in the understanding of superconductivity and the definition of electrical units in the International System of Units (SI).

Understanding Magnetic Flux

Magnetic flux is defined as the product of the magnetic field strength (B) and the area (A) over which the field is measured, as shown in the following equation:

Φ = B × A

The unit of magnetic flux is the weber (Wb), which is equivalent to tesla-square meter (T·m²).

Magnetic flux can be constant or variable, depending on the specific situation. For example, in a uniform magnetic field, the magnetic flux through a fixed area will remain constant. However, if the magnetic field or the area changes, the magnetic flux will also change accordingly.

The Magnetic Flux Quantum

The magnetic flux quantum, Φ₀, is a fundamental physical constant that represents the smallest possible change in magnetic flux. It is a crucial concept in the field of superconductivity and is defined as:

Φ₀ = h / (2e)

Where:
– h is the Planck constant (6.62607015 × 10⁻³⁴ J·s)
– e is the elementary charge (1.602176634 × 10⁻¹⁹ C)

The value of the magnetic flux quantum is approximately 2.067833848 × 10⁻¹⁵ Wb, or 2.067833848 × 10⁻⁷ Mx (where Mx is the unit of magnetic flux, the maxwell).

Importance of the Magnetic Flux Quantum

The magnetic flux quantum is a fundamental constant in the study of superconductivity and has several important applications:

1. Superconducting Quantum Interference Devices (SQUIDs): SQUIDs are highly sensitive magnetometers that can detect extremely small changes in magnetic flux, down to the level of the magnetic flux quantum. They are used in various applications, such as medical imaging, geophysical exploration, and fundamental physics research.

2. Quantum Hall Effect: The quantum Hall effect is a phenomenon observed in two-dimensional electron systems, where the Hall resistance takes on quantized values that are integer multiples of the ratio h/e², which is related to the magnetic flux quantum.

3. Josephson Effect: The Josephson effect describes the flow of current across a thin insulating barrier between two superconductors, and it is directly related to the magnetic flux quantum. This effect is used in the definition of the volt and the ampere in the SI system.

4. Fundamental Constants Measurement: Before the 2019 redefinition of the SI base units, the magnetic flux quantum was used to measure the Planck constant with high precision, which was then used to define the ampere and other electrical units.

Practical Applications and Numerical Examples

1. SQUID Magnetometers: SQUIDs can detect magnetic fields as small as 10⁻¹⁴ T, which corresponds to a change in magnetic flux of approximately 10⁻⁵ Φ₀. This sensitivity allows SQUIDs to be used in various applications, such as:
2. Measuring the magnetic fields of the human brain (magnetoencephalography)
3. Detecting the magnetic fields of the Earth’s crust (geophysical exploration)

4. Searching for dark matter (fundamental physics research)

5. Quantum Hall Effect: In the quantum Hall effect, the Hall resistance takes on quantized values given by the formula:

R_H = h / (n·e²)

Where:
– R_H is the Hall resistance
– h is the Planck constant
– e is the elementary charge
– n is an integer

For example, if n = 1, the Hall resistance would be approximately 25,812.807 Ω, which is a fundamental constant known as the von Klitzing constant.

1. Josephson Effect: The Josephson effect describes the flow of current across a thin insulating barrier between two superconductors. The voltage across the junction is related to the rate of change of the magnetic flux through the junction by the formula:

V = (Φ₀ / 2π) × (dΦ/dt)

Where:
– V is the voltage across the junction
– Φ₀ is the magnetic flux quantum
– dΦ/dt is the rate of change of the magnetic flux through the junction

This effect is used in the definition of the volt and the ampere in the SI system.

Conclusion

In summary, magnetic flux is a fundamental physical quantity that describes the amount of magnetic field passing through a given surface. The magnetic flux quantum, Φ₀, is a constant that represents the smallest possible change in magnetic flux and plays a crucial role in the understanding of superconductivity and the definition of electrical units in the SI system. The magnetic flux quantum has numerous practical applications, including in the development of highly sensitive magnetometers, the study of the quantum Hall effect, and the definition of electrical units.

References:

1. Magnetic Flux: Definition, Equation & Change | StudySmarter
2. Magnetic Induction (B) and Flux (F). Faraday’s Law explanation
3. Magnetic flux quantum – Wikipedia
4. Josephson effect – Wikipedia
5. Quantum Hall effect – Wikipedia
6. SQUID – Wikipedia

Magnetic flux is a crucial parameter in the operation of transformers, as it determines the efficiency and performance of these essential electrical devices. This comprehensive guide delves into the intricacies of magnetic flux in transformers, providing a detailed exploration of the underlying principles, mathematical relationships, and practical applications.

Understanding Magnetic Flux in Transformers

Magnetic flux, denoted by the symbol Φ (phi), is a measure of the strength of the magnetic field passing through a given area. In the context of transformers, the magnetic flux is directly proportional to the magnetic field strength (H) and the cross-sectional area (A) through which the field passes, as expressed by the formula:

Φ = H × A

The magnetic field strength, in turn, is related to the magnetomotive force (MMF) required to establish the magnetic flux in the core. This relationship is given by the equation:

H = N × I

where N is the number of turns in the winding, and I is the current flowing through the winding.

Ideal Transformer Assumptions

In an ideal transformer, the following assumptions are made:

1. The total magnetic flux is conserved, meaning that the sum of the fluxes in the individual windings determines the total magnetic flux (Φ) in the transformer core.
2. The voltage and current on each side of the transformer are inversely proportional to the turns ratio (N).

These assumptions allow for the derivation of the fundamental transformer equations, which relate the primary and secondary voltages and currents to the turns ratio.

Magnetic Flux Measurement Techniques

Accurately measuring the magnetic flux in a transformer is crucial for understanding its performance and efficiency. One novel test method for real-time magnetic flux measurement involves the use of a flux sensor integrated into the transformer core. This method has been modeled and tested, demonstrating accurate results.

Magnetic Field Evaluation

In addition to measuring the magnetic flux, evaluating the magnetic field patterns in transformers and inductors is essential for understanding and optimizing their performance. This analysis helps identify and mitigate issues such as leakage inductance and winding losses.

Magnetic Flux Calculations and Examples

To illustrate the practical application of magnetic flux in transformers, let’s consider a few examples:

Example 1: Calculating Magnetic Flux

Suppose a transformer has a magnetic field strength (H) of 1000 A/m and a cross-sectional area (A) of 0.01 m^2. Calculate the magnetic flux (Φ) in the transformer.

Given:
– Magnetic field strength (H) = 1000 A/m
– Cross-sectional area (A) = 0.01 m^2

Using the formula Φ = H × A, we can calculate the magnetic flux:
Φ = 1000 A/m × 0.01 m^2 = 10 Wb

Example 2: Determining Turns Ratio

A transformer has a primary winding with 500 turns and a secondary winding with 100 turns. Calculate the turns ratio and the relationship between the primary and secondary voltages and currents.

Given:
– Primary winding turns (N1) = 500
– Secondary winding turns (N2) = 100

Turns ratio = N1 / N2 = 500 / 100 = 5

In an ideal transformer, the voltage and current on each side are inversely proportional to the turns ratio. Therefore:
– Primary voltage (V1) / Secondary voltage (V2) = N1 / N2 = 5
– Primary current (I1) / Secondary current (I2) = N2 / N1 = 1/5

Example 3: Magnetic Flux Density and Transformer Efficiency

A transformer has a cross-sectional area of the core (A) of 0.05 m^2 and a maximum magnetic flux density (B) of 1.5 T. Calculate the maximum magnetic flux (Φ_max) and the relationship between the primary and secondary voltages.

Given:
– Cross-sectional area of the core (A) = 0.05 m^2
– Maximum magnetic flux density (B) = 1.5 T

Using the formula Φ = B × A, we can calculate the maximum magnetic flux:
Φ_max = 1.5 T × 0.05 m^2 = 0.075 Wb

In an ideal transformer, the primary and secondary voltages are proportional to the number of turns in each winding and the magnetic flux:
V1 / V2 = N1 / N2 = Φ_max(primary) / Φ_max(secondary)

Factors Affecting Magnetic Flux in Transformers

Several factors can influence the magnetic flux in a transformer, including:

1. Core Material: The type of core material, such as silicon steel or amorphous metal, can affect the magnetic properties and the maximum flux density.
2. Core Design: The shape and geometry of the transformer core can impact the magnetic flux distribution and leakage.
3. Winding Configuration: The arrangement and placement of the primary and secondary windings can influence the magnetic flux paths and the overall transformer efficiency.
4. Saturation: Exceeding the maximum flux density can lead to core saturation, which can cause increased losses and reduced transformer performance.

Understanding these factors is crucial for designing and optimizing transformers to achieve high efficiency and reliable operation.

Conclusion

Magnetic flux is a fundamental parameter in the operation of transformers, and its accurate measurement and analysis are essential for understanding and improving transformer performance. This comprehensive guide has provided a detailed exploration of the principles, calculations, and practical applications of magnetic flux in transformers, equipping you with the knowledge to tackle complex transformer-related challenges.

References

1. Magnetic flux and current directions of transformer – Physics Forums
2. Why we use constant value of magnetic flux in transformers
3. Magnetic Field Evaluation in Transformers and Inductors
4. A Novel Test Method for Real-time Magnetic Flux Measurement of Power Transformers
5. How to Solve Transformer Flux and Magnetic Circuits? – Study for FE

The magnetic field in a transformer is a crucial aspect of its operation, as it is responsible for the efficient transfer of electrical energy from the primary winding to the secondary winding. Understanding the behavior and characteristics of the magnetic field is essential for designing and optimizing transformer performance.

Magnetic Flux Density (B) and Faraday’s Law

The magnetic field in a transformer is often characterized by the magnetic flux density (B), which is the measure of the strength of the magnetic field passing through a given area. The magnetic flux density can be calculated using Faraday’s law of induction, which states that the voltage (V) induced in a coil is proportional to the rate of change of the magnetic flux (dΦ/dt) through the coil. The magnetic flux (Φ) is the product of the magnetic flux density (B) and the area (A) through which it passes, as expressed by the equation:

Φ = B × A

Faraday’s law of induction can be written as:

V = -N × (dΦ/dt)

where N is the number of turns in the coil.

Magnetic Field Generation in a Transformer

In a transformer, the primary winding generates a magnetic field when a current flows through it. This magnetic field induces a voltage in the secondary winding, which can be used to transfer power from the primary to the secondary winding. The magnetic flux density (B) in a transformer is determined by several factors:

1. Number of Turns in the Windings: The number of turns in the primary and secondary windings directly affects the magnetic flux density. Increasing the number of turns in the primary winding will increase the magnetic flux density, while increasing the number of turns in the secondary winding will decrease the magnetic flux density.

2. Current in the Primary Winding: The current flowing through the primary winding is another factor that determines the magnetic flux density. As the current increases, the magnetic flux density also increases, as described by the equation:

B = μ × N × I / l

where μ is the magnetic permeability of the core material, N is the number of turns in the primary winding, I is the current in the primary winding, and l is the length of the magnetic path.

1. Magnetic Properties of the Core Material: The magnetic properties of the core material, such as its permeability and saturation flux density, also play a crucial role in determining the magnetic flux density. Ferromagnetic materials, such as silicon steel, are commonly used in transformer cores due to their high permeability and low losses.

Measuring the Magnetic Field in a Transformer

The magnetic field in a transformer can be quantified by measuring the magnetic flux density (B) and the magnetic field strength (H) at various points within the transformer. Several techniques can be used for these measurements:

1. Hall Effect Sensors: Hall effect sensors measure the voltage generated by the magnetic field perpendicular to the direction of current flow in a conductive material. They can be used to measure the magnetic flux density (B) at specific locations within the transformer.

2. Fluxgate Magnetometers: Fluxgate magnetometers measure the magnetic field by detecting the distortion of a magnetic field produced by a coil wrapped around a magnetically permeable core. They can be used to measure both the magnitude and direction of the magnetic field.

3. Search Coils: Search coils measure the voltage induced in a coil by a changing magnetic field. They can be used to measure the rate of change of the magnetic flux (dΦ/dt), which can then be used to calculate the magnetic flux density (B) using Faraday’s law.

4. Induction Coils, Rogowski Coils, and Current Transformers: These devices can be used to measure the magnetic field strength (H) by measuring the current or voltage induced in the coils due to the changing magnetic field.

Magnetic Field Distribution and Visualization

In addition to measuring the magnetic field strength and flux density, it is also important to consider the distribution of the magnetic field within the transformer. The magnetic field distribution can be visualized using magnetic field plots, which show the magnetic field strength or flux density at various points within the transformer.

Magnetic field plots can be used to identify areas of high magnetic field strength or flux density, which can be a source of heat generation and energy loss in the transformer. These areas of high magnetic field can lead to increased core losses, eddy current losses, and hysteresis losses, all of which can reduce the overall efficiency of the transformer.

Frequency Dependence of the Magnetic Field

The magnetic field in a transformer can vary at different frequencies, depending on the operating conditions of the transformer. Measuring the magnetic field at different frequencies can help to identify any frequency-dependent effects, such as resonances or harmonic distortion.

For example, at higher frequencies, the skin effect and proximity effect can become more pronounced, leading to increased eddy current losses in the transformer windings and core. Measuring the magnetic field at these higher frequencies can help to quantify the impact of these effects and inform the design of the transformer to mitigate them.

Numerical Examples and Data Points

To illustrate the concepts discussed, let’s consider a few numerical examples and data points related to the magnetic field in a transformer:

1. Magnetic Flux Density Calculation: Consider a transformer with a primary winding of 500 turns and a secondary winding of 50 turns. If the primary winding carries a current of 10 A and the cross-sectional area of the core is 50 cm^2, the magnetic flux density can be calculated as:

B = μ × N × I / l
B = 4π × 10^-7 H/m × 500 × 10 A / 0.1 m
B = 0.314 T

1. Magnetic Field Strength Measurement: Using a Rogowski coil, the magnetic field strength (H) in a transformer core was measured to be 800 A/m. Assuming a relative permeability (μ_r) of the core material as 2000, the magnetic flux density (B) can be calculated as:

B = μ × H
B = 4π × 10^-7 H/m × 2000 × 800 A/m
B = 1.005 T

1. Magnetic Field Distribution: A magnetic field plot of a transformer core revealed that the magnetic flux density was highest near the corners of the core, reaching values up to 1.2 T. This uneven distribution of the magnetic field can lead to increased core losses and localized heating in the transformer.

2. Frequency Dependence: Measurements of the magnetic field in a transformer at different frequencies showed that the magnetic flux density decreased by 5% when the frequency was increased from 50 Hz to 400 Hz. This frequency-dependent behavior is due to the skin effect and proximity effect, which become more pronounced at higher frequencies.

These examples and data points illustrate the importance of understanding and quantifying the magnetic field in a transformer for optimizing its design and performance.

Conclusion

The magnetic field in a transformer is a complex and multifaceted phenomenon that plays a crucial role in the efficient operation of the device. By understanding the factors that influence the magnetic field, such as the number of turns, current, and core material properties, as well as the techniques for measuring and visualizing the magnetic field, engineers can design more efficient and reliable transformers for a wide range of applications.

References

1. Transformer Magnetic Fields and Cans / Covers – diyAudio
2. Induction in transformer coil relationship to magnetic field lines in core – Electronics Stack Exchange
3. Magnetic flux and current directions of transformer – Physics Forums
4. Magnetic Field Evaluation in Transformers and Inductors – Texas Instruments
5. Measurement of Magnetic and Magnetostrictive Characteristics of … – National Center for Biotechnology Information

Magnetic flux and electric flux are fundamental concepts in the field of electromagnetism, with wide-ranging applications in various areas of physics and engineering. This comprehensive guide aims to provide a detailed and technical understanding of these crucial topics, equipping physics students with the necessary knowledge and tools to excel in their studies and research.

Understanding Magnetic Flux

Magnetic flux (Φ) is the measure of the total magnetic field (B) passing through a given area (A). It is represented by the formula Φ = B * A * cos(θ), where B is the magnetic field strength, A is the area, and θ is the angle between the magnetic field and the normal vector to the area.

Magnetic Flux Formula and Units

The formula for magnetic flux is:

Φ = B * A * cos(θ)

Where:
– Φ is the magnetic flux, measured in webers (Wb)
– B is the magnetic field strength, measured in teslas (T)
– A is the area, measured in square meters (m²)
– θ is the angle between the magnetic field and the normal vector to the area, measured in radians (rad)

The SI unit of magnetic flux is the weber (Wb), which is equivalent to one tesla square meter (T·m²).

Magnetic Flux Density

Magnetic flux density, measured in teslas (T), is used to quantify the strength of a magnetic field. It is essential in designing magnetic sensors, electromagnets, and MRI machines, where precise control and measurement of magnetic fields are necessary.

The formula for magnetic flux density is:

B = Φ / A

Where:
– B is the magnetic flux density, measured in teslas (T)
– Φ is the magnetic flux, measured in webers (Wb)
– A is the area, measured in square meters (m²)

Applications of Magnetic Flux

Magnetic flux plays a crucial role in various applications, including:

1. Power generation: Magnetic flux is essential in the operation of generators, transformers, and electric motors, which are the backbone of power generation and distribution systems.
2. Electromechanical motion: Magnetic flux is responsible for the force that drives the motion of electric motors, solenoids, and other electromechanical devices.
3. Medical imaging: Magnetic flux is the foundation of magnetic resonance imaging (MRI) technology, which is widely used in medical diagnostics and research.
4. Structural health monitoring: Magnetic flux can be used to detect and monitor structural defects and changes in materials, making it a valuable tool for non-destructive testing and evaluation.

Understanding Electric Flux

Electric flux is the measure of the total electric field (E) passing through a given area (A). It is represented by the formula ΦE = E * A, where E is the electric field strength and A is the area.

Electric Flux Formula and Units

The formula for electric flux is:

ΦE = E * A

Where:
– ΦE is the electric flux, measured in coulombs per square meter (C/m²)
– E is the electric field strength, measured in volts per meter (V/m)
– A is the area, measured in square meters (m²)

The SI unit of electric flux is the coulomb per square meter (C/m²).

Electric Flux Density

Electric flux density, also known as electric displacement, is the measure of the electric field (E) per unit area (D = εE). It is represented by the formula ΦE = D * A, where D is the electric flux density and A is the area.

The formula for electric flux density is:

D = ΦE / A

Where:
– D is the electric flux density, measured in coulombs per square meter (C/m²)
– ΦE is the electric flux, measured in coulombs per square meter (C/m²)
– A is the area, measured in square meters (m²)

The SI unit of electric flux density is the coulomb per square meter (C/m²).

Applications of Electric Flux

Electric flux is crucial for understanding the behavior of electric fields and their interactions with charges and matter. It has numerous applications, including:

1. Capacitors: Electric flux is essential in the design and operation of capacitors, which are widely used in electronic circuits and power systems.
2. Dielectrics: Electric flux is used to analyze the behavior of dielectric materials, which are important in the design of various electrical and electronic devices.
3. Electromagnetic waves: Electric flux is a fundamental concept in the study of electromagnetic waves, such as those used in radio, television, and wireless communication.

Numerical Examples and Problems

To further solidify the understanding of magnetic flux and electric flux, let’s explore some numerical examples and problems.

Example 1: Calculating Magnetic Flux

Suppose a uniform magnetic field of 2 Tesla (T) is passing through a rectangular area of 0.5 m × 0.8 m, and the angle between the magnetic field and the normal vector to the area is 30 degrees.

Calculate the magnetic flux through the area.

Given:
– Magnetic field strength (B) = 2 T
– Area (A) = 0.5 m × 0.8 m = 0.4 m²
– Angle (θ) = 30 degrees

Using the formula for magnetic flux:
Φ = B * A * cos(θ)
Φ = 2 T * 0.4 m² * cos(30°)
Φ = 0.69 Wb

Therefore, the magnetic flux through the area is 0.69 Wb.

Example 2: Calculating Electric Flux

Consider a uniform electric field of 1000 V/m passing through a circular area with a radius of 0.2 m.

Calculate the electric flux through the area.

Given:
– Electric field strength (E) = 1000 V/m
– Area (A) = π * (0.2 m)² = 0.126 m²

Using the formula for electric flux:
ΦE = E * A
ΦE = 1000 V/m * 0.126 m²
ΦE = 126 C/m²

Therefore, the electric flux through the area is 126 C/m².

Problem 1: Magnetic Flux Density

A solenoid with 500 turns and a cross-sectional area of 0.02 m² carries a current of 5 A. Calculate the magnetic flux density inside the solenoid.

Given:
– Number of turns (N) = 500
– Cross-sectional area (A) = 0.02 m²
– Current (I) = 5 A

Using the formula for magnetic flux density:
B = μ0 * N * I / A
B = 4π × 10^-7 H/m * 500 * 5 A / 0.02 m²
B = 0.157 T

Therefore, the magnetic flux density inside the solenoid is 0.157 T.

Problem 2: Electric Flux Density

A parallel-plate capacitor has a plate area of 0.1 m² and a separation distance of 2 mm. If the capacitor is charged with a total charge of 10 μC, calculate the electric flux density between the plates.

Given:
– Plate area (A) = 0.1 m²
– Separation distance (d) = 2 mm = 0.002 m
– Total charge (Q) = 10 μC = 10 × 10^-6 C

Using the formula for electric flux density:
D = Q / A
D = (10 × 10^-6 C) / (0.1 m²)
D = 0.0001 C/m²

Therefore, the electric flux density between the plates of the capacitor is 0.0001 C/m².

Conclusion

Magnetic flux and electric flux are fundamental concepts in the field of electromagnetism, with wide-ranging applications in various areas of physics and engineering. This comprehensive guide has provided a detailed and technical understanding of these crucial topics, equipping physics students with the necessary knowledge and tools to excel in their studies and research.

Reference:

1. Magnetic Flux – an overview | ScienceDirect Topics
2. Fundamentals of Magnetic Flux and Reluctance – Study for FE
3. Electric Flux vs Magnetic Flux Units – Physics Stack Exchange
4. Applications of Magnetic Flux – GeeksforGeeks
5. Search Coil Experiment (measure magnetic field strength) – YouTube

Magnetic flux is a fundamental concept in electromagnetism, and understanding its behavior in a wire is crucial for various applications, such as electrical engineering, power generation, and magnetic field analysis. This comprehensive guide will delve into the technical details of magnetic flux in a wire, providing you with a thorough understanding of the topic.

Understanding Magnetic Flux

Magnetic flux, denoted by the Greek letter Φ, is a measure of the total magnetic field that passes through a given surface area. It is expressed in the SI unit of weber (Wb) or tesla-meter squared (Tm²). Magnetic flux is a vector quantity, meaning it has both magnitude and direction.

The magnetic flux through a surface area A perpendicular to the magnetic field B can be calculated using the formula:

Φ = B × A × cos(θ)

where θ is the angle between the magnetic field and the normal to the surface area.

Magnetic Field around a Current-Carrying Wire

When a current I flows through a wire, it generates a magnetic field around the wire. This magnetic field can be calculated using Ampere’s law, which states that the line integral of the magnetic field around a closed loop is proportional to the current enclosed by the loop.

For a long, straight wire carrying a current I, the magnetic field B at a distance r from the wire is given by:

B = (μ₀ × I) / (2π × r)

where μ₀ is the permeability of free space, which has a value of approximately 4π × 10⁻⁷ Tm/A.

Calculating Magnetic Flux in a Wire

To calculate the magnetic flux through a surface area A perpendicular to the magnetic field generated by a current-carrying wire, we can use the formula:

Φ = B × A × cos(θ)

where θ is the angle between the magnetic field and the normal to the surface area.

Let’s consider an example:
* Wire carrying a current of 2 A
* Surface area of 0.5 m²
* Distance from the wire: 0.1 m

The magnetic field at a distance of 0.1 m from the wire can be calculated as:

B = (μ₀ × I) / (2π × r)
B = (4π × 10⁻⁷ Tm/A × 2 A) / (2π × 0.1 m)
B = 4 × 10⁻⁵ T

Assuming the surface area is perpendicular to the magnetic field (θ = 0°), the magnetic flux can be calculated as:

Φ = B × A × cos(θ)
Φ = (4 × 10⁻⁵ T) × (0.5 m²) × 1
Φ = 2 × 10⁻⁵ Wb

Therefore, the magnetic flux through the surface area is 2 × 10⁻⁵ webers.

Factors Affecting Magnetic Flux in a Wire

Several factors can influence the magnetic flux in a wire, including:

1. Current Magnitude: The strength of the magnetic field, and consequently the magnetic flux, is directly proportional to the current flowing through the wire.

2. Distance from the Wire: As the distance from the wire increases, the magnetic field and magnetic flux decrease according to the inverse square law.

3. Angle between the Magnetic Field and the Surface Area: The magnetic flux is maximized when the surface area is perpendicular to the magnetic field (θ = 0°) and decreases as the angle increases.

4. Wire Geometry: The shape and configuration of the wire can affect the distribution and strength of the magnetic field, which in turn influences the magnetic flux.

5. Magnetic Properties of the Material: The permeability of the material surrounding the wire can also impact the magnetic flux, as it affects the magnetic field lines.

Measuring Magnetic Flux in a Wire

Magnetic flux in a wire can be measured using various instruments, such as:

1. Magnetometer: A magnetometer is a device that measures the strength and direction of the magnetic field. By placing a magnetometer near the wire, you can determine the magnetic flux density and calculate the total magnetic flux.

2. Flux Meter: A flux meter is a specialized instrument designed to directly measure the magnetic flux. It typically consists of a search coil that is placed in the magnetic field, and the induced voltage is used to calculate the flux.

3. Hall Effect Sensor: Hall effect sensors can be used to measure the magnetic field strength, which can then be used to calculate the magnetic flux.

4. Faraday’s Law of Electromagnetic Induction: By measuring the induced voltage in a loop of wire placed in the magnetic field, you can calculate the magnetic flux using Faraday’s law.

Applications of Magnetic Flux in Wires

Magnetic flux in wires is crucial for various applications, including:

1. Electrical Transformers: Transformers rely on the magnetic flux generated by the primary winding to induce a voltage in the secondary winding, enabling the transformation of voltage levels.

2. Electric Motors and Generators: The interaction between the magnetic flux and the current-carrying conductors in motors and generators is the fundamental principle behind their operation.

3. Magnetic Resonance Imaging (MRI): MRI machines use strong magnetic fields and magnetic flux to generate detailed images of the human body for medical diagnosis and research.

4. Magnetic Field Sensing: Magnetic flux measurements are used in various applications, such as position sensing, current sensing, and magnetic field mapping.

5. Electromagnetic Induction: The changing magnetic flux in a wire can induce a voltage in another wire, which is the basis for many electromagnetic induction phenomena, such as in transformers and generators.

Conclusion

Magnetic flux in a wire is a crucial concept in electromagnetism, with numerous applications in various fields. By understanding the principles, formulas, and factors affecting magnetic flux, you can effectively analyze and manipulate the magnetic fields in wires to achieve desired outcomes. This comprehensive guide has provided you with the necessary knowledge and tools to delve deeper into the world of magnetic flux in wires.

References

1. “Magnetic Flux” by BYJU’S, available at: https://byjus.com/physics/magnetic-flux/
2. “How to Calculate the Flux Perpendicular to a Loop of Wire” by Study.com, available at: https://study.com/skill/learn/how-to-calculate-the-flux-perpendicular-to-a-loop-of-wire-explanation.html
3. “The Magnetic Field due to a Current in a Straight Wire” by Nagwa, available at: https://www.nagwa.com/en/explainers/909137183476/
4. “Magnetic flux density measured for beginners: from fizzics.org” by The Engineering Mindset, available at: https://www.youtube.com/watch?v=3nGc44rYXww

Magnetic flux (Φ) is a fundamental concept in electromagnetism that quantifies the total magnetic field passing through a specified surface. It is a crucial parameter in various fields, including physics, engineering, and materials science. This comprehensive guide will delve into the intricacies of magnetic flux and its relationship with surface area, providing a detailed exploration for physics students.

Understanding Magnetic Flux

Magnetic flux is a measure of the total magnetic field that penetrates a specified closed surface. It is quantified by counting the magnetic field lines that intersect the surface. The SI unit of magnetic flux is the Weber (Wb), named after the German physicist Wilhelm Eduard Weber.

The formula for magnetic flux is given by:

ϕ_B = B ⋅ A = B A cos(θ)

Where:
– ϕ_B is the magnetic flux (in Webers)
– B is the magnetic field (in Teslas)
– A is the surface area (in square meters)
– θ is the angle between the magnetic field and the normal to the surface (in radians)

This equation demonstrates that the magnetic flux depends on the strength of the magnetic field, the area of the surface, and the angle at which the field lines pass through the surface. Magnetic flux is a scalar quantity, meaning its magnitude depends on these factors, but it does not have a specific direction.

Magnetic Flux Density

Closely related to magnetic flux is the concept of magnetic flux density, also known as magnetic induction or magnetic field strength. Magnetic flux density (B) is a measure of the concentration of magnetic field lines in a given area. It is defined as the number of magnetic field lines per unit area and is measured in Tesla (T).

The formula for magnetic flux density is:

B = ϕ_B / A

Where:
– B is the magnetic flux density (in Teslas)
– ϕ_B is the magnetic flux (in Webers)
– A is the surface area (in square meters)

Magnetic flux density is a vector quantity, meaning it has both magnitude and direction. It represents the strength and direction of the magnetic field at a specific point in space.

Measuring Magnetic Flux

The measurement of magnetic flux and magnetic flux density is crucial in various fields, including physics, engineering, and materials science. A device commonly used to measure magnetic flux is a magnetometer.

A magnetometer works by measuring the voltage induced in a coil by the magnetic field. The voltage is proportional to the rate of change of the magnetic flux with time, as described by Faraday’s law of electromagnetic induction:

ε = -dϕ_B/dt

Where:
– ε is the induced electromotive force (EMF) in the coil (in Volts)
– dϕ_B/dt is the rate of change of the magnetic flux with time (in Webers per second)

By measuring the induced voltage in the coil, the magnetometer can determine the magnitude and direction of the magnetic flux, which is then used to calculate the magnetic flux density.

Magnetic Flux Indicators and QQIs

In the context of magnetic particle testing, magnetic flux indicators and Quantitative Quality Indicators (QQIs) are used to verify the direction and strength of the magnetic field.

Magnetic Flux Indicators

Magnetic flux indicator strips are used to visualize the direction of the magnetic field. These strips are positioned so that their long dimension is perpendicular to the applied magnetic field. When the magnetic field is energized and magnetic particles (wet suspension or dry powder) are applied, the indications will be strongest and most well-defined when the strip is perpendicular to the magnetic flux. No indications will form if the strip is aligned parallel to the magnetic flux.

Quantitative Quality Indicators (QQIs)

QQIs are artificial flaw notched shims that are attached to example parts to demonstrate both the field strength and direction within a part. They are useful for setting up the magnetization parameters for part-specific techniques and can be used to create an example part for the daily system performance check of the magnetizing equipment.

By using magnetic flux indicators and QQIs, technicians can ensure that the magnetic field is properly oriented and of sufficient strength for effective magnetic particle testing.

Practical Applications of Magnetic Flux and Flux Density

The concepts of magnetic flux and flux density have numerous practical applications in various fields:

1. Electrical Machines: In electric motors and generators, the magnetic flux and flux density are crucial parameters that determine the efficiency, torque, and power output of the machine.

2. Transformers: The magnetic flux in the core of a transformer determines the voltage transformation ratio and the power transfer capability of the device.

3. Magnetic Resonance Imaging (MRI): MRI machines rely on the precise control and measurement of magnetic flux density to generate high-quality images of the human body.

4. Magnetic Levitation: Magnetic levitation trains and other levitation systems utilize the precise control of magnetic flux to achieve frictionless, high-speed transportation.

5. Magnetic Particle Testing: As mentioned earlier, magnetic flux indicators and QQIs are used in magnetic particle testing to ensure the proper orientation and strength of the magnetic field for non-destructive evaluation of materials.

6. Magnetic Data Storage: The storage and retrieval of data in magnetic media, such as hard drives and magnetic tapes, rely on the precise control and measurement of magnetic flux.

7. Magnetic Shielding: Understanding magnetic flux and flux density is crucial for designing effective magnetic shielding systems to protect sensitive electronic equipment from external magnetic fields.

Numerical Examples and Problems

To further solidify your understanding of magnetic flux and its relationship with surface area, let’s explore some numerical examples and problems:

1. Example 1: A uniform magnetic field of 0.5 Tesla is applied perpendicular to a rectangular surface with dimensions of 10 cm × 15 cm. Calculate the magnetic flux through the surface.

Given:
– Magnetic field (B) = 0.5 Tesla
– Surface area (A) = 10 cm × 15 cm = 0.15 m²
– Angle (θ) = 0° (perpendicular to the surface)

Magnetic flux (ϕ_B) = B × A × cos(θ)
ϕ_B = 0.5 T × 0.15 m² × cos(0°) = 0.075 Wb

1. Problem 1: A circular coil with a radius of 20 cm is placed in a uniform magnetic field of 1 Tesla. Calculate the magnetic flux through the coil if the angle between the normal to the coil and the magnetic field is 30°.

Given:
– Magnetic field (B) = 1 Tesla
– Coil radius (r) = 20 cm = 0.2 m
– Angle (θ) = 30°

Surface area (A) = π × r² = π × (0.2 m)² = 0.1256 m²
Magnetic flux (ϕ_B) = B × A × cos(θ)
ϕ_B = 1 T × 0.1256 m² × cos(30°) = 0.1088 Wb

1. Problem 2: A square coil with a side length of 50 cm is placed in a uniform magnetic field of 0.8 Tesla. Calculate the magnetic flux through the coil if the angle between the normal to the coil and the magnetic field is 45°.

Given:
– Magnetic field (B) = 0.8 Tesla
– Coil side length (s) = 50 cm = 0.5 m
– Angle (θ) = 45°

Surface area (A) = s² = (0.5 m)² = 0.25 m²
Magnetic flux (ϕ_B) = B × A × cos(θ)
ϕ_B = 0.8 T × 0.25 m² × cos(45°) = 0.1414 Wb

These examples and problems demonstrate the application of the magnetic flux formula and the importance of considering the angle between the magnetic field and the surface area. By working through these exercises, you can deepen your understanding of the concepts and their practical implications.

Conclusion

Magnetic flux and magnetic flux density are fundamental concepts in the study of electromagnetism. Understanding the relationship between magnetic flux, surface area, and the angle of the magnetic field is crucial for various applications in physics, engineering, and materials science.

This comprehensive guide has provided a detailed exploration of magnetic flux, including the formula, the concept of magnetic flux density, and the measurement of magnetic flux using magnetometers. Additionally, the practical applications of these concepts, as well as numerical examples and problems, have been discussed to enhance your understanding and problem-solving skills.

By mastering the intricacies of magnetic flux and area, you will be better equipped to tackle complex problems, design efficient electrical machines and devices, and contribute to the advancement of various fields that rely on the principles of electromagnetism.

References

1. Wired. (2014). How to Measure a Magnetic Field. [online] Available at: https://www.wired.com/2014/01/measure-magnetic-field/ [Accessed 15 May 2023].
2. e-magnetica.pl. (n.d.). Magnetic Flux Density. [online] Available at: https://www.e-magnetica.pl/doku.php/magnetic_flux_density [Accessed 15 May 2023].
3. GeeksforGeeks. (n.d.). Magnetic Flux. [online] Available at: https://www.geeksforgeeks.org/magnetic-flux/ [Accessed 15 May 2023].
4. Magnaflux. (n.d.). Flux Indicators and QQIs. [online] Available at: https://magnaflux.com/Magnaflux/Resources/Blog/Flux-Indicators-and-QQIs [Accessed 15 May 2023].
5. YouTube. (2018). Magnetic Flux Density and Magnetic Flux. [online] Available at: https://www.youtube.com/watch?v=N4H1X4i2OZ4 [Accessed 15 May 2023].

Magnetic flux is a fundamental concept in electromagnetism that describes the amount of magnetic field passing through a given surface. Interestingly, magnetic flux can indeed be negative, and its sign is determined by the direction of the magnetic field relative to the surface of integration. This concept is crucial in understanding various electromagnetic phenomena, such as Faraday’s law of induction and Lenz’s law.

Understanding Magnetic Flux

Magnetic flux, denoted by the symbol Φ, is defined as the product of the magnetic field strength (B) and the area (A) of the surface through which the field passes, with the angle (θ) between the magnetic field and the normal to the surface taken into account. Mathematically, the magnetic flux is expressed as:

Φ = ∫ B⋅dA = ∫ B cos(θ) dA

where the integral is taken over the surface of interest.

The sign of the magnetic flux is determined by the relative orientation of the magnetic field and the surface normal. If the magnetic field is parallel to the surface normal, the flux is positive. Conversely, if the magnetic field is antiparallel to the surface normal, the flux is negative.

Positive and Negative Magnetic Flux

To illustrate the concept of positive and negative magnetic flux, consider a simple example:

Imagine a rectangular surface with an area vector pointing in the positive z-direction. If the magnetic field is directed in the positive z-direction, the magnetic flux through the surface will be positive. However, if the magnetic field is directed in the negative z-direction, the magnetic flux through the surface will be negative.

This can be expressed mathematically as follows:

• If B is in the positive z-direction and the surface normal is also in the positive z-direction, then θ = 0°, and the magnetic flux is positive:
  Φ = ∫ B cos(0°) dA = ∫ B dA

• If B is in the negative z-direction and the surface normal is in the positive z-direction, then θ = 180°, and the magnetic flux is negative:
  Φ = ∫ B cos(180°) dA = -∫ B dA

The sign of the magnetic flux is crucial in understanding various electromagnetic phenomena, as it determines the direction of the induced electromotive force (EMF) and the induced current in a coil, as described by Faraday’s law of induction and Lenz’s law.

Faraday’s Law of Induction and Negative Magnetic Flux

Faraday’s law of induction states that the induced EMF in a coil is proportional to the negative of the time rate of change of the magnetic flux through the coil. Mathematically, this is expressed as:

ε = -N dΦ/dt

where ε is the induced EMF, N is the number of turns in the coil, and dΦ/dt is the time rate of change of the magnetic flux through the coil.

The negative sign in Faraday’s law indicates that the induced EMF opposes the change in magnetic flux. This is a consequence of Lenz’s law, which states that the direction of the induced current is such that it creates a magnetic field that opposes the change in the original magnetic flux.

If the magnetic flux through the coil is increasing, the induced EMF will be negative, as it opposes the increase in flux. Conversely, if the magnetic flux through the coil is decreasing, the induced EMF will be positive, as it opposes the decrease in flux.

Examples of Negative Magnetic Flux

1. Coil in a Changing Magnetic Field: Consider a coil placed in a magnetic field that is decreasing over time. As the magnetic field decreases, the magnetic flux through the coil also decreases. According to Faraday’s law, this will induce a positive EMF in the coil, as the induced current will create a magnetic field that opposes the decrease in the original magnetic flux.

2. Rotating Coil in a Uniform Magnetic Field: Imagine a coil rotating in a uniform magnetic field. As the coil rotates, the angle between the magnetic field and the surface normal changes, causing the magnetic flux through the coil to vary. When the coil is oriented such that the magnetic field is antiparallel to the surface normal, the magnetic flux will be negative, and the induced EMF will be positive, as it opposes the decrease in the original magnetic flux.

3. Magnetic Shielding: Magnetic shielding is a technique used to protect sensitive electronic devices from the effects of external magnetic fields. This is often achieved by surrounding the device with a material that has high magnetic permeability, such as mu-metal. The shielding material creates a region of negative magnetic flux, effectively canceling out the external magnetic field and protecting the device inside.

Numerical Example

To illustrate the concept of negative magnetic flux, let’s consider a numerical example:

Suppose a rectangular coil with an area of 0.1 m^2 is placed in a uniform magnetic field of 0.5 T, directed in the positive z-direction. The coil is then rotated such that the angle between the magnetic field and the surface normal changes from 0° to 180°.

1. When the coil is oriented such that the surface normal is parallel to the magnetic field (θ = 0°):
   Φ = ∫ B cos(0°) dA = ∫ (0.5 T) (1) (0.1 m^2) = 0.05 Wb

2. When the coil is oriented such that the surface normal is antiparallel to the magnetic field (θ = 180°):
   Φ = ∫ B cos(180°) dA = ∫ (0.5 T) (-1) (0.1 m^2) = -0.05 Wb

In this example, the magnetic flux changes from a positive value of 0.05 Wb to a negative value of -0.05 Wb as the coil is rotated by 180°. This change in the sign of the magnetic flux will induce a positive EMF in the coil, as the induced current will create a magnetic field that opposes the decrease in the original magnetic flux.

Conclusion

In summary, magnetic flux can indeed be negative, and its sign is determined by the relative orientation of the magnetic field and the surface normal. This concept is crucial in understanding various electromagnetic phenomena, such as Faraday’s law of induction and Lenz’s law. By understanding the behavior of positive and negative magnetic flux, physicists and engineers can better design and analyze electromagnetic systems, leading to improved performance and efficiency.

References:

1. Griffiths, D. J. (2013). Introduction to Electromagnetism (4th ed.). Pearson.
2. Serway, R. A., & Jewett, J. W. (2014). Physics for Scientists and Engineers with Modern Physics (9th ed.). Cengage Learning.
3. Halliday, D., Resnick, R., & Walker, J. (2013). Fundamentals of Physics (10th ed.). Wiley.
4. Zill, D. G., & Cullen, M. R. (2009). Advanced Engineering Mathematics (4th ed.). Jones & Bartlett Learning.