Cover Image Credit – Rufustelestrat, San Diego Reflecting Pond, CC BY-SA 3.0
Points of Discussions
- Introduction to Millman’s Theorem
- Theory of Millman’s Theorem
- Applications of Millman’s Theorem
- Problem Solving Steps for Millman’s Theorem
- Explanation of Theories
- Solved Problems on Millman’s Theorem
Introduction to Millman’s Theorem
In the previous Advanced Electrical Circuit Analysis articles, we have discussed some of the fundamental theories like – Thevenin’s Theorem, Norton’s Theorem, Superposition Theorem, etc. We have also come to know the Maximum Power transfer theorem for finding out the maximum load resistance to drain full power. In this article, we will learn about another important and fundamental electrical analysis to deal with complex circuits, known as Millman’s theorem. We will discuss the theory, the process to solve the problems related to this theory, the applications of this theory and other important aspects.
Professor Jacob Millman first proved the theorem, and that is why it is named after him. This theory helps us to simplify the circuit. Thus, it becomes easier to analyze the circuit. This theorem is also known as “Parallel generator theorem”. Millman’s theorem is applied in courses to calculate the voltage of some specified circuitries. It is one of the essential theorems in Electrical Engineering.
What is meant by Thevenin’s theorem? Click Here!
Theory of Millman’s Theorem
Millman’s Theorem: It states that if multiple voltage sources (having internal resistances) are connected in parallel, this specific circuit can be replaced by a simpler circuit of a single voltage source and a resistance in series.
This theory helps us to find out voltages at the end of parallel branches if the circuit is structured in parallel connections. The principal aim of this theory is nothing but to reduce the complexity of the circuit.
Applications of Millman’s Theorem
Millman’s theorem is one of the efficient theorems. That is why there are several real-world applications for this theory. Millman’s theorem is applicable for a circuit with multiple voltage sources with their internal resistances in a parallelly connected way. It helps to solve complex circuit theory problems. Unbalanced bridges, parallel circuit problems can be solved using this theorem.
What are network theorems? Click Here!
Steps for Solving Problems regarding Millman’s Theorem
Generally, the given steps are tracked for solving Millman’s Theory problems. There are several other paths, but following these below-mentioned steps will lead to a more efficient result.
Step 1: Find out the conductance value of every single voltage source.
Step 2: Remove the load resistance. Calculate the equivalent conductance of the circuit.
Step 3: The circuit is now ready to apply Millman’s Theorem. Apply the theorem to find out the equivalent source voltage V. The below equation gives the V value.
V = (± V1 G1 ± V2 G2 ± V3 G3 ± … ±Vn Gn) / G1 + G2 + G3 + … + Gn
V1, V2, V3 are the voltages and G1, G2, G3 are their respective conductance.
Step 4: Now, find out the equivalent series resistance of the circuit with the help of conductance value, calculated earlier. The equivalent series resistance is given by the expression: R = 1 / G
Step 5: At last, calculate the current through the load by the following equation.
IL = V / (R + RL)
Here, IL is the current through the load resistance. RL is the load resistance. R is the equivalent series resistance. V is the identical source voltage calculated with the help of conductance of their respective voltages.
What is Maximum Power Transfer Theorem? Click Here!
Explanation of Millman’s Theorem
To explain the theorem in details, let us take an example of a specified circuit. The below image describes the needed circuit. The picture shows a typical DC circuit with multiple parallel source voltages with their internal resistances and with the load resistance. RL gives the value of load resistance.

Let us assume that ‘I’ is the current value through the parallel current sources. G gives the equivalent conductance or admittance value. The resultant circuit is shown below.
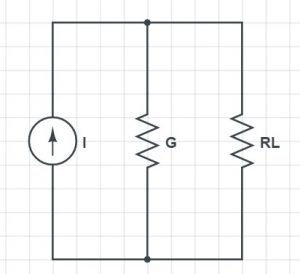
I = I1 + I2 +I3 + …
G = G1 + G2 + G3 + ….
Now, the final current source is replaced by an equivalent source voltage. The voltage ‘V’ can be written as: V = 1/G = (± I1 ± I2 ± I3 ± … ±In) / (G1 +G2 + G3 + … + Gn)
And equivalent series resistance comes as:
R = 1 / G = 1 / (G1 + G2 + G3 + … + Gn)
Now, we know that V = IR and R = 1 / G
So, V can be written as:
V = [± (V1 / R1) ± (V2 / R2) ± (V3 / R3) ± … ± (Vn / Rn)] / [ (1 / R1) ± (1 / R2) ± (1 / R3) ± … ± (1 / Rn)]
R is the equivalent series resistance.
Now, as per Millman’s theory, the equivalent voltage source comes to be:
V = (± V1 G1 ± V2 G2 ± V3 G3 ± … ±Vn Gn) / (G1 + G2 + G3 + … + Gn)
Or, V = Σ (n, k = 1) Vk Gk / Σ (n, k = 1) Gk
Gk = 1 /Rk
To know About Kirchhoff’s Laws: Click Here!
Solved Problems on Millman’s Theorem
1. A complex circuit is given below. Find the current through the 4 ohms resistance. Use Millman’s Theorem to solve the problem.
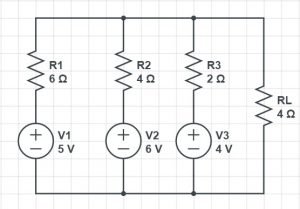
Solution: We will solve the problem by following the previously mentioned steps.
So, we have to find out the voltage value and the equivalent resistance value.
We know that the voltage is given by,
V = [± (V1 / R1) ± (V2 / R2) ± (V3 / R3) ± … ± (Vn / Rn)] / [ (1 / R1) ± (1 / R2) ± (1 / R3) ± … ± (1 / Rn)]
Here, we have three voltage source and three resistances. So, the updated equation will be,
VAB = [± (V1 / R1) ± (V2 / R2) ± (V3 / R3)] / [ (1 / R1) ± (1 / R2) ± (1 / R3)]
VAB = [(5 / 6) + (6 / 4) + (4 / 2)] / [(1 / 6) + (1 / 4) + (1 / 2)]
VAB = 4.33 / 0.9167
OR, VAB = 4.727 V
Now, we have to calculate the equivalent resistance of the circuit, or the Thevenin’s equivalent resistance is Rth.
RTH = [(1 / 6) + (1 / 4) + (1 / 2)] -1
Or, RTH = 1.09 ohms
At the last step, we will find out the current value through the load resistance, that is 4 ohms.
We know that, IL = VAB / (RTH + RL)
Or, IL = 4.727 / (1.09 + 4)
Or, IL = 4.727 / 5.09
Or, IL = 0.9287 A
So, The load current through 4 ohms load is 0.9287 A.
Learn About Basics of AC Circuit: Click Here!
2. A complex electrical circuit is given below. Find out the current through the 16 ohms load resistance. Use Millman’s theorem to solve the problems.
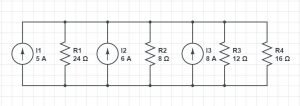
Solution: We will solve the problem by following the previously mentioned steps.
At first, we have to calculate the current value using Norton’s theorem.
The current ‘I’ can be written as: I = I1 + I2 + I3
Or, I = 10 + 6 – 8
Or, I = 8 A
Now we have to find out the Equivalent resistance value. We represent the equivalent resistances of R1, R2, R3 as RN.
So, RN = [(1 / R1) + (1 / R2) + (1 / R3)]-1
Or, RN = [(1/ 24) + (1 / 8) + (1 / 12)]-1
Or, RN = 4 ohms
We now redraw the circuit with equivalent voltage and resistances value and place the circuit’s load resistance.
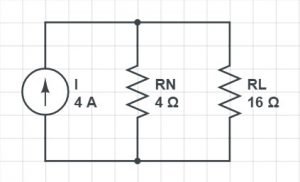
At the last step, we have to find out the load Current. So, IL = I x R / (R + RL)
Or, IL = 8 x 4 / (4 + 16)
Or, IL = 1.6 A.
So, the load current through the 8 ohms load resistor is 1.6 A.
Learn About Advanced AC Circuit: Click Here!
3. A complex AC network is given below. Compute the current passing through the Load ZL. Use Millman’s Theorem to solve the problem.
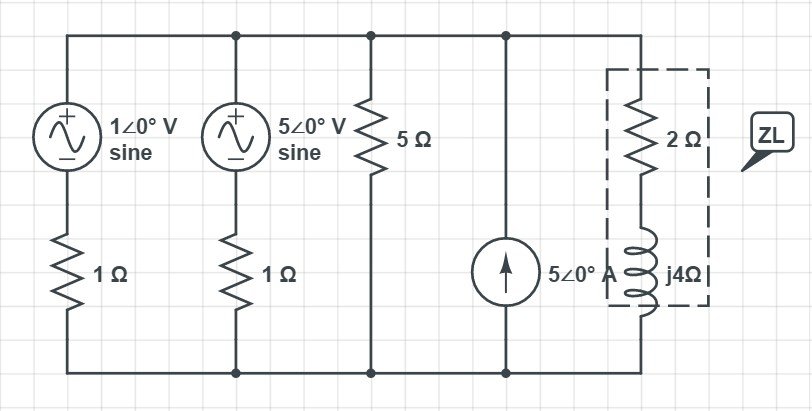
Solution: We will solve the problem by following the previously mentioned steps. In this problem, we can see that a current source is given. But we know that we cannot apply Millman’s Theory for a current source. So, It is possible to convert the current source to a voltage source.
Now, we apply Millman’s theorem and finds out the equivalent voltage.
We know that,
V = [± (V1 / R1) ± (V2 / R2) ± (V3 / R3)] / [ (1 / R1) ± (1 / R2) ± (1 / R3)]
So, V = (1 * 1 ∠0o + 1 * 5 ∠0o + 0.2 * 25 ∠0o) / ( 1 + 1 + 0.2)
Or, V = 11 / 2.2 = 5 ∠0o V.
IL gives the current through the load resistance.
As we know, V = IR.
Or, IL = V / ZL = 5 ∠0o / (2 + j4)
Or, IL = 1.12 ∠-63.43o A.
So, Current through the load resistance is 1.12 ∠-63.43o A.
Cover Photo By: Abyss

Hi, I am Sudipta Roy. I have done B. Tech in Electronics. I am an electronics enthusiast and am currently devoted to the field of Electronics and Communications. I have a keen interest in exploring modern technologies such as AI & Machine Learning. My writings are devoted to providing accurate and updated data to all learners. Helping someone in gaining knowledge gives me immense pleasure.
Let’s connect through LinkedIn –