The article discusses some facts about constant velocity in displacement time graph.
The displacement time graph, known as the position-time graph, represents an object’s motion. When we plot the moving object’s displacement on a y-axis as a dependent variable and time on an x-axis as an independent variable, its straight-line slope reveals an object moves with constant velocity.
Read more about Horizontal Velocity.
When we plot a moving object’s displacement values for respective time values, we get a slope that displays an object’s motion characteristics.
The connection between the slope of displacement time graph and constant velocity:
- If a slope appears as a horizontal straight line, it indicates that an object’s velocity is zero, as it is at rest.
- If a slope appears as a curved line, it indicates that an object’s velocity changes as it is accelerated.
- If a slope appears as a straight line, it indicates that an object is moving with constant velocity, as it is not accelerated.

The slope exhibits similar characteristics as the velocity. Let’s investigate the concept of constant velocity in the displacement-time graph. When an object travels with constant velocity, the displacement changes by a fixed value in each unit of time
The graph displays the type of motion and provides the value of velocity. The slope is a change in displacement per change in time values of the line draw, which is equal to the velocity. Simply saying, the calculated slope value is a value of an object’s velocity.
v → = s2-s1/t2-t1 = Δs→/Δt→

Calculate the constant velocity of the moving car from below displacement vs. time graph.
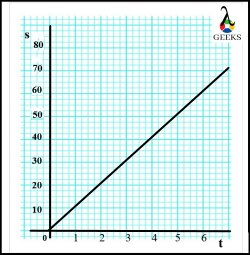
Given:
From graph,
s1 = 0m
s2 = 60m
t1 = 0sec
t2 = 6sec
To Find:
v → =?
Formula:
v → = s2-s1/t2-t1 = Δs→/Δt→
Solution:
The value of constant velocity of moving is calculated as,
v → = s2-s1/t2-t1 = Δs→/Δt→
Substituting all values,
v → = 60-0/6-0
v → = 10
The car moves with constant velocity of 10m/s.
What is Constant Velocity in Displacement Time Graph?
The constant velocity in the displacement time graph is the straight-line slope.
The displacement time graph displays various slopes based on the motion. But the slope emerges as a steep straight line signifies the constant velocity. The steeper or more vertical the graph’s slope line, the faster an object travels with constant velocity as no net force is acted.
When there is no net force on an object moving with constant velocity, it continues to move with constant speed in the constant direction. But its displacement is changing with respect to time, as shown below figure. If we plot the displacement time data for the moving car, then the resulting displacement time graph as below:
We observe that the car moves with a constant velocity until 50m. Further, it remains stationary for 2 seconds, and then it moves with constant velocity.
Read more about Speed due to Net Force.
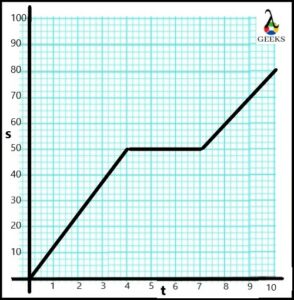
From the displacement – time graph above, calculate the following:
How far does the car travel in 8 secs?
What is the constant velocity of the car initially and laterally?
What is the average speed of the car?
Formula:
- v → = s2-s1/t2-t1
- v=sf-si/tf-ti
Solution:
1) The car travelled 60m in 8 seconds.
2) (i) The constant velocity of the car initially is calculated as,
v → = s2-s1/t2-t1
Subsisting values from the slope of graph,
v → = 50-0/5-0
v → = 50/5
v → = 10
The constant velocity of the car initially is 10m/s
(ii) The constant velocity of the car latterly is,
v → = s2-s1/t2-t1
Subsisting values from the slope of graph,
v →= 100-50/10-7
v →= 50/3
v → = 16.6
The constant velocity of the car latterly is 16.6m/s
3)The average speed of the car is calculated as,
v=sf-si/tf-ti
Substituting all values from the slope of graph,
v = 100-0/10-0
v = 10
The car’s average speed is 10m/s.
When Velocity is Constant in Displacement Time Graph?
The velocity is constant in the displacement time graph when its displacement changes.
The correlation between displacement and velocity gives rise to the diverse slope shapes in the displacement time graph. It depends on the positive or negative value of the constant velocity as per an object’s direction.
Let’s illustrate the displacement-time graph for high and low constant velocities for the car moving to the right. We observe the slope of the low constant velocity graph is more down than the high constant velocity graph. Both slopes of constant velocities is positive as the displacement changes in an increasing manner. That’s how we can imagine which object is moving faster than the other from the displacement time graph.
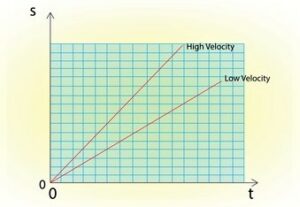
(credit: shutterstock)
On the other hand, if an object’s displacement decreases with time, it shows the constant negative velocity in the displacement time graph.

We have examined the subsequent facts in the displacement time graph:
- Greater the slope, the greater the constant velocity.
- If displacement increases, sloping upwards as time increases, and constant velocity is positive.
- If displacement decreases, sloping downwards as time increases, and constant velocity is negative.
- If displacement is zero, the slope is horizontal, and the constant velocity is zero.
Read more about Relative Motion.
Analyze and comment on different sections in the displacement time graph below. Also, calculate the constant positive velocity and constant negative velocity from the graph.
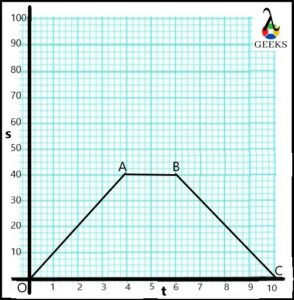
Solution:
1) The section OA represents the constant positive velocity as displacement is increasing.
The positive constant velocity is calculated as
v → = s2-s1/t2-t1
Substituting values from the section AB of the graph,
v → = 6-0/2-0
v → =3
The constant positive velocity is 3m/s.
2) The displacement does not change from section A to B for 4 seconds; that means an object remains stopped at 2m and its constant velocity is zero.
3) The section BC represents the constant negative velocity as displacement is decreasing.
The negative constant velocity is calculated as
v → = s2-s1/t2-t1
Substituting values from the section BC of the graph,
v → = 0-6/2-0
v → = -6/2
v → =-3
The constant negative velocity is -3m/s.
4) At point C, displacement is zero; that means the moving object with constant velocity returns to its original position and becomes stationary.
Also Read:
- Horizontal speed vs horizontal velocity
- How to find constant acceleration with velocity and time
- How to find velocity from displacement
- Orbital velocity of moon
- Rpm to angular velocity
- How to find velocity in m theory
- How to find angular velocity in rotational dynamics
- What is reflection velocity
- How to find magnetic field from velocity
- Position time graph to velocity time graph

Hello, I’m Manish Naik completed my MSc Physics with Solid-State Electronics as a specialization. I have three years of experience in Article Writing on Physics subject. Writing, which aimed to provide accurate information to all readers, from beginners and experts.
In my leisure time, I love to spend my time in nature or visiting historical places.
Looking forward to connecting you through LinkedIn –