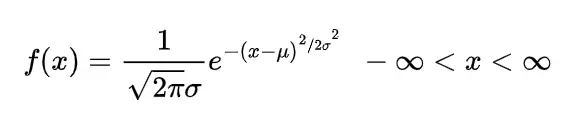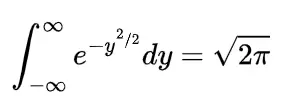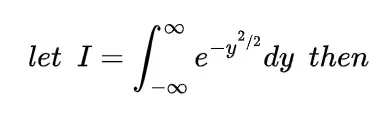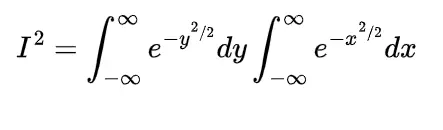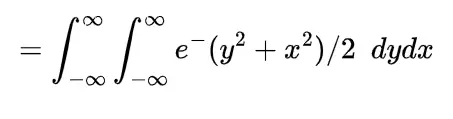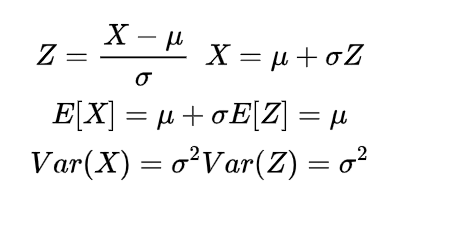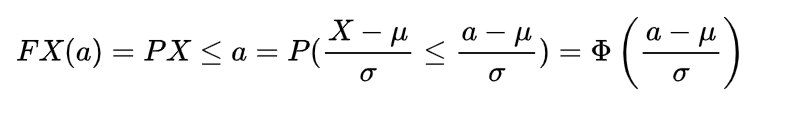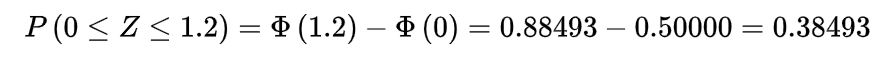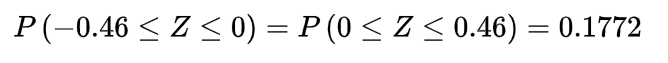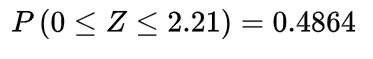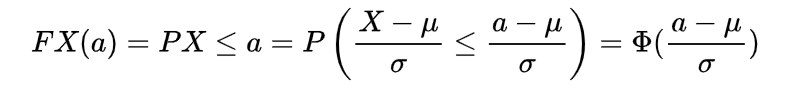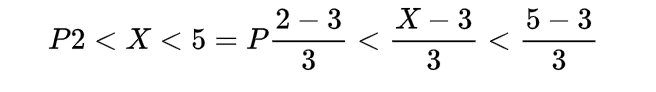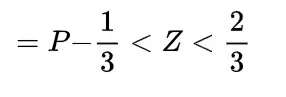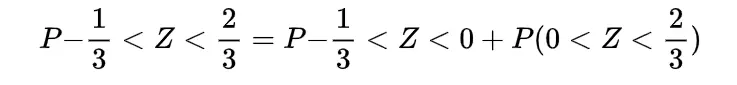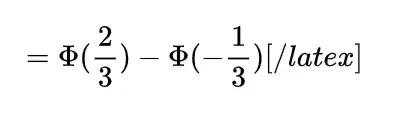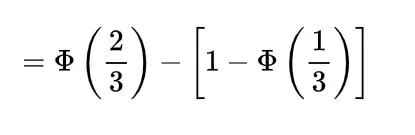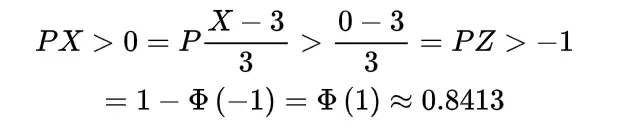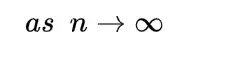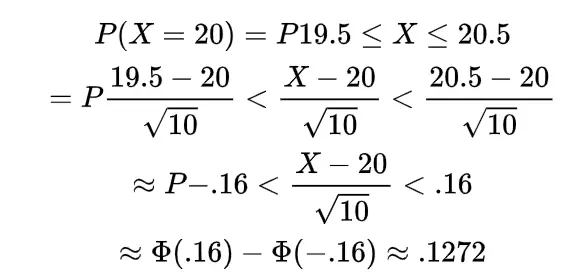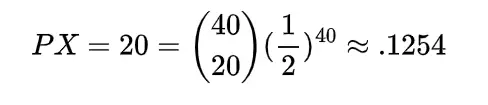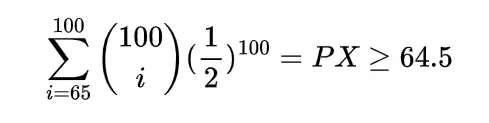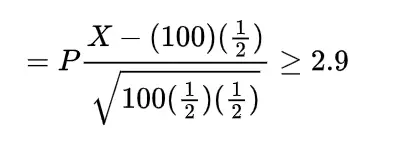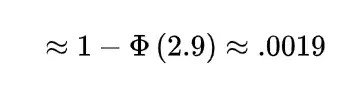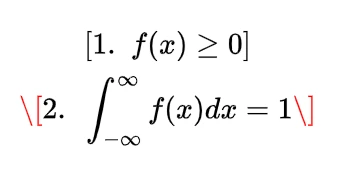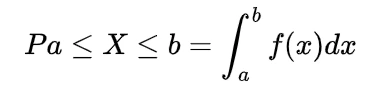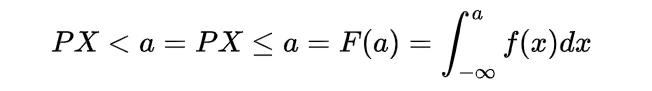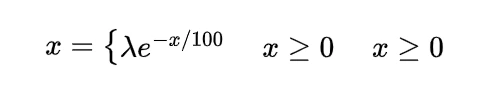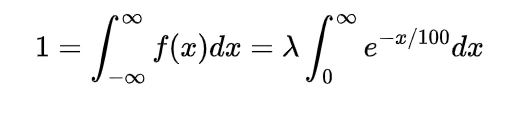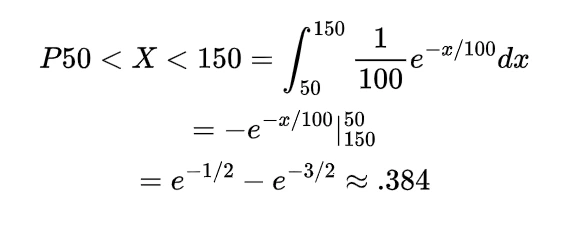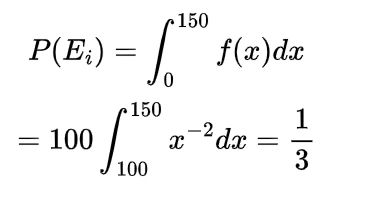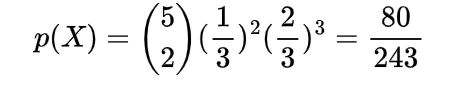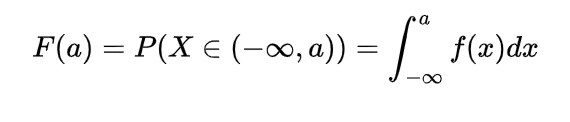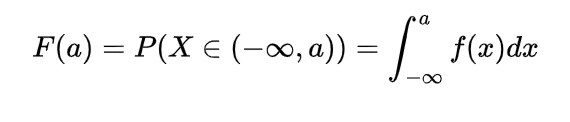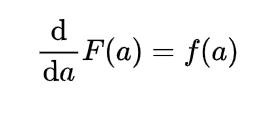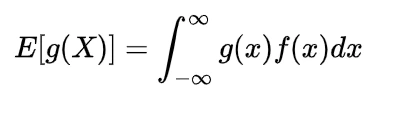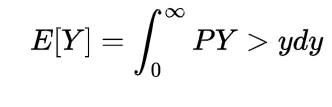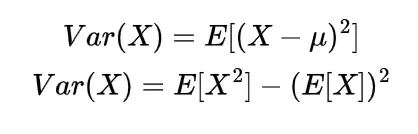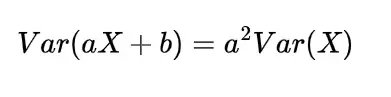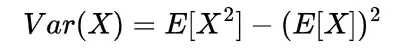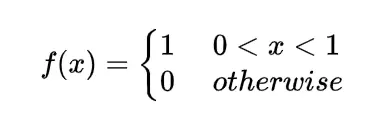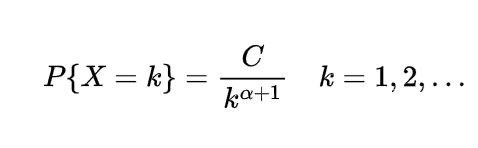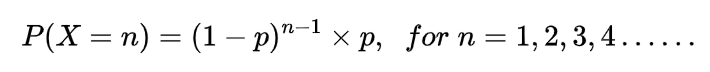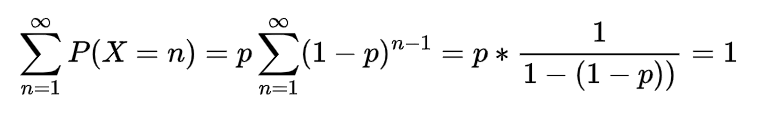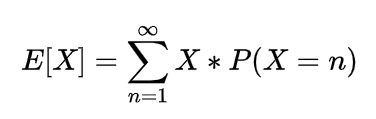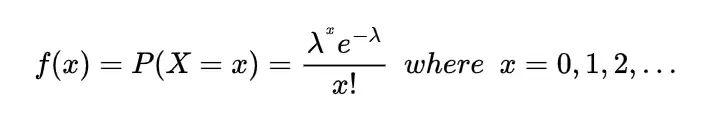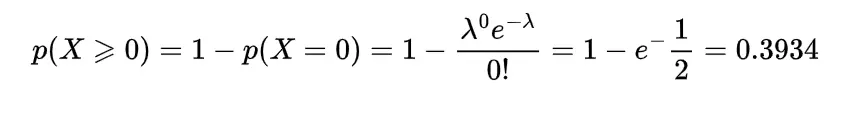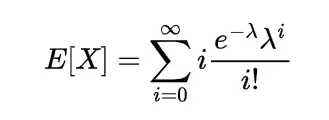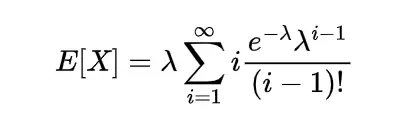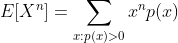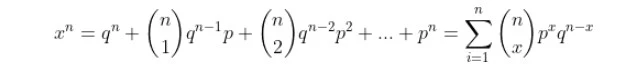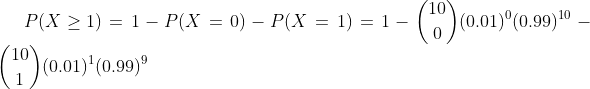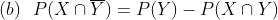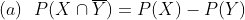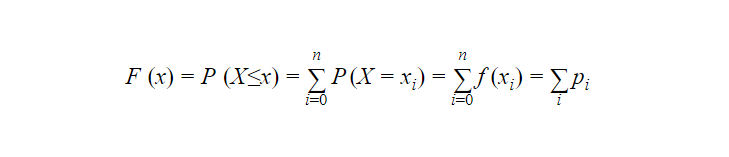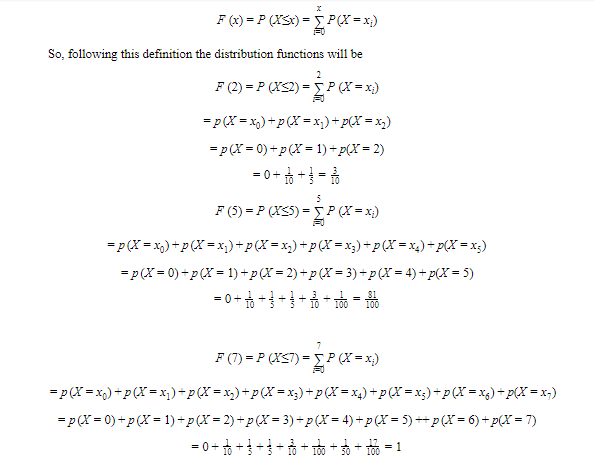A brief Description of Probability theory

In the previous articles, the probability we discussed was at very basic level, Probability is a means of expressing information that an occurrence of an event has occurred, In pure mathematics the concept of probability has been described in the form of probability theory which is widely used in the areas of real life as well as different branches of philosophy, science, gambling, finance, statistics and mathematics etc. for finding the likelihood of main events.

    Probability theory is the branch of mathematics which deals with the random experiment and its outcome, the core objects for dealing such analysis of the random experiment are events, random variable, stochastic processes, non-deterministic events etc.

Providing an example when we toss a coin or die this event although is random but when we repeat such trial number of times the result of such trial or event will result in a particular statistical arrangement which we can predict after studying via law of large numbers or the central limit theorems etc. so likewise we can use probability theory for the day to day activity of human beings e.g. large set of data can be analyze by quantitative analysis, for  explanation of those systems for which we have insufficient information we can use probability theory e.g. complex systems in statistical mechanics, for physical phenomena of atomic scales in quantum mechanics. 

    There are number of real life situations as well as applications where the probabilistic situation occurs the probability theory will be used provided the familiarity of the concept and the handling of the results and relations of probability theory.  In following we will get some differentiation of the situations with the help of some terms in probability theory.     

Discrete probability

Discrete probability theory is the study of random experiments in which the result can be counted numerically, so here the restriction is the events whatever occurred must be countable subset of given sample space. It includes the experiment of throwing coin or dice, random walk, picking cards from deck, balls in bags etc.

Continuous probability

Continuous probability theory is the study of random experiments in which the result is within the continuous intervals, so here restriction is the events whatever occurred must be in the form of continuous intervals as a subset of sample space.

Measure-theoretic probability

The Measure theoretic probability theory deals with the any of discrete and continuous random outcome, and differentiate in which situation what measure have to be used. The measure theoretic probability theory also deals with the probability distributions which is neither discrete nor continuous nor the mixture of both.

     So to study the probability we must know first of all what is the nature of random experiment either that is discrete, continuous or mixture of both or neither, depending on this we can set our strategies which way we have to follow.  we will discuss all the situation consecutively one by one.

EXPERIMENT

Any action that produces a result or an outcome is called as experiment. There are two types of experiment.

Deterministic Experiments	Non-deterministic Experiments (or Random Experiments)
Any experiment whose outcome we can predict in advance under some conditions.	Any experiment whose outcome or result we cannot able to predict in advance.
For example flow of current in specific circuit based on the power provided we know by some physical laws.	For example tossing of an unbiased coin we don’t know head will come or tail
We don’t need probability theory for such experiments outcome.	We need probability theory for such experiments outcome.

Theory of Probability is basically depending on the model of a random experiment, that implies an experiment whose outcome is unpredictable with certainty, before the experiment is run. People normally thinks that the experiment can be recurrent forever under fundamentally the same circumstances.   

   This presumption is important because the theory of Probability is concerned with the long-term practices as the experiment is recreated. Naturally, a proper definition of a random experiment needs a careful definition of specifically what information about the experiment is being recorded, that is, a careful definition of what constitutes an outcome.

SAMPLE SPACE

As already discussed Sample space is nothing but the set having all possible outcomes of non-deterministic or random experiment. In mathematical analysis random variable which is outcome of such experiment is a real valued function denoted by X i.e X:A ⊆ S → ℝ  that we will discuss in detail later.  Here also we can categorize sample space as finite or infinite.  Infinite sample spaces can be discrete or continuous.

Finite Sample Spaces	Infinite Discrete Sample Spaces
Tossing a coin or anything with two different result {H, T}	Repeatedly tossing a coin until first head shows possible outcome may be {H,TH,TTH,TTTH,…………}
Throwing a die {1, 2, 3, 4, 5, 6}	Throwing a die repeatedly till 6 come
Drawing a card from a deck of 52 cards	Drawing a card and replacing till queen come
Choosing a birthday from a year {1, 2, 3, 4, …, 365}.	Arriving time of two consecutive trains

EVENT

Event as already we know is subset of the sample space of random experiment for which we are discussing the probability.  In other word we can say any element in the power set of sample space for finite sample space is Event and for infinite we have to exclude some subsets.

Independent events	Dependent Events
If there is no effect of the events to other events	Occurrence of one event affect other events
For example tossing a coin	Drawing a card without returning.
Probabilities of the events also not affected	Probabilities of the events affected
P(A ⋂ B) = P (A) X P(B)	P(A ⋂ B) =P(A) X P(B/A) P(B/A) is the conditional prob. of B given A

RANDOM VARIABLE

The understanding of random variable is very important for the study of probability theory. Random variable  is very helpful to generalized the concept of probability which gives mathematical property to probabilities questions  and the use of measure theoretic probability is based on random variable.  Random variable which is outcome of random experiment is a real valued function denoted by X i.e X:A ⊆ S → ℝ

Discrete Random Variable	Continuous Random Variable
Countable outcome of random experiment	Outcome of random experiment in range
For a coin toss, the possible events are heads or tails. so random variable takes the values : X=1 if heads and X=0 if tails	a real number between zero and one
For throwing a die X=1,2,3,4,5,6	For the time of travelling X=(3,4)

A random variable can be thought of as an unknown value that may change every time it is inspected. Thus, a random variable can be thought of as a function mapping the sample space of a random process to the real numbers.

Probability Distributions

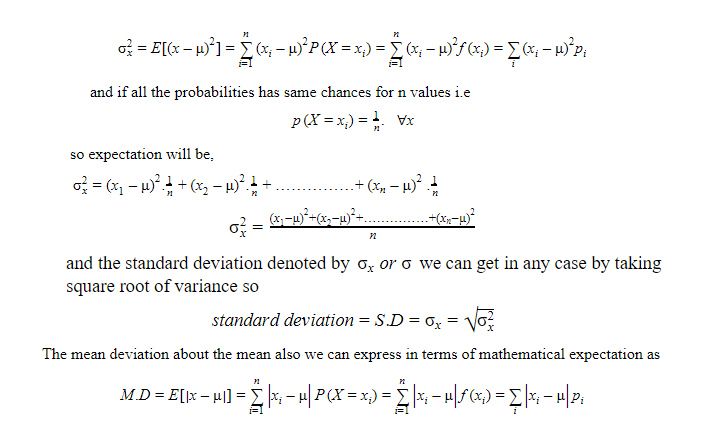
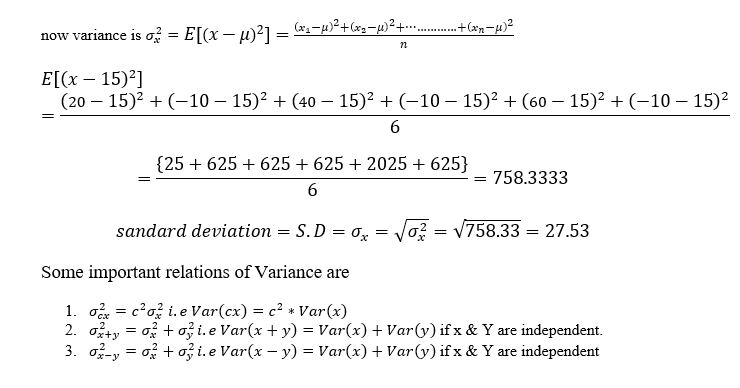
Probability distribution is defined as the collection of random variable with its probability,

so obviously depending on the nature of random variable we can categorize as

Discrete Probability Distribution	Continuous Probability Distribution
If random variable is discrete then probability distribution is known as discrete probability distribution	If random variable is continuous then probability distribution is known as continuous probability distribution
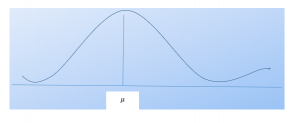
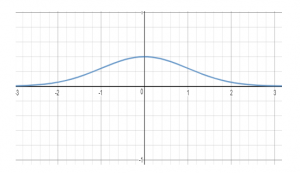
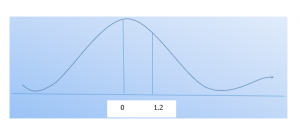
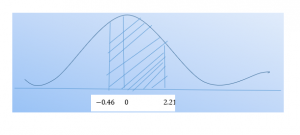
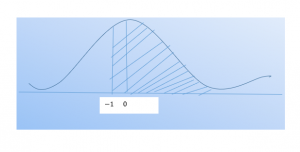
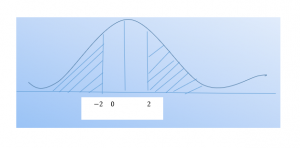
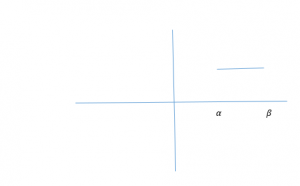
For example number of tails for tossing a coin two times  can be distributed as result will be TT,HH,TH,HT X(no of tails): 0 1 2 P(x) : 1/4 1/2 1/3	A continuous probability distribution differs from a discrete probability distribution so for the random variable X ≤ a its probability P(X ≤ a) can be considered as the area under the curve (See the below image)

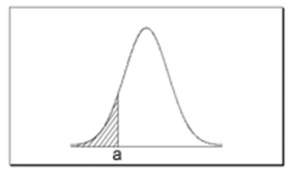
      In the similar way for dealing with probability of random variable depends on the nature of random variable, so the concepts we are using will depend on the nature of random variable.

Conclusion:

   In this article we mainly discuss the scenario of probability, how we can deal the probability and some concept comparatively. Before discussing the core subject this discussion is important so that the problems we deal stands where we know clearly. In the consecutive articles we relate probability to random variable and some familiar terms related to probability theory we will discuss, if you want further reading then go through:

Schaum’s Outlines of Probability and Statistics

https://en.wikipedia.org/wiki/Probability

For more topics on mathematics please check this page.

Normal Random variable and Normal distribution

      The random variable with uncountable set of values is known to be continuous random variable, and the probability density function with the help of integration as area under the curve gives the continuous distribution, Now we will focus one of the most used and frequent continuous random variable viz normal random variable which has another name as Gaussian random variable or Gaussian distribution.

Normal random variable

      Normal random variable is the continuous random variable with probability density function

having mean μ and variance σ² as the statistical parameters and geometrically the probability density function has the bell shaped curve which is symmetric about the mean μ.

We know that probability density function has the total probability as one so

by putting y= (x-μ)/σ

this double integration can be solved by converting it  into polar form

which is the required value so it is verified for the integral I.

• If X is normally distributed with parameter μ  and σ² then Y=aX+b is also normally distributed with the parameters  aμ+b and a²μ²

Expectation and Variance of Normal Random variable

The Expected value of the normal random variable and the variance we will get with the help of

where X is normally distributed with the parameters mean μ and standard deviation σ.

since mean of Z is zero so we have the variance as

by using integration by parts

for the variable Z the graphical interpretation is as follows

and the area under the curve for this variable Z which is known as standard normal variable, it is calculated for the reference (given in the table), as the curve is symmetric so for negative value the area will be same as that of positive values

z	0.00	0.01	0.02	0.03	0.04	0.05	0.06	0.07	0.08	0.09
0.0	0.50000	0.50399	0.50798	0.51197	0.51595	0.51994	0.52392	0.52790	0.53188	0.53586
0.1	0.53983	0.54380	0.54776	0.55172	0.55567	0.55962	0.56356	0.56749	0.57142	0.57535
0.2	0.57926	0.58317	0.58706	0.59095	0.59483	0.59871	0.60257	0.60642	0.61026	0.61409
0.3	0.61791	0.62172	0.62552	0.62930	0.63307	0.63683	0.64058	0.64431	0.64803	0.65173
0.4	0.65542	0.65910	0.66276	0.66640	0.67003	0.67364	0.67724	0.68082	0.68439	0.68793
0.5	0.69146	0.69497	0.69847	0.70194	0.70540	0.70884	0.71226	0.71566	0.71904	0.72240
0.6	0.72575	0.72907	0.73237	0.73565	0.73891	0.74215	0.74537	0.74857	0.75175	0.75490
0.7	0.75804	0.76115	0.76424	0.76730	0.77035	0.77337	0.77637	0.77935	0.78230	0.78524
0.8	0.78814	0.79103	0.79389	0.79673	0.79955	0.80234	0.80511	0.80785	0.81057	0.81327
0.9	0.81594	0.81859	0.82121	0.82381	0.82639	0.82894	0.83147	0.83398	0.83646	0.83891
1.0	0.84134	0.84375	0.84614	0.84849	0.85083	0.85314	0.85543	0.85769	0.85993	0.86214
1.1	0.86433	0.86650	0.86864	0.87076	0.87286	0.87493	0.87698	0.87900	0.88100	0.88298
1.2	0.88493	0.88686	0.88877	0.89065	0.89251	0.89435	0.89617	0.89796	0.89973	0.90147
1.3	0.90320	0.90490	0.90658	0.90824	0.90988	0.91149	0.91308	0.91466	0.91621	0.91774
1.4	0.91924	0.92073	0.92220	0.92364	0.92507	0.92647	0.92785	0.92922	0.93056	0.93189
1.5	0.93319	0.93448	0.93574	0.93699	0.93822	0.93943	0.94062	0.94179	0.94295	0.94408
1.6	0.94520	0.94630	0.94738	0.94845	0.94950	0.95053	0.95154	0.95254	0.95352	0.95449
1.7	0.95543	0.95637	0.95728	0.95818	0.95907	0.95994	0.96080	0.96164	0.96246	0.96327
1.8	0.96407	0.96485	0.96562	0.96638	0.96712	0.96784	0.96856	0.96926	0.96995	0.97062
1.9	0.97128	0.97193	0.97257	0.97320	0.97381	0.97441	0.97500	0.97558	0.97615	0.97670
2.0	0.97725	0.97778	0.97831	0.97882	0.97932	0.97982	0.98030	0.98077	0.98124	0.98169
2.1	0.98214	0.98257	0.98300	0.98341	0.98382	0.98422	0.98461	0.98500	0.98537	0.98574
2.2	0.98610	0.98645	0.98679	0.98713	0.98745	0.98778	0.98809	0.98840	0.98870	0.98899
2.3	0.98928	0.98956	0.98983	0.99010	0.99036	0.99061	0.99086	0.99111	0.99134	0.99158
2.4	0.99180	0.99202	0.99224	0.99245	0.99266	0.99286	0.99305	0.99324	0.99343	0.99361
2.5	0.99379	0.99396	0.99413	0.99430	0.99446	0.99461	0.99477	0.99492	0.99506	0.99520
2.6	0.99534	0.99547	0.99560	0.99573	0.99585	0.99598	0.99609	0.99621	0.99632	0.99643
2.7	0.99653	0.99664	0.99674	0.99683	0.99693	0.99702	0.99711	0.99720	0.99728	0.99736
2.8	0.99744	0.99752	0.99760	0.99767	0.99774	0.99781	0.99788	0.99795	0.99801	0.99807
2.9	0.99813	0.99819	0.99825	0.99831	0.99836	0.99841	0.99846	0.99851	0.99856	0.99861
3.0	0.99865	0.99869	0.99874	0.99878	0.99882	0.99886	0.99889	0.99893	0.99896	0.99900
3.1	0.99903	0.99906	0.99910	0.99913	0.99916	0.99918	0.99921	0.99924	0.99926	0.99929
3.2	0.99931	0.99934	0.99936	0.99938	0.99940	0.99942	0.99944	0.99946	0.99948	0.99950
3.3	0.99952	0.99953	0.99955	0.99957	0.99958	0.99960	0.99961	0.99962	0.99964	0.99965
3.4	0.99966	0.99968	0.99969	0.99970	0.99971	0.99972	0.99973	0.99974	0.99975	0.99976
3.5	0.99977	0.99978	0.99978	0.99979	0.99980	0.99981	0.99981	0.99982	0.99983	0.99983

since we have used the substitution

Here keep in mind that Z is standard normal variate where as continuous random variable X is normally distributed normal random variable with mean μ and standard deviation σ.

So to find the distribution function for the random variable we will use the conversion to the standard normal variate as

for any value of a.

Example: In the standard normal curve find the area between the points 0 and 1.2.

If we follow the table the value of 1.2 under the column 0 is 0.88493 and value of 0 is 0.5000 ,

Example: find the area for the standard normal curve within -0.46 to 2.21.

From the shaded region we can bifurcate this region from -0.46 to 0 and 0 to 2.21 because the normal curve is symmetric about y axis so the area from -0.46 to 0 is same as the are from 0 to 0.46 thus from the table

and

so we can write it as

Total Area =(area between z = -0.46 and z=0 ) + (area between z =0 and z=2.21)

= 0.1722 + 0.4864

= 0.6586

Example: If X is normal random variable with mean 3 and variance 9 then find the following probabilities

P2<X<5

P{X>0}

P|X-3|>6

Solution:  since we have

so bifurcating into the intervals -1/3 to 0 and 0 to 2/3 we will get the solution from the tabular values

or

=0.74537 -1 + 0.62930 =0.37467

and

Example: An observer in paternity case states that the length (in days) of human growth

is normally distributed with parameters mean  270 and variance 100. In this case the suspect  who is father of the child provided the proof that he was out of the country during a period that started 290 days before the birth of the child and ended 240 days earlier the birth. Find the probability that the mother could have had the very long or very short pregnancy indicated by the witness?

Let X denote the normally distributed random variable for gestation and consider the suspect is the father of the child. In that case the birth of the child happened within the specified time has the probability

Relation between Normal random variable and Binomial random variable

      In case of Binomial distribution the mean is np and the variance is npq so if we convert such binomial random variable with such  mean and standard deviation having n very large and p or q are very small going nearer to zero then standard normal variable Z with the help of these mean and variance is

here in terms of Bernouli trials X considers the number of successes in n trials. As n is increases and goes nearer to infinity this normal variate goes in the same way to become standard normal variate.

The relation of binomial and standard normal variate we can find with the help of following theorem.

DeMoivre Laplace limit theorem

If S_n denotes the number of successes that occur when n  independent trials, each resulting in a success with probability p , are performed, then, for any a < b ,

Example: With the help of normal approximation to the binomial random variable find the probability of occurrence of 20 times tail when a fair coin tossed 40 times.

Solution: Suppose the random variable X represents the occurrence of tail, since the binomial random variable is discrete random variable and normal random variable is continuous random variable so to convert the discrete into the continuous, we write it as

and if we solve the given example with the help of binomial distribution we will get it as

Example: To decide the efficiency of a definite nourishment in decreasing the extent of cholesterol in the blood circulation, 100 people are placed on the nourishment. The cholesterol count were observed for the define time after providing the nourishment. If from this sample 65 percent have low cholesterol count then nourishment will be approved. What is the probability that the nutritionist approves the new nourishment if, actually, it has no consequence on the cholesterol level?

solution:  Let the random variable express the cholesterol level if down by the nourishment so the probability for such random variable will be ½ for each person, if X denotes the low level number of people then the probability that result approved even there is no effect of nourishment to reduce the level of cholesterol is

Conclusion:

   In this article the concept of continuous random variable namely normal random variable and its distribution with probability density function were discussed and the statistical parameter mean, variance for the normal random variable is given. The conversion of normally distributed random variable to the new standard normal variate and area under the curve for such standard normal variate is given in tabulated form one of the relation with discrete random variable is also mentioned with example ,if you want further reading then go through:

Schaum’s Outlines of Probability and Statistics

https://en.wikipedia.org/wiki/Probability.

For more topics on mathematics please check this page.

Continuous random variable, types and its distribution

     The random variable which takes the finite or countably infinite values is known as discrete random variable and its pair with probability forms the distribution for the discrete random variable. Now for the random variable who takes the values as uncountable, what would be the probability and remaining characteristics that we are going to discuss.  Thus in brief the continuous random variable is the random variable whose set of values are uncountable. The real life example for the continuous random variable is the life span of electrical or electronic components and arrival of specific public vehicle on the stops etc.

Continuous random variable and probability density function

                Random variable  will be continuous random variable if for a non-negative real valued function f on x ∈ ℝ and B ⊆ ℝ and

this function f is known as Probability density function  of the given random variable X.

The probability density function obviously satisfies the following probability axioms

Since from the axioms of the probability we know that the total probability is one so

For the continuous random variable the probability will be calculated in terms of such function f, suppose we want to find the probability for the continuous interval say [a, b] then it would be

As we know the integration represents the area under the curve so this probability shows such area for the probability like

by equating a=b the value will be

and in similar way the probability for the value less than or equal to specific value by following this will be

Example: The continuous working time of the electronic component is expressed in the form of continuous random variable and the probability density function is given as

find the probability that the component will work effectively between 50 to 150 hours and the probability of less than 100 hours.

since the random variable represents the continuous random variable so the probability density function given in the question gives the total  probability as

So we will get the value of λ

λ =1/100

for the probability of 50 hrs to 150hrs we have

in the similar way the probability less than 100 will be

Example: The computer based device has number of chipsets with lifespan given by the probability density function

then after 150 hours find the probability that we have to replace 2 chipset from total 5 chips.

let us consider E_i be the event to replace the i-th chipset. so the probability of such event will be

as working of all the chips independent so the probability for 2 to be replace will be

Cumulative distribution function

  Cumulative distribution function for the continuous random variable is defined with the help of probability distribution function as

in another form

we can obtain the probability density function with the help of distribution function as

Mathematical Expectation and Variance of continuous random variable

Expectation

The mathematical expectation or mean of the continuous random variable  with probability density function  can be define as

• For any real valued function of continuous random variable X expectation will be

where g is the real valued function.

1. For any non-negative continuous random variable Y the expectation will be

• For any constants a and b

E[aX + b] = aE[X] + b

Variance

                The variance of the continuous random variable X with the parameter mean or expectation  can be define in the similar way as discrete random variable is

   The proof of all the above properties of expectation and variance we can easily obtain by just following the steps we have in discrete random variable and the definitions of expectation, variance and probability in terms of continuous random variable

Example: If the probability density function of continuous random variable X is given by

then find the expectation and variance of the continuous random variable X.

Solution:  For the given probability density function

the expected value by the definition will be

Now to find the variance we require E[X²]

Since

so

Uniform random variable

    If the continuous random variable X is having the probability density function given by

over the interval (0,1) then this distribution is known as uniform distribution and the random variable is known as uniform random variable.

• For any constants a and b such that 0<a<b<1

Expectation and Variance of Uniform random variable

      For the uniformly continuous random variable X on the general interval (α , β) the expectation by the definition will be

and variance we will get if we find first E[X²]

so

Example: At a particular station the trains for the given destination arrive with frequency of 15 minutes form 7 A.M. For the passenger who is on the station at a time between 7 to 7.30 distributed uniformly what will be the probability that the passenger get train within 5 minutes and what will be probability for more than 10 minutes.

Solution: As the time from 7 to 7.30 is distributed uniformly for the passenger to be at railway station denote this by uniform random variable X. so the interval will be (0, 30)

Since to get the train within 5 minutes passenger must be at the station between 7.10 to 7.15 or 7.25 to 7.30 so the probability will be

=1/3

In similar manner to get the train after waiting more than 10 minutes passenger must be at the station from 7 to 7.05 or 7.15 to 7.20 so the probability will be

Example: Find the probability for the uniform random variable X distributed over the interval (0,10 )

for X<3, X>6 and 3<X<8.

Solution: since the random variable is given as uniformly distributed so the probabilities will be

Example: (Bertrands Paradox) For any random chord of a circle. what would be the probability that the length of that random chord will be greater than the side of the equilateral triangle inscribed in the same circle.

This problems does not have clearance about the random chord so this problem were reformulated in terms of diameter or angle and then answer as 1/3 were obtained.

Conclusion:

   In this article the concept of continuous random variable and its distribution with probability density function were discussed and the statistical parameter mean, variance for the continuous random variable is given. The uniform random variable and its distribution with example is given which is the type of continuous random variable in the successive article we will focus some important types of continuous random variable with suitable examples and properties. ,if you want further reading then go through:

Schaum’s Outlines of Probability and Statistics

https://en.wikipedia.org/wiki/Probability

If you want to read more topics on Mathematics then go through Mathematics Page.

Some additional discrete random variable and its parameters

    The discrete random variable with its probability mass function combines the distribution of the probability and depending on the nature of the discrete random variable the probability distribution may have different names like binomial distribution, Poisson distribution etc., as already we has seen the types of discrete random variable, binomial random variable and Poisson random variable with the statistical parameters for these random variables. Most of the random variables are characterized depending on the nature of probability mass function, now we will see some more type of discrete random variables and its statistical parameters.

Geometric Random variable and its distribution

      A geometric random variable is the random variable which is assigned for the independent trials performed till the occurrence of success after continuous failure i.e if we perform an experiment n times and getting initially all failures n-1 times and then at the last we get success.  The probability mass function for such a discrete random variable will be

In this random variable the necessary condition for the outcome of the independent trial is the initial all the result must be failure before success.

Thus in brief the random variable which follows above probability mass function is known as geometric random variable.

It is easily observed that the sum of such probabilities will be 1 as the case for the probability.

Thus the geometric random variable with such probability mass function is geometric distribution.

Know more about Continuous random variable

Expectation of Geometric random variable

    As expectation is one of the important parameter for the random variable so the expectation for the geometric random variable will be 

E[X]=1/p

where p is the probability of success.

since

let the probability of failure be q=1-p

so

E[X]=qE[X]+1

(1-q)E[X]=1

pE[X]=1

thus we get

Thus the expected value or mean of the given information we can follow by just inverse value of probability of success in geometric random variable.

To get details about Normal Random Variable

Variance and standard deviation of the geometric random variable

In similar way we can obtain the other important statistical parameter variance and standard deviation for the geometric random variable and it would be

and

To obtain these values we use the relation

So let us calculate first

E[X²]

set q=1-p

so

thus we have

Negative Binomial Random Variable

    This random falls in another discrete random variable because of the nature of its probability mass function, in the negative binomial random variable and in its distribution from n trial of an independent experiment r successes must be obtained initially

In other words a random variable with above probability mass function is negative binomial random variable with parameters (r,p), note that if we restrict r=1 the negative binomial distribution turns to geometric distribution, we can specifically check

Expectation, Variance and standard deviation of the negative binomial random variable

The expectation and variance for the negative binomial random variable will be

with the help of probability mass function of negative binomial random variable and definition of expectation we can write

here Y is nothing but the negative binomial random variable now put k=1 we will get

Thus for variance

Exxample: If a die is throw to get 5 on the face of die till we get 4 times this value find the expectation and variance.Sine the random variable associated with this independent experiment is negative binomial random variable for r=4 and probability of success p=1/6 to get 5 in one throw

as we know for negative binomial random variable 

Hypergeometric random variable

       If we particularly choosing a sample of size n from a total N having m and N-m two types then the random variable for first was selected have the probability mass function as

for example suppose we have a sack from which a sample of size n books taken randomly without replacement containing N books of which m are mathematics and N-m are physics, If we assign the random variable to denote the number of mathematics books selected then the probability mass function for such selection will be as per above probability mass function.

  In other words the random variable with the above probability mass function is known to be the hypergeometric random variable.

Read more about Jointly Distributed Random Variables

Example: From a lot of some electronic components if 30% of the lots have four defective components and 70% have one defective, provided size of lot is 10 and to accept the lot three random components will be chosen and checked if all are non-defective then lot will be selected. Calculate that from the total lot what percent of lot get rejected.

here consider A is the event to accept the lot

N=10, m=4, n=3

for N=10, m=1, n=3

Thus the 46% lot will be rejected.

Expectation, Variance and standard deviation of the hypergeometric random variable

    The expectation, variance and standard deviation for the hypergeometric random variable with parameters n,m, and N would be

or  for the large value of N

and standard deviation is the square root of the variance.

By considering the definition of probability mass function of hypergeormetric function and the expectation we can write it as

here by using the relations and identities of the combinations we have

here Y plays the role of hypergeometric random variable with respective parameters now if we put k=1 we will get

E[X] = nm/N

and for k=2

so variance would be

for p=m/N and

we get

for very large value of N it would obviously

Zeta (Zipf) random variable

        A discrete random variable is said to be Zeta if its probability mass function is given by

for the positive values of alpha.

In the similar way we can find the values of the expectation, variance and standard deviation.

     In the similar way by using just the definition of the probability mass function and the mathematical expectation we can summarize the number of properties for the each of discrete random variable for example expected values of sums of random variables as

For random variables

$ X₁,X₂, X₃…$

Conclusion:

   In this article we mainly focused on some additional discrete random variable, its probability mass functions, distribution and the statistical parameters mean or expectation, standard deviation and variance,  The brief introduction and simple example we discussed to give just the idea the detail study remains to discuss In the next articles we will move on continuous random variables and concepts related to continuous random variable ,if you want further reading then go through suggested link below. For more topics on mathematics, please this link.

Schaum’s Outlines of Probability and Statistics

https://en.wikipedia.org/wiki/Probability

Binomial & Poisson random variable and its properties

    The random variable which deals with the success and failure outcome of the random experiment for n repetitions were known to be Binomial random variable the definition of its probability mass function deals with the probability of success p and probability of failure q only, the definition with examples already we has seen, now with the understanding we see some of the properties of such discrete random variable,

Expectation and Variance of the binomial random variable

Expectation and Variance of binomial random variable with n repetition and p as probability of success are

E[X]= np

and Var(X) = np(1-p)

now consider for showing these two the expectation of random variable of power k by following  the definition of probability mass function for binomial random variable as,

where Y is another binomial random variable with n-1 trials and p as the probability of success, If we take the value of k=1 then we will get

E[X]= np

and if we substitute k=2 we will get

E[X²] =npE[Y + 1]

=np[(n-1)p + 1]

so we will get easily

Var(X)=E[X²] – (E[X])²

=np[(n-1)p + 1] -(np)²

=np(1-p)

Example: For an unbiased coin do the experiment of tossing 100 times and for the number of tails that appearing in this case find the mean, variance and standard deviation of such experiment.

The tail for one toss has the probability of success p=1/2=0.5

so the mean of such experiment is

E[X]= np

since the experiment is binomial as only success or failure we will get for n number of repetitions

so as μ=np

μ=100x(0.5)=50

Similarly the variance and the standard deviation will be

Var(X)= np(1-p)

σ²= np(1-p)

The value would be

σ² =(100)(0.5)(0.5)=25

Example:     Find the mean and standard deviation for the probability of 0.1 defectiveness in bolt manufacturing company from the lot of 400 bolt.

here n=400, p=0.1, mean= np =400×0.1=40

since

σ²= np(1-p)

so standard deviation will be

Example: Find the probability of exactly, less than and at least 2 successes if the mean and standard deviation for the binomial random variable is 4 and 2 respectively.

Since mean = np= 4

and variance = np(1-p) = 2,

so 4(1-p)=2

(1-p)=1/2

p=1-(1/2)

putting this value in mean we get

np = 4

n(1/2)=4

n=8

probability of exactly 2 successes will be

probability of less than 2 successes will be

p(X < 2)

=P(0) +P(1) = ⁸C₀ p⁰q⁸ + ⁸C₁ p¹q⁷

=(1/256)+8 x (1/2) x (1/2)⁷ = 9/256

Probability of at least 2 successes

p(X>2)= 1- p(X<2)

=1-P(0) – P(1)= 1-[P(0) + P(1)] =1- (9/256)=247/256

Poisson Random Variable

    The discrete random variable X that takes the values 0,1,2…….. is known to be Poisson Random variable provided for any λ>0 its probability mass function must be

or

as

When n is very large and the probability of success p is very small in such case Poisson random variable with its probability mass function became the approximation of binomial random variate with respective p.m.f. because the expectation in this case which is np will be moderate and that would be λ= np .

Example: Find the probability that there is at least one typing error on each page of the book which has Poisson distribution with mean 1/2 for a single page.

Let the discrete random variable X denote the errors on the page. so the Poisson random variable has the probability mass function as

λ = 1/2

Example: Find the probability that the sample of 10 items produced by a machine with 0.1 chances of defective production has at the most one defective item.

This we can solve both by binomial probability mass function as well as Poisson probability mass function, so we solve this by Poisson

Expectation and variance of the Poisson random variable

Expectation and Variance of Poisson random variable with n repetition and p as probability of success are

E[X]= np= λ

and          

Var(X) = np= λ

Before showing the result the we must keep in mind that the Poisson random variable is nothing but the approximation of Binomial random variable so np= λ now expectation by using the probability mass function will be

This means the mathematical expected value of Poisson random variable is equal to its parameter, similarly for calculating the variance and standard deviation of Poisson random variable we require expectation of square of X so,

The above summation is obvious as two of the sums are expectation and sum of the probabilities.

Thus the value of variance we will get is

Var(X) = E[X²] – (E[X])²

=λ

so in the case of Poisson random variable the mean and variance have the same value i.e np as a parameter.

The Poisson random variable is the approximation good for the finding of diverse processes e.g. finding the occurrence of number of earthquakes within some specific time duration, finding the number of electron during a fixed time from the heated cathode, finding the possible number of deaths during specified time, or number of wars within specific year e.t.c.

Example : Calculate the probability that the total number of passengers in two days is less than 2. If the number of arrival of passengers with mean 5 follows Poisson random variable. mean=np=5

If we consider the number of passengers in two days less than 2 it would be

First day	Second day	In total
0	0	0
0	1	1
1	0	1

so the probability will be the combination of these two days as

=e^-10[1+5+5]

=11e^-10

=114.5410^-5

=4.994*10^-4

Example: Calculate the probability of 4 or more faulty condensers from a pack of 100 condensers provided the manufacturing defect for the condensers is 1%.

Here p=1% =0.01 and n= 100 * 0.01 =1

so we can use the Poisson random variables probability mass function P.M.F

mean= np = 100*0.01=1

so the probability for 4 or more faulty condensers will be

=1-[P(0)+P(1)+P(2)+P(3)]

Example: If 0.002 chances are there for a product to be defective from the manufacturing, for a pack containing 10 of such products what would be the probability that such a packet has no defective, one defective, and two defective products from the consignment of 50000 packets of same product.

Here for a single pack probability of defect i.e p=0.002, n=10

then the mean np=0.002*10= 0.020

we will find for each case as

So from the table it is clear that the number of defective blades in packets zero, one and two will be 4900,980,10 respectively.

Conclusion:

   In this article we discussed some properties of one of Binomial random variable, Poisson random variable and Random Experiment.  Also one more discrete random variable i.e Poisson random variable, discussed with properties. The distribution for the probability mass function, expectation , variance and standard deviation example also taken for better understanding , In the next articles we try to cover some more discrete random variables if you want further reading then go through Mathematics Page.

Schaum’s Outlines of Probability and Statistics

https://en.wikipedia.org/wiki/Probability

Discrete Random Variable and Mathematical Expectation-II

As already we now familiar with the discrete random variable, it is the random variable which takes countable number of possible values in a sequence. The two important concept related to the discrete random variables are the probability of discrete random variable and distribution function we restrict the name to such probability and distribution function as,

Probability Mass function (p.m.f)

                The Probability Mass function is the probability of the discrete random variable, so for any discrete random variables  x₁, x₂, x₃, x₄,……, x_k  the corresponding probabilities P(x₁), P(x₂), P(x₃), P(x₄)……, P(x_k) are the corresponding probability mass functions.

Specifically, for X=a, P(a)=P(X=a) is its p.m.f

We here onwards use probability mass function for discrete random variables probability.  All the probability characteristics for the probability will obviously applicable to probability mass function like positivity and summation of all p.m.f will be one e.t.c.

Cumulative Distribution Function (c.d.f)/Distribution Function

  The distribution function defined as

F(x)=P(X<=x)

for discrete random variable with probability mass function is the cumulative distribution function (c.d.f.) of the random variable.

and mathematical expectation for such random variable we defined was

we now see some of the results of mathematical expectations

1. If x₁, x₂, x₃, x₄,….. are the discrete random variables with respective probabilities P(x₁), P(x₂), P(x₃), P(x₄) … the expectation for the real valued function g will be

Example: for the following probability mass functions find the E(X³)

Here the g(X)=X³

So,

E (X³) = (-1)³ <em>0.2 + (0)³</em> 0.5 + (1)³ * 0.3

E (X³) = 0.1

In the similar way for any nth order we can write

Which is known as nth moment.

2. If a and b are constants then

E[aX + b]=aE[X] + b

This we can understand easily as

=aE[X] + b

Variance in terms of Expectation.

                For the mean denoted by μ the variance of the discrete random variable X denoted by var(X) or σ in terms of expectation will be

Var(X) =E[(X- μ)²]

and this we can further simplify as

Var(X) =E[(X- μ)²]

= E [X²] – 2μ² + μ²

= E [X²] – μ²

this means we can write the variance as the difference of the expectation of random variable square and square of expectation of random variable.

i.e. Var (X)= E[X²] – (E[X])²

Example:  when a die is thrown calculate the variance.

Solution:  here we know when die thrown the probabilities for each face will be

p(1)=p(2)=p(3)=p(4)=p(5)=p(6)=1/6

hence for calculating variance we will find expectation of random variable and its square as

E[X]=1.(1/6)+2.(1/6)+3.(1/6)+4.(1/6)+5.(1/6)+6.(1/6)=(7/2)

E[X²] =1².(1/6)+2².(1/6)+3².(1/6)+4².(1/6)+5².(1/6)+6².(1/6) =(1/6)(91)

and we just obtained the variance as

Var (X) =E[X²] – (E[X])²

so

Var (X)=(91/6) -(7/2)² =35/12

One of the important identity for variance is

1. For the arbitrary constants a and b we have

Var(aX + b) =a² Var(X)

This we can show easily as

Var(aX + b) =E[(aX+ b -aμ-b)² ]

=E[a²(X – μ)²]

=a² E[(X-μ)²]

=a² Var(X)

Bernoulli Random variable

      A Swiss mathematician James Bernoulli define the Bernoulli random variable as a random variable having either success or failure as only two outcomes for the random experiment.

i.e When the outcome is success X=1

When the outcome is failure X=0

So the probability mass function for the Bernoulli random variable is

p(0) = P{X=0}=1-p

p(1) =P{X=1}=p

where p is the probability of success and 1-p will be the probability of failure.

Here we can take 1-p=q also where q is the probability of failure.

As this type of random variable is obviously discrete so this is one of discrete random variable.

Example: Tossing a coin.

Binomial Random Variable

If for a random experiment which is having only outcome as success or failure we take n trials so each time we will get either success or failure then the random variable X representing outcome for such n trial random experiment is known as Binomial random variable.

                In other words if p is the probability mass function for the success in  the single Bernoulli trial and q=1-p is the probability for the failure then the probability for happening of event ‘x or i’ times in n trials will be

or

Example: If we toss two coins six times and getting head is success and remaining occurrences are failures then its probability will be

in the similar way we can calculate for any such experiment.

The Binomial random variable is having the name Binomial because it represent the expansion of

If we put in place of n=1 then this would turn into the Bernoulli’s random variable.

Example: If five coins were tossed and the outcome is taken independently then what would be the probability for number of heads occurred.

Here if we take random variable X as the number of heads then it would turns to the binomial random variable with n=5 and probability of  success as ½

So by following the probability mass function for the binomial random variable we will get

Example:

In a certain company the probability of defective is 0.01 from the production. The company manufacture and sells the product in a pack of 10 and to its customers offer money back guarantee that at most 1 of the 10 product is defective, so what proportion of sold products pack the company must replace.

Here If X is the random variable representing the defective products then it is of the binomial type with n=10 and p=0.01 then the probability that the pack will return is

Example: (chuck-a-luck/ wheel of fortune) In a specific game of fortune in hotel a player bet on any of the numbers from 1 to 6, three dice then rolled and if the number appears bet by the player once, twice or thrice the player that much units means if appear once then 1 unit if on two dice then 2 units and if on three dice then 3 units, check with the help of probability the game is fair for the player or not.

If we assume there will be no unfair means with the dice and con techniques then by assuming the outcome of the dice independently the probability of success for each dice is 1/6 and failure will be

 1-1/6 so this turns to be the example of binomial random variable with n=3

so first we will calculate the winning probabilities by assigning x as players win

Now to calculate the game is fair for the player or not we will calculate the expectation of the random variable

E[X] = -125+75+30+3/216

= -17/216

This means the probability of losing the game for the player when he plays 216 times is 17.

Conclusion:

   In this article we discussed some of the basic properties of a discrete random variable, probability mass function and variance.  In addition we has seen some types of a discrete random variable, Before we start the continuous random variable we try to cover all the types and properties of discrete random variable, if you want further reading then go through:

Schaum’s Outlines of Probability and Statistics

https://en.wikipedia.org/wiki/Probability

For more Topics on Mathematics, please follow this link

Conditional probability

Conditional probability theory come out from the concept of taking huge risk. there are many issue now a days that stalk from the game of chance, such as throwing coins, throwing a dice, and playing cards. 

Conditional probability theory is applied in many different domains and the flexibility of Conditional probability provides tools for almost so many different needs. probability theory and samples related to the study of the probability of the happening of events.

Consider X and Y both are two events of a incidental experiment. Afterwards, the probability of the happenings of X under the circumstance that Y has already happened with P (Y) ≠ 0, is known as conditional probability and is denoted by P (X / Y).

Therefore, P (X / Y) = The probability of the happening of X, if provided that Y has already happened.

P(X ⋂ Y)/P( Y ) = n(X ⋂ Y)/n (Y )

Similarly, P (Y / X) = The probability of the occurrence of Y, as X has already happened.

P(X ⋂ Y)/P( X ) = n(X ⋂ Y)/n (Y )

In brief for some cases, P (X / Y) is used to specify the probability of the occurrence of X when Y occurs. Similarly, P (Y / X) is used to specify the probability of Y happening while X happens.

What is Multiplication theorem on Probability?

If X and Y both are self-supporting (independent) events of  a arbitrary experiment, then

P( X ⋂ Y) = P( X ). P( X/Y ), if P ( X ) ≠ 0

P( X ⋂ Y) = P( Y ). P( Y/X ), if P ( Y ) ≠ 0

What is Multiplication theorems for independent events? 

If X and Y both are self-supporting (independent) events connected to  a arbitrary experiment, then   P(X ∩ Y) =P(X).P(Y)

i.e., the probability of simultaneous happening of two independent events is equal to the multiplication of their probabilities. By using multiplication theorem, we have  P(X ∩ Y) =P(Y).P(Y/X)

 As X and Y are independent events, therefore P(Y/X)=P(Y)

Implies, P(X ∩ Y) =P(X).P(Y)

While events are mutually exclusive : 

If X and Y are mutually exclusive events, then ⇒ n(X ∩ Y)= 0 , P(X ∩ Y) = 0

P(X U Y)=P(X) +P(Y)

For any three events X, Y, Z which are mutually exclusive, 

P(X ∩ Y)= P(Y ∩ Z) =P(Z ∩ X) =P(X ∩ Y ∩ Z) =0

P (X ⋃ Y ⋃ Z) = P(X) + P(Y) + P(Z)

While events are independent : 

If X and Y are unconstrained (or independent) events, then

P(X ∩ Y) = P(X).P(Y)

P(X U Y) = P(X) + P(Y) – P(X). P(Y)

Let X and Y be two events connected with a arbitrary (or random) experiment, then

If Y⊂ X, then

(b) P(Y) ≤ P(X)

Similarly if X⊂ Y, then

(b) P(X) ≤ P(Y)

Probability of occurrence of neither X nor Y is 

Example: If from a pack of cards a single card is picked. What is the possible chance that it is either a spade or a king?

solution:

P (A) = P (a spade card) =13/52

P (B) = P (a king card) =4/52

P (either a spade or a king card) = P (A or B)

=P(A∪B)=P(A)+P(B)-P(A∩B)

=P(A)+P(B)-P(A)P(B)

=13/52+4/52-{(13/52)*(4/52)}

=4/13

Example: Someone is known to hit the target with 3 out of 4 chances, while another person is known to hit the target with 2 out of 3 chances. Find out if the probability that target are likely to be hit at all when both people are trying.

solution:

 probability of  target hit by first person = P (A) =3/4

probability of  target hit by second person = P (B) =2/3

The two events are not mutually exclusive, since both persons hit the same target   =P (A or B)

=P(A∪B)=P(A)+P(B)-P(A∩B)

=P(A)+P(B)-P(A)P(B)

=3/4+2/3-{(3/4)*(2/3)}

=11/12

Example: If  A  and B are two events such that P(A)=0.4 , P(A+B)=0.7 and P(AB)=0.2 then P(B) ?

solution: Since we have P(A+B)=P(A) +P(B) -P(AB)

=> 0.7=0.4+ P(B)-0.2

=> P(B) =0.5

Example: A card is selected at arbitrary from a pack of cards. What is the possibility of the card being a red color card or a queen.

Solution: Required probability  is

P(Red + Queen)-P(Red ⋂ Queen)

=P(Red) +P(Queen)-P(Red ⋂ Queen)

=26/52+4/52-2/52=28/52=7/13

Example: If the probability of X failing in the test is 0.3 and that the probability of Y is 0.2, then find the probability that X or Y failed in the test?

Solution: Here P(X)=0.3 , P(Y)=0.2

Now P(X ∪ Y)= P(X) +P(Y) -P(X ⋂ Y)

Since these are independent events, so

P(X ⋂ Y) =P(X) . P(Y)

Thus required probability is 0.3+0.2 -0.06=0.44

Example: The chances to fail in Physics are 20% and the possibility to fail in Mathematics are 10%. What are the possibilities to fail in at least one subject ?

Solution: Let P(A) =20/100=1/5, P(B) =10/100=1/10

Since events are independent and we have to find 

P(A ∪ B)=P(A) +P(B) -P(A). P(B)

=(1/5)+(1/10)-(1/5). (1/10)= (3/10)-(1/50)=14/50

So the chance of fail in one subject is (14/50)X 100=28%

Example: The probability of solving a question by three students are 1/2,1/4, and 1/6 respectively. What  will be the possible chance of answering the question?

Solution:

(i) This question can also be solved by one student

(ii) This question can be answered by two students concurrently.

(iii) This question can be answered by three students all together.

P(A)=1/2, P(B)=1/4, P(C)=1/6

P(A ∪ B ∪ C)= P(A) + P(B) +P(C)- [P(A).P(B)+P(B).P(C)+P(C).P(A)] + [P(A).P(B).P(C)]

=(1/2)+(1/4)+(1/6)-[(1/2).(1/4)+(1/4).(1/6)+(1/6).(1/2)] +[(1/2).(1/4).(1/6)] =33/48

Example: A random variable X has the probability distribution

X	1	2	3	4	5	6	7	8
P(X)	0.15	0.23	0.12	0.10	0.20	0.08	0.07	0.05

For the events E ={X is prime number} and F={X<4},  find the probability of P(E ∪ F) .

Solution:

E ={ X is a prime number}

P(E) = P(2) +P(3)+ P(5) +P(7) =0.62

F ={X < 4}, P(F) =P(1)+P(2)+P(3)=0.50

and P(E ⋂ F) = P(2)+ P(3) =0.35

P(E ∪ F) =P(E)+P(F) – P(E ⋂ F)

      = 0.62+0.50 – 0.35 = 0.77

Example: Three coins are tossed. If one of them appears tail, then what would be the possible chance that all the three coins appear tail ?

Solution: Consider E is the event where all the three coins appears tail and F is the event where a coin appears tail. 

F= {HHT, HTH, THH, HTT, THT, TTH, TTT}

and E = {TTT}

Required probability = P(E/F)=P(E ⋂F )/P(E)=1/7

Total probability and Baye’s rule

The law of total probability :

For the sample space S and n mutually exclusive and exhaustive events E₁ E₂ ….E_n related with a random experiment. If X is a specific event which happens with the events E₁ or E₂ or …or E_n, then 

Baye’s rule : 

Consider S be a sample space and E₁, E₂, …..E_n be n incongruous (or mutually exclusive) events such that

and P(E_i) > 0 for i = 1,2,…,n

We can think of E_i’s as the factors leading to the outcome of the an experiment. The probabilities P(E_i), i = 1, 2, ….., n are called known as prior (or earlier) probabilities. If the assessment outcomes in an result of event X, where P(X) > 0. Then we have to perceive the possibility that the perceived the event X was due to cause E_i, that is, we look for the conditional probability P(E_i/X) . These probabilities are known as posterior probabilities, given by Baye’s rule as

Example: There are 3 Boxes which are known to contain 2 blue and 3 green marbles ; 4 blue and 1 green marbles and 3 blue and 7 green marbles respectively. A marble is drawn at random from one of the boxes and found to be a green ball. Then what is the probability that it was drawn from the Box containing the most green marbles.

Solution: Consider the following events :

A ->marble drawn is green;

E₁ -> Box 1 is chosen;

E₂ Box 2 is chosen

E₃ Box 3 is chosen.

P(E₁)=P(E₂)=P(E₃)=1/3 , p(A/E₁)=3/5

Then

P(A/E₂)=1/5, P(A/E₃)=7/10

Required probability =P(E₃/A)

P(E₃)P(A/E₃)/P(E₁)P(A/E₁)+P(E₂)P(A/E₂)+P(E₃)P(A/E₃)=7/15

Example: In an entrance test there are multiple choice questions. There are four probable correct answers to each question of which one is right. The possible chance that a pupil perceives the right answer to a particular question is 90%. If he gets the right answer to a particular question, then what is the probable chance that he was predicting.

Solution: We define the following events :

A₁ : He knows the answer.

A₂ : He might not know the answer.

E: He is aware of the right answer.

P(A₁) =9/10, P(A₂) =1-9/10=1/10, P(E/A₁)=1,

P(E/A₂) = 1/4

So the expected probability

Example: Bucket A contains 4 Yellow and 3 Black Marbles and Bucket B contains 4 Black and 3 Yellow Marbles. One Bucket is taken at random and a Marble is drawn and noted it is Yellow. What is the probability that it comes Bucket B.

Solution: It is based on Baye’s theorem. 

Probability of picked Bucket A , P(A)=1/2

Probability of picked Bucket B , P(B)=1/2

Probability of Yellow Marble picked from Bucket A  =P(A). P(G/A)=(1/2)x (4/7)=2/7 

Probability of Yellow Marble picked from Bucket B = P(B).P(G/B)=(1/2)x(3/7)=3/14

Total probability of Yellow Marbles= (2/7)+(3/14)=1/2

Probability of fact that Yellow Marbles is drawn from Bucket B  

P(G/B)={P(B).P(G/B)}/{P(A).P(G/A)+P(B).P(G/B)}={(1/2)x(3/7)}/{[(1/2)x(4/7)]+[(1/2)+(3/7)]} =3/7

Conclusion:

 In this article we mainly discuss on the Conditional Probability and Bayes theorem with the examples of these the direct and dependent consequence of the trial we discuss so far now in the consecutive articles we relate probability to random variable and some familiar terms related to probability theory we will discuss, if you want further reading then go through:

Schaum’s Outlines of Probability and Statistics and Wikipedia page.

For further study, please refer our mathematics page.

  After discussing the definitions and basic concepts we will enlist all the results and relations of permutation and combination, depending on all those we will get more familiar with the concept of permutation and combination by solving miscellaneous examples.

Points to remember (Permutation)

1. The number of ways of ordering = ⁿP_r={n(n-1)(n-2)…..(n-r+1)((n-r)!)}/(n-r)!= n!/{(n-r)!}
2. The number of arranging of n different objects taken all together at a time is = ⁿP_n =n!
3. ⁿP₀ =n!/n!=1
4. P=n. ^n-1P_r-1
5. 0!=1
6. 1/(-r)!=0 , (-r)!=∞ (r∈ N)
7. The number of ways of filling r places where every place can be filled by any one of n objects, The count of permutations = The number of ways of stuffing r places =(n)^r   

Example: How many numbers between 999 and 10000 can be generated with the help of numbers 0, 2, 3,6,7,8 where the digits must not be duplicated?

Solution: The numbers in between 999 and 10000 all are of four digit numbers.

                   The four-digit numbers constructed by digits 0, 2, 3,6,7,8 are

  But here those numbers are also involved which begin from 0. So we can take the numbers which are formed with three digits.

Taking initial digit 0, the number of ways to arrange pending 3 places from five digits 2, 3,6,7,8 are ⁵P₃ =5!/(5-3)!=2!*3*4*5/2!= 60

So the required numbers = 360-60 = 300.

Example: How many books can be set out in a row so that the two books mentioned are not together?

Solution: Total number of orders of n different books =n!.                                                                                                                

           If two mentioned books always together then number of ways =(n-1)!x2

Example: How many ways are there divided by 10 balls between two boys, one getting two and the other eight.

Solution: A gets 2, B  gets 8;  10!/2!8!=45

                  A gets 8, B gets 2; 10!/(8!2!)=45

that means 45+45=90 ways the ball will be divided.

Example: Search the number of arranging of the alphabets of the word “CALCUTTA”.

Solution: Required number of ways =8!/(2!2!2!)=5040

Example: Twenty people have been invited to the party. How many different ways in which they and the host can sit at a round table, if the two people have to sit on either side of the keeper.

Solution: There will be total 20 + 1 = 21 persons in all.

The two specified persons and the host be considered as one unit so that these remain 21 –  3 + 1 = 19 persons to be arranged in 18 ! ways.

 But the two particular person on either side of the host can themselves be arranged in 2! ways.

  Hence there are 2 ! *18 ! ways.

Example : In how many ways a garland can be made from exactly 10 flowers.

Solution:  n flowers’ garland can be made in (n-1)! ways.

Using 10 flowers garland can be prepared in 9!/2 different ways.

Example: Find the specific four-digit number which should be formed by 0, 1, 2, 3, 4, 5, 6, 7 so that each and every number has the number 1.

Solution: After securing 1 at first position out of 4 places 3 places can be filled by⁷P₃ =7!/(7-3)!=5*6*7=210 ways.

But some numbers whose fourth digit is zero, so such type of ways =⁶P₂=6!/(6-2)!=20.

                   Total ways = ⁷P₃ – ⁶P₂ =210-20=180

Keep these Points in mind for Combination

In continuation we will solve some examples  

Example: If ¹⁵C_r=¹⁵C_r+5 , then what is the value of r?

Solution: Here we will use the above

 ⁿC_r=ⁿC_n-r on the left side of the equation

¹⁵C_r=¹⁵C_r+5 => ¹⁵C_15-r =¹⁵C_r+5

=> 15-r=r+5 => 2r=10 => r=10/2=5

so the value of r is 5 implies the problem of 15 CHOOSE 5.

Example: If ²ⁿC₃ : ⁿC₂ =44:3   find the value of r, So that the value of ⁿC_r  will be 15.

 Solution: Here the given term is the ratio of 2n choose 3 and n choose 2 as

by the definition of combination

(2n)!/{(2n-3)!x3!} X {2!x(n-2)!}/n!=44/3

=> (2n)(2n-1)(2n-2)/{3n(n-1)}=44/3

=> 4(2n-1)=44 =>2n=12 => n=6

                   Now ⁶C_r=15 => ⁶C_r=⁶C₂   or ⁶C₄ => r=2, 4

so the problem is turned out to be 6 choose 2 or 6 choose 4

Example:  If  ⁿC_r-1= 36 ⁿC_r=84 and ⁿC_r+1=126 , then what would be the value of r ?

 Solution : Here ⁿC_r-1 / ⁿC_r =36/84 and ⁿC_r /ⁿC_r+1 =84/126 .

(n)!/{(n-r+1)!x(r-1)!} X {(r)!x(n-r)!}/(n)!=36/84

r/(n-r+1)=3/7 => 7r=3n-3r+3

=> 3n-10r=-3, and similarly from second ration we get

4n-10r=6

On solving, we get n=9, r=3

so the problem turned out to be 9 choose 3 , 9 choose 2 and 9 choose 4.

Example: Everyone in the room shakes hands with everyone. The total count of handshaking are 66. Find the number of person in the room.

ⁿC₂ =66 => n!/{2!(n-2)!}=66 => n(n-1)=132 => n=12

Solution: so the value of n is 12 implies the total number of people in the room is 12 and the problem is 12 choose 2.

Example: In a football tournament, 153 games were played. All teams played one game. find the number of groups involved in tournament.

Solution:

here ⁿC₂ =153 => n!/{2!(n-2)} = 153 => n(n-1)/2=153 => n=18

so the total number of teams participated in the tournament were 18 and the combination is 18 choose 2 .

Example During the Deepawali ceremony each club member sends greeting cards to others. If there are 20 members in the club, what would be the total number of ways greeting cards exchanged by the members.

Solution: Since two members can exchange cards each other in two ways so there is 20 choose 2 two times

2 x ²⁰C₂ =2 x (20!)/{2!(20-2)!}=2*190=380, there would be 380 ways to exchange greeting cards.

Example: Six plus ‘+’ and four minus ‘-’ symbols should be arranged in such a straight line so that no two ‘-’ symbols meet, find  the total number of ways .

 Solution: The ordering can be make as -+-+-+-+-+-+- the (-) signs can be put in 7 vacant (pointed) place.

Hence required number of ways = ⁷C₄ =35 .

Example: If ⁿC₂₁ =ⁿC₆ , then  find ⁿC₁₅ =?

Solution: Given ⁿC₂₁ =ⁿC₆

21+6=n => n=27

Hence ²⁷C₁₅ =27!/{15!(27-15)!} =17383860

which is the 27 choose 15.

Conclusion

Some examples are taken depending on the relations and results, as number of examples we can take on each of the result but the important thing here I want to show was how we can use any result depending on the situation if you require further reading you can go through the content or if any personal help then you can free to contact us some of the related content you can find from:

For more topics on Mathematics, please check this link.

SCHAUM’S OUTLINE OF Theory and Problems of DISCRETE MATHEMATICS

https://en.wikipedia.org/wiki/Permutation

https://en.wikipedia.org/wiki/Combination

Discrete Random Variable and Mathematical Expectation

Usually we are not interested in all the possible outcome of any random or non-random experiment instead we are interested in some probability or numerical value for the favorable events, for example suppose we are throwing two dice for the sum as 8 then we are not interested in the outcome as first dice having 2 second dice as 6 or (3,5), (5,3), (4,4), (6,2),etc. likewise for the random of experiment of reservoir in daily life we are not interested in the daily increase or decrease of water level but only interested in the rainy season water level after completion.

So such numerical quantities in which we are interested are considered as random variable of the respective random experiment.  For this purpose we assign the possible real values to the outcomes of the random experiment numerically. For illustration of assigning numerical value to the outcome consider the experiment of tossing a coin, we assign numerical value 0 and 1 for head and trail respectively in sample space of the random experiment. 

Discrete Random Variable

Discrete random variable can be define as the random variable which are finite or countably infinite in number and those who are not finite or countably infinite are Non-discrete random variables.  For every element of sample space we are assigning a real number, this can be interpreted in terms of real valued function denoted by X i.e. X:S→R. We call this function as a random variable or stochastic function, which has some physical, geometric or any other importance.

Example: Consider an experiment of throwing two dice then suppose random variable or stochastic function represent sum of the points appeared on the dice then the possible values for the sample space

S={(1,1), (1, 2), (1,3), (1,4), (1,5) , (1,6) ,

          (2,1), (2,2), (2,3), (2,4), (2,5), (2,6),

          (3,1), (3,2), (3,3), (3,4), (3,5), (3,6),

        (4,1), (4,2), (4,3), (4,4), (4,5), (4,6),

        (5,1), (5,2), (5,3), (5,4), (5,5), (5,6),

        (6,1), (6,2), (6,3), (6,4), (6,5), (6,6)}

will be X=2, for (1,1)

X=3 for (1,2), (2,1) etc from the following we can understand easily

X=2	(1,1)	(1,2)	(1,3)	(1,4)	(1,5)	(1,6)
X=3	(2,1)	(2,2)	(2,3)	(2,4)	(2,5)	(2,6)
X=4	(3,1)	(3,2)	(3,3)	(3,4)	(3,5)	(3,6)
	(4,1)	(4,2)	(4,3)	(4,4)	(4,5)	(4,6)
X=5	(5,1)	(5,2)	(5,3)	(5,4)	(5,5)	(5,6)
X=6	(6,1)	(6,2)	(6,3)	(6,4)	(6,5)	(6,6)
X=7	X=8	X=9	X=10	X=11		X=12

In the above table the diagonal elements from right to left will give the sum expressed by the random variable or stochastic function.

The probability for the respective random variable can be expressed as follows

Discrete Probability Distribution

Discrete probability distribution is the probabilities of the random variables which are discrete in nature, in particular if x₁, x₂, x₃, x₄, ………., x_k are the values of discrete random variable X then P(x₁), P(x₂), P(x₃), P(x₄), ……… .P(x_k) are the corresponding probabilities.

Probability function/probability distribution we can denote as 

P(X=x)=f(x)

and following the definition of the probability this function satisfies following conditions.

1. f(x)≥0
2. Σ f(x)=1, where this summation is total summation for x.

Example: If a coin tossed two times, then if we express the number of trails come up as random variable X, then it would be 

Outcomes	TT	TH	HT	HH
X	2	1	1	0

If we take the fair coin then the above will be the outcome for tossing twice and the probability for such random variable will be

P (X=0) = P (H,H) =1/4

P (X=1) = P (TH or HT) = P (TH ∪ HT) = P ( TH) + P ( HT)=1/4+1/4=1/2

and P ( X=2) = P (TT) =1/4

This probability distribution we can tabulate as follows

X	0	1	2
P(X=x)=f(x)	¼	½	1/4

Cumulative Distribution Function (c.d.f)/Distribution Function

We will define Distribution function or Cumulative Distribution Function (c.d.f) for the discrete random variable X denoted by F(x), for-∞≤x≤∞ as

F(x)=P(X≤x)

Provided it follows

1. For any x,y , x≤y, F(x) ≤ F(y) i.e. cumulative distribution function F(x) is non-decreasing.
2. F(x) =0 and F(x)=1
3. F(x+h)=F(x),   ∀ x i.e. . cumulative distribution function F(x) is right continuous.

Since for the discrete random variable probability for X=x is P(X=x), for x₁<X<x₂ will be P(x₁<X<x₂) and for X≤x is P(X≤x).

We can write the Distribution function for discrete distribution function as follows

we can obtain the probability function from the distribution function as

P (X=x) = f(x) =F(x)-F(u)

Example: The probability for the discrete random variable is given as follows

X	0	1	2	3	4	5	6	7
P(x)	0	1/10	1/5	1/5	3/10	1/100	1/50	17/100

Find F2, F5, F(7)?

Solution:

Mathematical Expectation 

   Mathematical expectation is very important concept for the probability theory as well as statistics point of view it is also known as the expectation or expected value, it can be defined as the summation of random variables and its probabilities in multiplication i.e if x₁, x₂, x₃, x₄, ……….x_n are the values of discrete random variable X then P(x₁),P(x₂),P(x₃),P(x₄),……….P(xn) are the corresponding probabilities then mathematical expectation of random variable X denoted by E(x)  as

Example: From a pack of 72 cards numbered from 1 to 72 at a time 8 cards are drawn, find the expected value of the sum of numbers on tickets drawn.

Solution:.  consider the random variables x₁, x₂, x₃, x₄,……….x_n representing the cards numbered 1, 2, 3, 4, ………, 72

so probability of any x out of 72 card is 

P(x_i)=1/n=1/72

since then expectation will be

E(x)=x₁.(1/n)+x₂.(1/n)+x₃.(1/n)+……………+x_n.(1/n)

E(x)=1.(1/n)+2.(1/n)+3.(1/n)+……………+72.(1/n)

={1+2+3+……………..+72}*(1/72)=72*(72+1)/2*(1/72)=73/2

Now the expected value for 8 such cards will be 

E(x)=x₁.(1/n)+x₂.(1/n)+x₃.(1/n)+……………+x₈.(1/n)

E(x)=1.(1/n)+2.(1/n)+3.(1/n)+……………+8.(1/n)

={1+2+3+……………..+8}*(1/72)

=8*(8+1)/2*(1/72)=12

Variance, Standard deviation and Mean deviation by Mathematical Expectation

The important concepts of the statistics standard deviation and variance we can express in terms of mathematical expectation, so if the random variables x₁, x₂, x₃, x₄, ……….x_n with the corresponding probabilities  P(x₁), P(x₂), P(x₃), P(x₄), ……….P(x_n)  then variance will be

Example: In a game if a fair dice is used and player will win if any odd value come on dice  and prize money will be given Rs 20 if 1 come, Rs 40  for 3, and Rs 60 for  5 and if any other face of the dice came Rs 10 loss for the player. find the expected money that can be won with variance and standard deviation.

Solution:

For the fair dice we know the distribution of the probabilities,

X	1	2	3	4	5	6
P(X=x)	1/6	1/6	1/6	1/6	1/6	1/6

Let X be the random variable for the dice convert as per the game requirement money won or loss when face came as follows,

X	+20	-10	40	-10	60	-10
P(X=x)	1/6	1/6	1/6	1/6	1/6	1/6

so the expected amount won by any player will be

  E(x)=(20).(1/6)+(-10).(1/6)+(40).(1/6)+(-10).(1/6)+(60).(1/6)+(-10).(1/6)=15

so the expected amount won by any player would be μ=15

The result of the mathematical expectation as well as variance can be generalized for more than two variables as per requirement.

Conclusion:

   In this article we mainly discussed the discrete random variable, probability distribution and distribution function known as cdf cumulative distribution function, Also the concept of Mathematical Expectation for discrete random variable and what would be the mean deviation, variance and standard deviation for such discrete random  variable is explained with the help of suitable examples in next article we will discuss the same for continuous random variable, if you want further reading then go through:

For more topic on Mathematics, please follow this link.

Schaum’s Outlines of Probability and Statistics

https://en.wikipedia.org/wiki/Probability

Properties of Permutation and combination

  When discussing permutation and combination as we are dealing with selection and arrangement with or without order considerations, depending on the situation there are different types and properties for the permutation and combination, these differences among permutations and combinations we will explain here with justified examples.

permutations without repetition

  This is the normal permutation which arranges n objects taken r at a time i.e nPr

ⁿ P_r=n!/(n-r)!

number of orderings of n different objects taken all at a time ⁿ P_n =n!

In addition, we have

ⁿP₀ = n!/n!=1

ⁿP_r = n.^n-1P_r-1

0!=1

1/(-r)!= 0 or (-r)!=∞

permutations with repetition

 Number of permutations (arrangements) for different items, taken r at a time, where each item can happen once, twice, three times, …….. r-times as many in any arrangement = Number of ways to fill r areas where each item can be filled with any of the n items.

The number of permutations = The number of ways of filling r places = (n)^r

The number of orders that can be organized using n objects out of which p are alike (and of one kind) q are alike (and of another kind), r are similar (and of another kind) and the rest are distinct is ⁿP_r =n!/(p!q!r!)

Example:

In how many ways can 5 apples be allocated among four boys when every boy can take one or more apples.      

Solution: This is the example of permutation with repetition as we know that for such cases we have

The number of permutations = The number of ways of filling r places =n^r

Required number of ways are 4⁵ =10, Since each apple can be distributed in 4 ways.

Example: Find the number of words can be organized with the letters of the word MATHEMATICS by regrouping them.

Solution: Here we can observe that there are 2 M’s, 2 A’s and 2T’s this is the example of permutation with repetition

=n!/(p!q!r!)

 Required number of ways are =11!/(2!2!2!)=4989600

Example: How many ways in which the number of tails equal to the number of heads if six identical coins are arranged in a row.

Solution: Here we can observe that

No. of heads=3

No. of tails =3

And since coins are identical this is the example of permutation with repetition =n!/(p!q!r!)

Required number of ways =6!/(3!3!)= 720/(6X6)=20

Circular permutation:

In circular permutation, most importantly the ordering of the object is respect to the others.

So, in circular permutation, we adjust the position of one object and arrange the other objects in all directions.

Circular permutation is split into two ways:

(i) Circular permutation where clockwise and anti-clockwise settings suggest different permutation, e.g. Arrangements for seating people around the table.

(ii) Circular permutation where clockwise and anti-clockwise settings display same permutation, e.g. arranging certain beads to create a necklace.

Clockwise and anti-clockwise arrangement

If the anti-clockwise and clockwise order and movement are not different eg, bead arrangement in necklace, flower arrangement in garland etc, then the number of circular permutations of n distinct items is (n-1)!/2

1. The number of circular permutation for n different items, taken r at a time, when the orders for the clockwise and the anti-clockwise  are considered to be different by ⁿP_r /r
2. The number of circular permutation for n different items, taken r at a time, when clockwise and anti-clockwise orders are not different from ⁿP_r /2r
3. The number of circular permutations of n different objects are (n-1)!
4. The number of ways in which n different boys can be seated round a circular table is (n-1)!
5. The number of ways in which n different gems can be set up to form a necklet, is (n-1)!/2

Example:

How many ways can five keys be placed in the ring

Solution:

Since clockwise and anticlockwise are same in case of ring.

If the anti-clockwise and clockwise sequence and movement are not different then the number of circular permutations of n distinct items is

=(n-1)!/2

Required number of ways = (5-1)!/2= 4!/2=12     

Example:

What would be the number of arrangements, If eleven members of a committee sit at a round table so that the President and Secretary always sit together.

Solution:

By fundamental property of circular permutation

The count of circular permutations of n different things are (n-1)!

Since two positions are fix so we have

Required number of ways (11-2)!*2=9!*2=725760

Example: What would be number of ways 6 men and 5 women can eat at a round table if no two women can sit together

Solution: By fundamental property of circular permutation.

The count of circular permutations of n different things are (n-1)!

Number of ways in which 6 men can be arranged at a round table = (6 – 1)!  =5!

Now women can be arranged in 6! ways and Total Number of ways = 6! × 5!

Combinations without repetition

This is the usual Combination which is “The number of combinations (selections or groups) that can be formed from n different objects taken r at at a time is ⁿC_r =n!/(n-r)!r!

Also    ⁿC_r =ⁿC_r-r

              ⁿ P_r /r! =n!/(n-r)! =ⁿC_r

Example: Find the number of options to fill 12 vacancies if there are 25 candidates and five of them are from the scheduled category, provided that 3 vacancies are reserved for the S.C candidates mean while the remaining are open to everyone.

Solution: Since 3 vacant positions are filled from 5 applicants in ⁵ C₃  ways (i.e 5 CHOOSE 3) and now remaining candidates are 22 and remaining seats are 9 so it would be ²²C₉ (22 CHOOSE 9) The selection can be made in ⁵ C₃  X ²²C₉ ={5!/3!(5-3)! }X{22!/9!(22-9)!}

⁵ C₃  X ²²C₉ = {(3!X4X5)/(3!X2!)}X {22!/(9!X13!)}=4974200

So the selection can be made in 4974200 ways. 

Example: There are 10 candidates and three vacancies in the election. in how many ways a voter can cast his or her vote?

Solution: Since there are only 3 vacancies for 10 candidates so this is the problem of 10 CHOOSE 1, 10 CHOOSE 2, and 10 CHOOSE 3 Examples,

A voter can vote in ¹⁰C₁+¹⁰C₂+¹⁰C₃ = {10!/1!(10-1)!}+{10!/2!(10-2)!}+{10!/3!(10-3)!} =10+45+120= 175 ways.

 So in 175 ways voter can vote.

Example:There are 9 chairs in a room for 4 people, one of which is a single-seat guest with one specific chair. How many ways can they sit?

Solution: Since 3 chairs can be select in ⁸C₃ and then 3 persons can be arranged in 3! ways.

3 persons are to be seated on 8 chairs ⁸C₃ (i.e 8 CHOOSE 3) arrangement

=⁸C₃ X3! = {8! /3!(8-3)!} X3!

=56X6=336

In 336 ways they can sit.

Example: For five men and 4 women, a group of 6 will be formed. In how many ways this can be done so that the group has more men.

Solution: Here the problem include different combinations like 5 CHOOSE 5, 5 CHOOSE 4, 5 CHOOSE 3 for men and for women it include 4 CHOOSE 1, 4 CHOOSE 2 and 4 CHOOSE 3 as given in the followings

1 woman and 5 men =⁴C₁ X ⁵C₅ ={4!/1!(4-1)!} X{5!/5!(5-5)!}=4

           2 women and 4 men =⁴C₂ X ⁵C₄ = {4!/2!(4-2)!} X{5!/4!(5-4)!}=30

           3 women and 3 men =⁴C₃ X ⁵C₃ = {4!/3!(4-3)!} X {5!/3!(5-3)!} =40

    Hence total ways = 4+30+40=74.

Example: The number of ways 12 boys can travel in three cars so that 4 boys in each car, assuming that three particular boys will not go in the same car.

Solution: First omit three particular boys, remaining 9 boys may be 3 in each car. This can be done in 9 CHOOSE 3 i.e ⁹C₃ ways,

The three particular boys may be placed in three ways one in each car. Therefore total number of ways are =3X⁹C₃.

={9!/3!(9-3)!}X3= 252

so in 252 ways they can be placed.

Example: How many ways did 2 green and 2 black balls come out of a bag containing 7 green and 8 black balls?

Solution: Here bag contains 7 green from that we have to choose 2 so it is 7 CHOOSE 2 problem and 8 black balls from that we have to choose 2 so it is 8 CHOOSE 2 problem.

Hence the Required number = ⁷C₂ X ⁸C₂ = {7!/2!(7-2)!}X{8!/2!(8-2)!}=21X28=588

so in 588 ways we can select 2 green and 2 black from that bag.

Example: Twelve different characters of English words are provided. From these letters, 2 alphabetical names are formed. How many words can be created when at least one letter is repeated.

Solution: here we have to choose 2 letter words from 12 letters so it is 12 CHOOSE 2 problem.

No. of words of 2 letters in which letters have been recurrent any times = 12²

        But no. of words on having two different letters out of 12 =¹²C₂ = {12!/2!(12-2)!} =66

        Required number of words = 12²-66=144-66=78.

Example: There are 12 points on the plane where six are collinear, then how many lines can be drawn by joining these points.

Solution: For 12 points in a plane to make line we require 2 points same for six collinear points, so this is 12 CHOOSE 2 and 6 CHOOSE 2 problem.

The number of lines is = ¹²C₂ – ⁶C₂ +1={12!/2!(12-2)!}-{6!/2!(6-2)!}+1 =66-15+1=52

So in 52 number of ways it lines can be drawn.

Example: Find the numeral of ways in which a 6-member cabinet can be set up from 8 gentlemen and 4 ladies so that the cabinet consists of at least 3 ladies.

Solution: For forming the committee, we can choose from 3 each men and women and 2men 4 women so the problem includes 8 CHOOSE 3, 4 CHOOSE 3, 8 CHOOSE 2 and 4 CHOOSE 4.

Two types of cabinet can be formed

        (i) Having 3 men and 3 ladies

        (ii) Having 2 men and 4 ladies

        Possible no. of ways =  (⁸C₃ X ⁴C₃) + (⁸C₂ X⁴C₄)= {8!/3!(8-3)!}X{4!/3!(4-3)!} +{8!/2!(8-2)!}X{4!/4!(4-4)!} = 56X4+ 28X1 =252      

So In 252 ways we can form such cabinet.

       These are some examples where we can compare the situation of ⁿP_r vs ⁿC_r in the case of permutation, the way things are organized is important. However in Combination the order means nothing.

Conclusion

A brief description of Permutation and combination when repeated and non-repeated with the basic formula and important results is provided in the form of real examples, in this series of articles we will discuss in detail the various outcomes and formulas with relevant examples, if you want to continue reading:

SCHAUM’S OUTLINE OF Theory and Problems of DISCRETE MATHEMATICS

https://en.wikipedia.org/wiki/Permutation

https://en.wikipedia.org/wiki/Combination

For more Article on Mathematics, please follow this Link