Concept of mutual inductance | Mutual inductance definition
In two adjacent conductor coils, the variation in current in one coil will cause induced emf in the other coil, This phenomenon is called mutual induction. Mutual induction is not a single coil’s property as both/multiple the inductor/inductors are impacted by this property at same time. The primary coil is the coil in which variation of current takes place, and the 2nd coil in which emf is induced named secondary.
Unit of mutual inductance | SI unit of mutual inductance
The unit of mutual inductance is same as inductance, i.e. So SI unit of mutual inductance is Henry(H).
Dimension of mutual inductance
Dimension of mutual inductance = dimension of magnetic flux/dimension of current = [MLT-2I-2]
Mutual inductance equation
Mutual induction is the principle that current running through a conductor will generate a magnetic field, and a changing magnetic field will induce a current in another conductor.
From Faraday’s law and Lenz’s law, we can write,
E = -(dφ/dt)
E ∝ dφ/dt
We already know, ? ∝ i [ as B=μ0ni and ?=nBA]
Therefore, E ∝ di/dt; E =-Mdi/dt [M is proportionality constant]
This M is called the mutual inductance.
M = -E/(di/dt)= emf induced in the secondary coil/rate of change of current in the primary coil
We can also write by comparing that,
-Mdi/dt = dφ/dt
Integrating both sides, we get, ? = Mi
Define Mutual Inductance of 1 Henry
This is the measurement in one coil having 1 m2 area, produced 1 V by the variation of the inducing current of 1 Amp/sec in other coil in the existence of 1 T magnetic field.
Derive an expression for mutual inductance
Mutual inductance circuit analysis | Mutual inductance equivalent circuit
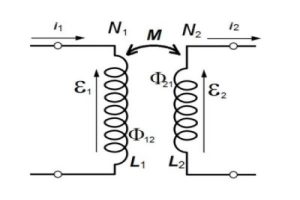
Let us consider, two inductor coils with self-inductance, L1 and L2, are kept in close contact with each other. Current i1 flows through the first one, and i2 flows through the second one. When i1 changes with time, the magnetic field also varies and leads to a change in magnetic flux linked to the 2nd coil, the EMF is induced in the 2nd coil due to the change in current in the 1st coil and can be expressed as,
E21 = -N2(dφ21/dt)
Therefore, N2φ21 ∝ i1
Or, N2φ21 = M21i1
Or, M21= N2φ21/i1
This proportionality constant M21 is called the mutual inductance
Similarly we can write, N1φ12 = M12}i2 or M12 = N1φ12 /i2
M12 is called another mutual inductance
Mutual inductance of a coil
Define mutual inductance between a pair of coils
The mutual inductance of a pair of coils is the ratio of magnetic flux linked with one coil and current passing through another coil.
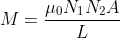
Where, μ0=permeability of free space
N1, N2 are turns of the coil.
A is the cross-sectional area of the coil.
L is the length of the coil.
Mutual inductance formula | Mutual inductance of two solenoids
Mutual inductance between two coils,
M = μ0N1N2A/L if there’s no core in between two coils
M = μ0\\μrN1N2A/L if the soft iron core is placed between the coils
How to find the mutual inductance of two long co-axial solenoid ?
Derivation of mutual inductance of two long coaxial solenoids
Let us assume that two solenoids S1 and S2, are placed in close contact with each other. Due to the phenomenon of mutual induction, the current passing through 1st coil will induce EMF in the another coil. Now, we connect S1 with a battery through a switch and S2 with a galvanometer. The galvanometer detects the presence of current and its direction.
Due to the flow of current in S1, magnetic flux is generated in S2, and a change in magnetic flux causes the current in S2. Due to this current, the galvanometer needle shows deflection. Therefore we can say current i of S1 is proportional to ? in S2.
? ∝ i
? = Mi
Here M is called mutual inductance.
Now, in the case of coaxial solenoids, one coil is placed inside another so that they share the same axis. Suppose S1 and S2 have turns N1, N2, and areas A1, A2 respectively.
Mutual inductance formula derivation
For inner coil S1:
When current i1 flows through S1, magnetic field, B1 =μ0N1i1
Magnetic flux linked with S2, φ21 = B1A1 = μ0N1i1A1
This is the flux for a single turn. [Though the area of S2 is A2, the flux will only generate in the area A1]
Therefore for N2 turns φ21 = μ0N1i1A1 x N2/L …..(1), where L is the length of the solenoids
We know,
? = Mi
?21 = M21i1…….(2)
Equating (1) and (2), we get,
M21i1 = μ0N1i1A1N2/L
M21 = μ0N1A1N2/L
For outer coil S2:
When current i2 flows through S2, magnetic field, B2 = μ0N1i2
Magnetic flux linked with S1 for N1 turns, φ12 = N1/L x B2A1 = μ0N1N2i2A1/L ….(3)
Similar to the inner coil we can write,
?12 = M12i2……(4)
Equating (1) and (2), we get,
M12i2= μ0N1N2i2A1/L
M12 = μ0N1N2A1/L
From the above two findings, we can say that M12=M21 = M. This is the mutual inductance of the system.
Mutual inductance of a coil inside a solenoid | Mutual inductance between two loops
A coil with N2 bindings is placed inside a long thin solenoid that contains N1 number of bindings. Let us assume that the bindings of the coil and the solenoid are A2 and A1, respectively, and the length of the solenoid is L.
It is known that the magnetic field inside a solenoid due to current i1 is,
B = μ0N1i1/L
Magnetic flux that passes through the coil due to the solenoid,
?21 = BA2cos? [? is the angle between the magnetic field vector B and area vector A2]
φ21 = μ0N1i1/L x A2 cosθ
Mutual inductance, M = φ21N2/i1= μ0N1N2 A2 cosθ/L
Mutual inductance in parallel
In this circuit 2-inductors having self-inductance L1 and L2, are adjoined in parallel, Let us assume the total current is i, the sum of i1( current through L1) and i2(current through L2) Mutual inductance between considered as M.
i= i1 + i2
di/dt = di1/dt+ di2/dt
Effective flux through L1, ?1 = L1i1 + Mi2
Effective flux through L2, ?2 = L2i2 + Mi1
Induced EMF in L1,
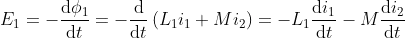
Induced EMF in L2,
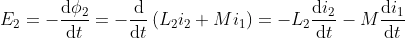
We know in case of parallel connection, E1 = E2
-L1(di1/dt) – Mdi2/dt = E … (1)
-L1(di2/dt) – Mdi1/dt = E … (2)
Solving the two equations, we get,
di1/dt = E(M-L2)/L1L2 – M2
di2/dt = E(M-L)/L1L2 – M2

We know, E = -Leff (di/dt)
Or, Leff =-E/(di/dt) = L1L2 – M2/L1-L2-2M
To know more about the Inductors in series and parallel click here
Calculating mutual inductance between circular coils | Mutual inductance of two circular loops
Let us take two circular coils of radii r1 and r2 sharing the same axis. The number of turns in the coils are N1 and N2.
The total magnetic field in the primary coil due to current i,
B = μ0N1i2r1
Magnetic flux produced in the secondary coil because of B,
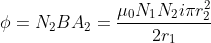
We know mutual inductance,
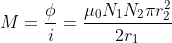
Factors affecting mutual inductance | Mutual inductance M is dependent on what factors
- Material of the core- Air core or Solid core
- No of Turn (N) of the coils
- Length (L) of the coil.
- Cross-sectional area(A).
- Distance(d) between the coils.
- Alignment/Orientation of the coil.
Mutual inductance coupling | Coupling coefficient k
The fraction of the magnetic flux generated in one coil that is linked with another coil is known as the coefficient of coupling. It is denoted by k.
Coefficient of mutual inductance,
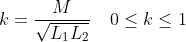
- If coils are not coupled, k = 0
- If coils are loosely coupled, k<½ If coils are tightly coupled, k>½
- If coils are perfectly coupled, k = 1
The formula for self-inductance and mutual inductance
Self-inductance L = N?/i = number of turns in the coil x magnetic flux linked with the coil/current flowing through the coil
Mutual inductance M = ?/i = magnetic flux linked with one coil/current passing through another coil
Mutual inductance between two parallel wires
Let us think that two parallel cylindrical wires carrying equal current, each of l length and radius a. Their centers are d distance apart.
The mutual inductance between them is determined with the help of Neumann’s formula.
M = 2l[ln(2d/a) -1 + d/l] (Approximately)
Where, l>>d
What is the difference between self and mutual inductance ?
| Self-inductance | Mutual inductance |
| Self-inductance is the property of an individual coil. | Mutual inductance is shared by both the coils |
| It is the ratio of the total magnetic flux produced in the coil and the current. | It is the ratio of the total magnetic flux produced in one coil and the current passing through another coil. |
| If the own current increases, the induced current opposes that. | If the own current of one coil increases, the induced current in the other coil opposes that. |
What are the application of self induction and mutual induction ?
Applications of self-inductance
The principle of self-induction is used in the following devices-
- Choke coils.
- Sensors.
- Relays
- DC to AC converter.
- Ac filter.
- Oscillator circuit.
Applications of mutual inductance
The principle of mutual induction is used in the following devices-
- Transformers.
- Metal detector.
- Generators.
- Radio receiver.
- Pacemaker.
- Electric motors.
Mutual inductance circuits | Mutual inductance circuit example
T-circuit:
Three inductors are connected like a T-shape as shown in the figure. The circuit is analyzed with the two-port network concept.
Π-circuit:
Contrarily, two coupled inductors can be created using a π equivalent circuit with optional ideal transformers at each port. The circuit can look complicated initially, but it can further be generalized into circuits that have more than two coupled inductors.
What is the Difference between mutual induction and mutual inductance ?
Mutual induction vs Mutual inductance
Mutual inductance is the property shared by two inductive coils in which varying current in one coil induces EMF in the another one, If mutual induction is the cause, mutual inductance can be said to be its effect.
Mutual inductance dot convention
The relative polarity of the mutually coupled inductors decides whether the induced EMF is additive or subtractive. This relative polarity is expressed with dot convention. It is denoted by a dot sign at the ends of the coil. At any instance, if the current enters a coil through the dotted end, mutually induced EMF on the other coil will have a positive polarity at the dotted end of that coil.
Energy stored in mutually coupled inductors
Let us assume that two mutually coupled inductors have self-inductance values L1 and L2. Currents i1 and i2 travel in them. Initially, the current in both the coils is zero. So the energy is also zero. The value of i1 rises from 0 to I1, while i2 is zero. So the power in inductor one,
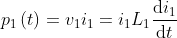
So, the energy stored,
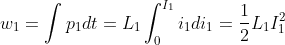
Now, if we keep i1 = I1 and increase i2 from zero to I2, the mutually induced EMF in inductor one is M12 di2/dt, while the mutually induced EMF in inductor two is zero since i1 does not change.
So, the power of inductor two due to mutual induction,
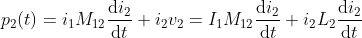
Energy stored,
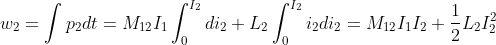
The total energy stored in the inductors when both i1 and i2 have reached constant values is,
w = w1 + w2 = 1/2L1I12 + 1/2L2I22 – MI1I2
If we reverse the current increments, that is, increase i2 from zero to I2 first and later increase i1 from zero to I1, the total energy stored in the inductors is,
w = w1 + w2 = 1/2L1I12 + 1/2L2I22 – MI1I2
Since, M12 = M21, we can conclude that the total energy of mutually coupled inductors is,
w = w1 + w2 = 1/2L1I12 + 12L2I22 + MI1I2
This formula is correct only when both the currents enter dotted terminals. If one current enters the dotted terminal and the other leave, the energy stored will be,
w = w1 + w2 = 1/2L1I12 + 1/2L2I22 – MI1I2
Mutual inductance devices
Mutual inductance transformer model
An AC voltage can be increased or reduced according to the requirements of any electrical circuit by using a static device. It is called a transformer. It is a four-terminal device that consists of two or more mutually coupled coils.
Transformers follow the principle of mutual induction. They transfer electric energy from one circuit to another when the circuits are not electrically connected.
Linear transformer:
If the coils in the transformer are wound on magnetically linear material, then it is called a linear transformer. Magnetically linear materials have constant permeability.
In a linear transformer, magnetic flux is proportional to the current passing through the windings. The coil that is directly joined to a voltage source is known as the primary coil and the coil adjoined to load impedance is entitled as secondary. If R1 is connected in the circuit with the voltage source and R2 is connected in the circuit with the load.
Applying Kirchhoff’s voltage law in two meshes, we can write,
V = (R1 + jΩL1)I1 – jΩMI2……(1)
-jΩ MI1 + (R2 + jΩL2 + ZL)I2 = 0.…..(2)
Input impedance in the primary coil,
Zin = V/I1 = R1+ jΩL1 + Ω2M2/R2+jΩL2 + ZL
The first term (R1+jωL1) is called the primary impedance and the other second term is called the reflected impedance ZR.
ZR = Ω2M2/R2+jΩ L2 + ZL
Ideal transformer
A transformer that doesn’t have any type of loss is called an ideal transformer.

Characteristics:
- An ideal transformer has zero primary and secondary winding resistance.
- Permeability of the core is considered as infinite.
- No leakage flux is there in an ideal case.
- Hysteresis does not take place.
- The value of eddy current loss is zero.
- The ideal transformer is said to be 100% efficient.
Mutual inductance of transformer formula-
There’s zero power loss in an ideal transformer. So, the input power = output power
W1i1cosφ = W2i2cosφ or W1i1 = W2i2
Therefore, i1/i2 = W2/W1
Since voltage is directly proportional to the no. of turns in the coil.,
we can write,
V2/V1 = W2/W1= N2/N1 = i1/i2
If V2>V1, then the transformer is called a step-up transformer.
If V2<V1, then the transformer is called a step-down transformer.
Applications of transformer:
- A transformer can electrically isolate two circuits
- The most important application of a transformer is to step up ( increase) or step down (decrease) the voltage. It can raise or lower the value of current and voltage so that if any of the quantities increase or decrease, power remains the same.
- It can also increase or decrease the impedance, capacitance, or inductance values in a circuit. In other words, the transformer can perform impedance matching.
- Transformer will prevent carrying direct current from one circuit to other.
- It is used in mobile chargers to avoid damages caused by high voltage.
- It is used to generate a neutral in three-phase power supply.
Heaviside Mutual Inductance Bridge | Mutual Inductance measurement bridge
We use mutual inductance in various circuits to determine the values of self-inductance, frequency, capacitance, etc. Heaviside bridge is a component where we can measure mutual inductance with the help of a known self-inductance. A modified version of this bridge can be used in performing the reverse application i.e. measuring self-inductance with the help of known mutual inductance.
Operation
Let us take a combination of elements in the form of the bridge circuit shown in the figure. The coil S1 with mutual inductance M is not the part of the bridge but it is mutually coupled with the coil S2 in the bridge which has self-inductance L1. Current passing through S1 produces flux that is linked with S2. As per the dot convention, we can say, current i passes through S1 and further gets divided into i1 and i2. The current i1 passes through S2.
Under balanced condition,
i3=i1; i4=i2 ; i=i1+i2
As no current passes through the galvanometer, the potential of B is equal to the potential of D.
Therefore we can say, E1=E2
Or, (i1+i2)jΩM + i1(R1+jΩ L1) = i2(R2+jΩ L2)
i1R1+jΩ (L1i1+ M(i1+i2))= i2R2 + jΩ L2i2 …..(1)
i1[R1+jΩ (L1+M) = i2[R2+jΩ (L2-M)] ……(2)
Similarly, E3=E4
i3R3=i4R4
Or, i1R3=i2R4…….(3)
Dividing (1) by (3) we get,
R1+jΩ (L1+M)/R3 = R2 + jΩ (L2-M)/R4
Taking the real parts of both sides, we can write,
R1/R3=R2/R4
Taking the imaginary parts of both sides, we can write,
L1+M/R3=L2-M/R4
So, M=R3L2-R4L1/R3+R4
We can conclude from the above equation that the value of L1 must be known. Now if R3=R4,
R1=R2 and M = L2-L1/2
Or, L2= L1+2M
This way we can find out the value of unknown inductance L2
The bridge that measures the unknown mutual inductance in terms of two known self-inductance L1 and L2, is called the mutual inductance measurement bridge or Campbell bridge.
The field-armature mutual inductance of the synchronous motor
In an AC rotating synchronous motor, steady-state speed is proportional to the frequency of the current passing through its armature. Therefore, a magnetic field is produced. The current rotates at the same speed as that of the rotating synchronous speed of the field current on the rotor. Due to this phenomenon, a mutual induction develops between the armature and the field wingdings. It is known as field-armature mutual inductance.

Hi……I am Kaushikee Banerjee completed my master’s in Electronics and Communications. I am an electronics enthusiast and am currently devoted to the field of Electronics and Communications. My interest lies in exploring cutting-edge technologies. I’m an enthusiastic learner and I tinker around with open-source electronics.