Physics

When an equilibrium is a static equilibrium refers to a state where the net force and net torque acting on a system are zero, and the system is not accelerating. This concept is fundamental in physics and is used to describe the behavior of structures and objects in equilibrium.

Understanding Static Equilibrium

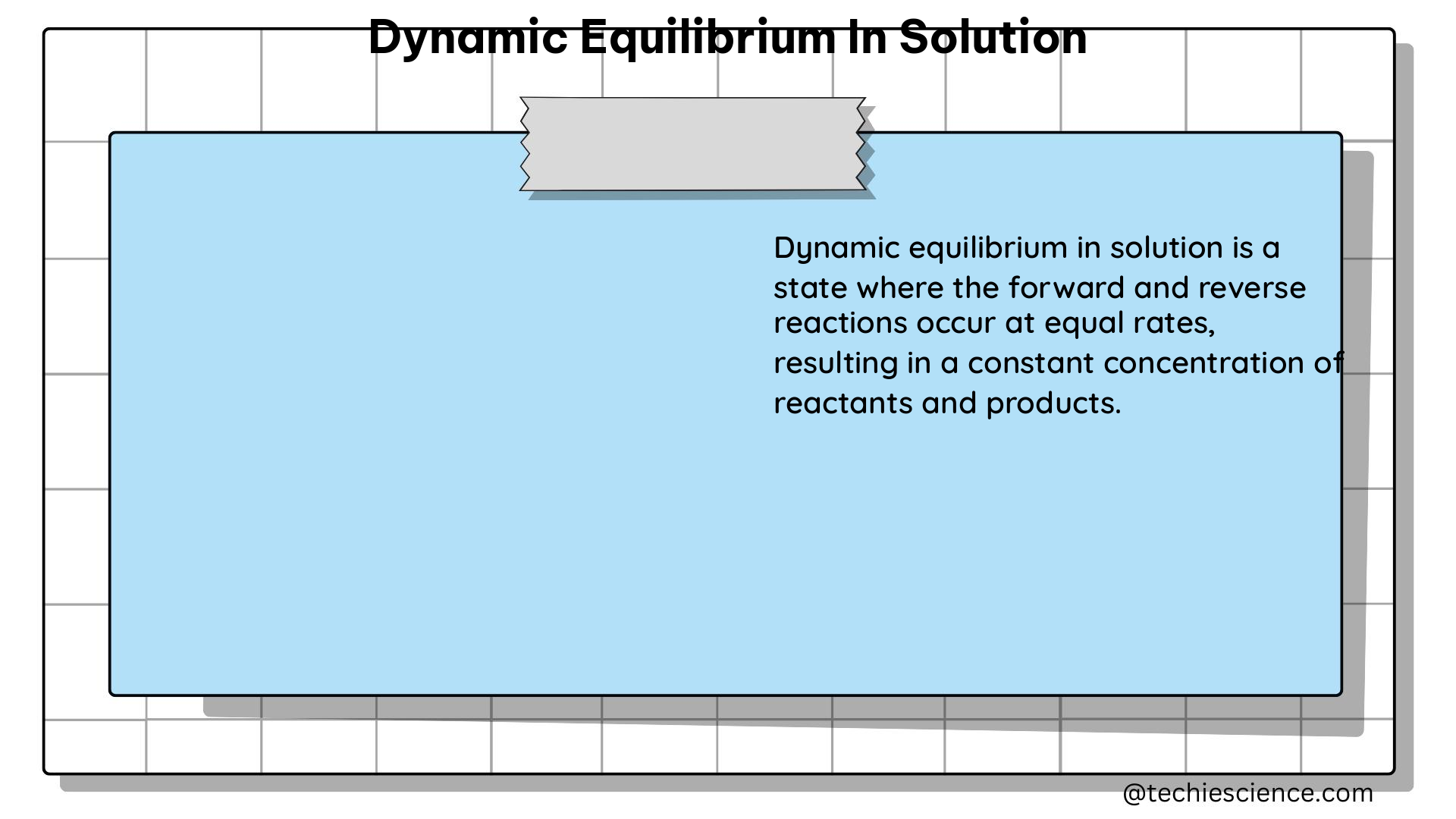
Static equilibrium is a state of a system where the net force and net torque acting on the system are zero, and the system is not accelerating. This means that the system is in a state of balance, and there is no net change in its position or orientation.

The conditions for static equilibrium can be expressed mathematically as:

1. The sum of all forces acting on the system is zero:
   ∑F = 0

2. The sum of all torques acting on the system is zero:
   ∑τ = 0

These equations indicate that the net force and net torque are zero, which means that the system is in equilibrium and not accelerating.

Conditions for Static Equilibrium

For a system to be in static equilibrium, the following conditions must be met:

1. No Net Force: The sum of all forces acting on the system must be zero. This means that the vector sum of all the forces acting on the system is zero.

2. No Net Torque: The sum of all torques acting on the system must be zero. This means that the vector sum of all the torques acting on the system is zero.

3. No Acceleration: The system must not be accelerating. This means that the system is not changing its position or orientation over time.

These conditions ensure that the system is in a state of balance and is not experiencing any net change in its state.

Examples of Static Equilibrium

1. Seesaw: Consider a simple seesaw with two children of different weights sitting at equal distances from the fulcrum. The system is in static equilibrium when the net force and net torque are zero. This can be expressed as:

F1 + F2 = 0 (Net Force)
d1F1 = d2F2 (Net Torque)

where F1 and F2 are the forces exerted by the children, and d1 and d2 are the distances from the fulcrum.

1. Bridge: In a more complex system, such as a bridge, the static equilibrium can be described by the following equations:

∑Fx = 0 (Net Force in x-direction)
∑Fy = 0 (Net Force in y-direction)
∑M = 0 (Net Torque)

where ∑Fx and ∑Fy are the sums of all forces acting in the x and y directions, respectively, and ∑M is the sum of all moments acting on the system.

1. Ladder against a Wall: Consider a ladder leaning against a wall. The ladder is in static equilibrium when the net force and net torque are zero. This can be expressed as:

∑Fx = 0 (Net Force in x-direction)
∑Fy = 0 (Net Force in y-direction)
∑M = 0 (Net Torque)

where the forces and torques acting on the ladder, such as the weight of the ladder, the normal force from the wall, and the friction force, are balanced.

1. Suspended Object: A suspended object, such as a chandelier or a weight hanging from a rope, is in static equilibrium when the net force and net torque are zero. This can be expressed as:

∑Fx = 0 (Net Force in x-direction)
∑Fy = 0 (Net Force in y-direction)
∑M = 0 (Net Torque)

where the weight of the object is balanced by the tension in the rope or the supporting structure.

Importance of Static Equilibrium

Static equilibrium is a fundamental concept in physics and engineering, and it has several important applications:

1. Structural Design: Understanding static equilibrium is crucial in the design of structures, such as buildings, bridges, and machines, to ensure their stability and safety.

2. Mechanical Systems: Static equilibrium is essential in the analysis and design of mechanical systems, such as levers, pulleys, and gears, to ensure their proper functioning and load-bearing capabilities.

3. Robotics and Automation: Static equilibrium principles are used in the design and control of robotic systems, ensuring their stability and precise movement.

4. Biomechanics: Static equilibrium concepts are applied in the study of human and animal biomechanics, helping to understand the mechanics of movement and the forces acting on the body.

5. Geophysics: Static equilibrium principles are used in the study of geological structures, such as tectonic plates and fault lines, to understand the forces and stresses acting on the Earth’s surface.

Conclusion

In summary, static equilibrium is a state where the net force and net torque acting on a system are zero, and the system is not accelerating. This concept is fundamental in physics and is used to describe the behavior of structures and objects in equilibrium. Understanding the conditions for static equilibrium and its applications is crucial in various fields, including structural design, mechanical systems, robotics, biomechanics, and geophysics.

References:

1. Introduction to Chemical Equilibrium; Qualitative View of Chemical Equilibrium, Disturbances to Equilibrium, and Le Châtelier’s Principle | Chemistry LibreTexts. (n.d.). Retrieved from https://chem.libretexts.org/Courses/University_of_California_Davis/Chem_107B%3A_Chemical_Equilibrium_and_Spectroscopy/02%3A_Introduction_to_Chemical_Equilibrium/2.01%3A_Qualitative_View_of_Chemical_Equilibrium_Disturbances_to_Equilibrium_and_Le_Chateliers_Principle
2. A Progression of Static Equilibrium Laboratory Exercises | American Journal of Physics. (2013). Retrieved from https://aapt.scitation.org/doi/10.1119/1.4823059
3. Static Equilibrium – YouTube. (2022, April 14). Retrieved from https://www.youtube.com/watch?v=nTLewJ68eaE
4. PHYS207 Lab 6 Static Equilibrium Instructional Goals – Course Hero. (n.d.). Retrieved from https://www.coursehero.com/file/22009817/6-Static-Equilibrium/
5. Static Equilibrium, Reactions, and Supports | PPT – SlideShare. (2015, February 4). Retrieved from https://www.slideshare.net/slideshow/spc209-staticequilibrium/44300747

Dynamic equilibrium in solution is a fundamental concept in chemistry that describes the state where the forward and reverse reactions occur at equal rates, resulting in no net change in the concentrations of reactants and products. This concept is crucial in understanding various chemical reactions, particularly those involving acid-base equilibria. In this comprehensive guide, we will delve into the intricacies of dynamic equilibrium in solution, providing a wealth of technical and advanced details to help you gain a deeper understanding of this important topic.

Understanding Dynamic Equilibrium

Dynamic equilibrium in a solution occurs when the rate of the forward reaction is equal to the rate of the reverse reaction. This means that the concentrations of the reactants and products remain constant over time, even though the individual molecules are continuously undergoing the forward and reverse reactions.

The key characteristics of dynamic equilibrium in solution are:

1. Constant Concentrations: The concentrations of the reactants and products remain constant at equilibrium, despite the ongoing forward and reverse reactions.
2. Equal Rates: The rate of the forward reaction is equal to the rate of the reverse reaction, resulting in no net change in the concentrations.
3. Reversible Reactions: Dynamic equilibrium can only be achieved in reversible reactions, where the forward and reverse reactions can both occur.
4. Temperature Dependence: The equilibrium constant (Keq) for a reaction is temperature-dependent, meaning that changes in temperature can shift the position of the equilibrium.

Equilibrium Constant (Keq)

The equilibrium constant (Keq) is a quantitative measure of the extent of a reaction at equilibrium. It is defined as the ratio of the concentrations of the products raised to their stoichiometric coefficients to the concentrations of the reactants raised to their stoichiometric coefficients, all at equilibrium.

The general expression for the equilibrium constant is:

Keq = [C]^c * [D]^d / ([A]^a * [B]^b)

Where:
– [A], [B], [C], and [D] are the equilibrium concentrations of the reactants and products
– a, b, c, and d are the stoichiometric coefficients of the reactants and products

The equilibrium constant is a constant for a particular reaction at a given temperature and does not depend on the initial concentrations of the reactants or products.

Factors Affecting Equilibrium Constant

The value of the equilibrium constant (Keq) can be affected by several factors:

1. Temperature: The equilibrium constant is temperature-dependent. As the temperature changes, the value of Keq will also change, following the van ‘t Hoff equation:

ln(Keq2/Keq1) = -ΔH°/R * (1/T2 – 1/T1)

Where:
– Keq1 and Keq2 are the equilibrium constants at temperatures T1 and T2, respectively
– ΔH° is the standard enthalpy change of the reaction
– R is the universal gas constant

1. Pressure: For reactions involving gases, the equilibrium constant can be affected by changes in pressure. However, for reactions in solution, the effect of pressure is generally negligible.
2. Ionic Strength: In solutions with high ionic strength, the activity coefficients of the ions can affect the equilibrium constant, leading to deviations from the ideal behavior.

Calculating Equilibrium Constant

The equilibrium constant (Keq) can be calculated using the following steps:

1. Write the balanced chemical equation for the reaction.
2. Identify the stoichiometric coefficients of the reactants and products.
3. Measure the equilibrium concentrations of the reactants and products.
4. Substitute the equilibrium concentrations into the equilibrium constant expression and calculate the value of Keq.

For example, consider the reaction:

H2(g) + I2(g) ⇌ 2HI(g)

At equilibrium, the concentrations are:
[H2] = 0.2 M
[I2] = 0.1 M
[HI] = 0.6 M

The equilibrium constant can be calculated as:

Keq = [HI]^2 / ([H2] * [I2])
Keq = (0.6)^2 / (0.2 * 0.1)
Keq = 0.36 / 0.02
Keq = 18

Acid-Base Equilibria

In the context of acid-base equilibria, the equilibrium constant is often referred to as the acid dissociation constant (Ka) or the base dissociation constant (Kb). These constants are used to quantify the strength of acids and bases, respectively.

Acid Dissociation Constant (Ka)

The acid dissociation constant (Ka) is the equilibrium constant for the dissociation of an acid in water. It is defined as the ratio of the concentrations of the dissociated products (H+ and the conjugate base) to the concentration of the undissociated acid at equilibrium.

The general expression for the acid dissociation constant is:

Ka = [H+] * [A-] / [HA]

Where:
– [H+] is the equilibrium concentration of hydrogen ions
– [A-] is the equilibrium concentration of the conjugate base
– [HA] is the equilibrium concentration of the undissociated acid

The value of Ka provides information about the strength of the acid. A larger Ka value indicates a stronger acid, as it dissociates more in water.

Base Dissociation Constant (Kb)

The base dissociation constant (Kb) is the equilibrium constant for the dissociation of a base in water. It is defined as the ratio of the concentrations of the dissociated products (the conjugate base and OH-) to the concentration of the undissociated base at equilibrium.

The general expression for the base dissociation constant is:

Kb = [B-] * [OH-] / [B]

Where:
– [B-] is the equilibrium concentration of the conjugate base
– [OH-] is the equilibrium concentration of hydroxide ions
– [B] is the equilibrium concentration of the undissociated base

The value of Kb provides information about the strength of the base. A larger Kb value indicates a stronger base, as it dissociates more in water.

Applications of Dynamic Equilibrium in Solution

Dynamic equilibrium in solution has numerous applications in various fields of chemistry, including:

1. Acid-Base Titrations: The concept of dynamic equilibrium is crucial in understanding the behavior of acids and bases during titrations, which are used to determine the concentration of an unknown acid or base.
2. Buffer Solutions: Buffer solutions maintain a relatively constant pH by utilizing the dynamic equilibrium between an acid and its conjugate base or a base and its conjugate acid.
3. Solubility Equilibria: The solubility of sparingly soluble salts in water is governed by dynamic equilibrium, which can be described using the solubility product constant (Ksp).
4. Precipitation Reactions: Dynamic equilibrium plays a role in precipitation reactions, where the formation and dissolution of precipitates are in equilibrium.
5. Biological Systems: Many biological processes, such as the regulation of pH in the human body, involve dynamic equilibria in solution.

Conclusion

Dynamic equilibrium in solution is a fundamental concept in chemistry that describes the state where the forward and reverse reactions occur at equal rates, resulting in no net change in the concentrations of reactants and products. Understanding the principles of dynamic equilibrium, the equilibrium constant (Keq), and its applications in various chemical systems is crucial for students and researchers in the field of chemistry.

This comprehensive guide has provided a wealth of technical and advanced details on dynamic equilibrium in solution, including the factors affecting the equilibrium constant, the calculation of Keq, and the specific applications in acid-base equilibria and other chemical systems. By mastering the concepts presented in this guide, you will be well-equipped to tackle complex problems and deepen your understanding of the dynamic nature of chemical reactions in solution.

Reference:

1. Dynamic Equilibrium – Chemistry LibreTexts
2. Acid-Base Equilibrium – Chemistry LibreTexts
3. Equilibria 16–18 | Resource – RSC Education
4. Equilibrium Constant (Keq) – Chemistry LibreTexts
5. Acid Dissociation Constant (Ka) – Chemistry LibreTexts
6. Base Dissociation Constant (Kb) – Chemistry LibreTexts

A dynamic equilibrium is a state of a reversible reaction where the rate of the forward reaction equals the rate of the backward reaction, and the concentrations of reactants and products remain constant. This means that the reaction is constantly occurring in both directions, but the net change in the concentrations of the reactants and products is zero.

Understanding the Concept of Dynamic Equilibrium

In a dynamic equilibrium, the concentrations of the reactants and products are not necessarily equal, but they are constant. This can be represented by the equation:

$\text{A} + \text{B} \rightleftharpoons \text{C} + \text{D}$

where the concentrations of A, B, C, and D are not changing, but they may not be equal.

The concept of dynamic equilibrium can be further illustrated using the example of a beaker of water with a small amount of food coloring added. As the food coloring spreads throughout the water, the system reaches a state of dynamic equilibrium, where the rate of diffusion of the food coloring in one direction is equal to the rate of diffusion in the opposite direction.

It’s important to note that dynamic equilibria only occur in closed systems, where no matter or energy can enter or leave the system. In an open system, the concentrations of reactants and products can change over time due to the input or output of matter or energy.

Characteristics of Dynamic Equilibrium

1. Constant Concentrations: In a dynamic equilibrium, the concentrations of the reactants and products remain constant over time, even though the forward and backward reactions are continuously occurring.

2. Equality of Reaction Rates: The rate of the forward reaction is equal to the rate of the backward reaction, resulting in a net change of zero in the concentrations of the reactants and products.

3. Reversibility: The reaction is reversible, meaning that the reactants can form the products, and the products can reform the reactants.

4. Closed System: Dynamic equilibria only occur in closed systems, where no matter or energy can enter or leave the system.

5. Equilibrium Constant: The equilibrium constant, denoted as $K_{eq}$, is a measure of the relative concentrations of the reactants and products at equilibrium. It is defined as the ratio of the product concentrations raised to their stoichiometric coefficients to the reactant concentrations raised to their stoichiometric coefficients.

For the reaction $\text{A} + \text{B} \rightleftharpoons \text{C} + \text{D}$, the equilibrium constant is given by:

$K_{eq} = \frac{[C]^c[D]^d}{[A]^a[B]^b}$

where $a$, $b$, $c$, and $d$ are the stoichiometric coefficients of the reactants and products, respectively.

Examples of Dynamic Equilibria

1. Haber Process: The Haber process is an industrial process used to produce ammonia (NH3) from nitrogen (N2) and hydrogen (H2) in a reversible reaction:

$\text{N}_2 + 3\text{H}_2 \rightleftharpoons 2\text{NH}_3$

The equilibrium constant for this reaction is given by:

$K_{eq} = \frac{[NH_3]^2}{[N_2][H_2]^3}$

1. Dissociation of Acetic Acid: The dissociation of acetic acid (CH3COOH) in water is a reversible reaction:

$\text{CH}_3\text{COOH} + \text{H}_2\text{O} \rightleftharpoons \text{CH}_3\text{COO}^- + \text{H}^+$

The equilibrium constant for this reaction is given by:

$K_{eq} = \frac{[CH_3COO^-][H^+]}{[CH_3COOH]}$

1. Evaporation and Condensation of Water: The evaporation and condensation of water in a closed container is a dynamic equilibrium:

$\text{H}_2\text{O}(l) \rightleftharpoons \text{H}_2\text{O}(g)$

The equilibrium constant for this reaction is given by the ratio of the partial pressure of water vapor to the vapor pressure of pure water at the same temperature.

Factors Affecting Dynamic Equilibrium

The position of a dynamic equilibrium can be affected by various factors, such as:

1. Temperature: Changes in temperature can shift the equilibrium position according to the Le Chatelier’s principle. For example, an increase in temperature will favor the endothermic (backward) reaction, while a decrease in temperature will favor the exothermic (forward) reaction.

2. Pressure: Changes in pressure can shift the equilibrium position for reactions involving gases, as described by Le Chatelier’s principle. Increasing the pressure will favor the reaction that produces fewer moles of gas.

3. Concentration: Adding or removing reactants or products can shift the equilibrium position, as described by Le Chatelier’s principle. Increasing the concentration of a reactant will favor the forward reaction, while increasing the concentration of a product will favor the backward reaction.

4. Catalysts: The addition of a catalyst can increase the rates of both the forward and backward reactions, but it does not affect the equilibrium position.

Numerical Problems and Calculations

1. Problem: Consider the reversible reaction: $2\text{SO}2 + \text{O}_2 \rightleftharpoons 2\text{SO}_3$. If the equilibrium concentrations are $[SO_2] = 0.2 \text{M}$, $[O_2] = 0.1 \text{M}$, and $[SO_3] = 0.4 \text{M}$, calculate the equilibrium constant $K{eq}$.

Solution:
The equilibrium constant is given by:
$K_{eq} = \frac{[SO_3]^2}{[SO_2]^2[O_2]}$
Substituting the given values:
$K_{eq} = \frac{(0.4)^2}{(0.2)^2(0.1)} = 8$

1. Problem: The equilibrium constant for the reaction $\text{N}2 + 3\text{H}_2 \rightleftharpoons 2\text{NH}_3$ at a certain temperature is $K{eq} = 1.0 \times 10^4$. If the initial concentrations are $[N_2] = 0.10 \text{M}$ and $[H_2] = 0.30 \text{M}$, calculate the equilibrium concentrations of $\text{N}_2$, $\text{H}_2$, and $\text{NH}_3$.

Solution:
Let the change in concentration of $\text{N}2$ and $\text{H}_2$ be $x$, and the change in concentration of $\text{NH}_3$ be $2x$.
At equilibrium:
$[N_2] = 0.10 – x$
$[H_2] = 0.30 – 3x$
$[NH_3] = 2x$
Substituting these values into the equilibrium constant expression:
$K{eq} = \frac{[NH_3]^2}{[N_2][H_2]^3} = \frac{(2x)^2}{(0.10 – x)(0.30 – 3x)^3} = 1.0 \times 10^4$
Solving this equation numerically, we get $x = 0.0447 \text{M}$.
Therefore, the equilibrium concentrations are:
$[N_2] = 0.0553 \text{M}$
$[H_2] = 0.1359 \text{M}$
$[NH_3] = 0.0894 \text{M}$

Conclusion

In summary, a dynamic equilibrium is a state of a reversible reaction where the rate of the forward reaction equals the rate of the backward reaction, and the concentrations of reactants and products remain constant. This concept is crucial for understanding the behavior of chemical systems and predicting the outcomes of chemical reactions. By understanding the characteristics, factors, and calculations involved in dynamic equilibria, you can gain a deeper insight into the fundamental principles of chemistry.

References

1. Chemical Equilibria Flashcards – Quizlet. Retrieved from https://quizlet.com/569483296/chemical-equilibria-flash-cards/
2. Dynamic Equilibrium – Class 11 Chemistry MCQ – Sanfoundry. Retrieved from https://www.sanfoundry.com/chemistry-questions-answers-equilibrium-chemical-processes-dynamic-equilibrium/
3. Dynamic equilibrium (video) – Khan Academy. Retrieved from https://www.khanacademy.org/science/ap-chemistry-beta/x2eef969c74e0d802:equilibrium/x2eef969c74e0d802:introduction-to-equilibrium/v/dynamic-equilibrium
4. Chem.libretexts.org. (2022). 15.3: The Idea of Dynamic Chemical Equilibrium. Retrieved from https://chem.libretexts.org/Bookshelves/Introductory_Chemistry/Introductory_Chemistry/15:_Chemical_Equilibrium/15.03:_The_Idea_of_Dynamic_Chemical_Equilibrium
5. StudySmarter.co.uk. (n.d.). The Dance of Balance: Understanding Dynamic Equilibrium. Retrieved from https://www.studysmarter.co.uk/explanations/chemistry/physical-chemistry/dynamic-equilibrium/.

Dew point and bubble point are fundamental concepts in thermodynamics and fluid mechanics, crucial for understanding phase transitions and the behavior of refrigeration systems. These parameters play a vital role in various industries, including HVAC, meteorology, and process engineering. In this comprehensive guide, we will delve into the technical details, formulas, and practical applications of dew point and bubble point, providing a valuable resource for physics students.

Understanding Dew Point

Dew point is the temperature at which a gas, typically water vapor, begins to condense into a liquid when the gas is cooled. At a given temperature, there is a maximum amount of water vapor that the air can hold before it reaches its dew point and condensation occurs. The dew point temperature is an essential measurement in various applications, as it helps determine the moisture content in the air and the potential for condensation formation.

The Antoine Equation and Dew Point Calculation

The relationship between dew point, temperature, and pressure is described by the Antoine equation, a semi-empirical formula used to estimate the vapor pressure of a pure component at a given temperature. The Antoine equation is expressed as:

log10(P) = A - (B / (C + T))

where:
– P is the vapor pressure (in appropriate units)
– T is the temperature in Kelvin
– A, B, and C are component-specific constants

To calculate the dew point, you can rearrange the Antoine equation to solve for the temperature T when the vapor pressure P is known, typically the saturation vapor pressure of the gas at the given conditions.

Dew Point in Refrigeration Systems

In the context of zeotropic refrigerants, the dew point occurs at a higher temperature during the condensation process compared to the bubble point during boiling. This is because the temperature difference between the refrigerant and the surrounding environment is greater during condensation than during boiling. This temperature difference drives the phase change process, with the dew point occurring at a higher temperature when the refrigerant is condensing.

Understanding Bubble Point

Bubble point, on the other hand, is the temperature at which a liquid begins to vaporize when it is heated. It is the highest temperature at which a liquid and its vapor can coexist in equilibrium. The bubble point pressure is the pressure at which the liquid begins to boil, and it is an essential measurement in the design and operation of distillation columns and other separation processes.

The Antoine Equation and Bubble Point Calculation

Similar to the dew point, the bubble point can be calculated using the Antoine equation. In this case, you would need to rearrange the equation to solve for the temperature T when the vapor pressure P is known, typically the saturation vapor pressure of the liquid at the given conditions.

Bubble Point in Refrigeration Systems

In the context of zeotropic refrigerants, the bubble point occurs at a lower temperature during the boiling process compared to the dew point during condensation. This is because the temperature difference between the refrigerant and the surrounding environment is greater during condensation than during boiling. This temperature difference drives the phase change process, with the bubble point occurring at a lower temperature when the refrigerant is boiling.

Hysteresis Effects in Nanopores

The bubble point and dew point of hydrocarbons in nanopores exhibit hysteresis effects, meaning that the bubble point and dew point curves do not overlap. This phenomenon is due to the pore structure and surface chemistry of nanopores, which can significantly affect the phase behavior of hydrocarbons.

Calculating Dew Point and Bubble Point using Aspen HYSYS

Aspen HYSYS, a widely used process simulation software, provides tools for calculating both dew point and bubble point. The Peng-Robinson equation of state or other thermodynamic models available in the software can be used to determine these important parameters.

Practical Applications and Importance

Dew point and bubble point are crucial measurements in various industries, including:

1. HVAC: Dew point is used to determine the moisture content in the air and the potential for condensation, which is essential for the design and operation of HVAC systems.
2. Meteorology: Dew point is a key parameter in weather forecasting and understanding atmospheric conditions.
3. Process Engineering: Bubble point and dew point are important in the design and operation of distillation columns, evaporators, and other separation processes.
4. Refrigeration Systems: The temperature difference between the bubble point and dew point of zeotropic refrigerants is a crucial factor in the performance and efficiency of refrigeration systems.

Conclusion

Dew point and bubble point are fundamental concepts in thermodynamics and fluid mechanics, with far-reaching applications in various industries. By understanding the technical details, formulas, and practical implications of these parameters, physics students can gain a deeper understanding of phase transitions and the behavior of complex systems. This comprehensive guide provides a valuable resource for students to explore the intricacies of dew point and bubble point, equipping them with the knowledge to tackle real-world problems and advance their studies in the field of physics.

References

1. Can someone help me understand bubble point and dew point?
2. Bubble/dew point and hysteresis of hydrocarbons in nanopores from molecular perspective
3. Calculating Bubble Point and Dew Point using Aspen HYSYS

Summary

Length is a fundamental physical quantity that is considered an extensive property, meaning it depends on the size or extent of the system being measured. This article delves into the technical details of length as an extensive quantity, providing a comprehensive guide for physics students and enthusiasts. We will explore the various units used to measure length, the instruments and techniques employed, the mathematical formulas and calculations involved, and the practical applications of length measurements in different fields. By the end of this article, you will have a deep understanding of the extensive nature of length and how to effectively work with it in your studies and research.

Understanding Extensive Quantities

Extensive quantities are physical properties that depend on the size or extent of the system being measured. In contrast, intensive quantities are independent of the system’s size and are typically ratios or concentrations. Length, along with other properties such as volume, mass, and charge, are considered extensive quantities.

The key characteristic of an extensive quantity is that it is additive. For example, if you have two rods of lengths 5 meters and 3 meters, the total length of the combined rods is 8 meters (5 m + 3 m). This additive property is a defining feature of extensive quantities.

Measuring Length

Length is typically measured using various units, such as:

1. SI units: Meter (m), centimeter (cm), millimeter (mm), micrometer (μm), nanometer (nm)
2. Imperial units: Inch (in), foot (ft), yard (yd), mile (mi)

The choice of unit depends on the scale of the object being measured and the required precision. For example, measuring the length of a building would typically use meters or feet, while measuring the thickness of a human hair would use micrometers or nanometers.

Measurement Instruments

There are several instruments used to measure length, including:

1. Ruler: A simple tool with marked increments, often used for small-scale measurements.
2. Tape measure: A flexible measuring tool that can be used for longer distances.
3. Caliper: A device with two jaws that can measure the distance between two parallel surfaces, useful for precise measurements.
4. Micrometer: A specialized instrument that can measure dimensions to a high degree of accuracy, typically used for small-scale measurements.
5. Laser interferometer: An advanced instrument that uses the interference of laser beams to measure length with extremely high precision, often used in scientific research and engineering applications.

Measurement Techniques

When measuring length, it is important to follow standardized procedures to ensure accuracy and reliability. Some common techniques include:

1. Direct measurement: Placing the object directly against the measuring instrument, such as a ruler or tape measure.
2. Indirect measurement: Using mathematical formulas to calculate the length based on other measured quantities, such as the circumference of a circle to determine its diameter.
3. Comparative measurement: Comparing the object being measured to a known reference standard, such as using a calibrated gauge block to verify the accuracy of a micrometer.

Calculating Length-Related Quantities

In addition to directly measuring length, there are various mathematical formulas and calculations that involve length as an extensive quantity. Some examples include:

Area Calculation

The area of a two-dimensional shape is calculated by multiplying its length and width. For example, the area of a rectangle with a length of 5 meters and a width of 3 meters would be:

Area = Length × Width
Area = 5 m × 3 m = 15 m²

Volume Calculation

The volume of a three-dimensional object is calculated by multiplying its length, width, and height. For example, the volume of a cube with a side length of 2 meters would be:

Volume = Length × Width × Height
Volume = 2 m × 2 m × 2 m = 8 m³

Perimeter Calculation

The perimeter of a two-dimensional shape is the sum of the lengths of all its sides. For example, the perimeter of a square with a side length of 4 meters would be:

Perimeter = 4 × Length of one side
Perimeter = 4 × 4 m = 16 m

Circumference Calculation

The circumference of a circle is calculated using the formula:

Circumference = 2 × π × Radius
or
Circumference = π × Diameter

Where π (pi) is the mathematical constant approximately equal to 3.14159.

Applications of Length Measurements

Length measurements have a wide range of applications in various fields, including:

1. Engineering and Construction: Measuring the dimensions of buildings, bridges, machinery, and other structures to ensure proper design and construction.
2. Manufacturing: Precisely measuring the dimensions of parts and components to ensure quality control and interchangeability.
3. Scientific Research: Measuring the size and scale of objects in fields like astronomy, biology, and nanotechnology.
4. Transportation: Measuring the dimensions of vehicles, roads, and infrastructure to ensure safe and efficient transportation.
5. Surveying and Mapping: Measuring the distances and elevations of land features to create accurate maps and plans.
6. Medical and Biological Applications: Measuring the size and dimensions of organs, tissues, and cells for diagnostic and research purposes.

Numerical Examples and Problems

1. Example 1: A rectangular room has a length of 5 meters and a width of 3 meters. Calculate the:
2. Area of the room

3. Perimeter of the room

4. Example 2: A cylindrical storage tank has a diameter of 2.5 meters and a height of 4 meters. Calculate the:

5. Volume of the tank

6. Circumference of the tank

7. Problem 1: A rectangular plot of land has a length of 50 meters and a width of 30 meters. If the plot is divided into 10 equal-sized smaller plots, what is the area of each smaller plot?

8. Problem 2: A metal rod has a length of 1.2 meters. If the rod is cut into 6 equal-sized pieces, what is the length of each piece?

9. Problem 3: A circular swimming pool has a diameter of 12 meters. Calculate the:

10. Circumference of the pool
11. Area of the pool

Conclusion

Length is a fundamental extensive quantity that plays a crucial role in various fields of study and practical applications. By understanding the concepts of extensive quantities, the units and instruments used for length measurement, and the mathematical formulas involved, you can effectively work with length-related problems and gain a deeper understanding of the physical world around you.

References

1. What is Quantitative Data? [Definition, Examples & FAQ]
2. Quantitative Data 101: What is quantitative data?
3. Measuring Data Quality – 7 Metrics to Assess Your Data
4. What is Quantitative Data? Types, Examples & Analysis

Summary

In the realm of physics and thermodynamics, the concept of “is area intensive” is a crucial topic that delves into the understanding of intensive properties. Intensive properties are physical quantities whose values do not depend on the amount of substance being measured, and they play a vital role in various applications, from material science to energy systems. This comprehensive guide will explore the intricacies of area-intensive properties, providing physics students with a deep dive into the theoretical foundations, practical applications, and quantifiable data that define this essential concept.

Understanding Intensive Properties

Intensive properties are a fundamental concept in physics and thermodynamics, and they are characterized by their independence from the size or amount of the system being studied. These properties are in contrast to extensive properties, which do depend on the size or amount of the system.

Defining Intensive Properties

Intensive properties are physical quantities that remain constant regardless of the size or amount of the system. Some examples of intensive properties include:

1. Temperature: The temperature of a substance is an intensive property, as it does not change with the amount of the substance.
2. Pressure: The pressure exerted by a fluid or gas is an intensive property, as it is independent of the volume of the system.
3. Density: The density of a material is an intensive property, as it is the mass per unit volume and does not depend on the total mass or volume of the system.

Relationship between Intensive and Extensive Properties

Extensive properties, on the other hand, are physical quantities that depend on the size or amount of the system. Examples of extensive properties include:

1. Mass: The total mass of a system is an extensive property, as it depends on the amount of material present.
2. Volume: The total volume of a system is an extensive property, as it depends on the size of the system.
3. Energy: The total energy of a system is an extensive property, as it depends on the amount of matter and energy present.

The relationship between intensive and extensive properties is crucial in understanding the behavior of physical systems. Intensive properties can be used to describe the state of a system, while extensive properties can be used to quantify the size or amount of the system.

Area Density: The Quintessential Area-Intensive Property

In the context of area-intensive properties, the concept of area density is particularly important. Area density is defined as the ratio of an extensive property, such as mass or charge, to the area over which it is distributed.

Defining Area Density

Area density, denoted as σ (sigma), is calculated as:

σ = Q / A

Where:
– σ is the area density
– Q is the extensive property (e.g., mass, charge)
– A is the area over which the extensive property is distributed

The key characteristic of area density is that it is an intensive property, meaning its value remains constant regardless of the size of the system, as long as the substance’s properties per unit area remain unchanged.

Examples of Area Density

1. Mass Area Density: If we have a metal plate with a uniform distribution of mass, the mass area density can be calculated as the mass of the plate divided by its surface area. This value will remain constant regardless of the size of the plate.

2. Charge Area Density: In the case of a charged capacitor with a uniform distribution of charge, the charge area density can be calculated as the charge of the capacitor divided by its surface area. Again, this value will remain constant regardless of the size of the capacitor.

3. Energy Area Density: The energy area density of a solar panel can be calculated as the total energy output divided by the surface area of the panel. This value represents the energy generated per unit area and is an intensive property.

Practical Applications of Area Density

Area density is a crucial concept in various fields of physics and engineering, including:

1. Material Science: Area density is used to characterize the properties of thin films, coatings, and surface-based materials, where the distribution of mass or charge per unit area is of importance.

2. Electromagnetism: In the study of electromagnetic fields, the concept of charge area density is used to understand the distribution of electric charge on the surface of conductors and the resulting electric field.

3. Energy Systems: Area density is particularly relevant in the design and analysis of energy systems, such as solar panels, where the energy output per unit area is a critical performance metric.

4. Biomedical Engineering: In biomedical applications, area density can be used to characterize the distribution of biological molecules or cells on a surface, which is important in the development of biosensors and diagnostic devices.

Quantifying Area Density: Formulas and Calculations

To quantify the area density of a system, we can use various formulas and calculations based on the specific physical properties involved.

Mass Area Density

The mass area density, σ_m, is calculated as:

σ_m = m / A

Where:
– σ_m is the mass area density
– m is the mass of the system
– A is the surface area of the system

For example, if a metal plate has a mass of 5 kg and a surface area of 2 m^2, the mass area density would be:

σ_m = 5 kg / 2 m^2 = 2.5 kg/m^2

Charge Area Density

The charge area density, σ_q, is calculated as:

σ_q = Q / A

Where:
– σ_q is the charge area density
– Q is the total charge of the system
– A is the surface area of the system

For instance, if a charged capacitor has a total charge of 10 μC and a surface area of 0.5 m^2, the charge area density would be:

σ_q = 10 μC / 0.5 m^2 = 20 μC/m^2

Energy Area Density

The energy area density, σ_E, is calculated as:

σ_E = E / A

Where:
– σ_E is the energy area density
– E is the total energy of the system
– A is the surface area of the system

For example, if a solar panel has a total energy output of 500 W and a surface area of 2 m^2, the energy area density would be:

σ_E = 500 W / 2 m^2 = 250 W/m^2

Numerical Examples

1. Metal Plate:
2. Mass: 10 kg
3. Surface Area: 4 m^2

4. Mass Area Density: σ_m = 10 kg / 4 m^2 = 2.5 kg/m^2

5. Charged Capacitor:

6. Charge: 50 μC
7. Surface Area: 0.2 m^2

8. Charge Area Density: σ_q = 50 μC / 0.2 m^2 = 250 μC/m^2

9. Solar Panel:

10. Energy Output: 1 kW
11. Surface Area: 5 m^2
12. Energy Area Density: σ_E = 1 kW / 5 m^2 = 200 W/m^2

These examples demonstrate how to calculate the area density for different physical properties and systems, highlighting the importance of understanding the relationship between the extensive property and the area over which it is distributed.

Advanced Concepts and Considerations

As you delve deeper into the topic of area-intensive properties, there are several advanced concepts and considerations that you should be aware of.

Tensor Representation of Area Density

In some cases, area density can be represented as a tensor quantity, which takes into account the directionality and anisotropy of the physical property being measured. This is particularly relevant in the study of electromagnetic fields, where the charge area density can be represented as a tensor to account for the directional distribution of charge on a surface.

Relationship to Surface Integral

The area density of a physical property can be related to the surface integral of that property over the area of interest. This connection allows for the use of integral calculus in the analysis of area-intensive properties, providing a powerful mathematical framework for understanding and quantifying these concepts.

Dimensional Analysis and Units

When working with area-intensive properties, it is crucial to pay attention to the dimensional analysis and units of the quantities involved. Ensuring the consistency and proper units of the variables used in the calculations is essential for obtaining meaningful and accurate results.

Limitations and Assumptions

It is important to note that the concept of area density, like any other physical property, is subject to certain limitations and assumptions. For example, the assumption of a uniform distribution of the extensive property over the area may not always hold true, and the effects of edge cases or non-uniform distributions should be considered in the analysis.

Conclusion

In the realm of physics and thermodynamics, the concept of “is area intensive” is a fundamental topic that delves into the understanding of intensive properties. By exploring the intricacies of area-intensive properties, particularly the concept of area density, this comprehensive guide has provided physics students with a deep dive into the theoretical foundations, practical applications, and quantifiable data that define this essential concept.

Through the discussion of intensive and extensive properties, the definition and examples of area density, and the formulas and calculations for quantifying area-intensive properties, this guide has equipped readers with the necessary knowledge and tools to navigate the complexities of this topic. Additionally, the exploration of advanced concepts, such as tensor representation and the relationship to surface integrals, has further expanded the understanding of the nuances involved in the study of area-intensive properties.

By mastering the concepts presented in this guide, physics students will be better equipped to apply their knowledge in various fields, from material science and electromagnetism to energy systems and biomedical engineering. The ability to quantify and analyze area-intensive properties is a crucial skill that will serve them well in their academic and professional pursuits.

References

1. Wikipedia, “Intensive and extensive properties”
2. Investopedia, “Quantitative Analysis (QA): What It Is and How It’s Used in Finance”
3. Unimrkt Research, “What are the strengths of quantitative research?”
4. Fullstory, “What is Quantitative Data? Types, Examples & Analysis”

Radioactive decay occurs when an unstable nucleus releases energy through radiation and becomes a stable nuclei. Radioactive disintegration can be in the form of alpha particles, beta particles, gamma rays, positron emission, electron capture,etc. Few radioactive decay examples are discussed in detail in this article.

• Alpha decay of Uranium-238 nucleus
• Beta decay of Thorium-234 nucleus
• Alpha decay of Polonium-210 nucleus
• Beta decay of Iodine-131 nucleus
• Gamma decay of Cobalt-60 nucleus
• Positron emission of Oxygen-15 nucleus
• Electron capture of Potassium-40
• Alpha decay of Uranium-234 nucleus
• Alpha decay of Thorium-230 nucleus
• Alpha decay of Radium-226
• Alpha decay of Polonium-218 nucleus
• Alpha decay of Radon-222 nucleus
• Beta decay of Lead-214
• Beta decay of Bismuth-214
• Alpha decay of Polonium-214
• Beta decay of Lead-210
• Beta decay of Bismuth-210

Alpha decay of Uranium-238 nucleus

Uranium-238, most common isotope of Uranium, undergoes alpha decay and forms Thorium-234. During this reaction, unstable uranium-238 nucleus loses 2 protons and 2 neutrons to form thorium-234. The alpha particle can be regarded as a Helium nucleus. 

The alpha particles are less penetrating than other forms of radiation. Sometimes weak gamma rays are also emitted during the decay process. Of all the radioactive disintegration processes, alpha decay is the least dangerous.

The radioactive decay can be shown as

Beta decay of Thorium-234 nucleus

The thorium-234 nuclide undergoes beta decay by releasing an electron and protactinium-234 is formed. This kind of beta decay is known as beta minus decay since an energetic negative electron is released. 

The decay process can be depicted by the following balanced equation:

The

represents anti-neutrino.

As mentioned earlier, the decay of thorium-234 to protactinium-234 is a beta minus decay. The underlying process is that a neutron breaks into a proton plus an electron; and the electron is released out of the nucleus while the proton stays inside the nucleus.

Alpha decay of Polonium-210 nucleus

Polonium is one of the naturally occurring radioactive element and occurs in relatively very low concentrations in the Earth’s crust.

Polonium-210, stable isotope of polonium, decays into a stable nucleus lead-206 by emitting an alpha particle. The alpha particles emitted from polonium-210 are capable of ionizing adjacent air which in turn, neutralizes static electricity on the surfaces that are in contact with air.

The decay process can be represented as follows:

Polonium-210 finds applications in many static eliminators which are essentially used to eliminate static electricity in certain devices due to the property of the emitted alpha particles.

Beta decay of Iodine-131 nucleus

Iodine-131 nucleus undergoes beta decay and forms a stable xenon-131 nucleus. This is also a beta-minus decay. 

The decay reaction is as given below:

Since both beta particle and gamma ray are emitted, it is also known as a beta-gamma emitter. This makes it useful in the field of nuclear medicine.

Gamma decay of Cobalt-60 nucleus

Cobalt-60 is a radioactive isotope of cobalt but not naturally occurring.

The actual reaction takes place by the beta decay of Cobalt-60 to produce stable Nickel-60 and this nucleus emits two gamma rays.

The reaction can be represented as:

Being a high intensity gamma emitter, Cobalt-60 has several applications like radiation source for radiotherapy, food irradiation, pest insect sterilization, and so on.

Positron emission of Oxygen-15 nucleus

The neutron to proton ratio is a key factor that determines the stability of any nucleus. Radioactive decays takes place to stabilize the nucleus.

In oxygen-15, the number of neutrons is 7 which is less than the number of protons i.e., 8. Hence it undergoes positron emission and nitrogen-15 is formed. Positron emission is otherwise known as beta plus decay.

This is what happens in a positron emission:

The reaction of beta plus decay of oxygen-15 can be represented as:

Electron capture of Potassium-40

Potassium-40 is an example for a naturally occurring radioactive isotope of potassium, but relatively in very small fraction, around 0.012%.

Electron capture is a radioactive decay process when there is an abundance of protons in the nucleus compared to neutrons in addition to the insufficient energy for positron emission.

During an electron capture, nucleus captures an atomic electron and hence proton is transformed to neutron.

The electron capture of potassium-40 is

Alpha decay of Uranium-234 nucleus

The uranium-234 is an indirect decay product of uranium-238 and is immediately converted to thorium-230 by alpha decay.

Emitted alpha particle is comparatively less penetrative and thorium-230 is formed.

The decay reaction is:

Alpha decay of Thorium-230 nucleus

Thorium-230 is one of the naturally occurring radioactive isotopes of thorium.

Thorium-230 is a part of the uranium decay series and radium-226 is the product of radioactive decay of this thorium nucleus. Alpha particles are emitted during the process.

The alpha decay can be shown as:

Thorium-230, being a decay product of Uranium-238, is found in uranium deposits and in uranium mill tailings.

Alpha decay of Radium-226

Radium is an alpha particle radiator, a decay product of uranium-238 decay series and is present in rocks and soils in different amounts.

Radium-226 yields radon-222, a radioactive inert gas upon alpha particle emission.

The decay reaction is:

Radium is highly radioactive as it is about one million times more radioactive than uranium and the decay product, radon is used nowadays to treat various forms of cancer.

Alpha decay of Polonium-218 nucleus

Polonium-218 disintegrates mainly by alpha decay although it is observed that beta emission takes place in fewer amounts in some cases.

Alpha disintegration of polonium-218 can be represented by the following reaction:

Alpha decay of Radon-222 nucleus

Radon-222, a highly radioactive gaseous element, is radon’s most stable isotope. Radon-222 is one of the leading causes of lung cancer as it is a gas and radioactive.

Radon-222 undergoes alpha disintegration and polonium-218 is produced.

The disintegration reaction is:

Radon is a major cancer-causing agent as it can be inhaled and before its exhalation, it undergoes decay producing alpha particles and/or gamma rays which can damage our cells. Hence radon can cause lung cancer.

Beta decay of Lead-214

Lead-214 undergoes beta emission and forms Bismuth-214. The type of beta decay is beta minus decay.

The radioactive process can be shown as:

Beta decay of Bismuth-214

Bismuth-214 undergoes beta disintegration to form Polonium-214 nuclide. The decay process is beta minus decay.

The underlying reaction is:

Alpha decay of Polonium-214

The alpha decay of polonium-214 yields lead-210.

The representation of the decay reaction is:

Beta decay of Lead-210

Lead-210 is a naturally occurring radioactive nuclide of the uranium decay series.

A beta minus decay of lead-210 yields bismuth-210. This process is accompanied by emission of energy through gamma rays.

The reaction for the beta minus disintegration can be represented in the following way:

Beta decay of Bismuth-210

Bismuth-210 undergoes beta disintegration and forms polonium-210.

The beta minus decay can be depicted as follows:

In nature, polonium is found more concentrated in tobacco. Being an alpha emitter when tobacco is smoked, polonium gets inhaled leading to the damage of cells due to the emitted alpha particles from polonium.

Conclusion

In this article, several radioactive decay examples have been discussed in detail. Eventhough exposure to radiation is harmful in several contexts; some radioactive decay processes find application in medical field, especially to treat cancer. Apart from medical applications, several industrial processes make use of decay process depending on the needs.

Also Read:

• Why is vitamin d often called the sunshine vitamin
• Where do phenomena like halos and sun dogs come from in meteorology
• Amplitude
• Movable pulley examples
• Galaxy definition formation 5 interesting facts
• Is malleability a physical property
• Fixed pulley examples
• Loudness and amplitude
• Is brass malleable
• Is hardness a physical property