Mass - lambdageeks.com

How To Find Torque with Mass: With Problem Examples

January 21, 2024May 22, 2022 by Harshitha H N

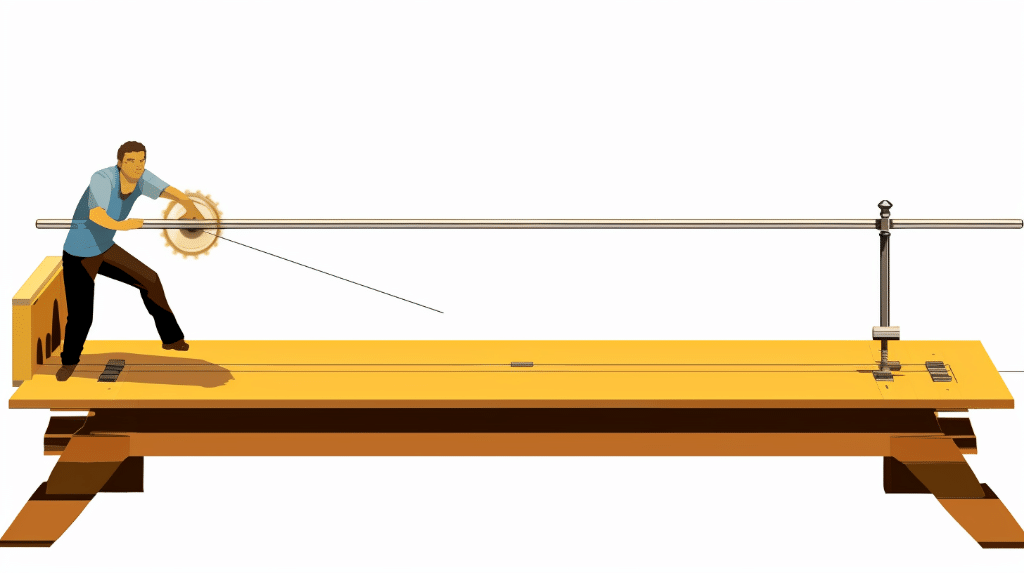
Torque is a fundamental concept in physics that describes the rotational force applied to an object. It plays a crucial role in understanding rotational motion and is essential for various applications, such as engineering, mechanics, and even sports. In this blog post, we will explore how to find torque with mass and distance, providing you with a clear understanding of the underlying principles and practical calculations involved.

How to Calculate Torque with Mass and Distance

The Torque Equation

To calculate torque, we use the equation:

$\text{Torque} = \text{Force} \times \text{Distance}$

In this equation, the force refers to the force applied to the object, and the distance is the perpendicular distance between the axis of rotation and the point where the force is applied. The unit of torque is typically expressed in Newton-meters (Nm) or foot-pounds (ft-lb), depending on the unit system used.

Steps to Calculate Torque Given Mass and Distance

To calculate torque using mass and distance, you need to follow these steps:

Identify the force applied to the object. This force can be due to various factors, such as gravity, friction, or an external force applied intentionally.
Determine the distance from the axis of rotation to the point where the force is applied. This distance should be measured perpendicular to the line of action of the force.
Multiply the force by the distance to calculate the torque.

Let’s walk through an example to illustrate this process.

Worked Out Example: Calculating Torque with Mass and Distance

Suppose we have a wooden beam of mass 10 kg, and we apply a force of 20 N at a distance of 2 meters from the axis of rotation. To find the torque, we can use the equation mentioned earlier:

$\text{Torque} = \text{Force} \times \text{Distance}$

Substituting the values, we get:

$\text{Torque} = 20 \, \text{N} \times 2 \, \text{m} = 40 \, \text{Nm}$

Thus, the torque applied to the wooden beam is 40 Nm.

Advanced Concepts in Finding Torque

Finding Torque for Angled Forces

In some cases, the force may not act perpendicular to the line connecting the point of application to the axis of rotation. In such situations, we can find the torque by considering the perpendicular component of the force.

To calculate the torque when the force is at an angle, we use the following modified equation:

$\text{Torque} = \text{Force} \times \text{Distance} \times \sin(\theta)$

Where:
– $\theta$ is the angle between the force vector and the line connecting the point of application to the axis of rotation.

How to Find Torque without Mass

While mass is commonly used to calculate torque, it is essential to note that torque can also be determined without explicitly considering the mass of the object. In such cases, we rely on the concept of moment of inertia.

The moment of inertia $\(I$ ) of an object is a measure of its resistance to rotational motion. It depends on both the mass distribution and the axis of rotation. The torque can be calculated using the following equation:

$\text{Torque} = \text{Moment of Inertia} \times \text{Angular Acceleration}$

Where:
– $\text{Moment of Inertia}$ is a property of the object determined by its mass distribution and shape,
– $\text{Angular Acceleration}$ refers to the rate of change of angular velocity.

How to Find Torque without Force

Similarly, torque can also be determined without explicitly knowing the force applied to the object. This is possible when the angular acceleration is known. In such cases, we can use the following equation to calculate torque:

$\text{Torque} = \text{Moment of Inertia} \times \text{Angular Acceleration}$

This equation is derived from Newton’s second law for rotational motion, which states that the torque on an object is equal to the product of moment of inertia and angular acceleration.

Finding the Magnitude of the Torque

In certain scenarios, it may be necessary to find the magnitude of the torque acting on an object without considering its direction. To do so, we can use the following equation:

$\text{Magnitude of Torque} = \text{Force} \times \text{Lever Arm Length}$

The lever arm length is the perpendicular distance between the axis of rotation and the line of action of the force.

Practical Applications of Torque Calculations

How to Find Torque on a Wheel

One practical application of torque calculations is determining the torque exerted on a wheel. This is particularly important in the automotive industry, where torque is used to measure the force that drives a vehicle’s wheels. By accurately calculating the torque, engineers can design more efficient and powerful vehicles.

How to Find the Mass of a Ruler Using Torque

how to find torque with mass — Image by Er. Darshan Dodia – Wikimedia Commons, Wikimedia Commons, Licensed under CC BY-SA 4.0.

Torque can also be used to find the mass of an object with a known distance and force applied. For example, consider a ruler balanced on a pivot point. By applying a known force at a measured distance from the pivot, we can calculate the mass of the ruler using the torque equation.

How to Find Center of Mass with Torque

Torque calculations can be used to determine the center of mass of an object. By applying a force to the object at different distances from the axis of rotation and measuring the resulting torque, we can find the position of the center of mass. This information is crucial in various fields, including engineering, physics, and biomechanics.

By understanding how to find torque with mass and distance, you can apply this knowledge to solve various real-world problems involving rotational motion.

Numerical Problems on how to find torque with mass

A disk with a mass of 2 kg is rotating with an angular velocity of 3 radians per second. Calculate the torque exerted on the disk.

Solution:

Given:
Mass of the disk, $m = 2 \, \text{kg}$
Angular velocity, $\omega = 3 \, \text{rad/s}$

The formula to calculate torque is given by:
$\text{Torque} = I \cdot \alpha$

Where:
$I$ is the moment of inertia of the disk
$\alpha$ is the angular acceleration of the disk

To find the moment of inertia, we can use the formula:
$I = m \cdot r^2$

Where:
$r$ is the radius of the disk

Assuming the disk has a radius of 0.5 meters, we can calculate the moment of inertia:
$I = 2 \, \text{kg} \cdot (0.5 \, \text{m})^2 = 0.5 \, \text{kg} \cdot \text{m}^2$

Since the angular acceleration is zero (as there is no change in angular velocity), the torque exerted on the disk is also zero.

Therefore, the torque exerted on the disk is 0 Nm.

$//$ : # (This is a comment in Markdown format, the LaTeX expression should not be rendered by the markdown renderer.)

A uniform rod of length 1.5 meters and mass 4 kg is pivoted at one end and is at rest. A force of 10 N is applied perpendicular to the rod at a distance of 0.5 meters from the pivot point. Find the torque exerted on the rod by the force.

Solution:

Given:
Length of the rod, $L = 1.5 \, \text{m}$
Mass of the rod, $m = 4 \, \text{kg}$
Force applied, $F = 10 \, \text{N}$
Distance from the pivot point, $r = 0.5 \, \text{m}$

The formula to calculate torque is given by:
$\text{Torque} = F \cdot r$

Substituting the given values:
$\text{Torque} = 10 \, \text{N} \cdot 0.5 \, \text{m} = 5 \, \text{Nm}$

Therefore, the torque exerted on the rod by the force is 5 Nm.

A wheel with a moment of inertia of 0.2 kg·m² is rotating with an angular velocity of 4 radians per second. Calculate the torque required to stop the wheel in 2 seconds.

Solution:

Given:
Moment of inertia of the wheel, $I = 0.2 \, \text{kg} \cdot \text{m}^2$
Angular velocity, $\omega = 4 \, \text{rad/s}$
Time, $t = 2 \, \text{s}$

The formula to calculate torque is given by:
$\text{Torque} = I \cdot \alpha$

Where:
$\alpha$ is the angular acceleration of the wheel

Since we want to stop the wheel, the final angular velocity will be 0 rad/s. We can find the angular acceleration using the formula:
$\omega = \omega_0 + \alpha \cdot t$

Substituting the given values:
$0 = 4 \, \text{rad/s} + \alpha \cdot 2 \, \text{s}$

Solving for $\alpha$ :
$\alpha = \frac{-4 \, \text{rad/s}}{2 \, \text{s}} = -2 \, \text{rad/s}^2$

Substituting the values of $I$ and $\alpha$ in the torque formula:
$\text{Torque} = 0.2 \, \text{kg} \cdot \text{m}^2 \cdot (-2 \, \text{rad/s}^2) = -0.4 \, \text{Nm}$

Therefore, the torque required to stop the wheel in 2 seconds is -0.4 Nm.

Also Read:

Does Energy Have Mass?

June 24, 2024May 21, 2022 by AKSHITA MAPARI

The idea that energy and mass are interchangeable is a fundamental concept in modern physics, encapsulated in Einstein’s famous equation E=mc^2. This equation states that energy (E) is equal to mass (m) times the speed of light (c) squared, suggesting that mass can be converted into energy, and vice versa.

The Principle of Mass-Energy Equivalence

The principle of mass-energy equivalence is a cornerstone of modern physics, with far-reaching implications for our understanding of the universe. This principle is based on the following key points:

Energy has Mass: According to Einstein’s equation, E=mc^2, energy and mass are equivalent, and can be converted into one another. This means that energy itself has a certain amount of mass associated with it.
Relativistic Energy: The total energy of an object in motion, including its kinetic energy and rest mass energy, is known as its relativistic energy. This is given by the equation E=γmc^2, where γ (the Lorentz factor) depends on the velocity of the object.
Relativistic Work-Energy Theorem: This theorem states that the net work done on an object is equal to the change in its relativistic energy. This can be used to calculate the kinetic energy of an object in motion, given by the equation KE=γmc^2-mc^2.
Conservation of Mass-Energy: In classical physics, mass and energy were separately conserved. However, in relativistic physics, mass and energy are different forms of the same thing, and are conserved together. This means that mass can be converted into energy, and energy can be converted into mass, as long as the total mass-energy is conserved.

Examples of Mass-Energy Conversion

The principle of mass-energy equivalence is demonstrated in various physical phenomena:

Nuclear Fission: In nuclear fission, the mass of a heavy atomic nucleus is converted into energy when it is split into smaller nuclei. The mass of the original nucleus is slightly more than the combined mass of the resulting fragments, and this difference is converted into energy, which can be harnessed to generate electricity in nuclear power plants.
Matter-Antimatter Annihilation: When a particle and its antiparticle collide, they annihilate each other and produce energy in the form of gamma rays. Conversely, when energy is converted into matter, as in the Big Bang or in particle accelerators, the resulting particles have mass.
Particle Accelerators: In particle accelerators, such as the Large Hadron Collider (LHC), high-energy collisions between particles can create new particles with mass, demonstrating the conversion of energy into mass.

Quantifying Mass-Energy Equivalence

The relationship between mass and energy can be quantified using the following equations:

Einstein’s Mass-Energy Equivalence Equation: E = mc^2, where E is the energy, m is the mass, and c is the speed of light.
Relativistic Energy Equation: E = γmc^2, where γ (the Lorentz factor) is given by γ = 1/√(1 – v^2/c^2), and v is the velocity of the object.
Relativistic Kinetic Energy Equation: KE = γmc^2 – mc^2, where KE is the kinetic energy of the object.

These equations allow us to calculate the energy equivalent of a given mass, or the mass equivalent of a given energy, and to understand the relationship between energy, mass, and velocity in the context of relativistic physics.

Experimental Verification

The principle of mass-energy equivalence has been extensively tested and verified through numerous experiments, including:

Atomic Mass Measurements: Precise measurements of the masses of atomic nuclei before and after nuclear reactions have confirmed the conversion of mass into energy.
Particle Collisions: Experiments in particle accelerators have demonstrated the creation of new particles with mass from the conversion of energy during high-energy collisions.
Gravitational Lensing: Observations of gravitational lensing, where the bending of light by massive objects is used to infer the presence of dark matter, have provided indirect evidence for the mass-energy equivalence.
Atomic Clocks: Experiments with atomic clocks have verified the time dilation predicted by special relativity, which is a consequence of the mass-energy equivalence.

These experimental results have firmly established the principle of mass-energy equivalence as a fundamental tenet of modern physics.

Implications and Applications

The principle of mass-energy equivalence has far-reaching implications and applications in various fields of physics and technology:

Nuclear Energy: The conversion of mass into energy is the basis for nuclear power generation, where the energy released during nuclear fission or fusion reactions is harnessed to produce electricity.
Particle Physics: The creation and annihilation of particles in high-energy collisions, as well as the properties of subatomic particles, are directly related to the mass-energy equivalence.
Astrophysics and Cosmology: The mass-energy equivalence is crucial for understanding the evolution of the universe, the formation of stars and galaxies, and the nature of dark matter and dark energy.
General Relativity: The mass-energy equivalence is a fundamental aspect of Einstein’s theory of general relativity, which describes the relationship between gravity, space, and time.
Technology Applications: The mass-energy equivalence has enabled the development of technologies such as particle accelerators, nuclear weapons, and medical imaging techniques like positron emission tomography (PET) scans.

The principle of mass-energy equivalence is a cornerstone of modern physics, with profound implications for our understanding of the universe and the development of transformative technologies.

Conclusion

The principle of mass-energy equivalence, as expressed in Einstein’s famous equation E=mc^2, is a fundamental concept in modern physics. This principle demonstrates that energy and mass are interchangeable, and that energy itself has a certain amount of mass associated with it. The relationship between energy, mass, and velocity can be quantified using various equations, such as the relativistic energy equation and the relativistic kinetic energy equation.

The principle of mass-energy equivalence has been extensively verified through numerous experiments, and it has far-reaching implications and applications in fields such as nuclear energy, particle physics, astrophysics, and technology. Understanding the mass-energy equivalence is crucial for our continued exploration and understanding of the physical world around us.

References:
1. Relativistic Energy | Physics – Lumen Learning, https://courses.lumenlearning.com/suny-physics/chapter/28-6-relativistic-energy/
2. How exactly does energy “become” mass? – Physics Forums, https://www.physicsforums.com/threads/how-exactly-does-energy-become-mass.284089/
3. Matter and Energy: A False Dichotomy – Of Particular Significance, https://profmattstrassler.com/articles-and-posts/particle-physics-basics/mass-energy-matter-etc/matter-and-energy-a-false-dichotomy/
4. Does energy have mass? – ResearchGate, https://www.researchgate.net/post/Does_energy_have_mass
5. Is Information Physical and Does It Have Mass? – MDPI, https://www.mdpi.com/2078-2489/13/11/540

Advantages of Biomass Energy: A Comprehensive Guide for Physics Students

June 24, 2024May 21, 2022 by Ankita Biswas

Biomass energy is a renewable and sustainable source of energy that has gained significant attention in recent years due to its numerous advantages. From reducing greenhouse gas emissions to providing economic benefits, biomass energy offers a compelling alternative to traditional fossil fuels. In this comprehensive guide, we will delve into the technical details and specific advantages of biomass energy, equipping physics students with a deep understanding of this versatile energy source.

Quantifiable Environmental Benefits

According to a report by the National Renewable Energy Laboratory (NREL), the easily quantifiable environmental benefits of biomass energy production in the United States are worth 7.6 cents per kilowatt-hour (kWh). This value covers the value of avoided greenhouse gas emissions, which is a significant contributor to the computed value of biomass energy benefits.

Greenhouse Gas Emission Reduction

The value of avoided greenhouse gas emissions is a crucial advantage of biomass energy. Assuming a zero value for greenhouse gases leaves a residual value for the other computed benefits of biomass energy production with the base-case data set of 4.0 ¢/kWh. However, estimating the value of avoided greenhouse gas emissions is complicated by the timeframe used to judge the delayed emissions of methane (CH4) and carbon dioxide (CO2) from landfills.

The base case includes all emissions released over a 20-year period following the use or burial of the biomass fuels. Using a longer-term time perspective, such as a 75-year timeframe, increases the calculated benefits with otherwise base-case assumptions to 14.1 ¢/kWh. This highlights the importance of considering the long-term impact of biomass energy on greenhouse gas emissions.

Waste Reduction

Biomass energy production also reduces the amount of waste sent to landfills, providing additional environmental benefits. According to the NREL report, using residues for energy production instead of open burning provides a benefit of 8.9 ¢/kWh, and residues diverted from landfill disposal provide a benefit of 14.9 ¢/kWh.

The reduction in waste sent to landfills is a significant advantage of biomass energy, as it helps to mitigate the environmental impact of landfill disposal, such as the release of methane and the potential contamination of soil and groundwater.

Economic and Social Benefits

In addition to the quantifiable environmental benefits, biomass energy production also provides less tangible but equally important economic and social benefits.

Energy Independence

Biomass energy can contribute to energy independence by reducing the reliance on imported fossil fuels. This can have far-reaching implications for a country’s energy security and economic stability, as it reduces the vulnerability to fluctuations in global energy prices and supply disruptions.

Rural Economic Development

Biomass energy production often involves the utilization of agricultural and forestry residues, which can provide economic opportunities for rural communities. This can lead to the creation of new jobs, the development of local industries, and the revitalization of rural economies.

Job Creation

The biomass energy industry is a significant employer, with the potential to create a wide range of jobs, from biomass feedstock production and collection to the construction and operation of biomass power plants. This job creation can have a positive impact on local communities and contribute to overall economic growth.

Technical Advantages

Biomass energy also offers several technical advantages that make it a compelling choice for energy production.

Renewable and Sustainable

Biomass energy is a renewable and sustainable energy source, as it is derived from organic matter that can be replenished over time. This contrasts with the finite nature of fossil fuels, making biomass energy a more reliable and long-term energy solution.

Versatile Feedstock

Biomass energy can be produced from a wide range of feedstocks, including agricultural residues, forestry waste, municipal solid waste, and dedicated energy crops. This versatility allows for the utilization of various waste streams and the optimization of biomass energy production based on local resource availability.

Dispatchable Power Generation

Unlike intermittent renewable energy sources like solar and wind, biomass power plants can provide dispatchable power generation, meaning they can be turned on and off as needed to meet fluctuating energy demands. This flexibility is a valuable asset in the energy grid, as it helps to ensure a reliable and stable power supply.

Efficient Energy Conversion

Biomass energy conversion technologies, such as combustion, gasification, and anaerobic digestion, have become increasingly efficient over time. Modern biomass power plants can achieve conversion efficiencies of up to 40%, making them a competitive option compared to other energy sources.

Waste-to-Energy Potential

Biomass energy production can also be integrated with waste management systems, allowing for the conversion of various waste streams, such as municipal solid waste and agricultural waste, into valuable energy. This waste-to-energy approach helps to reduce the environmental impact of waste disposal and provides an additional revenue stream for biomass energy producers.

Conclusion

Biomass energy offers a multitude of advantages, from quantifiable environmental benefits to economic and social impacts. By understanding the technical details and specific advantages of biomass energy, physics students can gain a comprehensive understanding of this renewable energy source and its role in the transition towards a sustainable energy future.

References

The Value of the Benefits of U.S. Biomass Power – NREL, https://www.nrel.gov/docs/fy00osti/27541.pdf
Energy from biomass: advantages and disadvantages of this alternative source – EDP, https://www.edp.com/en/edp-yes/energy-from-biomass-advantages-and-disadvantages-alternative-source
Biomass Advantages and Disadvantages – SynTech Bioenergy, https://www.syntechbioenergy.com/blog/biomass-advantages-disadvantages
Biomass Energy: Advantages and Disadvantages – EnergySage, https://www.energysage.com/about-clean-energy/biomass/pros-and-cons-biomass/
Biomass Power – an overview | ScienceDirect Topics, https://www.sciencedirect.com/topics/earth-and-planetary-sciences/biomass-power

21+ Biomass Energy Examples: Uses And Detailed Facts

January 21, 2024April 24, 2022 by AKSHITA MAPARI

Biomass energy is a renewable source of energy and is easily available from the waste remains of the flora and fauna.

Biomass has plenty of uses and benefits and can obtain a huge amount of energy for various purposes. Let us discuss some of the biomass energy examples as listed below:-

Biogas

It is a renewable source produced from the organic remnant of plants and animals.

The bacteria digest the waste organic matter in absence of oxygen in the digesters. It is a green source of energy and produces heat energy and electricity.

Photosynthesis

It is a process through which plant produces food and supplies oxygen to the atmosphere. The radiant energy received from the Sun is converted into chemical energy and stores in the form of food. The food thus produced by the plant is rich in chemical potential energy, minerals, and vitamin, essential for humans and animals.

Food

The food that we eat provides us with the chemical potential energy which is stored in the form of chemicals. This chemical potential is released as required during the activities. The chemical bonds break and the energy thus released is utilized by us while performing any task and daily activities.

Agriculture

Agriculture provides us with a variety of food grains to boost our energy and grass to the herbivorous animals.

The waste remains from agriculture can be used to produce manure which can improve the productivity of the crops and can also be used in the production of biogas.

Mushroom Plantation

The mushrooms are a rich source of protein, fiber, and antioxidants. There are different ways of plantation of varieties of mushrooms. Some use dry paddy straws whereas some required rich fertile soil with manure made out of flora.

Leguminous Plant

These are the nitrogen-fixing plant because the bacteria present on the roots of these plants inhale nitrogen gas thus increasing the nitrogen quantity essential for the growth of these plants. These bacteria present at the roots of leguminous plants are namely rhizobium. Some examples of leguminous plants include beans, clover, peas, peanuts, soybeans, etc.

Charcoal

The charcoal is produced upon burning the wood before it turns into ash.

This charcoal is a good cleanser and is used in some facial creams and toothpaste. In the olden days, charcoal was used to clean teeth.

Bio-methane

It is released during the decomposition process of organic matter. When the organic waste is exposed to the sunlight, the heat energy supplied to the matter breaks the chemical bond and for decomposition to take place. The breakdown of the substance releases bio-methane which is used for the production of electricity, supply heat energy, and as a fuel for the vehicle.

Seashells

The seashells are made up of calcium carbonate. These shells are dried and crushed into powder and used in many manufacturing industries.

seashell g2a805dd5b 640 — **Seashells; Image Credit: Pixabay**

This is used to make chalk, paints, building material, ceramic tiles, etc. It is also used in medicine as an antacid to get relief from heartburn, and acidity.

Wood as Fuel

Wood is used as a fuel in many industries and factories to generate heat and steam to drive the turbine and produce electrical energy and power. The plants utilized carbon dioxide from the air to produce their own food, and this is released back into the air when burnt.

Fossil Fuel

Fossil fuel is extracted from the deeply buried matter by the method of drilling or mining.

pumping gas g762de3d1d 640 — **CNG used in vehicles; Image Credit: Pixabay**

The coal is removed by the mining while crude oil can be extracted by drilling. Natural gas is also present around the crude oil deposition.

Coal

It is produced by the organic matter trapped beneath the ground for millions of years. Organic matter is mostly composed of substances made up of carbon bonding structure and hence as it remains buried under the ground for several years, the only element that remains is carbon and hence the coal is entirely made up of carbon. This is used as a fuel in industries and trains.

Liquid Petroleum Gas

It is used as a cooking fuel in the LPG cylinder. This gas is in the liquid form in the cylinder which is converted back into the gaseous form and escapes from the burner on removing the pressure by turning ON the knob.

appliance ga2eaf0e3f 640 — **Liquid petroleum gas; Image Credit: Pixabay**

This is a biofuel because it is formed by the organic matter trapped under the great pressure and heat beneath the landmass.

Compressed Natural Gas

Compressed natural gas is made up of petrol which is partly composed of methane. It is stored in the container maintaining a pressure range between 20-25 MPa. Petrol is extracted from the Earth through the process called drilling. This is used to fuel the vehicles and emits low carbon dioxide in the air.

Grass

The paddy grass and shrubs produce in the field are rich in starch. The cows can digest a more quantity of starch edibles. Hence the agricultural remnant is used as food for the herbivorous animal. This grass is dried and stored for the food of animals.

Cow Dung

Cow dung is a waste produced by the cows. It is used as manure and also to increase the fertility of the soil.

It is a renewable source of biomass and is converted into energy by feeding it into the digester in a biogas plant.

Manure

Manures are good to grow vegetables and in paddy fields. It is prepared from the organic waste of flora and fauna. The thick layer of sand is initially made on the bottom layer in a container and above it, alternate layers of waste and soil are set and the earthworms are released on the top of the mixture. The earthworms mix the components properly and the manure becomes ready within 3-4 months.

Sewage Water

The sewage water has a good concentration of nitrogen and phosphates and hence is used for irrigation and also in the aquaculture.

sewage treatment plant g9a4e4fac1 640 — **Sewage water treatment plant; Image Credit: Pixabay**

The organic matter and nutrients present in the sewage water fertilize the soil and can yield better production.

Alcohol

The alcohol is produced from different fruits and herbs. It is composed of hydrogen and oxygen atom bonded with a single carbon and it is also volatile and can easily convert into the gaseous form thus catching fire easily. Hence alcohol can be used to light the fire.

Coconut Shells

The remnant of the crushed coconut shells is used as a fuel to gain heat energy. The dry coconut shells are also used to design art crafts and decorative pieces. These are also used to produce biogas and to make manure.

Frequently Asked Questions

What is meant by biomass?

It is the organic matter which can be transformed to produce some sort of energy.

Biomass is a product of organic material and the waste remains from flora and fauna which can be used as a fuel to generate heat and electricity and transform the energy from one form to another.

What are the different sources of biomass?

Biomass is the matter produced by the living organism.

The main sources of biomass are agriculture, forest, animals, human beings, green waste, sewage, industrial waste, sea organisms, etc.

What is the process to obtain a biofuel?

The fuel produced by using organic matter is called biofuel and is completely a green fuel.

The biofuel is produced by the combustion of organic remains in anaerobic decomposition and the electrical energy is directly produced during this process. This is available in liquid as well as the gaseous form.

How biomass energy is beneficial?

It is a renewable source of energy generated by organic matter.

Biomass energy is a green source of energy and can reduce a large amount of harmful emissions of gases into the atmosphere, thus protecting the environment and its sustainability.

Also Read:

How to Find Conservation of Mass: A Comprehensive Guide for Physics Students

June 24, 2024April 23, 2022 by Deeksha Dinesh

The principle of conservation of mass is a fundamental concept in physics, stating that the total mass of an isolated system remains constant, regardless of any physical or chemical changes. This principle is based on the law of conservation of mass, which states that matter is neither created nor destroyed in a chemical reaction. Understanding and demonstrating the conservation of mass is crucial for physics students, as it underpins many important theories and phenomena in the field.

Understanding the Principle of Conservation of Mass

The principle of conservation of mass is a direct consequence of the first law of thermodynamics, which states that energy can be transformed, but not created or destroyed. In a closed system, the total mass of the system must remain constant, as matter cannot be created or destroyed. This principle can be expressed mathematically as:

m_initial = m_final

Where m_initial is the total mass of the system before a reaction or process, and m_final is the total mass of the system after the reaction or process.

This principle holds true for both physical and chemical changes. In a physical change, such as a phase transition, the total mass of the system remains the same, even though the physical properties of the substance may change. In a chemical reaction, the total mass of the reactants must equal the total mass of the products, as the atoms involved in the reaction are rearranged, but not created or destroyed.

Experimental Demonstration of Conservation of Mass

To demonstrate the conservation of mass experimentally, you can conduct a simple experiment using a closed system, such as a sealed plastic bag. The steps to set up the experiment are as follows:

Acquire an empty, sealable plastic bag: Choose a sturdy, leak-proof plastic bag that can be sealed tightly.
Weigh the bag and record the mass: Use a precise digital scale to measure the mass of the empty bag and record the value.
Add a set amount of baking soda to the bag: Measure a specific amount of baking soda (e.g., 5 grams) and add it to the bag.
Weigh the bag with the baking soda and record the mass: Measure the mass of the bag with the added baking soda and record the new value.
Repeat the process with a specific amount of vinegar: Measure a specific amount of vinegar (e.g., 10 milliliters) and add it to the bag.
Seal the bag, ensuring it’s leak-proof: Carefully seal the bag, making sure there are no air leaks.
Thoroughly mix the contents inside by shaking or squeezing the bag: Agitate the bag to ensure the baking soda and vinegar react completely.
Weigh the bag one last time and record the mass: After the reaction is complete, weigh the bag and record the final mass.

If the Law of Conservation of Mass holds, the bag and its contents should still weigh the same as before the reaction. This is because the total mass of the reactants (baking soda and vinegar) is equal to the total mass of the products (carbon dioxide, water, and any other byproducts).

Analyzing the Results

To analyze the results of the conservation of mass experiment, you need to answer the main question: Does the total mass before the reaction equal the total mass after the reaction?

Calculate the total mass before the reaction: Add the mass of the empty bag, the mass of the baking soda, and the mass of the vinegar.
Calculate the total mass after the reaction: Weigh the bag with the final reaction products.
Compare the total masses: If the total mass before the reaction is equal to the total mass after the reaction, within the limits of experimental error, then the experiment supports the Conservation of Mass.

If the total masses are not equal, there may be an error in the experiment. Potential sources of error include:

Leaks in the bag: If the bag is not properly sealed, gases or other products may escape, leading to a loss of mass.
Inaccurate or improperly calibrated scales: Ensure that the scales used to measure the masses are accurate and properly calibrated.
External factors: Changes in temperature, humidity, or other environmental conditions may affect the results.

To ensure accurate results, it’s important to carefully control the experimental conditions and consider potential sources of error.

Theoretical Basis for Conservation of Mass

The conservation of mass is a fundamental principle in physics, and it is closely related to the concept of the conservation of energy. The theoretical basis for the conservation of mass can be found in the work of several renowned physicists, including:

Antoine Lavoisier: Lavoisier, often referred to as the “father of modern chemistry,” conducted experiments that demonstrated the conservation of mass in chemical reactions. He formulated the law of conservation of mass, which states that the total mass of the reactants in a chemical reaction is equal to the total mass of the products.
Albert Einstein: Einstein’s famous equation, E = mc^2, establishes the equivalence between mass and energy. This equation suggests that mass and energy are interchangeable, and that the total mass-energy of an isolated system remains constant.
Emmy Noether: Noether’s theorem, a fundamental result in theoretical physics, states that the conservation of mass is a direct consequence of the translational symmetry of space-time. This means that the laws of physics are the same regardless of the location in space or time, which leads to the conservation of mass.

These theoretical foundations, combined with experimental evidence, provide a robust understanding of the conservation of mass and its importance in the study of physics.

Practical Applications of Conservation of Mass

The principle of conservation of mass has numerous practical applications in various fields of science and engineering, including:

Chemical Reactions: The conservation of mass is essential in understanding and predicting the outcomes of chemical reactions. By applying the principle, chemists can balance chemical equations and determine the quantities of reactants and products.
Environmental Science: The conservation of mass is crucial in understanding and modeling environmental processes, such as the cycling of materials in ecosystems, the transport of pollutants, and the global carbon cycle.
Aerospace Engineering: The conservation of mass is a fundamental principle in the design and analysis of aerospace systems, such as rockets and spacecraft, where the mass of the vehicle and its propellant must be carefully managed.
Forensic Science: The conservation of mass can be used in forensic investigations to analyze the composition and origin of materials, such as explosives or gunpowder residues.
Nutrition and Metabolism: The conservation of mass is a key concept in understanding the relationship between food intake, energy expenditure, and body weight changes in living organisms.

By understanding and applying the principle of conservation of mass, physics students can develop a deeper understanding of the fundamental laws of nature and their practical implications across various scientific and engineering disciplines.

Numerical Examples and Problems

To further solidify your understanding of the conservation of mass, let’s explore some numerical examples and problems:

Example 1: A sealed container with an initial mass of 100 grams contains 50 grams of baking soda and 25 grams of vinegar. After the reaction, the final mass of the container is measured to be 120 grams. Determine if the Law of Conservation of Mass is upheld in this experiment.

Solution:
– Initial mass = 100 grams
– Mass of baking soda = 50 grams
– Mass of vinegar = 25 grams
– Total mass before reaction = 100 + 50 + 25 = 175 grams
– Final mass after reaction = 120 grams
– Since the final mass (120 grams) is not equal to the initial mass (175 grams), the Law of Conservation of Mass is not upheld in this experiment. There is a discrepancy of 55 grams, which suggests a potential source of error in the experiment.

Problem 1: A sealed plastic bag with an initial mass of 10 grams contains 3 grams of sodium bicarbonate (baking soda) and 5 grams of acetic acid (vinegar). After the reaction, the final mass of the bag is measured to be 15 grams. Determine if the Law of Conservation of Mass is upheld in this experiment.

Solution:
– Initial mass of the bag = 10 grams
– Mass of sodium bicarbonate = 3 grams
– Mass of acetic acid = 5 grams
– Total mass before reaction = 10 + 3 + 5 = 18 grams
– Final mass after reaction = 15 grams
– Since the final mass (15 grams) is not equal to the initial mass (18 grams), the Law of Conservation of Mass is not upheld in this experiment. There is a discrepancy of 3 grams, which suggests a potential source of error in the experiment.

Example 2: A sealed container with an initial mass of 50 grams contains 20 grams of sodium hydroxide (NaOH) and 15 grams of hydrochloric acid (HCl). After the reaction, the final mass of the container is measured to be 52 grams. Determine if the Law of Conservation of Mass is upheld in this experiment.

Solution:
– Initial mass of the container = 50 grams
– Mass of sodium hydroxide = 20 grams
– Mass of hydrochloric acid = 15 grams
– Total mass before reaction = 50 + 20 + 15 = 85 grams
– Final mass after reaction = 52 grams
– Since the final mass (52 grams) is not equal to the initial mass (85 grams), the Law of Conservation of Mass is not upheld in this experiment. There is a discrepancy of 33 grams, which suggests a potential source of error in the experiment.

These examples and problems demonstrate the importance of carefully measuring and accounting for the masses of all the components involved in a chemical reaction to verify the conservation of mass. By working through these exercises, physics students can develop a deeper understanding of the practical application of this fundamental principle.

Conclusion

The principle of conservation of mass is a cornerstone of physics, with far-reaching implications across various scientific and engineering disciplines. By understanding and demonstrating the conservation of mass through carefully designed experiments, physics students can gain valuable insights into the fundamental laws of nature and their practical applications.

This comprehensive guide has provided you with the necessary knowledge and tools to find conservation of mass, including the theoretical basis, experimental demonstration, and analysis of results. By applying the principles and techniques outlined in this guide, you can deepen your understanding of the conservation of mass and become a more proficient physics student.

Remember, the key to success in finding conservation of mass lies in your ability to carefully control the experimental conditions, accurately measure the masses involved, and critically analyze the results. With practice and a solid grasp of the underlying principles, you will be well on your way to mastering this essential concept in physics.

References:

Lavoisier, A. (1789). Traité Élémentaire de Chimie. Paris: Chez Cuchet.
Einstein, A. (1905). “Ist die Trägheit eines Körpers von seinem Energieinhalt abhängig?” Annalen der Physik, 323(13), 639-641.
Noether, E. (1918). “Invariante Variationsprobleme.” Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse, 1918, 235-257.
Atkins, P., & de Paula, J. (2014). Atkins’ Physical Chemistry (10th ed.). Oxford University Press.
Serway, R. A., & Jewett, J. W. (2018). Physics for Scientists and Engineers with Modern Physics (10th ed.). Cengage Learning.

Can the Conservation of Mass Change?

June 25, 2024April 16, 2022 by Deeksha Dinesh

The conservation of mass is a fundamental principle in physics and chemistry, which states that the total mass of a closed system remains constant, regardless of the physical or chemical changes that occur within the system. This principle is a direct consequence of the law of energy conservation and the principle of mass-energy equivalence, as stated by Einstein’s famous equation, E=mc^2.

Theorem of Conservation of Mass

The theorem of conservation of mass can be stated as follows:

In a closed system, the total mass remains constant, regardless of the physical or chemical changes that occur within the system.

Physics Formula for Conservation of Mass

The principle of conservation of mass can be expressed mathematically as:

Δm = 0

where Δm is the change in mass of the system.

Physics Examples of Conservation of Mass

Burning a Candle: When a candle burns, the wax is converted into carbon dioxide and water vapor. Despite the apparent disappearance of the wax, the total mass of the candle and the products of combustion remains constant.
Dissolving a Sugar Cube in Water: When a sugar cube is dissolved in water, the sugar molecules become evenly distributed throughout the water. Although the sugar appears to have disappeared, the total mass of the sugar and water remains constant.
Chemical Reactions: In a chemical reaction, the total mass of the reactants must be equal to the total mass of the products, regardless of their physical state. This can be demonstrated through experiments, where the mass of the reactants and products is measured before and after the reaction. The results of these experiments consistently show that the total mass remains constant, supporting the conservation of mass principle.

Physics Numerical Problems on Conservation of Mass

Consider the following numerical problem to apply the conservation of mass principle:

A chemist mixes 50.0 g of sodium carbonate (Na2CO3) with 50.0 g of calcium nitrate (Ca(NO3)2) in a closed container. After the reaction is complete, the chemist measures the mass of the container and finds that it is 99.9 g. What is the mass of the products formed in the reaction?

Solution:
According to the principle of conservation of mass, the total mass of the reactants must be equal to the total mass of the products. Therefore, the mass of the products is equal to the mass of the reactants, which is 50.0 g + 50.0 g = 100.0 g. However, the chemist measured a total mass of 99.9 g, which is less than the expected mass. This discrepancy can be attributed to experimental error, such as the inaccuracy of the balance or the loss of some material during the reaction.

Figures, Data Points, Values, and Measurements

The following figures, data points, values, and measurements can be used to illustrate the conservation of mass principle:

Figure 1: A graph showing the mass of a sample of reactants and products over time, with the total mass remaining constant.

Data Point 1: The mass of a sample of reactants before a chemical reaction is 100.0 g.
Data Point 2: The mass of the same sample of reactants after the chemical reaction is 100.0 g.
Data Point 3: The mass of the products formed in the reaction is 100.0 g.

Value 1: The principle of conservation of mass states that the total mass of a closed system remains constant.
Measurement 1: The mass of a sample of reactants and products can be measured using a balance or scale.

Exceptions to the Conservation of Mass

It is important to note that the conservation of mass only applies to closed systems, where no mass enters or leaves the system. In an open system, mass can be exchanged with the surroundings, and the total mass of the system can change.

For example, in a chemical reaction that involves the release of a gas, the total mass of the system may decrease as the gas escapes the system. Similarly, in a nuclear reaction, the mass of the products may be slightly different from the mass of the reactants due to the conversion of mass into energy, as described by Einstein’s equation, E=mc^2.

Conclusion

The conservation of mass is a well-established principle in physics and chemistry, supported by numerous experimental observations and theoretical considerations. It is a fundamental concept that underlies our understanding of chemical reactions and the behavior of matter. However, it is important to recognize the limitations of this principle and the exceptions that can occur in open systems or in the context of nuclear reactions.

Reference:

How To Calculate Friction Force Without Mass: Detailed Explanations and Problem Examples

January 21, 2024March 29, 2022 by AKSHITA MAPARI

How to Calculate Friction Force Without Mass

Friction is a force that opposes the motion of an object when it comes into contact with a surface. It plays a crucial role in physics and everyday life. Understanding how to calculate friction force without mass is essential for solving various problems in physics and engineering. In this blog post, we will explore different methods to calculate friction force without mass, accompanied by relevant examples and formulas.

Understanding the Basic Concepts of Friction

Before diving into the calculation methods, let’s briefly review the basic concepts of friction. There are two types of friction: static friction and kinetic friction.

Static friction occurs when two surfaces are at rest relative to each other. It prevents objects from sliding against each other until a certain force, called the threshold force, is applied. Once the threshold force is exceeded, the object starts moving, and kinetic friction comes into play.

Kinetic friction, also known as sliding friction, acts on objects that are already in motion. It opposes the motion of an object sliding across a surface. The strength of the friction force depends on the nature of the surfaces in contact and the normal force acting between them.

Importance of Friction in Physics and Everyday Life

Friction plays a significant role in various aspects of our lives. From walking on the ground to driving a car, friction allows us to move and control objects. In physics, friction is crucial for understanding the laws of motion, calculating forces, and predicting the behavior of objects in different situations.

Friction is also essential for practical applications. It helps vehicles stop by providing necessary braking force, enables us to write with a pen on paper, and allows machines to grip and manipulate objects. Without friction, life as we know it would be very different.

Methods to Calculate Friction Force Without Mass

Using the Coefficient of Friction and Normal Force

One common method to calculate friction force without mass is by utilizing the coefficient of friction and the normal force. The coefficient of friction, denoted as μ, represents the frictional characteristics of the surfaces in contact. It is a dimensionless quantity that varies depending on the materials involved.

To calculate friction force using the coefficient of friction and normal force, we can use the formula:

$F_{\text{friction}} = \mu \times F_{\text{normal}}$

where:
– $F_{\text{friction}}$ is the friction force
– $\mu$ is the coefficient of friction
– $F_{\text{normal}}$ is the normal force

By substituting the known values for the coefficient of friction and the normal force into the equation, we can determine the magnitude of the frictional force.

Utilizing Velocity and Acceleration

Another method to calculate friction force without mass involves utilizing the concepts of velocity and acceleration. This method is particularly useful when dealing with moving objects.

When an object is in motion, the friction force can be determined using Newton’s second law of motion, which states that the net force acting on an object is equal to the product of its mass and acceleration. In this case, the net force is the force of friction.

The formula to calculate friction force using velocity and acceleration is:

$F_{\text{friction}} = m \times a$

where:
– $F_{\text{friction}}$ is the friction force
– $m$ is the mass of the object
– $a$ is the acceleration of the object

By substituting the known values for mass and acceleration into the equation, we can determine the frictional force acting on the moving object.

Considering the Angle and Distance

In certain situations, it may be necessary to calculate the friction force without knowing the coefficient of friction or the mass of the object. One way to do this is by considering the angle and distance involved.

For example, if an object is sliding down a ramp inclined at a certain angle, the friction force can be calculated using the following formula:

$F_{\text{friction}} = m \times g \times \sin(\theta)$

where:
– $F_{\text{friction}}$ is the friction force
– $m$ is the mass of the object
– $g$ is the acceleration due to gravity (approximately 9.8 m/s^2)
– $\theta$ is the angle of the incline

By substituting the known values for mass and the angle of the incline into the equation, we can determine the frictional force.

Worked Out Examples

To solidify our understanding, let’s work through a few examples to calculate friction force without mass using the methods we discussed.

Calculating Friction Force with Given Coefficient and Normal Force

Example: A box with a coefficient of friction of 0.5 is pushed horizontally with a normal force of 50 N. Calculate the friction force acting on the box.

Solution:
Using the formula $F_{\text{friction}} = \mu \times F_{\text{normal}}$ , we can substitute the given values:
$F_{\text{friction}} = 0.5 \times 50 = 25 \, \text{N}$

Therefore, the friction force acting on the box is 25 N.

Determining Friction Force with Known Velocity and Acceleration

Example: A car with a mass of 1000 kg accelerates at a rate of 5 m/s^2. Calculate the friction force acting on the car.

Solution:
Using the formula $F_{\text{friction}} = m \times a$ , we can substitute the given values:
$F_{\text{friction}} = 1000 \times 5 = 5000 \, \text{N}$

Therefore, the friction force acting on the car is 5000 N.

Finding Friction Force with Provided Angle and Distance

Example: A bicycle with a mass of 20 kg slides down a ramp inclined at an angle of 30 degrees. Calculate the friction force acting on the bicycle.

Solution:
Using the formula $F_{\text{friction}} = m \times g \times \sin(\theta$ ), we can substitute the given values:
$F_{\text{friction}} = 20 \times 9.8 \times \sin(30) = 98 \, \text{N}$

Therefore, the friction force acting on the bicycle is 98 N.

Frequently Asked Questions About Calculating Friction Force Without Mass

How to Calculate Total Friction Force?

To calculate the total friction force acting on an object, you need to consider the magnitude of both static and kinetic friction, depending on the situation. The total friction force can be determined by using the appropriate formula based on the given conditions.

How to Calculate Friction Force with Weight?

The weight of an object is typically related to the normal force acting on it. To calculate the friction force with weight, you can use the formula $F_{\text{friction}} = \mu \times F_{\text{normal}}$ , where the normal force is equal to the weight of the object.

How to Calculate Coefficient of Friction Without Mass?

To calculate the coefficient of friction without mass, experimental methods are usually employed. By measuring the force required to move an object against a surface at different angles or under different conditions, the coefficient of friction can be determined.

By understanding the methods to calculate friction force without mass and practicing with different examples, you will become proficient in solving friction-related problems in physics and engineering. Remember to consider the specific conditions and variables involved, and utilize the appropriate formulas to arrive at accurate results.

Keep exploring the fascinating world of friction and its applications, as it is an essential concept that governs the behavior of objects in our everyday lives.

Numerical Problems on how to calculate friction force without mass

Problem 1:

A box is being pushed with a force of 50 N along a horizontal surface. The coefficient of friction between the box and the surface is 0.3. Calculate the friction force acting on the box.

Solution:

Given:
Force applied, F = 50 N
Coefficient of friction, μ = 0.3

The friction force can be calculated using the formula:

$F_{\text{friction}} = \text{Coefficient of friction} \times \text{Normal force}$

As the box is pushed horizontally on a horizontal surface, the normal force is equal to the weight of the box. Therefore,

$\text{Normal force} = \text{Weight} = m \times g$

Since the mass of the box is not provided in the problem, we cannot calculate the normal force or the friction force without knowing the mass.

Problem 2:

A car is moving with a constant speed of 20 m/s on a level road. The coefficient of friction between the tires of the car and the road is 0.4. Calculate the friction force acting on the car.

Solution:

Given:
Speed of the car, v = 20 m/s
Coefficient of friction, μ = 0.4

When the car is moving with a constant speed, the friction force acting on the car is equal to the force required to overcome the friction. This force can be calculated using the formula:

$F_{\text{friction}} = \text{Coefficient of friction} \times \text{Normal force}$

As the car is on a level road, the normal force is equal to the weight of the car. Therefore,

$\text{Normal force} = \text{Weight} = m \times g$

Since the mass of the car is not provided in the problem, we cannot calculate the normal force or the friction force without knowing the mass.

Problem 3:

A block is placed on an inclined plane with an angle of inclination of 30 degrees. The coefficient of friction between the block and the plane is 0.2. Calculate the friction force acting on the block.

Solution:

Given:
Angle of inclination, θ = 30 degrees
Coefficient of friction, μ = 0.2

The friction force can be calculated using the formula:

$F_{\text{friction}} = \text{Coefficient of friction} \times \text{Normal force}$

To calculate the normal force, we need to decompose the weight of the block into components parallel and perpendicular to the inclined plane. The perpendicular component is equal to the weight of the block, while the parallel component is equal to the weight multiplied by the sine of the angle of inclination.

$\text{Normal force} = \text{Weight} \cos(\theta)$

Therefore,

$F_{\text{friction}} = \text{Coefficient of friction} \times \text{Weight} \cos(\theta)$

Since the weight of the block is not provided in the problem, we cannot calculate the normal force or the friction force without knowing the weight.

Also Read:

How to Find Potential Energy Without Mass: A Comprehensive Guide

June 25, 2024March 24, 2022 by Core SME lambdageeks.com

Potential energy is a fundamental concept in physics, describing the energy stored within a system due to its position or configuration. While the traditional approach to calculating potential energy involves the mass of the object, it is possible to determine potential energy without explicitly considering the mass. This comprehensive guide will delve into the principles, … Read more

Is Mass an Extensive Property?

June 25, 2024March 22, 2022 by Abhishek

Mass is a fundamental physical quantity that is widely recognized as an extensive property. Extensive properties are those that depend on the amount of matter or substance present in a system, and mass is a direct measure of the quantity of matter. In this comprehensive blog post, we will delve into the intricacies of mass as an extensive property, exploring its characteristics, applications, and the underlying principles that govern its behavior.

Understanding Extensive Properties

Extensive properties are physical quantities that scale with the size or amount of a system. In other words, the value of an extensive property is directly proportional to the quantity of matter or substance present. Some common examples of extensive properties include:

Mass
Volume
Energy
Charge
Amount of substance (moles)

Extensive properties are in contrast to intensive properties, which do not depend on the size or amount of a system. Intensive properties, such as temperature, pressure, and density, remain constant regardless of the quantity of matter present.

Mass as an Extensive Property

Mass is a fundamental extensive property that quantifies the amount of matter in an object or system. It is a measure of the inertia of an object, which is the resistance to changes in its motion. The mass of an object or system is directly proportional to the amount of matter it contains.

Characteristics of Mass as an Extensive Property

Additive Nature: The mass of a system is the sum of the masses of its individual components. If you combine two objects with masses m1 and m2, the total mass of the combined system is m1 + m2.
Proportionality: The mass of an object or system is proportional to the amount of matter it contains. If you double the amount of matter in a system, the mass of the system will also double.
Invariance: Mass is an invariant property, meaning it does not change with the location or state of the object. The mass of an object remains the same regardless of its position, temperature, or other physical conditions.

Measuring Mass

Mass is typically measured using a balance or scale, which compares the gravitational force acting on the object to the force acting on a known standard mass. The SI unit of mass is the kilogram (kg), and common smaller units include the gram (g) and the milligram (mg).

Relationship with Other Extensive Properties

Mass is closely related to other extensive properties, such as volume and weight. The volume of an object is the amount of space it occupies, and it is also an extensive property. The weight of an object is the force exerted on it by gravity, which is proportional to its mass and the acceleration due to gravity.

The relationship between mass, volume, and density (an intensive property) can be expressed as:

Density = Mass / Volume

This equation shows that mass and volume are both extensive properties, while density is an intensive property that does not depend on the amount of matter present.

Applications of Mass as an Extensive Property

The extensive nature of mass has numerous applications in various fields of science and engineering. Some of the key applications include:

Measurement and Quantification: Mass is used to measure and quantify the amount of matter in objects, substances, and systems. This is essential for various scientific and industrial processes, such as chemical reactions, material processing, and product manufacturing.
Conservation of Mass: The principle of conservation of mass states that the total mass of a closed system remains constant during any physical or chemical process. This principle is fundamental to many laws and theories in physics and chemistry.
Density Calculations: As mentioned earlier, the relationship between mass and volume can be used to calculate the density of a substance, which is an important property in fields like materials science, engineering, and geology.
Gravitational Interactions: The mass of an object determines its gravitational attraction to other objects, which is crucial in understanding celestial mechanics, orbital dynamics, and the behavior of massive objects in the universe.
Inertia and Momentum: Mass is a measure of an object’s inertia, which is its resistance to changes in motion. This property is essential in understanding the dynamics of moving objects, such as in mechanics, engineering, and transportation.
Atomic and Subatomic Particles: In the realm of particle physics, the mass of subatomic particles, such as protons, neutrons, and electrons, is a fundamental property that governs their behavior and interactions.

Numerical Examples and Calculations

To further illustrate the extensive nature of mass, let’s consider some numerical examples and calculations:

Mass of a Substance: Suppose you have a sample of pure copper with a mass of 50 grams. If you double the amount of copper, the mass of the new sample will be 100 grams.
Mass and Volume Relationship: A cube-shaped block of aluminum has a mass of 500 grams and a volume of 200 cubic centimeters. The density of the aluminum can be calculated as:
Density = Mass / Volume Density = 500 g / 200 cm^3 = 2.5 g/cm^3
If the volume of the block is doubled, the mass will also double to 1000 grams, while the density remains the same.
Mass and Weight Relationship: The weight of an object is the force exerted on it by gravity, which is proportional to its mass. If an object has a mass of 10 kilograms and the acceleration due to gravity is 9.8 m/s^2, the weight of the object can be calculated as:
Weight = Mass × Acceleration due to gravity Weight = 10 kg × 9.8 m/s^2 = 98 N
If the mass of the object is doubled, its weight will also double to 196 N.

These examples demonstrate how the extensive nature of mass allows for the calculation and prediction of various physical properties and relationships.

Conclusion

In summary, mass is an extensive property that depends on the amount of matter present in a system. The mass of an object or substance is directly proportional to the quantity of matter it contains, and this property has numerous applications in various fields of science and engineering. Understanding the characteristics and behavior of mass as an extensive property is crucial for understanding and analyzing a wide range of physical phenomena.

References:

Serway, R. A., & Jewett, J. W. (2018). Physics for Scientists and Engineers with Modern Physics (10th ed.). Cengage Learning.
Halliday, D., Resnick, R., & Walker, J. (2013). Fundamentals of Physics (10th ed.). Wiley.
Tipler, P. A., & Mosca, G. (2008). Physics for Scientists and Engineers (6th ed.). W. H. Freeman.
Young, H. D., & Freedman, R. A. (2016). University Physics with Modern Physics (14th ed.). Pearson.

How to Find Power with Mass, Distance, and Time: A Comprehensive Guide

June 25, 2024March 18, 2022 by Core SME lambdageeks.com

In the realm of physics, understanding the relationship between mass, distance, and time is crucial for calculating power, a fundamental concept that underpins many physical phenomena. This comprehensive guide will delve into the formulas, examples, and theoretical explanations to help you master the art of finding power using these key variables. Work (W) The first … Read more