Star Delta Connection: 5 Important Factors Related To It

Image Credit – Pravin Mishra, Milky Way Galaxy As Seen From Amphulaptsa Base Camp, CC BY-SA 4.0

Points of Discussion

• Introduction to Star & Delta Connection
• Star Connection
  • The relation between Phase Voltage and Link voltage of Star Connection
  • The relation between Phase Current and Link Current of Star Connection
• Delta Connection
  • The relation between phase voltage and line voltage of Delta connection
  • The relation between phase current and line current of Delta connection
• Difference Between Star and Delta Connections
• Conversion from star to delta and delta to star

Star delta connection | Star delta transformation

Introduction to Star Connection and Delta Connection

Star and delta connections are the two very well-known methods for establishing a three-phase system. They are an essential and widely used system. This article will discuss the basics of both star and delta connections and relations between phase and link voltage and current within the system. We will also find out the significant differences between star and delta connection.

Star Connection

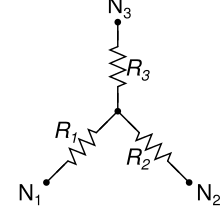
Star connection is the method where the similar types of terminals (all three windings) are connected to a single point, known as star point or neutral point. There are also line conductors, which are the free three terminals. The designing of wires at the external circuits makes it a three phase, three wire circuit and makes the star connection. There may be another wire named a neutral wire that makes the system a three phase, four-wire system.

What is meant by Thevenin’s theorem? Click Here!

The relation between Phase Voltage and Link Voltage of Star Connection

The system is considered as a balanced system. For a balanced-systems, an equal amount of current will pass through all 3-phase. That is why, R, Y, B has the same value of current. Now it has consequences. This uniform distribution of current makes the magnitudes of the voltages – E_NR, E_NY, E_NB same and they get displaced by 120 degrees from one another. 

In the above images, the arrow represents the direction of currents and voltages (not the actual order though). As we have discussed earlier, due to the uniform current distribution, the three arms’ voltage is equal so that we can write –

E_NR = E_NY = E_NB = _Eph.

And we can observe that the voltages in-between two lines is a two-phase voltage.

So, observing the NRYN loop, we can write that,

E_NR` + E<sub>RY</sub>` – E_NY` = 0

Or, E_RY` = E<sub>NY</sub>` – E_NR`

Now, from vector algebra,

E_RY = √ (E_NY² + E_NR² + 2 * E_NY * E_NR Cos60^o)

Or, E_L = √ (E_ph² + E_ph² + 2 * E_ph * E_ph x 0.5)

Or, E_L = √ (3E_ph²)

Or, E_l = √3 E_ph

In the same way, we can write, E_YB = E_NB – E_NY.

OR, E_L = √3 E_ph

And,

E_BR = E_NR – E_NB

Or, El = √3 Eph

So, we can say that the relation between the line voltage and phase voltage is:

Line Voltage = √3 x Phase voltage

What is Millman’s Theorem? Click Here!

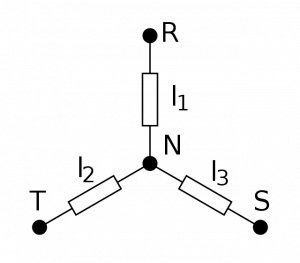
Relation Between Phase Current and Line Current in Star Connection

The uniform current flow in phase windings is the similar as the current flow in the line conductor.

We can write –

I_R = I_NR

I_Y = I_NY

And I_B = I_NB

Now, the phase current will be –

I_NR = I_NY = I_NB = I_ph

And the line current will be – I_R = I_Y = I_B = I_L

So, we can say that, I_R = I_Y = I_B = I_L

What is Maximum Power Transfer Theorem? Click Here!

Delta connection

Delta connection is another method to establish three phases of an electrical system. The end terminal of the windings is attached to the starting of the other terminals. Three-line conductors are connected from three junctions. The delta connection is set up by tying the ends. For that we combine a₂ with b₁, b₂ with c₁ and c₂ with a₁. Line conductors are the R, Y, B which run from three junctions. The below image depicts a typical delta connection and shows the end-to-end connections.

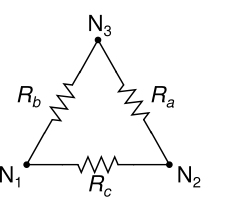
The relation between phase voltage and the line voltage of the Delta connection

Let us find out the relation between phase voltage of a delta circuit with the circuit’s line voltage. For that, observe the above image carefully. We can say that the value of the voltage at both the terminal 1 and terminal 2 is the same as the terminal R and terminal Y.

So, we can write – E₁₂ = E_RY.

In the same way, we can conclude by observing the circuit, E₂₃ = E_YE.

And E₃₁ = E_BR

The phase voltages are written as: E₁₂ = E₂₃ = E₃₁ = E_ph

The line voltages are written as: E_RY = E_YB = E_BR = E_L.

So, we can conclude that, in case of a delta connection, the phase voltage will be equal to the circuit’s line voltage.

To know About Kirchhoff’s Laws: Click Here!

The relation between phase current and line current in delta connection

For a balanced delta connection, the constant voltage value affects the current values. The current values of I₁₂, I₂₃, I₃₁ are equal, but they are displaced by 120 degrees from one another. Observe the below-given phasor diagram.

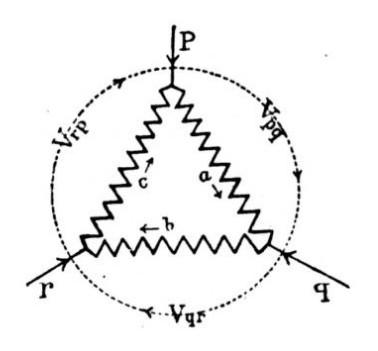
We can write, I₁₂ = I₂₃ = I₃₁ = I_ph

Now, by applying Kirchhoff’s law at junction 1,

We know that the algebraic sum of the current of a node is zero.

So, I₃₁` = I<sub>R</sub>` + I₁₂`

The vectoral differences come as I_R` = I<sub>31</sub>` – I₁₂`

By applying vector algebra,

I_R = √ (I₃₁² + I₁₂² + 2 * I₃₁ * I₁₂ * Cos 60^o)

Or, I_R = √ (I_ph² + I_ph² + 2 * I_ph * I_ph x 0.5)

As, we have discussed earlier, I_R = I_L.

Or, I_L = √ (3I_ph²)

Or, I_L = √3 * I_ph

In the same way, I_Y` = I<sub>12</sub>` – I₂₃.`

Or, I_L = √ 3 * I_ph

And, I_B` = I<sub>23</sub>` – I₃₁`

Or, I_L = √ 3 I_ph

So, the relation between line current and phase current can be written as:

Line Current = √3 x Phase Current

Difference between Star and Delta Connection

Star and delta methods are two renowned methods for three phase systems. Depending on various factors, there are some fundamental differences between them. Let us discuss some of them.

POINTS OF COMPARISION	STAR CONNECTION	DELTA CONNECTION
Definition	The three terminals are allied at a common point. This type of circuit is called a Star connection.	Three end terminals of the circuits are connected with each other to form a closed loop known as delta connection.
Neutral Point	There is a neutral point in star connection.	No such neutral point exists in delta connection.
The relation between phase and line voltage	Line voltage is calculated as √three times of phase voltage for star connection.	Phase voltage and line voltages are equal to each other for delta connections.
The relation between phase current and line current	Phase current and line current for star connection is equal to each other.	Line current is √three times of phase current for delta connections.
Speed as starters	Star connected motors are usually slower as they get 1/√3 rd of the voltage.	Delta connected motors are usually faster as they get the full line voltage.
Phase Voltage	The value of phase voltage for a star connection is lower as they get just 1/√3 part of the line voltage.	The value of phase voltage is higher as phase voltage, and line voltages are equal.
Requirement of Insulation	Low level of insulation required for a star connection.	High level of insulation is required for delta connection.
Usage	Power transmission networks use a star connection.	Power distribution system uses a delta connection.
The number of turns required.	Star connection requires a lesser number of turns.	Delta connection requires a higher number of turns.
Received voltage	Every single winding receives 230 volts of voltage in star connection.	In delta connection, every single winding receives 414 volts of voltage.
Available systems	Star connection of three wire three phases and four wire three phase systems are available.	Delta connection of three wire three phase systems, and four-wire three phase systems are available.

Learn About Basics of AC Circuit: Click Here!

Star delta transformation

Conversion from Star to Delta and Delta to Star

A star network can be converted into a delta network, and a delta connected network can be converted into a star network if needed. Conversion of circuits is necessary to simplify the complicated course, and thus the calculation becomes more effortless.

Conversion from Star to Delta

In this conversion, a connected star network is replaced by its equivalent delta connected network. The star and replaced delta figure are given. Observe the equations.

The value of Z₁, Z₂, Z₃ is given in terms of Z_A, Z_B, Z_C.

Z₁ = (Z_A Z_B + Z_B Z_C + Z_C Z_A) / Z_C = Σ (Z_A Z_B) / Z_C

Z₂ = (Z_A Z_B + Z_B Z_C + Z_C Z_A) / Z_B = Σ (Z_A Z_B) / Z_B

Z₃ = (Z_A Z_B + Z_B Z_C + Z_C Z_A) / Z_A = Σ (Z_A Z_B) / Z_A

We can easily convert a connected star network into a delta connected if we know the star-connected network’s value.

Learn About Advanced AC Circuit: Click Here!

Conversion from Delta to star

In this conversion, a delta connected network is replaced by its equivalent star connected network. The delta and replaced star figure are given. Observe the equations.

The value of Z_A, Z_B, Z_C is given in terms of Z₁, Z₂, Z_3.

Z_A = (Z₁ Z₂) / (Z₁ + Z₂ + Z₃)

Z_B = (Z₂ Z₃) / (Z₁ + Z₂ + Z₃)

Z_C = (Z₁ Z₃) / (Z₁ + Z₂ + Z₃)

We can easily convert a delta connected network into a star connected if we know the value of the delta connected network.

Cover GIF by: GIPHY