Definition Polytropic process
“A polytropic process is a thermodynamic process that obeys the relation: PVn = C, where where p is the pressure, V is volume, n is the polytropic index, and C is a constant. The polytropic process equation can describe multiple expansion and compression processes which include heat transfer.”
Polytropic Equation | Polytropic equation of state
The polytropic process can be defined by the equation
![]()
the exponent n is called polytropic index. It depends upon the material and varies from 1.0 to 1.4. This is constant specific heat procedure, in which heat absorption of gas taken into consideration because of unit rise in temperature is fixed.

Polytropic index
Some important relations between Pressure [P], Volume [V] and temperature [T] in Polytropic process for an Ideal Gas
Polytropic equation is,
![]()
![Rendered by QuickLaTeX.com \\\\P_1V_1^n=P_2V_2^n\\\\ \\\\\\frac{P_2}{P_1}=[\\frac{V_1}{V_2}]^n](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-2f79f958a45d3de1b856d5a94f13b960_l3.png)
………………………. Relations between Pressure [P] and Volume [V]
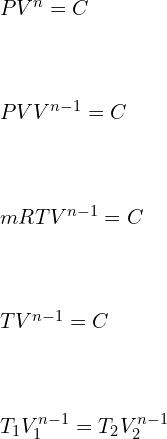
![]()
………………………. Relations between Volume [V] and Temperature [T]
![]()
………………………. Relations between Pressure [P] and Temperature [T]
Polytropic Work
Ideal gas equation for polytropic process is given by
![Rendered by QuickLaTeX.com \\\\W=\\int_{1}^{2}Pdv\\\\ \\\\W=\\int_{1}^{2}\\frac{C}{V^n}dv\\\\ \\\\W=C[\\frac{V^{-n+1}}{-n+1}]^2_1\\\\ \\\\W=\\frac{P_1V_1V_1^{-n+1}-P_2V_2V_2^{-n+1}}{n-1}\\\\ \\\\W=\\frac{P_1V_1-P_2V_2}{n-1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-34673bf8e32b5351c5b578248dee5bc8_l3.png)
Polytropic Heat transfer
According to 1st law of thermodynamics,
dQ=dU+W
![Rendered by QuickLaTeX.com \\\\dQ=mC_v [T_2-T_1 ]+\\frac{P_1 V_1-P_2 V_2}{n-1}\\\\ \\\\dQ=\\frac{mR}{\\gamma -1} [T_2-T_1 ]+\\frac{P_1 V_1-P_2 V_2}{n-1}\\\\ \\\\dQ=\\frac{P_1 V_1-P_2 V_2}{\\gamma-1}+\\frac{P_1 V_1-P_2 V_2}{n-1}\\\\ \\\\dQ=P_1 V_1 [\\frac{1}{n-1}-\\frac{1}{\\gamma-1}]-P_2 V_2 [\\frac{1}{n-1}-\\frac{1}{\\gamma-1}]\\\\ \\\\dQ=\\frac{\\gamma -n}{\\gamma -1}\\frac{P_1 V_1-P_2 V_2}{n-1}\\\\ \\\\dQ=\\frac{\\gamma -n}{\\gamma -1}W_{poly}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-30c37e1c1015acd433b3e3770a8fe0c2_l3.png)
Polytropic vs isentropic process
Polytropic process is a thermodynamic process which follows the equation
PVn = C
This process takes into consideration the frictional losses and irreversibility factor of a process. It is a real-life actual process followed by the gas under specific conditions.
Isentropic Process also known as reversible Adiabatic process is an ideal process in which no energy transfer or heat transfer takes place across the boundaries of the system. In this process system is assumed to have an insulated boundary. Since Heat transfer is zero. dQ = 0
According to first law of thermodynamics,
![]()
Polytropic process vs adiabatic process
Polytropic process is a thermodynamic process which follows the equation
PVn = C
This process takes into consideration the frictional losses and irreversibility factor of a process. It is a real-life actual process followed by the gas under specific conditions.
Adiabatic process is a special and specific condition of polytropic process in which .
Similar to Isentropic process in this process too, no energy transfer or heat transfer takes place across the boundaries of the system. In this process system is assumed to have an insulated boundary.
Polytropic efficiency
“Polytropic Efficiency well-defined as the ratio of Ideal work of compression for a differential pressure change in a multi-stage Compressor, to the Actual work of compression for a differential pressure change in a multi-stage Compressor.”
In simple terms it is an isentropic efficiency of the process for an infinitesimally small stage in a multi-stage compressor.
![]()
Where, γ = Adiabatic index
Pd = Delivery Pressure
Ps = Suction Pressure
Td = Delivery Temperature
Ts = Suction temperature
Polytropic head
Polytropic Head can be defined as the Pressure Head developed by a centrifugal compressor as the gas or air is being polytropically compressed. The amount of pressure developed depends upon the density of the gas is compressed and that varies with variation in density of gas.
![Rendered by QuickLaTeX.com H_p=53.3*z_{avg}*\\frac{T_s}{S}(\\frac{\\gamma \\eta _p}{\\gamma -1})[(\\frac{P_d}{P_s})^\\frac{\\gamma -1}{\\gamma \\eta _p}-1]](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-9c919db20c42b8e740e81a820f601ceb_l3.png)
Where,
γ= Adiabatic index
zavg = Average compressibility factor
η = Polytropic efficiency
Pd = Delivery Pressure
Ps = Suction Pressure
S = Specific gravity of gas
Ts = Suction temperature
Polytropic process for air | Polytropic process for an ideal gas
Air is assumed to be an Ideal gas and thus the laws of ideal gas is applicable to air.
Polytropic equation is,
![]()
![Rendered by QuickLaTeX.com \\\\P_1V_1^n=P_2V_2^n\\\\ \\\\\\frac{P_2}{P_1}=[\\frac{V_1}{V_2}]^n](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-2f79f958a45d3de1b856d5a94f13b960_l3.png)
………………………. Relations between Pressure [P] and Volume [V]
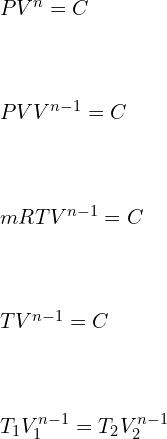
![]()
………………………. Relations between Volume [V] and Temperature [T]
![]()
………………………. Relations between Pressure [P] and Temperature [T]
Polytropic process examples
1. Consider a polytropic process having polytropic index n = (1.1). Initial conditions are: P1 = 0, V1 = 0 and ends with P2= 600 kPa, V2 = 0.01 m3. Evaluate the work done and Heat Transfer.
Answer: Work done by Polytropic process is given by
![]()
![]()
Heat Transfer is given by
![]()

2. A piston-cylinder contains Oxygen at 200 kPa, with volume of 0.1 m3 and at 200°C. Mass is added at such that the gas compresses with PV1.2 = constant to a final temperature of 400°C. Calculate the work done.
Ans: Polytropic work done is given by
![Rendered by QuickLaTeX.com \\\\W=\\frac{P_1V_1-P_2V_2}{n-1}\\\\W=\\frac{mR[T_2-T_1]}{n-1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-e851f1e9ecda47f4624cdfbe61caf7a5_l3.png)
![Rendered by QuickLaTeX.com \\\\\\frac{P_1V_1}{T_1} =mR \\\\mR=\\frac{200*10^3*0.1}{200}\\\\ \\\\mR=100 J/(kg. K) \\\\ \\\\W=\\frac{100*[400-200]}{1.22-1}\\\\ \\\\W=90.909 kJ](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-4a2c065006d2428fe2857f0a0f21c6fa_l3.png)
3. Consider Argon at 600 kPa, 30°C is compressed to 90°C in a polytropic process with n = 1.33. Find the work done on the Gas.
Ans: Polytropic work done is given by
![Rendered by QuickLaTeX.com \\\\W=\\frac{P_1V_1-P_2V_2}{n-1}\\\\W=\\frac{mR[T_2-T_1]}{n-1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-e851f1e9ecda47f4624cdfbe61caf7a5_l3.png)
for Argon at 30°C is 208.1 J/kg. K
Assuming m = 1 kg
work done is
![Rendered by QuickLaTeX.com W=\\frac{1*208.1[90-30]}{1.33-1}\\\\ \\\\W=37.836\\;kJ](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-f983a3808c53dd20f2f92333dd859293_l3.png)
4. Assume mass of 10kg of Xenon is stored in a cylinder at 500 K, 2 MPa, expansion is a Polytropic process (n = 1.28) with final pressure 100 kPa. Calculate the work done. Consider the system has constant specific heat.
Ans: Polytropic work done is given by
![Rendered by QuickLaTeX.com \\\\W=\\frac{P_1V_1-P_2V_2}{n-1}\\\\W=\\frac{mR[T_2-T_1]}{n-1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-e851f1e9ecda47f4624cdfbe61caf7a5_l3.png)
We know that,
![]()
![]()
for Xenon at 30°C is 63.33 J/kg. K
Assuming m = 10 kg
work done is
![Rendered by QuickLaTeX.com \\\\W=\\frac{10*63.33*[259.63-500]}{1.28-1}\\\\ \\\\W=-543.66\\;kJ](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-f3ef4aecd99714fbc6ce8f1b80373ad1_l3.png)
5. Take into consideration a cylinder-piston having initial volume 0.3 containing 5kg methane gas at 200 kPa. The gas is compressed polytropically (n = 1.32) to a pressure of 1 MPa and volume 0.005 . Calculate the Heat transfer during the process.
Ans: Polytropic Heat Transfer is given by
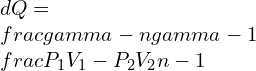

6. Take into consideration a cylinder-piston containing 1kg methane gas at 500 kPa, 20°C. The gas is compressed polytropically to a pressure of 800 kPa. Calculate the Heat Transfer with exponent n = 1.15.
Ans: Polytropic Heat Transfer is given by
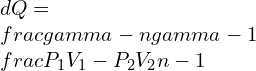
![Rendered by QuickLaTeX.com dQ=\\frac{\\gamma -n}{\\gamma -1}\\frac{mR[T_2-T_1]}{n-1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-9623d0173cc15e05297ccd524759531a_l3.png)
We know that, R for methane = 518.2 J/kg. K
![]()
![]()
![Rendered by QuickLaTeX.com \\\\dQ=\\frac{1.4 -1.15}{1.4 -1}\\frac{1*518.2*[311.52-293]}{1.15-1}\\\\\\\\dQ=39.997\\;kJ](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-0ddd733cda78faa73a241c473fe6bf77_l3.png)
7. 1 kg of Helium is stored in a piston – cylinder arrangement at 303 K, 200 kPa is compressed to 400K in a reversible polytropic process with exponent n = 1.24. Helium is an ideal gas characteristics so specific heat will be fixed. Find the work and Heat Transfer.
Ans: Polytropic work done is given by
![Rendered by QuickLaTeX.com \\\\W=\\frac{P_1V_1-P_2V_2}{n-1}\\\\W=\\frac{mR[T_2-T_1]}{n-1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-e851f1e9ecda47f4624cdfbe61caf7a5_l3.png)
R for Helium is 2077.1 J/kg
![Rendered by QuickLaTeX.com \\\\W=\\frac{2077.1*[400-303]}{1.24-1}=839.494\\;kJ](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-58fbc142958923099feceb48f6431e40_l3.png)
Polytropic Heat Transfer is given by
![]()

8.Assume air stored in a cylinder having volume of 0.3 Liters at 3 MPa, 2000K. Air expands following a reversible polytropic process with exponent, n = 1.7, a volume ratio is observed as 8:1 in this case. Calculate the polytropic work for the process and compare it with adiabatic work if the expansion process follows reversible adiabatic expansion.
Ans: We are given with
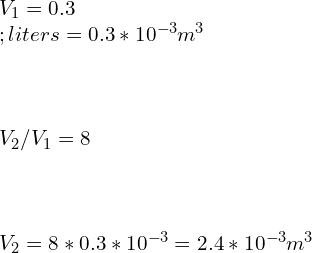
Relations between Pressure [P] and Volume [V]
![Rendered by QuickLaTeX.com \\\\P_1V_1^n=P_2V_2^n\\\\ \\\\\\frac{P_2}{P_1}=[\\frac{V_1}{V_2}]^n](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-2f79f958a45d3de1b856d5a94f13b960_l3.png)
![Rendered by QuickLaTeX.com \\\\\\frac{P_2}{3}=[\\frac{0.3}{2.4}]^{1.7}\\\\\\\\P_2=0.0874\\;MPa](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-a09eba21348fdf1e95edca72e436526b_l3.png)
Polytropic work done is given by
![]()
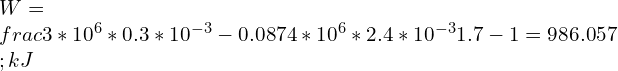
Adiabatic work done is given by
![]()
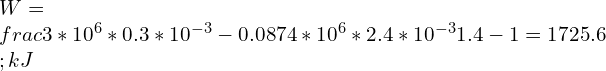
For expansion process the Work done through reversible adiabatic process is greater than the Work done through reversible Polytropic process.
9. A closed container contains 200L of gas at 35°C, 120 kPa. The gas is in compressng in a polytropic process till it reaches to 200°C, 800 kPa. Find the polytropic work done by the air for n = 1.29.
Ans: Relations between Pressure [P] and Volume [V]
![Rendered by QuickLaTeX.com \\\\P_1V_1^n=P_2V_2^n\\\\ \\\\\\frac{P_2}{P_1}=[\\frac{V_1}{V_2}]^n](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-2f79f958a45d3de1b856d5a94f13b960_l3.png)
![Rendered by QuickLaTeX.com \\\\\\frac{800}{120}=[\\frac{200}{V_2}]^{1.29} \\\\\\\\V_2=45.95\\;L](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-41323679bbdf82666782c64b16cfa457_l3.png)
Polytropic work done is given by
![]()
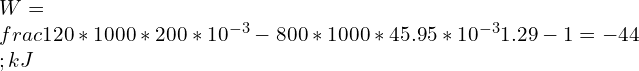
10. A mass of 12 kg methane gas at 150°C, 700 kPa, undergoes a polytropic expansion with n = 1.1, to a final temperature of 30°C. Find the Heat Transfer?
Ans: We know that, R for methane = 518.2 J/kg. K
Polytropic Heat Transfer is given by
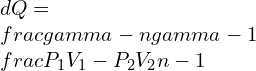
![Rendered by QuickLaTeX.com dQ=\\frac{\\gamma -n}{\\gamma -1}\\frac{mR[T_2-T_1]}{n-1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-9623d0173cc15e05297ccd524759531a_l3.png)
![Rendered by QuickLaTeX.com dQ=\\frac{1.4-1.1}{1.4 -1}\\frac{12*518.2*[30-150]}{1.1-1}=-5.596\\;MJ](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-6ebe82b84e38a99ceddb14a01559df47_l3.png)
11. A cylinder-piston Assembly contains R-134a at 10°C; the volume is 5 Liters. The Coolant is compressed to 100°C, 3 MPa Following a reversible polytropic process. calculate the work done and Heat Transfer?
Ans: We know that, R for R-134a = 81.49 J/kg. K
Polytropic work done is given by
![]()
![Rendered by QuickLaTeX.com W=\\frac{1*81.49*[100-10]}{1.33-1}=22.224\\;kJ](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-f99d83d9c42cc7b2180db4866e1d97ed_l3.png)
Polytropic Heat Transfer is given by
![]()

12. Is a polytropic process isothermal in nature?
Ans: When n becomes 1 for a polytropic process: Under the Assumption of Ideal Gas Law, The PV = C represents the Constant Temperature or Isothermal Process.
13. Is a polytropic process reversible?
Ans: a polytropic processes are internally reversible. Some examples are:
n = 0: P = C: Represents an isobaric process or constant pressure process.
n = 1: PV = C: Under the Assumption of Ideal Gas Law, The PVγ = C represents the Constant Temperature or Isothermal Process.
n = γ: Under the assumption of ideal gas law, represents the Constant entropy or Isentropic Process or reversible adiabatic process.
n = Infinity : Represents an isochoric process or constant volume process.
14. Is adiabatic polytropic process?
Ans: when n = γ: Under the assumption of ideal gas law PVγ = C, represents the Constant entropy or Isentropic Process or reversible adiabatic process.
14. What is Polytropic efficiency?
Ans: Polytropic Efficiency can be defined as the ratio of Ideal work of compression, to the Actual work of compression for a differential pressure change in a multi-stage Compressor. In simple terms it is an isentropic efficiency of the process for an infinitesimally small stage in a multi-stage compressor.
In simple terms it is an isentropic efficiency of the process for an infinitesimally small stage in a multi-stage compressor.
![]()
Where, γ = Adiabatic index
Pd = Delivery Pressure
Ps = Suction Pressure
Td = Delivery Temperature
Ts = Suction temperature
15. What is Gamma in Polytropic process?
Ans: In a Polytropic process when n = γ: Under the assumption of ideal gas law PVγ = C, represents the Constant entropy or Isentropic Process or reversible adiabatic process.
16. what is n in polytropic process?
Ans: The polytropic process can be defined by the equation,
PVn = C
the exponent n is called polytropic index. It depends upon the material and varies from 1.0 to 1.4. It is also called as constant specific heat process, in which heat absorbed by the gas taken into consideration because of unit rise in temperature is constant.
17. What conclusions can be made for a polytropic process with n=1?
Ans: when n = 1: PVn = C : Under the Assumption of Ideal Gas Law becomes The PV = C represents the Constant Temperature or Isothermal Process.
18. What is a non-polytropic process?
Ans: The polytropic process can be defined by the equation PVn = C , the exponent n is called polytropic index. When,
- n < 0: Negative Polytropic index denotes a process where Work and heat transfer occurs simultaneously through the boundaries of system. However, such spontaneous process violates the Second law of Thermodynamics. These special cases are used in thermal interaction for astrophysics and chemical energy.
- n = 0: P = C: Represents an isobaric process or constant pressure process.
- n = 1: PV = C: Under the Assumption of Ideal Gas Law, The PV = C represents the Constant Temperature or Isothermal Process.
- 1 < n < γ: Under the assumption of ideal gas law, In these Process the heat and work flow move in opposite direction (K>0) Like in Vapor compression cycles, Heat lost to hot surrounding.
- n = γ: Under the assumption of ideal gas law, PVγ = C represents the Constant entropy or Isentropic Process or reversible adiabatic process.
- γ<n < Infinity : In this process it is assumed that heat and work flow move in same direction like in IC engine when some amount of generated heat is lost to the cylinder walls etc.
- n = Infinity : Represents an isochoric process or constant volume process
19. Why is heat transfer negative in a polytropic process?
Ans: Polytropic Heat Transfer is given by
![]()
When γ < n < Infinity : In this process it is assumed that heat and work flow move in same direction. The change in temperature is due to change in internal energy rather than heat supplied. Thus, even though heat is added in a polytropic expansion the temperature of the gas decreases.
20. Why does the temperature decrease on heat addition in the polytropic process?
Ans: Polytropic Heat Transfer is given by
![]()
For the condition: 1 < n < γ: Under the assumption of ideal gas law, In these Process the heat and work flow move in opposite direction (K>0) Like in Vapor compression cycles, Heat lost to hot surrounding. The change in temperature is due to change in internal energy rather than heat supplied. The work produced exceeds the amount of heat supplied or added. Thus, even though heat is added in a polytropic expansion the temperature of the gas decreases.
21. In a polytropic process where PVn =constant, is temperature constant as well?
Ans: In a polytropic process where PVn =constant, the temperature remains constant only when the polytropic index n = 1. For n = 1: PV = C: Under the Assumption of Ideal Gas Law, The PV = C represents the Constant Temperature or Isothermal Process.
To know about Simply Supported Beam (click here)and Cantilever beam (Click here)

I am Hakimuddin Bawangaonwala , A Mechanical Design Engineer with Expertise in Mechanical Design and Development. I have Completed M. Tech in Design Engineering and has 2.5 years of Research Experience. Till now Published Two research papers on Hard Turning and Finite Element Analysis of Heat Treatment Fixtures. My Area of Interest is Machine Design, Strength of Material, Heat Transfer, Thermal Engineering etc. Proficient in CATIA and ANSYS Software for CAD and CAE. Apart from Research.