When a deformable material is stretched in a particular direction, its length increases in that direction, and thickness reduces in the lateral one. Similarly, the material is compressed in a specific direction and, its length decreases in that direction, and thickness increases in the lateral one. Poisson’s ratio is a parameter that relates these deformations, which is useful in material selection and application.
Poisson’s Ratio Definition | Poisson’s Ratio Equation
When we apply tensile stress on the material, there is elongation in the direction of applied force and shrinkage in the transverse/lateral movement. Thus the strain gets produced in both directions. The ratio of strain produced in the transverse direction to the strain produced in the direction of tensile stress application is known as Poisson’s ratio.
Its symbol is ʋ or μ.
The ratio obtained has a negative sign, as the ratio obtained is always negative.
Thus,
Poisson’s Ratio= Transverse Strain/ axial Strain
ʋ= -(εx/εy)
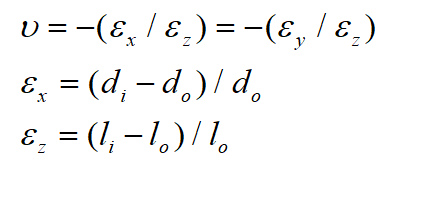
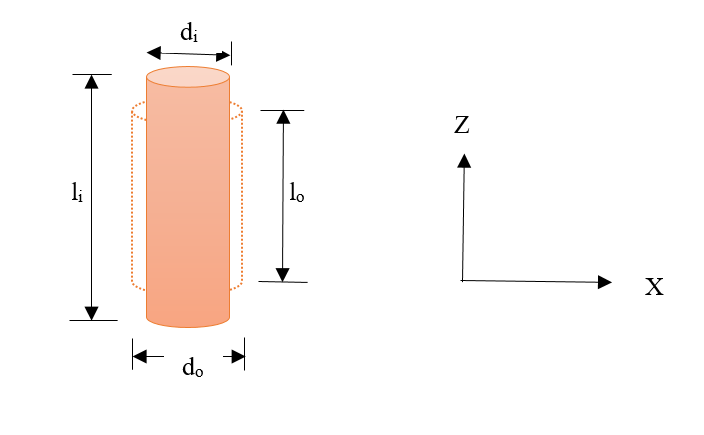
Similarly, if compressive stress is applied to the material, there is shrinkage in the direction of applied force and thickening in the transverse/ lateral direction. Thus, the strain gets produced in both directions. The ratio of strain produced in the transverse direction to the strain produced in the direction of compressive stress application is also known as Poisson’s ratio.
Generally, it ranges from 0 to 0.5 for engineering materials. Its value increases under tensile stress and decreases under compressive stress.
For more details click here!
Poisson’s Ratio of Steel
- The value of Poisson’s ratio for steel ranges from 0.25 to 0.33.
- The average value of Poisson’s ratio for steel 0.28.
- It depends on the steel type used.
Following is the list of Poisson’s ratio for different steels
| Steel Type | Poisson’s Ratio |
| High Carbon Steel | 0.295 |
| Mild Steel | 0.303 |
| Cast Steel | 0.265 |
| Cold Rolled Steel | 0.287 |
| Stainless Steel 18-8 | 0.305( 0.30-0.31) |
Poisson’s Ratio of Aluminum
- The value of Poisson’s ratio for aluminum ranges from 0.33 to 0.34.
- The average value of Poisson’s ratio for aluminum is 0.33 and for aluminum alloy 0.32.
- It depends on the type of aluminum or aluminum alloy used.
Following is the list of Poisson’s ratio for different aluminum
| Aluminum Type | Poisson’s Ratio |
| Aluminum Bronze | 0.30 |
| Rolled Aluminum | 0.337/0.339 |
| Rolled Pure Aluminum | 0.327 |
Poisson’s Ratio of Concrete
- The value of Poisson’s ratio for concrete ranges from 0.15 to 0.25.
- Its general value is taken as 0.2.
- It depends on the type of concrete (wet, dry, saturated) and loading conditions.
- Its value for high strength concrete is 0.1, and for low strength concrete, it is o.2.
Poisson’s Ratio of Copper
- The value of Poisson’s ratio ranges from 0.34 to 0.35.
- Its general value is taken as 0.355.
- It depends on the type of copper or copper alloy used.
Following is the list of Poisson’s ratio for different copper
| Copper Type | Poisson’s Ratio |
| Normal Brass | 0.34 |
| Brass, 70-30 | 0.331 |
| Brass, cast | 0.357 |
| Bronze | 0.34 |
Poisson’s Ratio of Rubber
- The value of Poisson’s ratio for rubber is from 0.48 to 0.50.
- For most of the rubbers, it is equal to 0.5.
- Its value for natural rubber is 0.5.
- It has the highest value of Poisson’s Ratio.
Poisson’s Ratio of Plastic
- The Poisson’s ratio of plastics generally increases with time, strain, and temperature and decreases with strain rate.
- Following is the list of Poisson’s ratio for different plastics
| Plastic Type | Poisson’s Ratio |
| PAMS | 0.32 |
| PPMS | 0.34 |
| PS | 0.35 |
| PVC | 0.40 |
Poisson’s Ratio and Young’s Modulus
The materials for which elastic behavior does not vary with the crystallographic direction are known as elastically isotropic materials. Using Poisson’s ratio of the material, we can obtain a relation between Modulus of Rigidity and Modulus of Elasticity for isotropic materials as follows.
Y= 2*G*(1+ʋ)
Where, Y= Modulus of Elasticity
G= Modulus of Rigidity
ʋ= Poisson’s Ratio
Questions and Answers
What is meant by Poisson’s ratio?
When we apply tensile stress on the material, there is elongation in the direction of applied force and shrinkage in the transverse/lateral direction. Thus the strain gets produced in both directions. The ratio of strain produced in the transverse direction to the strain produced in the direction of tensile stress application is known as Poisson’s ratio.
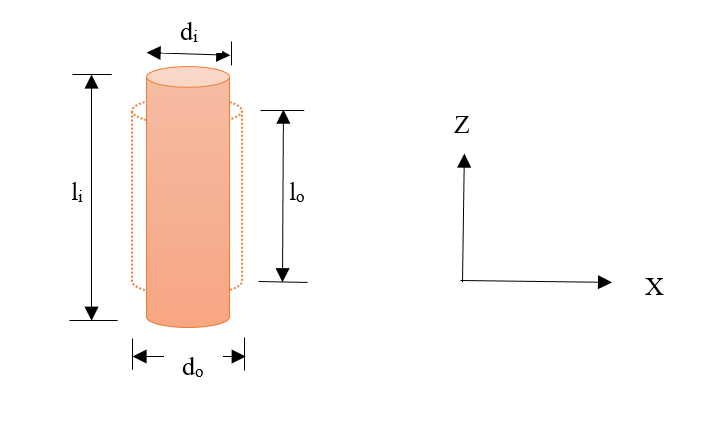
What does a Poisson ratio of 0.5 mean?
Poisson’s ratio of precisely 0.5 means the material is perfectly incompressible isotropic material deformed elastically at small strains.
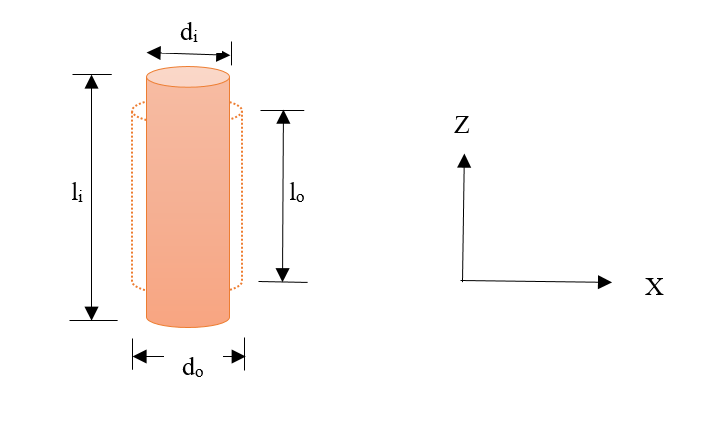
How is Poisson’s ratio calculated?
Poisson’s Ratio= Transverse Strain/ axial Strain
ʋ=-εx/εy

What is the Poisson’s ratio for steel?
The value of Poisson’s ratio for steel ranges between 0.25 to 0.33.
The average value of Poisson’s ratio for steel 0.28.
What is Poisson’s ratio for aluminum?
The value of Poisson’s ratio for aluminum ranges between 0.33 to 0.34.
The average value of Poisson’s ratio for aluminum is 0.33 and for aluminum alloy 0.32.
What is Poisson’s ratio for concrete?
The value of Poisson’s ratio for concrete ranges between 0.15 to 0.25.
Its general value is taken as 0.2.
It depends on the type of concrete (wet, dry, saturated) and loading conditions.
Its value for high strength concrete is 0.1, and for low strength concrete, it is 0.2.
What is the relation between Poisson’s Ratio and Young’s Modulus of Elasticity?
Y= 2*G*(1+ʋ)
Where, Y= Modulus of Elasticity
G= Modulus of Rigidity
ʋ= Poisson’s Ratio
Which parameters affect the Poisson’s Ratio of Polymers?
The Poisson’s ratio of polymeric materials like plastic generally increases with time, strain, and temperature and decreases with strain rate.
What if Poisson’s ratio is zero?
If the Poisson’s ratio is zero, the material is not deformable; hence, it is a rigid body.
Which material has the highest Poisson’s ratio?
Rubber has the highest Poisson’s Ratio, almost equal to 0.5.
Why is Poisson’s ratio always positive?
Poisson’s ratio is the negative of the ratio of lateral strain to axial strain. The ratio of lateral strain to axial strain is always negative because elongation causes contraction in diameter, which ultimately makes the ratio negative .similarly, compression causes elongation in diameter, which makes the ratio negative.
Is Poisson’s ratio constant?
For the stresses in the elastic range, Poisson’s ratio is almost constant.
Does Poisson’s ratio dependent on temperature?
Yes. With the increasing temperature, Poisson’s ratio decreases.
Objective Questions
Tensile stress is applied along the longitudinal axis of a cylindrical brass rod with a diameter of 10mm. Determine the magnitude of the strain produced in the transverse direction where the load is required to produce a 2.5 *10-3 change in diameter if the deformation is entirely elastic. Poisson’s ratio of brass is 0.34.
- 3.5*10-3
- 5.5*10-3
- 7.35*10-3
- 1.0*10-3
Solution: Answer is option 3.
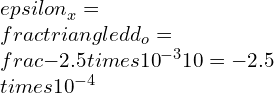
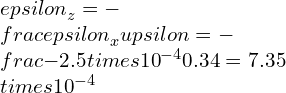
A wire of length 2 m is loaded, and an elongation of 2mm is produced. If the wire’s diameter is 5 mm, find the change in the diameter of the wire when elongated. Poisson’s ratio of the wire is 0.35
Solution: L= 2m
Del L= 2mm
D= 1mm
ʋ= 0.24
Longitudinal Strain= 2*10-3/2=10-3
Lateral Strain= Poisson’s Ratio*Longitudinal Strain
= 0.35*10-3
Lateral Strain= Change in diameter/ Original Diameter=0.35*10-3
Change in Diameter=0.35*10-3*5*10-3
= 1.75*10-6
=1.75*10-7
Thus, the Change in diameter is 1.75*10-7mm.
A wire of steel having a cross-sectional area of 2 mm2 is stretched by 20 N. Find the lateral strain produced in the wire. Young’s Modulus for steel is 2*1011N/m2, and Poisson’s ratio is 0.311.
Solution: A= 2mm2= 2*10-6mm2
F= 20N
Y= Longitudinal Stress/ Longitudinal Strain
=F/ (A*Longitudinal Strain)
Longitudinal Strain= F/(Y*A)
=20/ (1*10-6*2*1011) = 10-4
Poisson’s Ratio= Lateral Strain/ Longitudinal Strain
Lateral Strain= Poisson’s Ratio*Longitudinal Strain
= 0.311*10-4
Lateral Strain=0.311*10-4
Conclusion
In this articles, all the important concepts related to Poisson’s Ratio are discussed in detailed . Numerical and subjective type of questions are added for practice.
To learn more on strength of material Click Here!

The lambdageeksScience Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.