When objects slide or move on an inclined plane, the coefficient of friction plays a crucial role in determining the resistance to motion. The coefficient of friction is a measure of the interaction between two surfaces and determines the frictional force between them. In this blog post, we will explore how to find the coefficient of friction on an inclined plane.
We will cover the necessary tools and materials, the step-by-step procedure, and provide worked-out examples. We will also differentiate between the coefficients of static and kinetic friction on an inclined plane to gain a deeper understanding of their differences.
Determining the Coefficient of Friction on an Inclined Plane
Required Tools and Materials
Before we dive into the procedure, let’s gather the tools and materials we need. Here’s a list of what you’ll require:
– Inclined plane
– Object to slide
– Protractor or angle measuring device
– Weighing scale
– Measuring tape or ruler
Step-by-step Procedure
Now, let’s walk through the step-by-step procedure to find the coefficient of friction on an inclined plane:
- 1. Set up the inclined plane at the desired angle of inclination. Make sure it is stable and secure.
- 2. Measure the angle of inclination using a protractor or angle measuring device. This angle will be denoted as θ.
- 3. Place the object on the inclined plane and adjust its position until it remains stationary without any external force acting on it.
- 4. Measure the weight of the object using a weighing scale. This weight will be denoted as W.
- 5. Calculate the normal force acting on the object, which is the component of the weight perpendicular to the inclined plane. The normal force (N) can be calculated using the formula N = W * cos(θ).
- 6. Gradually increase the inclination of the plane until the object starts sliding. Note down the angle of inclination at which the object begins to slide. This angle will be denoted as θs.
- 7. Measure the sliding distance of the object along the inclined plane.
- 8. Calculate the coefficient of static friction (μs) using the formula μs = tan(θs).
- 9. Calculate the coefficient of kinetic friction (μk) using the formula μk = tan(θ).
Worked-out Example
To illustrate the procedure, let’s consider an example:
- 1. The inclined plane has an angle of inclination (θ) of 30 degrees.
- 2. The object on the inclined plane has a weight (W) of 20 N.
- 3. The object begins to slide at an angle of inclination (θs) of 20 degrees.
- 4. The sliding distance of the object is measured to be 2 meters.
Using the given values, we can calculate the coefficients of static and kinetic friction:
– Normal force (N) = W * cos(θ) = 20 N * cos(30 degrees) = 17.32 N
– Coefficient of static friction (μs) = tan(θs) = tan(20 degrees) ≈ 0.364
– Coefficient of kinetic friction (μk) = tan(θ) = tan(30 degrees) ≈ 0.577
Therefore, the coefficient of static friction on the inclined plane is approximately 0.364, while the coefficient of kinetic friction is approximately 0.577.
Finding the Coefficient of Friction on an Inclined Plane without Mass
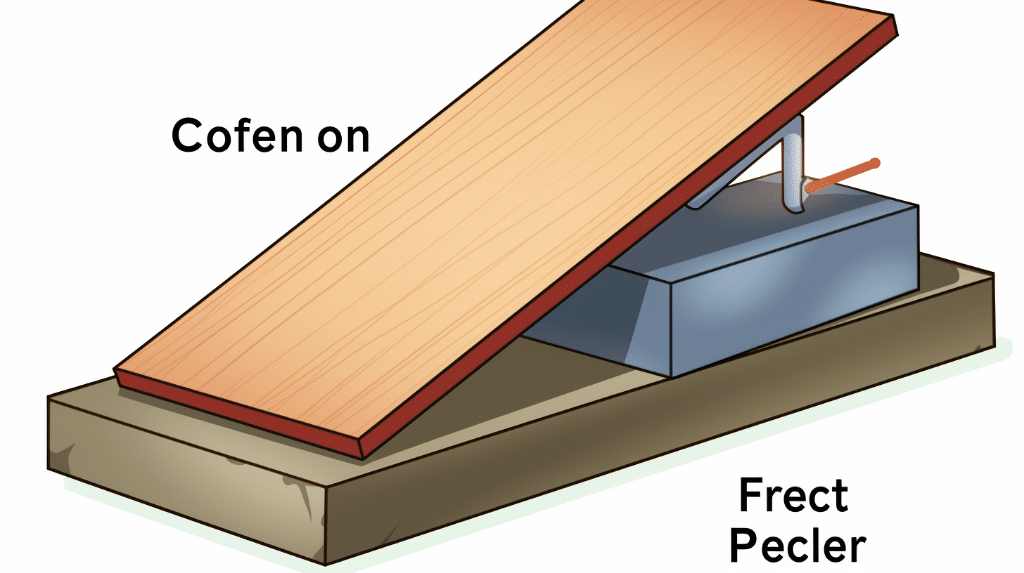
Theoretical Background
Now, let’s explore how to find the coefficient of friction on an inclined plane without knowing the mass of the object. This method utilizes the relationship between the angle of inclination and the coefficient of friction.
Detailed Procedure
Here’s a detailed procedure to find the coefficient of friction on an inclined plane without mass:
- 1. Set up the inclined plane at the desired angle of inclination and ensure its stability.
- 2. Measure the angle of inclination using a protractor or angle measuring device. Let’s denote this angle as θ.
- 3. Place the object on the inclined plane and adjust its position until it remains stationary without any external force acting on it.
- 4. Gradually increase the inclination of the plane until the object starts sliding. Note down the angle of inclination at which the object begins to slide. This angle will be denoted as θs.
- 5. Calculate the coefficient of static friction (μs) using the formula μs = tan(θs).
- 6. Calculate the coefficient of kinetic friction (μk) using the formula μk = tan(θ).
Practical Example
Let’s consider a practical example to understand this method better:
- 1. The inclined plane has an angle of inclination (θ) of 45 degrees.
- 2. The object begins to slide at an angle of inclination (θs) of 30 degrees.
Using the formulas mentioned above, we can calculate the coefficients of static and kinetic friction:
- – Coefficient of static friction (μs) = tan(θs) = tan(30 degrees) ≈ 0.577
- – Coefficient of kinetic friction (μk) = tan(θ) = tan(45 degrees) ≈ 1
Hence, the coefficient of static friction on the inclined plane is approximately 0.577, and the coefficient of kinetic friction is approximately 1.
Differentiating Between Coefficient of Static and Kinetic Friction on an Inclined Plane
Defining Static and Kinetic Friction
Before understanding how to calculate each coefficient, let’s define static and kinetic friction.
- – Static friction occurs when two surfaces are in contact but not sliding relative to each other. It prevents the object from moving until a certain force is applied.
- – Kinetic friction, on the other hand, occurs when two surfaces are sliding relative to each other. It opposes the motion of the object.
How to Calculate Each Coefficient
To calculate the coefficient of static friction (μs) and the coefficient of kinetic friction (μk) on an inclined plane, we use the following formulas:
- – Coefficient of static friction (μs) = tan(θs), where θs is the angle of inclination at which the object begins to slide.
- – Coefficient of kinetic friction (μk) = tan(θ), where θ is the angle of inclination of the inclined plane.
Examples for Better Understanding
Let’s consider an example to differentiate between the coefficients of static and kinetic friction:
– The inclined plane has an angle of inclination (θ) of 20 degrees.
– The object begins to slide at an angle of inclination (θs) of 15 degrees.
Using the formulas mentioned earlier, we can calculate the coefficients of static and kinetic friction:
– Coefficient of static friction (μs) = tan(θs) = tan(15 degrees) ≈ 0.268
– Coefficient of kinetic friction (μk) = tan(θ) = tan(20 degrees) ≈ 0.364
In this example, the coefficient of static friction is approximately 0.268, while the coefficient of kinetic friction is approximately 0.364.
By understanding the distinction between static and kinetic friction, we can better comprehend the nature of the forces at play on an inclined plane.
Numerical Problems on how to find coefficient of friction on an inclined plane
Problem 1
A block of mass 5 kg is placed on an inclined plane with an angle of 30 degrees. The block is on the verge of sliding down the plane, and the force required to just prevent the block from sliding is 30 N. Find the coefficient of friction between the block and the plane.
Solution:
Given:
Mass of the block, m = 5 kg
Angle of the inclined plane, θ = 30 degrees
Force required to prevent sliding, F = 30 N
The force required to prevent sliding can be calculated using the equation:
![]()
where g is the acceleration due to gravity and μ is the coefficient of friction.
Rearranging the equation to solve for μ:
![]()
Substituting the given values:
![]()
Simplifying the equation gives:
![]()
Therefore, the coefficient of friction between the block and the inclined plane is approximately 0.232.
Problem 2
A box of mass 10 kg slides down an inclined plane with a constant acceleration of 2 m/s². The angle of the inclined plane is 45 degrees. Calculate the coefficient of friction between the box and the plane.
Solution:
Given:
Mass of the box, m = 10 kg
Acceleration of the box, a = 2 m/s²
Angle of the inclined plane, θ = 45 degrees
The acceleration of the box can be related to the force of friction using the equation:
![]()
where g is the acceleration due to gravity and μ is the coefficient of friction.
Rearranging the equation to solve for μ:
![]()
Substituting the given values:
![]()
Simplifying the equation gives:
![]()
Therefore, the coefficient of friction between the box and the inclined plane is approximately 0.414.
Problem 3
A block of mass 2 kg is placed on an inclined plane with an angle of 60 degrees. The block is at rest and requires a force of 7 N to start sliding down the plane. Determine the coefficient of static friction between the block and the plane.
Solution:
Given:
Mass of the block, m = 2 kg
Angle of the inclined plane, θ = 60 degrees
Force required to start sliding, F = 7 N
The force required to start sliding can be calculated using the equation:
![]()
where g is the acceleration due to gravity and μ_s is the coefficient of static friction.
Rearranging the equation to solve for μ_s:
![]()
Substituting the given values:
![]()
Simplifying the equation gives:
![]()
Therefore, the coefficient of static friction between the block and the inclined plane is approximately 0.577.
Also Read:
- Telescopic sights
- Where is friction not useful
- Is deceleration negative
- Problems on gravitation
- Dynamic equilibrium conditions
- Stable equilibrium example
- Adaptive optics
- What is frictionless surface
- Why static friction is greater than kinetic
- Exothermic reaction 2
Hi ….I am Abhishek Khambhata, have pursued B. Tech in Mechanical Engineering. Throughout four years of my engineering, I have designed and flown unmanned aerial vehicles. My forte is fluid mechanics and thermal engineering. My fourth-year project was based on the performance enhancement of unmanned aerial vehicles using solar technology. I would like to connect with like-minded people.