CARNOT CYCLE
Nicolas Léonard Sadi-Carnot, a French mechanical engineer, Scientist, and physicist, introduced a heat engine known as the Carnot Engine in the book “Reflections on the Motive Power of Fire. It leads to being the foundation of the Second law of thermodynamics and entropy. Carnot’s contribution holds a remark which gave him the title of “Father of Thermodynamics.
Table of Content
Carnot cycle in thermodynamics | working principle of Carnot cycle | ideal Carnot cycle | Carnot cycle thermodynamics | Carnot cycle definition | Carnot cycle working principle | air standard Carnot cycle| Carnot cycle reversible.
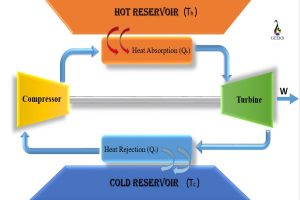
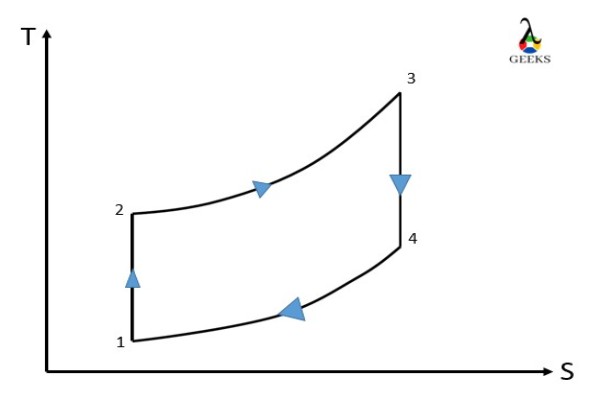
Carnot cycle is the theoretical cycle that works under two thermal reservoirs (Th & Tc) undergoing compression and expansion simultaneously.
It consists of four reversible processes, of which two are isothermal, i.e., constant temperature followed alternately by two reversible adiabatic processes.
The working medium used in the Sadi-Carnot cycle is atmospheric air.
Heat addition and Heat rejection are carried out at a constant temperature, but no phase change is considered.
Importance of Carnot Cycle
The invention of the Carnot cycle was a very big step in the history of thermodynamics. First, it gave theoretical working of heat engine used for the design of an actual heat engine. Then, reversing the cycle, we get refrigeration effect (mentioned below).
Carnot cycle work between two thermal reservoirs (Th & Tc), and its efficiency depends only on this temperature and doesn’t depend on the fluid type. That is Carnot’s cycle efficiency is fluid independent.
Carnot cycle pv diagram | Carnot cycle ts diagram | pv and ts diagram of Carnot cycle | Carnot cycle pv ts | Carnot cycle graph | Carnot cycle pv diagram explained | Carnot cycle ts diagram explained
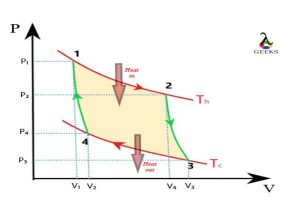

Process 1-2: Isothermal expansion
In this process, the air is expanded with constant temperature while gaining heat.
That is, constant temperature heat addition takes place.
Expansion => pressure ↑ => results Temperature ↓
Heat Addition => Temperature ↑
Hence Temperature remain constant
Process 2-3: Reversible adiabatic expansion
In this process, the air is expanded, keeping entropy constant and with no heat interaction.
That is no change in entropy, and the system is insulated
We get work output in this process
Process 3-4: isothermal compression
In this process, the air is compressed with a constant temperature while losing heat.
That is, constant temperature heat rejection takes place.
Compression => pressure ↓ => results: Temperature ↑
Heat Addition => Temperature ↓
Hence Temperature remain constant
Process 4-1: Reversible Adiabatic Compression
In this process, the air is compressed, keeping entropy constant and no heat interaction.
That is no change in entropy, and the system is insulated
We supply work in this process
Carnot cycle consists of | Carnot cycle diagram | Carnot cycle steps | 4 stages of Carnot cycle | Carnot cycle work| isothermal expansion in Carnot cycle| Carnot cycle experiment
Process 1-2:
The expansion process is carried out where temperature Th is kept constant, and heat (Qh) is added to the system. The temperature is kept constant as follows: The rise in temperature due to heat addition is compensated by the decrease in temperature due to expansion.
Hence the process carried out results as constant temperature as the start and end temperature of the process is same.
Process 2-3:
As we can see, the process is reversible (change in internal energy = 0) Adiabatic (only work transfer, no heat involvement), the expansion carried out just results in a change in temperature (from Th to Tc), keeping the entropy constant.
System act as being insulated for this part of the expansion.
Sensible cooling is taking place.
Process3-4:
The compression process is carried out where temperature Tc is kept constant, and heat is removed from the system. The temperature is kept constant as follows: The decrease in temperature due to heat rejection is compensated by the increase in temperature due to compression.
Hence the process carried out results as constant temperature as the start and end temperature of the process is same.
Similar to processes 1-2 but in the exact opposite manner.
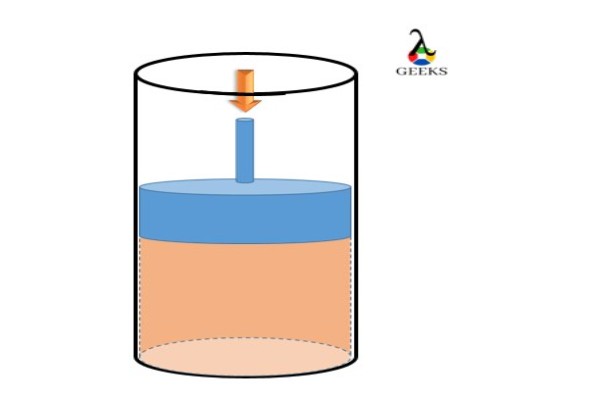
Process 4-1:
As we can see, the process is reversible (change in internal energy = 0) Adiabatic (only work transfer, no heat involvement), the compression carried out just results in a change in temperature (from Tc to Th), keeping the entropy constant.
System act as being insulated for this part of the compression.
Sensible heating is taking place.
Carnot cycle equations| Carnot cycle derivation
Process 1-2: Isothermal expansion
as Th is kept constant. [Internal energy (du) = 0] ( PV = K)
Qh = W ,
therefore, 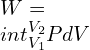
![]()
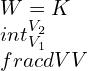
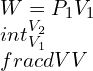
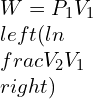
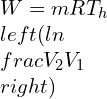
Process 2-3: Reversible adiabatic expansion
![]()
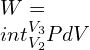
![]()
therefore 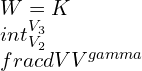
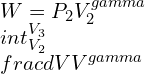

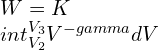
![Rendered by QuickLaTeX.com W = K left [ frac{V^{1-gamma }}{1-gamma } right ]_{2}^{3}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-bfaf0fe480662f031800cc9bfa53ad06_l3.png)
![]()
![Rendered by QuickLaTeX.com W=left [ frac{P_{3}V^{gamma }_{3}V_{3}^{1-gamma }-P_{2}V^{gamma }_{2}V_{2}^{1-gamma }}{1-gamma } right ]](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-bbd3e149152f16faaf0d4a489e50cb74_l3.png)
![Rendered by QuickLaTeX.com W=left [ frac{P_{3}V_{3}-P_{2}V_{2}}{1-gamma } right ]](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-901471515d0dd0f8071dddc426ca39f0_l3.png)
Also
![]()
![Rendered by QuickLaTeX.com left [ frac{T_{2}}{T_{3}} right ] =left [ frac{V_{3}}{V_{2}} right ]^{gamma -1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-91e649eb42cb3f5904f72969eb3cb41a_l3.png)
As process is Adiabatic , Q = 0
therefore W = -du
Process 3-4: isothermal compression
similar to process 1-2, we can get
as Tc is kept constant. [Internal energy (du) = 0] ( PV = K)
Qc = W ,

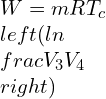
Process 4-1: Reversible Adiabatic Compression
similar to process 2-3, we can get
![Rendered by QuickLaTeX.com W=left [ frac{P_{1}V_{1}-P_{4}V_{4}}{1-gamma } right ]](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-a87c4523eb09b0daf20f2448784386fd_l3.png)
![]()
![Rendered by QuickLaTeX.com left [ frac{T_{1}}{T_{4}} right ] =left [ frac{V_{4}}{V_{1}} right ]^{gamma -1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-b5071dcab86067c0c30e711d1eddf574_l3.png)
Carnot cycle work done derivation
According to first law of thermodynamics
Wnet = Qtotal
Wnet = Qh-Qc
Wnet = 
Derivation of entropy from carnot cycle | entropy change in carnot cycle | change in entropy carnot cycle | derivation of entropy from carnot cycle | entropy change in carnot cycle
To make cycle reversible, Change in entropy is zero (du = 0).
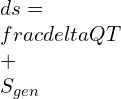
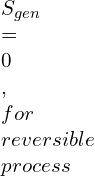
that means,
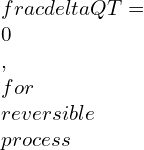
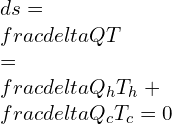
For process :1-2
![]()
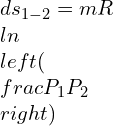
For process :1-2
![]()
![]()
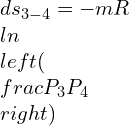


carnot cycle efficiency| carnot cycle efficiency calculation| carnot cycle efficiency equation| carnot cycle efficiency formula | carnot cycle efficiency proof | carnot cycle maximum efficiency | carnot cycle efficiency is maximum when | maximum efficiency of carnot cycle
Carnot cycle efficiency has maximum efficiency considering the Th as the hot reservoir and Tc as a cold reservoir to eliminate any losses.
It is a ratio of Amount of work done by the Heat engine to the Amount of heat required by the heat engine.
![]()
![]()
![]()
![]()
As from above equation we know,
![Rendered by QuickLaTeX.com left [ frac{T_{1}}{T_{4}} right ] =left [ frac{V_{4}}{V_{1}} right ]^{gamma -1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-b5071dcab86067c0c30e711d1eddf574_l3.png)
&
![Rendered by QuickLaTeX.com left [ frac{T_{2}}{T_{3}} right ] =left [ frac{V_{3}}{V_{2}} right ]^{gamma -1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-91e649eb42cb3f5904f72969eb3cb41a_l3.png)
but
![]()
![]()
![]()
![]()
We can get an efficiency of 100% if we get to reject heat at 0 k (Tc = 0)
Carnot holds a maximum efficiency of all the engines performing under the same thermal reservoir as Carnot cycle work reversible, making assumptions of eliminating all the losses and making cycle a frictionless cycle, which is never possible in practice.
Hence all practical cycles will have efficiency less than Carnot efficiency.
Reverse carnot cycle | the reversed carnot cycle | reversed carnot refrigeration cycle
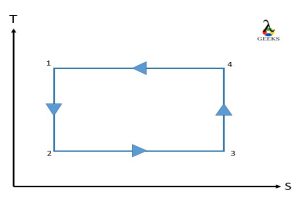
Reverse Carnot cycle:
As all the processes carried out in the Carnot cycle are reversible, We can make it work in a reverse manner, i.e., to take heat from the lower temperature body and dumped to a higher temperature body, making it a refrigeration cycle.
.

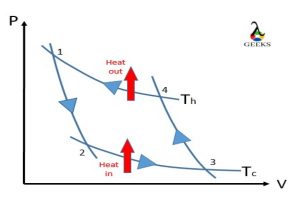

Process 1-2: Reversible adiabatic expansion
In this process, the air is expanded, temperature is reduced to Tc, keeping entropy constant and with no heat interaction.
That is no change in entropy, and the system is insulated
Process 2-3: Isothermal expansion
In this process, the air is expanded with constant temperature while gaining heat. The heat is gain from the Heat sink at low temperature. Heat addition takes place while keep temperature(Tc) is kept constant.
Process 3-4: Reversible Adiabatic Compression
In this process, the air is compressed, rising the temperature to Th, keeping entropy constant and no heat interaction.
That is no change in entropy, and the system is insulated
Process 4-1: isothermal compression
In this process, the air is compressed with a constant temperature while losing heat. Heat is rejected to the hot reservoir. Heat rejection takes place while keep temperature(Th) is kept constant.
Reverse carnot cycle efficiency
The efficiency of reversed Carnot cycle is termed as Coefficient of performance.
COP is defined as the ratio of the desired output to the energy supplied.
![]()
Carnot refrigeration cycle| carnot refrigeration cycle efficiency | coefficient of performance carnot refrigeration cycle | carnot cycle refrigerator efficiency
The refrigeration cycle works on reversed Carnot cycle. The main objective of this cycle is to reduce the temperature of the heat source/ hot reservoir.
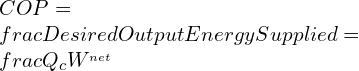
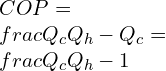
Application: Air- conditioning, refrigeration system
Carnot cycle heat pump
The heat pump works on reversed Carnot cycle. The main objective of the Heat pump is to transmit heat from one body to another, most from lower temperature body to higher temperature body with the help of supplied work.
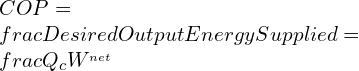
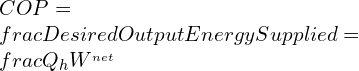

![]()
Comparison of carnot and rankine cycle | difference between carnot and rankine cycle
Comparison:
| Parameter | Carnot cycle | Rankine cycle |
| definition | Carnot cycle is an ideal thermodynamic cycle that works under two thermal reservoirs. | Rankine cycle is a practical cycle of the steam engine and turbine |
| T-S diagram |  |
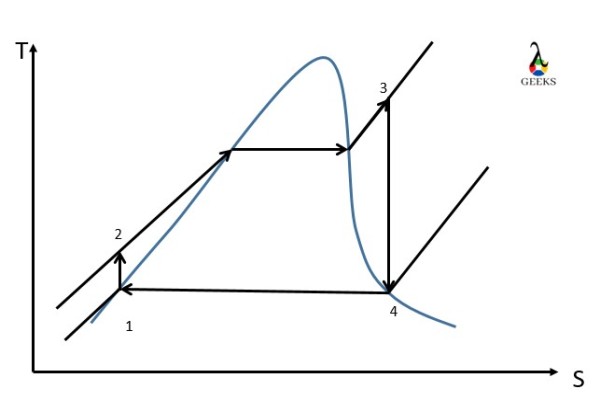 |
| Heat addition and rejection | Heat addition and rejection take place at a constant temperature.(isothermal) | Heat addition and rejection take place at constant pressure (isobaric) |
| Working medium | The working medium in Carnot is atmospheric air. Single-phase system | The working medium in Carnot is water/steam. Handles two phases |
| Efficiency | Carnot efficiency is maximum among all cycles. | Rankine efficiency is less than Carnot. |
| application | Carnot cycle is used for designing of heat engine. | Rankine cycle is used for designing of steam engine/turbine. |
Difference between otto cycle and carnot cycle
| Parameter | Carnot cycle | Otto Cycle |
| definition | Carnot cycle is an ideal thermodynamic cycle that works under two thermal reservoirs. | Otto cycle is an ideal thermodynamic combustion cycle. |
| T-s diagram |  |
 |
| Processes | Two isothermal and two Isentropic | Two isochoric and two isentropic. |
| Heat addition and rejection | Heat addition and rejection take place at a constant temperature.(isothermal) | Heat is produced at constant volume and rejected at the exhaust. No external heat source is required. It produces heat by chemical processes that are the combustion of a petrol air mixture with help spark plug at high pressure. |
| Working medium | The working medium in Carnot is atmospheric air. | Petrol and air mixture is used. |
| Efficiency | Carnot efficiency is maximum among all cycles. | Otto cycle has Less efficiency than Carnot cycle. |
| application | Carnot cycle is used for designing of heat engine. | Otto cycle is used for internal combustion SI engine. |
Carnot cycle irreversible
When the Carnot cycle runs on Adiabatic and not on reversible adiabatic, it comes under the category of irreversible Carnot cycle.
Entropy is not maintained constant in Process 2-3 and 4-1, (ds is not equal to zero)
as shown below:

Work produce under irreversible cycle is comparatively less than reversible Carnot cycle
Hence, the Efficiency of the irreversible Carnot cycle is less than the reversible Carnot cycle.
Why Carnot cycle is reversible
According to Carnot, the Carnot cycle is a theoretical cycle that provides maximum efficiency. To get this maximum efficiency, we must eliminate all the losses and consider the system reversible.
If we consider any losses, the cycle will fall under the irreversible category and would not provide maximum efficiency.
Carnot cycle volume ratio
![Rendered by QuickLaTeX.com left [ frac{T_{1}}{T_{4}} right ] =left [ frac{V_{4}}{V_{1}} right ]^{gamma -1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-b5071dcab86067c0c30e711d1eddf574_l3.png)
&
![Rendered by QuickLaTeX.com left [ frac{T_{2}}{T_{3}} right ] =left [ frac{V_{3}}{V_{2}} right ]^{gamma -1}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-91e649eb42cb3f5904f72969eb3cb41a_l3.png)
but![]()
![]()
![]()
Hence the volume ratio is maintain constant.
Advantages of carnot cycle
- Carnot cycle is an ideal cycle that gives maximum efficiency among all the cycle available.
- Carnot cycle helps in designing the actual Engine to get maximum output.
- It helps to decide the possibility of any cycle to build. As long as the Engine maintains efficiency less than Carnot, the Engine is possible; otherwise, it is not.
Disadvantages of Carnot cycle
- It is impossible to supply heat and reject the heat at a constant temperature without phase change in the working material.
- It is impossible to construct a reciprocating heat engine to travel a piston at a very slow speed from the beginning of the expansion to the middle to satisfy isothermal expansion and then very rapid to help the reversible adiabatic process.
Why Carnot cycle is not used in power plant
Carnot cycle has isothermal to adiabatic transmission. Now to carry out isothermal, we have to either make the process very slow or deal with phase change. Next is reversible adiabatic, which must be carried out quickly to avoid heat interaction.
Hence making the system difficult to construct as the half-cycle run very slow and the other half run very fast.
carnot cycle application | carnot cycle example | application of carnot cycle in daily life
Thermal devices like
- heat pump: to supply heat
- Refrigerator: to produce cooling effect by removal of heat
- Steam turbine: to produce power i.e. thermal energy to mechanical energy.
- Combustion engines: to produce power i.e. thermal energy to mechanical energy.
Carnot vapor cycle | carnot vapour cycle
In Carnot vapor cycle steam is working fluid
| Process 1-2: Isothermal expansion | Heating of fluid by keeping temperature constant in the boiler. |
| Process 2-3: Reversible adiabatic expansion | Fluid is expanded isentropically i.e. entropy constant in a turbine. |
| Process 3-4: isothermal compression | Condensation of fluid by keeping temperature constant in the condenser. |
| Process 4-1: Reversible Adiabatic Compression | Fluid is compressed isentropically i.e. entropy constant and brought back to original state. |
Its impracticalities:
1) It is not difficult to add or reject at constant temperature from two phase system, since maintaining it at constant temperature will fix up the temperature at saturation value. But limiting the heat rejection or absorption process to the mixed phase fluid will affect the thermal efficiency of the cycle.
2) The reversible adiabatic expansion process can be achieved by a well-designed turbine. But, the quality of the steam will reduce during this process. This is not be favorable as turbines cannot handle steam having more than 10% of liquid.
3) The reversible adiabatic compression process involves the compression of a liquid – vapour mixture to a saturated liquid. It is difficult to control the condensation process so precisely to achieve state 4. It is not possible to design a compressor that will handle mixed phase.
carnot cycle questions | carnot cycle problems | carnot cycle example problems
Q1.) Cyclic heat engine operators between source at 900 K and sink at 380 K. a) what will be the efficiency? b) what will be heat rejection per KW net ouput of the engine?
Ans = given: ![]() and
and ![]()
![]()
![]()
![]() %
%
b) Heat reject (Qc) per KW net output
![]()
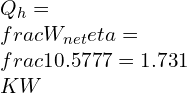
![]()
Heat reject per KW net output = 0.731 KW
Q2.) Carnot engine working at 40% efficiency with heat sink at 360 K. what will be temperature of heat source? If efficiency of the engine is increased to 55%, what will be the effect on temperature of heat source?
Ans = given : 
![]()
![]()
![]()
If ![]()
![]()
![]()
Q3.) A Carnot engine working with 1.5 kJ of heat at 360 K, and rejecting 420 J of heat. What is the temperature at the sink?
Ans = given: Qh=1500 J, Th= 360 K , Qc= 420 J
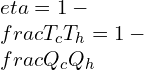
![]()
![]()
![]()
![]()
FAQ
What is a practical application of a Carnot cycle
- heat pump: to supply heat
- Refrigerator: to produce cooling effect by removal of heat
- Steam turbine: to produce power i.e. thermal energy to mechanical energy.
- Combustion engines: to produce power i.e. thermal energy to mechanical energy.
carnot cycle vs stirling cycle
Stirling, the Carnot cycle’s isentropic compression and isentropic expansion process are substituted by a constant volume regeneration process. The other two methods are the same as the Carnot cycle it isothermal heat addition and rejection.
What is the difference between a Carnot cycle and a reversed Carnot cycle
Simple carnot cycle works as power developing while reversed carnot work as power consuming.
Carnot cycle is used to design heat engine, while reversed cycle is used to design Heat pump and refrigeration system.
Why carnot cycle is more efficient than any other ideal cycles like otto diesel brayton ideal VCR
Carnot cycle work between two thermal reservoirs (Th & Tc), and its efficiency depends only on this temperature and doesn’t depend on the fluid type. That is Carnot’s cycle efficiency is fluid independent.
Carnot holds a maximum efficiency of all the engines performing under the same thermal reservoir as Carnot cycle work reversible, making assumptions of eliminating all the losses and making cycle a frictionless cycle, which is never possible in practice.
What is the net change in entropy during a Carnot cycle
Net change in entropy during a Carnot cycle is zero.
why carnot cycle is not possible
Carnot cycle has isothermal to adiabatic transmission. Now to carry out isothermal, we have to either make the process very slow or deal with phase change.
Next is reversible adiabatic, which must be carried out quickly to avoid heat interaction.
Hence making the system difficult to construct as the half-cycle run very slow and the other half run very fast.
why is the carnot cycle the most efficient
Carnot cycle work between two thermal reservoirs (Th & Tc), and its efficiency depends only on this temperature and doesn’t depend on the fluid type. That is Carnot’s cycle efficiency is fluid independent.
Carnot holds a maximum efficiency of all the engines performing under the same thermal reservoir as Carnot cycle work reversible, making assumptions of eliminating all the losses and making cycle a frictionless cycle, which is never possible in practice.
Why does the Carnot cycle involve only the isothermal and adiabatic process and not other processes like isochoric or isobaric
The main aim of Carnot Cycle is to achieve maximum efficiency, which leads to make system reversible, so to make system reversible no heat interaction process should me maintain, i.e adiabatic process.
And to get maximum work output we use Isothermal process.
How is the Carnot cycle related to a Stirling cycle?
Stirling, the Carnot cycle’s isentropic compression and isentropic expansion process are substituted by a constant volume regeneration process. The other two methods are the same as the Carnot cycle it isothermal heat addition and rejection.
What will happen with efficiency of two Carnot engine works with same source and sink?
Efficiency will be the same, as Carnot cycle efficiency is only dependent on the temperature of the source and sink.
Combination of Carnot cycle and Carnot refrigerator
The work output of Carnot heat engine supplied as work input for Carnot refrigeration system.
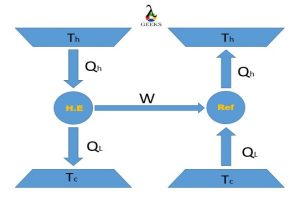
Is it necessary that refrigerators should only work on Carnot cycle?
To get the maximum Coefficient of performance (COP), theoretically we net refrigeration cycle to work on Carnot.
The temperature of two reservoirs of a Carnot engine are increased by same amount How will be the efficiency be affected?
The increase in temperature of both reservoirs in same will tend to decrease in efficiency
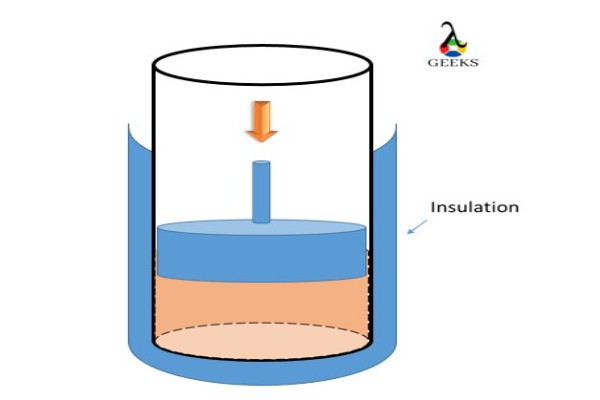
Uses of stand in Carnot cycle?
The stand is used to carry out an adiabatic process. It is made up of non-conduction material.
Important results for Carnot engine cycle?
Any number of engines working under the Carnot principle and having the same source and sink will have the same efficiency.
Terminal of Carnot engine?
Carnot engine will consist of: Hot reservoir, Cold sink & Insulating stand.
Definition of insulating stand which is one of the part of Carnot’s engine?
The stand is used to carry out an adiabatic process, and it is made up of non-conduction material.
For more Articles regarding Mechanical & Thermal, do visit our Home page.

The lambdageeksScience Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.