In this article “Ideal gas examples” and ideal gas examples related facts will be discuss. Ideal gas examples are based on law of ideal gas. But in practical life ideal gas is not present in the universe.
3+ Ideal Gas Examples are listed below,
Example 1:-
Calculate the amount of density for the Nitrogen gas at the pressure of 256 Torr and 25 degree centigrade temperature.
Solution: – Given data are,
P = 256 Torr = 256 Torr x 1 atm/760 Torr = 0.3368 atm
V =?
T = (25 + 273) K = 298 K
n =?
Now we apply the formula for ideal gas,
PV = nRT ………. eqn (1)
So, we also can write the density is,
ρ = m/v ………. eqn (2)
Where,
ρ = Density of the ideal gas
m = Mass of the ideal gas
v = Volume of the ideal gas
Now, m = M x n ………. eqn (3)
Where,
m = Mass
M = Molar mass
n = Moles
From eqn (2) and eqn (3) we get,
ρ = m/v …… (4)
Arranging the eqn (2) and eqn (3) we get,
ρ = M x n/V ……eqn(5)
ρ/M = n/V……eqn(6)
Now applying the equation of ideal gas,
PV = nRT
n/V =ρ /M ……eqn(7)
n/V = P/RT ……eqn(8)
From eqn (6) and eqn (8) we get,
ρ/M} = P/RT ……eqn(9)
Isolate density,
ρ = PM/RT……eqn(10)
ρ = (0.3368 atm)(2 x 14.01gram/mol)/(0.08206 L*atm*mol-1*K-1 )(298 K)
ρ = 0.3859 gram / mol
The amount of density for the Nitrogen gas at the pressure of 256 Torr and 25 degree centigrade temperature is 0.3859 gram / mol.
Image Credit – Wikimedia Commons
Example 2:-
A container which is filled with the Neon gas. The amount Neon in container is 5.00 Litre that time the temperature is 26 degree Centigrade at 750 mm Hg. A carbon dioxide vapour is now added to the container. The quantity of carbon dioxide added to the container is 0.627 gram.
Now determine these factors,
Partial pressure for Neon in atm.
Partial pressure for carbon dioxide in atm.
Total pressure present in the container.
Solution: – Given data are,
P = 750 mm Hg -> 1.01 atm
V = 5.00 Litre
T = (26 + 273) K= 299 K
nne =?
nco2 =?
For Carbon dioxide the number of mole is,
nco2 = 0.627 gram CO2 = 1 mol/44 gram = 0.01425 mol CO2
Now for Neon the number of mole is,
nNe= 0.206 mol Ne
Before adding the carbon dioxide to the container we can get only pressure for neon. So the partial pressure for neon is definitely the amount of pressure is already discussed in question.
Now for the carbon dioxide,
Using the equation of ideal gas equation we can write,
For the both Carbon dioxide and Neon Temperature, volume and gas constant remain same.
So,
1.01 atm/0.206 mol Ne = PCO2/0.01425 mol CO2
PCO2 = 0.698 atm
Total pressure,
Ptotal = PNe + PCO2
Ptotal= 1.01 atm + 0.698 atm
Ptotal = 1.708 atm
Partial pressure for Neon is 1.01 atm.
Partial pressure for carbon dioxide 0.698 atm.
Total pressure present in the container is 1.708 atm.
Example 3:-
Determine the amount of volume.
In a glass container carbon dioxide gas is present. The temperature of the carbon dioxide gas is 29 degree centigrade, pressure is 0.85 atm and the mass of the carbon dioxide gas is 29 gram.
Solution: – Given data are,
P = 0.85 atm
m = 29 gram
T = (273 + 29) K = 302 K
The mathematical form of the ideal gas is,
PV = nRT ……..eqn (1)
Where,
P = Pressure for the ideal gas
V = Volume for the ideal gas
n = Molar number for the ideal gas
R = Universal gas constant for the ideal gas
T = Temperature for the ideal gas
If in a matter M denoted as molar mass and mass of a matter denoted as m then the total number of moles for that particular matter can be expressed s,
n = m/M ……..eqn (2)
Combine the ……..eqn (1) and ……..eqn (2) we get,
PV = mRT/M ……..eqn (3)
We know the value of molar mass for carbon dioxide is,
M = 44.01 gram/ mol
From eqn (3) we can write,
V = mRT/M = 29 gram x 0.0820574 L*atm*mol-1*K-1 x 302/44.01 gram/mol x 0.85 atm
V = 19.21 Litre
In a glass container carbon dioxide gas is present. The temperature of the carbon dioxide gas is 29 degree centigrade, pressure is 0.85 atm and the mass of the carbon dioxide gas is 29 gram. Then the amount of volume is 19.21 Litre.
Image Credit – Wikipedia
Real gas vs. Ideal gas:
Ideal gases follow the law of gas in a particular constant condition but real gas does not follow the law of gas in a particular constant condition. In practical life ideal gas is not exist but real gas is exist.
The major points are derive about the difference between real gas and ideal gas,
| Parameter | Ideal gas | Real gas |
| Definition | The gas which are follow the law of gas at particular condition of constant pressure and temperature | The gas which are not follow the law of gas at particular condition of constant pressure and temperature |
| Movement of particles | The particle present in the ideal gas is free to move and the particle does not attend in interparticle interaction. | The particle present in the real gas is not free to move and compete with each other, the particle attend in interparticle interaction. |
| Volume occupied | Negligible | Not negligible |
| Pressure | High pressure is present | Lower pressure than the ideal gas pressure |
| Force present | Intermolecular force of attraction is not present | Intermolecular force of attraction is present |
| Formula | The formula which ideal gas is follow, PV = nRT Where, P = Pressure V = Volume n = Amount of substance R = Ideal gas constant T = Temperature | The formula which real gas is follow, (P + an2/V2)(V – nb) = nRT Where, P = Pressure a = Parameter which need to determine empirically for individual gas V = Volume b = Parameter which need to determine empirically for individual gas n = Amount of substance R = Ideal gas constant T = Temperature |
| Availability | Not exist | Exist |
Read more about Isothermal process : Its’s all Important facts with 13 FAQs
Frequent asked questions:-
Question: – Derive the limitations of ideal gas.
Solution: – The limitations of ideal gas is listed below,
- Ideal gas could not work in high density, low temperature and high pressure
- Ideal gas not applicable for heavy gases
- Ideal gas not applicable strong intermolecular forces.
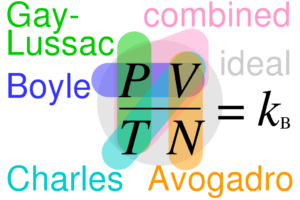
Image Credit – Wikipedia
Read more about Gauge Pressure : It’s Important Properties with 30 FAQs
Question: – Write down the assumptions about the ideal gas.
Solution: – Actually in our surrounding ideal gas is no present. The law of ideal gas is a simple equation by which we can understand the relation between pressures, volume and temperature for gases.
The assumptions about the ideal gas is listed below,
- The gas particles of ideal gas have negligible volume.
- The size of the gas particles of ideal gas is equal and they don’t have intermolecular force.
- The gas particles of ideal gas have follows the law of motion of Newton’s.
- There is no loss of energy.
- The gas particles of ideal gas have elastic collision.
Question: – Derive the different form equation for ideal gas.
Solution: – Ideal gas formula actually combination of Boyle’s law, Avogadro’s law, Charle’s law and Gay Lussac’s law.
The different form equation for ideal gas is briefly summarize below,
Common form of ideal gas:
PV = nRT = nkbNAT = NkBT
Where,
P = Pressure for the ideal gas measured in Pascal
V = Volume for the ideal gas measured in cubic meter
n = The total of ideal gas which is measured in moles measured in mole
R = Gas constant for the ideal gas which value is 8.314 J/K.mol = 0.0820574 L*atm*mol-1*K-1
T = Temperature for the ideal gas measured in Kelvin
N = The total number of the ideal gas molecules
kb = Boltzmann constant for the ideal gas
NA = Avogadro constant
Molar form of ideal gas:
Pv = Rspecific T
P = Pressure for the ideal gas
v = Specific volume for the ideal gas
Rspecific = Specific gas constant for the ideal gas
T = Temperature for the ideal gas
Statistical form of ideal gas:
P = kb/μ mμρ T
Where,
P = Pressure for the ideal gas
kb = Boltzmann constant for the ideal gas
μ= Average partial mass for the ideal gas
mμ = Atomic mass constant for the ideal gas
ρ = Density for the ideal gas
T = Temperature for the ideal gas
Combined gas law:-
PV/T = k
P = Pressure
V = Volume
T = Temperature
k = Constant
When same matter in present two different condition that time we can write,
P1V1/T1 = P2V2/T2
Question: –Derive the law of Boyle.
Solution: – Boyle’s law is a gas law. The gas law of Boyle’s derive that the pressure exerted by a gaseous substance(of a given mass, kept at a constant temperature) is inversely proportional to the volume occupied by it.
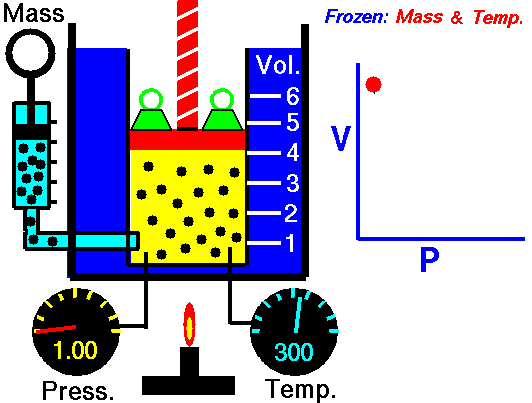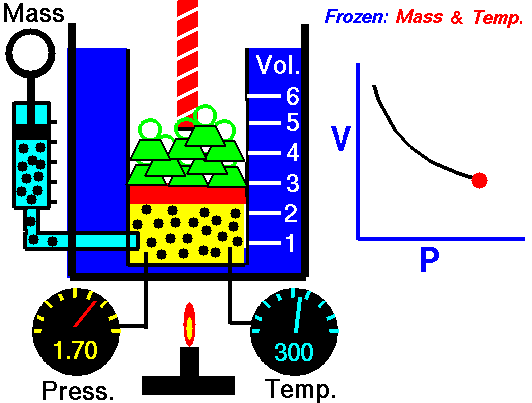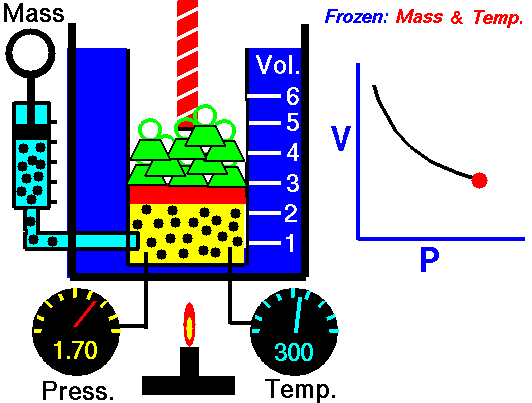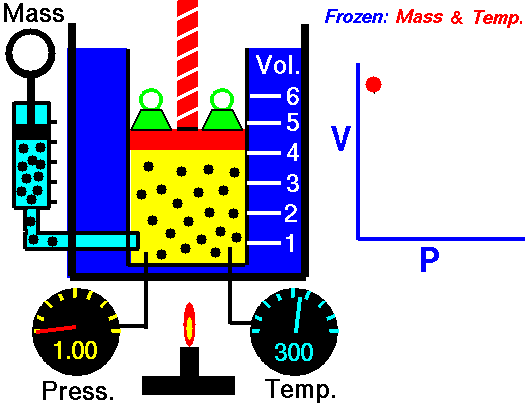
Image Credit – Wikimedia
In other words, the pressure and volume of a gas are indirectly proportional to each other to the temperature and the quantity of gas are kept constant.
The gas law of Boyle’s can be expressed mathematically as follows:
P1V1 = P2V2
Where,
P1 = The initial pressure exerted by the gaseous substance
V1 = The initial volume occupied by the gaseous substance
P2 = The final pressure exerted by the gaseous substance
V2 = The final volume occupied by the gaseous substance
This expression can be obtained from the pressure-volume relationship suggested by Boyle’s law. For a fixed amount of gas kept at a constant temperature, PV = k. Therefore,
P1V1= k (initial pressure x initial volume)
P2V2 = k (final pressure x final volume)
∴ P1V1 = P2V2
As per Boyle’s law, any change in the volume occupied by a gas (at constant quantity and temperature) will result in a change in the pressure exerted by it.
Hi..I am Indrani Banerjee. I completed my bachelor’s degree in mechanical engineering. I am an enthusiastic person and I am a person who is positive about every aspect of life. I like to read Books and listen to music.