Contents: Thermal stress
Thermal stress definition
“Thermal stress is the stress in the material due to the temperature change and this stress will lead to plastic deformation in the material.”
Thermal stress equation | Thermal stress formula:
The stress induced due to temperature change:
σ=Eα∆T
It is documented that changes in temp will cause elements to enlarge or contract and if increment in the length of a uniform bar of length L and ∆L is the change f length because of its temp has been changed from T0 to T then ∆L could be represented as
∆L = αL (T – T0)
where α the coefficient of thermal expansion.
Thermal stress unit:
SI unit: N/m^2
Thermal hoop stress:
Stress generated for thermal change.
Let us assume a thin tire having diameter ‘d’ has fitted on to the wheel of diameter ‘D’.
If the temp of the tire has been changed in such a way that the diameter of the tire is increased and it has become equal to the diameter of the wheel and if temp of the tire is decreased to original, the tire diameter tries to return to its original dimension and because of this process a stress has been generated in the tire material. This stress is an example of Thermal hoop stress.
so, the temperature difference=t degree.
thermal strain=D-d/d
Hoop stress= e. E
Hence,
Hoop stress=(D-d).E/d
Thermal Analysis:
Thermal stress analysis in ANSYS Workbench| Ansys thermal stress| Abaqus thermal stress analysis:
The objective of the thermal analysis is to study the behavior of material after applying thermal loading and thermal stress. To study heat transfer within an object or between objects and thermal analysis is utilized for temp measurement, thermal gradient, and warmth flux distributions of the body.
Types of thermal analysis:
There are two sorts of thermal analysis:
Steady-state thermal analysis:
Steady-state thermal analysis aims to seek out the temperature or heat flux distribution in structures when an equilibrium is reached.
Transient thermal analysis:
Transient thermal analysis sets bent determine the time history of how the temperature profile and other thermal quantities change with time
Also, thermal expansion or contraction of engineering materials often results in thermal stress in structures, which may be examined by conducting thermal-stress analysis.
Thermal stress importance:
Thermal Stress Analysis is essential to determine the thermal stresses due to temperature changes in structures. We can proceed to
Solve Equation K. T = q
⦁ To obtain the temperature change fields initially apply the temperature change ΔT as initial strain
⦁ The stress-strain relations due to temperature change were determined by first using 1D case materials.
The thermal strain (or initial strain): εo = αΔT
Case study with ANSYS Workbench:
Material: Aluminum
k = 170 W/(m · K)
ρ = 2800 kg/m3;
c = 870 J/(kg · K)
E = 70GPa;
v = 0.3
α = 22 × 10–6/°C
Boundary conditions: Air temperature of 28°C; h = 30 W/(m2 · °C). Steady state: q′ = 1000 W/m2 on the base.
Initial conditions: Steady-state: Uniform temperature of 28°C.
- Start ANSYS workbench
- Create a steady-state thermal analysis system:
- Add new material: provided with all given data.
- Launch design modeler program.
- Create body
- Launch the steady-state thermal program
- Generate mesh
- Apply boundary conditions.
- Solve and retrieve results.
Thermal analysis of water-cooled engine:
The following steps are followed after finalizing the engine specification.
- Design of water-core and head-core system.
- Design of liner system. (Based on its parameters like bore, stoke and thickness etc.)
- Design of water pump and installation.
- Cooling system design and it’s subsystems such as radiators, fans, oil-cooler design.
Aspects of thermal analysis of engine block:
- Cylinder head valve bridge water velocities (design of cross section in headwater core).
- Piston and valve cooling aspect analysis.
- Liner cavitation analysis.
- Cylinder head gasket design analysis.
Thermal stress weathering:
Thermal stress weathering is the thermal fracture is a mechanical breakdown of rock due to thermal expansion or contraction caused by the change in temperature.
Effects of thermal stresses in weld joints:
Thermal stress in welding and in bonded joints:
The temperature of the body is uniformly1 increased,
The normal strain of the body is,
x = y = z = α(T)
Here,
α is the co-efficient of thermal expansion.
T is the temp variation.
The stress is represented as
σ1 =− E =−α(T)E
in a similar means, if a consistent flat plate is restrained at the sides and also subjected to a constant temp rise.
σ2 =− α(T)E(1−ν)
The stresses σ1, σ2 are called thermal stresses. They arise due to a natural process during a clamped or restrained member.
Thermal stress equation for cylinder| Thermal stress in thick walled cylinder:

Thin-walled Cylinder:
![]()

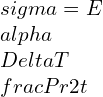
Thick-walled cylinder:
![]()
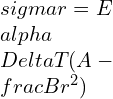
Thermal stress relief process:
The process of heat treatment is used to decrease the residual thermal stresses in the materials.
First, the part needs to be heated at 1100-1200degree F, leading to relief of the stresses and hold it there for an hour per inch of thickness, and then left to chill in tranquil air at temperature.
Thermal Expansion:
When a solid material experiences an increase in temp or temperature difference, the volume of the structure of solid material increases, this phenomenon is acknowledged as thermal expansion and this volume increment will lead to an increase in stress of the structure.
Coefficients of Thermal Expansion:
- (Linear Mean Coefficients for the Temperature Range 0–100°C):
- Aluminum: 23.9(10)−6 Brass, cast: 18.7(10)−6
- Carbon steel: 10.8(10)−6 Cast iron: 10.6(10)−6
- Magnesium :25.2(10)−6 Nickel steel: 13.1(10)−6
- Stainless steel: 17.3(10)−6 Tungsten: 4.3(10)−6
Thermal stresses in composite bars formula:
Thermal stress in compound bars:
Compound bars and composite bars, when undergoing temperature change, tend to contract or expand. Generally thermal-strain is a reversible process so material will return to its actual shape when the temp also decreased to its actual value, though there are some materials that does not behave according to thermal expansion and contraction.
Bars in series:
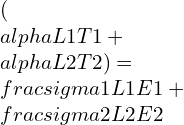
Thermal stress and strain:
Thermal stress and strain definition:
The stress produced due to change in temperature is known as thermal-stress.
Thermal stress=α(t2-t1).E
The strain corresponding to thermal stress is known as thermal strain.
Thermal strain=α(t2-t1)
Thermal stress example:
Thermal stress on rails.

Thermal stress applications:
Engine, radiator, exhaust, heat exchangers, power plants, satellite design, etc.
Thermal residual stress:
Differences within the temperatures during the manufacturing and dealing environment are the most explanation for thermal (residual) stresses.
Thermally induced stress
σ=E ∆L/L
Thermal stress calculation in pipe:
Pipes expand and contract due to variable temperatures.
The coefficient of thermal expansion shows the rate of thermal expansion and contraction.
Factors affecting thermal stress:
- Temperature gradient.
- Thermal expansion contraction.
- Thermal shocks.
Thermal stress is dependent on the thermal expansion coefficient of the material and if change of temp is more, then the stress will be more too.
Modulus of Elasticity in thermal expansion:
If the bar is prevented from completely expanding within the axial direction, then the typical compressive stress-induced is
σ=E ∆L/L
where E is the modulus of elasticity.
So the thermal stress needed is,
α = –αE (T – T0)
In general, in an elastic continuum, the natural process is non-uniform throughout and this is a function of time and space usually.
therefore the space coordinates (x, y, z), i.e. T = T(t, x, y, z).
Limitations of thermal stress analysis:
The body into account could also be restrained from expansion or movement in some regions, and external tractions could also be applied to other regions and stress calculation under such circumstances may be quite complex and difficult to compute. This also having following case is constrained.
- Thin circular disks with equal temp difference.
- Long circular cylinder. (This could be hollow and solid)
- Sphere having radial temperature variation. (This could be hollow and solid)
- Straight beam of arbitrary cross section.
- Curved beam case.
Thermal stress problems and solutions:
1) A steel rod of length 20m having temperature 10-degree Celsius. Temperature is raised to 50 degrees Celsius. Find the thermal stress produced.
Given: T1=10, T2=50, l=20, α=1210^-6, E=20010^9
Thermal stress=α(t2-t1).E
=1210^-6(50-10)20010^9
=9610^6 N/m^2.
FAQ/Short Notes:
What is the effect of thermal stresses ?
This has a significant effect on the materials and can lead to fracturing, and plastic deformation depends on the temperature and material type.
Which material can be used as thermal insulator and why ?
Cellulose. Because it blocks air better than fiberglass and has low thermal conductivity.
What are the three most common types of heat stress ?
Types of heat stress commonly used:
- Tangential
- radial
- axial.
How to calculate thermal stresses in glass ?
Thermal stress in glass is varied at different temperatures.
Thermal stress and deformation:
Thermal deformation is the property of a substance to expand with heating and contract with cooling, normally kind of deformation because of temp change and this is stated by linear expansion coefficient α.
α=ΔL/L×Δt
Here,
⦁ α is that the linear expansion coefficient of a substance (1/K).
⦁ ΔL is that the expansion or contraction value of a specimen(mm).
⦁ L is the actual length.
⦁ Δt is the temperature difference measured in Kelvin or degree Celsius.
The higher the thermal expansion coefficient, gives higher the value of thermal deformation.
Thermal stress weathering:
Thermal stress weathering is the thermal fracture a, mechanical breakdown of rock due to thermal expansion or contraction caused by the change in temperature.
What is the formula for thermal expansion stress and strain?
Thermal stress formula:
α(t2-t1). E
Thermal strain formula:
α(t2-t1).
What is the relationship between thermal stress and thermal strain?
Thermal stress and Thermal strain in 2D-3D cases:
Temperature changes do not yield shear strains. In both 2-D and 3-D cases, the entire strain is often given by the following vector equation:
ε = εe + εo
And the stress-strain relation is given by
σ = Eεe = E(ε − εo).
Which parameters have to be defined for isotropic materials for structural and thermal analysis in ANSYS?
- Isotropic Thermal Conductivity
- Material
- Heat transfer coefficient
If strain causes stress, then in free thermal expansion why is stress absent even though there is thermal strain:
Stress is the internal resistance when applied to an external load. When the material is undergone any load or force, the material tries to resist the force leading to stress generation.
If the material is undergoing free thermal expansion, the material won’t experience any internal stress leading to no stress generation.
What are some examples of thermal expansion in everyday life?
⦁ Thermometers
⦁ Electrical pylons
⦁ Bimetallic strips
⦁ Railway lines.
What is the application of thermal diffusivity in real world ?
⦁ Insulation.
Does Hooke’s law fails in case of thermal expansion ?
Hook’s law applies to a thermal expansion only when there is a restriction to the object undergoing thermal stress. If there is no applied stress, there won’t be any expansion and Hook’s law states that stress is directly proportionate to strain.
Why does copper have such a low thermal expansion ?
If the coefficient of thermal expansion is nearly equal for both steel and concrete, then why is a concrete structure considered a better firefighter
If the coefficient of thermal expansion is almost equal for both steel and concrete, then why is a concrete structure considered a better firefighter:
A concrete structure has low thermal conductivity and does not heat up quickly. Hence If the coefficient of thermal expansion is nearly equal for both steel and concrete, then why is a concrete structure considered a better firefighter.
Why we do static structure buckling modal thermal nonlinear fatigue based on stress and strain in Ansys?
It is a finite element method. To predict the exact and accurate strength of the structures, nonlinear analysis is performed. It takes into the changes in the parameters as the load is applied.
What does thermal capacity mean?
The thermal capacity of the material is the amount of heat required to change the material temp by unit mass of material.
What is the difference between the thermal expansion coefficients of steel and copper?
Thermal expansion coefficients 20 °C (x10−6 K−1)
copper=17
steel=11-13.
What is the use of thermal conductivity?
Thermal conductivity is that the ability of an object to conduct heat. It measures the amount of heat that transfers through the material.
Do any materials have a zero thermal expansion coefficient?
There exist few materials which have zero thermal expansion coefficient.
Mesopores.
Hooke’s law| Hooke’s law for thermal stress:
σth = Eϵth
If the material is undergoing free thermal expansion, the fabric won’t experience any internal stress leading to no stress generation.
What is thermal shrinkage in concrete:
When the hot concrete cooled down at ambient temperature, the volume of the concrete reduces; this process is called thermal contraction or thermal shrinkage in concrete.
What is the best simulation and analysis software for mechanical engineering mainly structural analysis and dynamic analysis thermal not required?
Ansys, Nasttan, Abaqus, 1-deas NX, etc.
Thermal stress-strain: Why the bar does not bend when it is heated from the bottom with one end alone fixed:
Thermal stresses in cantilever beams:
Case1: Fixed free bar:
If a rod is heated through temp rise, the rod will tend to expand by amount εo=αLΔT, if the rod is free at other ends, undergoes thermal expansion ε=αΔT,
ε = εo, εe = 0,
σ =E(ε- εo)=E(αΔT- αΔT)= 0
That is, there is no thermal stress in this case.
Case2: Fixed-fixed bar
If there’s a constraint on the right-hand side, that is, the bar cannot expand to the proper, then we have:
ε = 0,
εe =−εo
σ=E(ε-εo)=E(0- αΔT)= = −αΔT,
σ = −EαΔT
Thus, thermal stress exists.
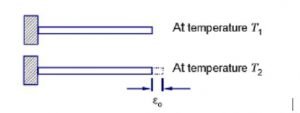
Shear strains do not change only normal strains change.
If the temperature changes, the size of the body changes, though it will not change the shape of the body. So, considering this fact, the shear-strain of the body does not change.
For more articles, click here.

I am Sulochana. I am a Mechanical Design Engineer—M.tech in design Engineering, B.tech in Mechanical Engineering. I have worked as an intern at Hindustan Aeronautics limited in the design of the armament department. I have experience working in R&D and design. I am skilled in CAD/CAM/CAE: CATIA | CREO | ANSYS Apdl | ANSYS Workbench | HYPER MESH | Nastran Patran as well as in Programming languages Python, MATLAB and SQL.
I have expertise on Finite Element Analysis, Design for Manufacturing and Assembly(DFMEA), Optimization, Advanced Vibrations, Mechanics of Composite Materials, Computer-Aided Design.
I am passionate about work and a keen learner. My purpose in life is to get a life of purpose, and I believe in hard work. I am here to excel in the field of Engineering by working in a challenging, enjoyable & professionally bright environment where I can fully utilize my technical and logical skills, constantly upgrade myself & benchmark against the best.
Looking forward to connect you through LinkedIn –