Strength of Materials: 27 Complete Quick Facts

There are two types of body: rigid body and deformable-body. Distance between any two points remains constant with force applied on a body is known as a rigid body and the body in which this distance change is known as a deformable body. Strength of material is the study of deformable bodies.  In this, we study the different properties of materials by applying force on it. Study of the strength of materials helps to select material for different applications according to their properties. Strength of Material is also referred as Mechanics of Material. Strength of Material includes stress, strain, stress-strain curve etc.

Engineering Stress

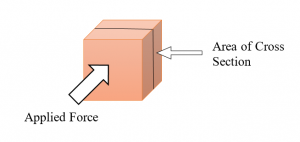
• Instantaneous load or force applied per unit original area of cross-section (Before any deformation) is known as engineering stress.
•  It is denoted by σ (sigma). SI unit of engineering stress is N/m² or Pascal (Pa).

Engineering Stress= (Force Applied)/ (Original Area)

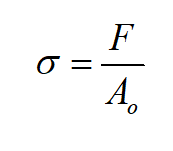
Click Here! for more details

Classification of Stress

Generally following engineering stresses are classified in strength of materials studies.

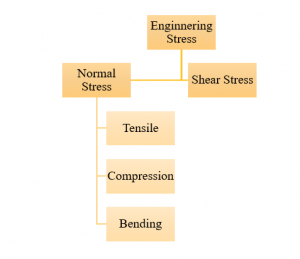
Normal Stress

• When the applied force is perpendicular to the given cross-section of the specimen (axial load), then the corresponding stress produced in the material is known as normal stress.
•  Many times force applied on the surface is not uniform; in that case, we take an average of the applied force.

Normal Stress= (Perpendicular component of Applied Force)/ Area

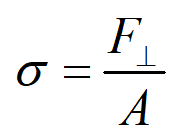
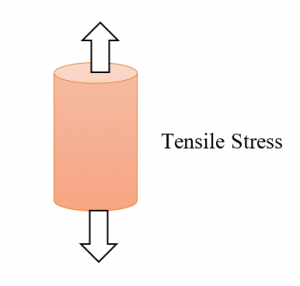
Tensile Stress

When the applied force is away from the material, then the Stress produced is known as tensile stress.

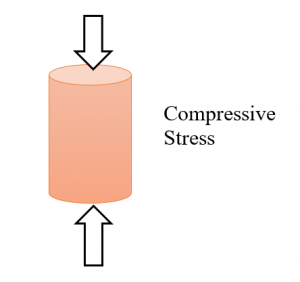
Compressive Stress

When the applied force is in towards the object, then the Stress produced is known as compression stress.

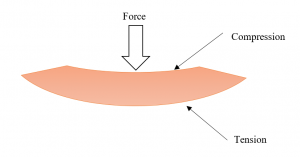
Bending Stress

• When force is applied on the beam-shaped material, the material’s top surface undergoes a compressive type of stress, and the bottom surface undergoes tension-type of Stress and middle of the beam remains neutral. Such stress is known as bending Stress.
•  It is also known as flexural Stress.

Shear Stress

When the applied force is parallel to the area on which it is applied, the Stress is known as shear stress.

Shear Stress Formula

Shear Stress= (Force imposed parallel to the upper and lower faces) / Area.

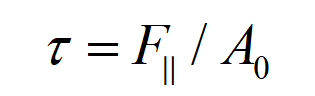
Tensile Stress vs Shear Stress

Tensile Stress	Shear Stress
The applied force is perpendicular to the surface.	The applied force is parallel to the surface.
It is denoted by σ.	It is denoted by τ.

Combined Stress Equation

While studying strength of materials in real-life examples, we can have cases in which more than one type of Stress is acting on the material, in that case, we need to have an equation which can combine different type of stresses

Following is the equation which combines shear and tensile stresses.

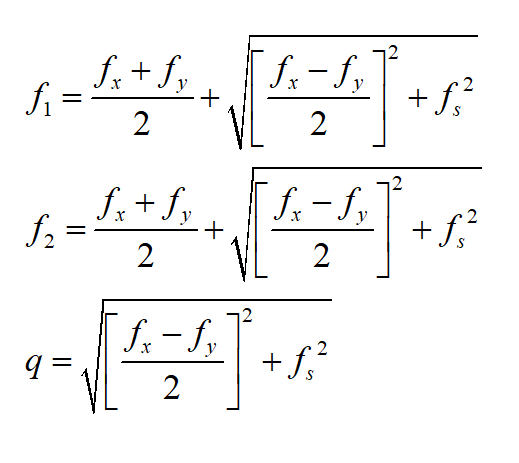
Where,

f_x= tensile or compressive stress in the x-direction

f_y= tensile or compressive stress in the y-direction

f_s= shear stresses acting on the faces in x and y-direction

f₁= maximum principle Stress

f₂= minimum tensile Stress

q= maximum shear stress

Stress Concentration Factor

• In the studies of Strength of Materials, many times the material on which we are applying Stress is not uniform. It may have some irregularities in its geometry or within the structure formed due to nicks, scratches holes, fillets, grooves, etc., which causes the concentration of stress to be very high at some point on the material known as stress concentration or stress riser/raiser.
• The degree of this concentration is expressed as the ratio of maximum Stress to reference Stress, where reference stress is total Stress within an element under the same loading conditions, without any concentration or discontinuity.

Stress Concentration Factor Formula:

Stress Concentration= maximum Stress / Reference Stress

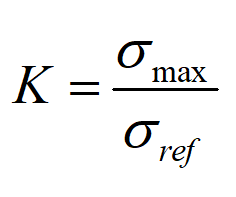
Click Here! for more details

Factor of Safety

• While studying strength of Materials, there are always some uncertainties in the measured values of stresses; therefore, the stress that we are going to consider for our use known as working stress (σ_w) is always less than the experimental value of stress. In most of the applications, we consider yield strength (σ_y).
• Working Stress is determined by reducing the yield strength by a factor; that factor is known as the factor of safety. So, the factor of safety is a ratio of yield strength to working stress. Its symbol is N. It is a unitless quantity.

            Factor of Safety= Yield Strength/ Working Stress

Engineering Strain

• Change in length at some instant of the material per unit original length (Before any application of force) is known as engineering strain.
•  It is denoted by ε (Epsilon) or γ (Gamma). It’s a unitless quantity.

              Engineering Strain= (Change in length)/ (Original Length)

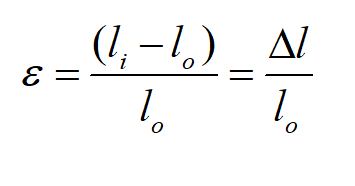
Poisson’s Ratio

• When tensile stress is applied to the material, there is elongation along the applied load axis and shortening along with perpendicular directions to the applied Stress. Thus, the strain produced in the applied stress direction is known as axial strain and the strain produced in the perpendicular direction the applied Stress is known as lateral strain or transverse strain.
• The ratioof the lateral strain and axial strain is known as Poisson’s Ratio. It is denoted by ʋ (nu). It is a very important constant for a given material.

            Poisson’s Ratio= – (Lateral Strain/ Axial Strain)

Let the applied load is in z-direction and strain produced in that direction is ε_x  and  material is isotropic and homogeneous ( ) then Poisson’s ratio is

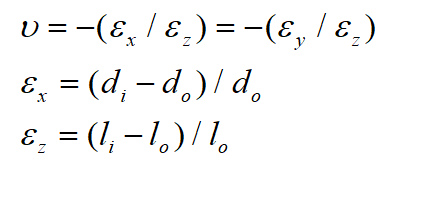
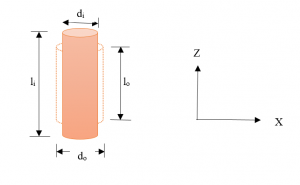
To learn in detail on Poisson’s Ratio Visit here

Stress-Strain Curve

• Plotting of stress to strain gives a considerable number of properties of the material in strength of materials study.
• The stress-strain curve is stress versus strain curve in which strain is on independent axis i.e., x-axis and stress is on dependent i.e. y-axis. It is an important characteristic of the material.
• On the load application, two types of deformation occur in the material depending upon the strain value, first is elastic deformation and second is plastic deformation.

True Stress-Strain Curve

It is a stress-strain curve in which true Stress is plotted against true strain. Both Stress and strain are based on instantaneous measurement. Hence, the instantaneous cross-section area is considered instead of original cross-section, and instantaneous length is considered instead of the original length.

Elastic Deformation

• Elastic deformation is the deformation in which material regains its original shape on the removal of the force.
• This region has a proportional limit, elastic limit, upper yield point and lower yield point.

Modulus of Elasticity | Hooke’s Law

• When this type of deformation occurs, the strain in the metal piece is nearly proportional to the stress; therefore, this deformation occurs as a straight line in Stress versus strain plot except for some materials like grey cast iron, concrete and many polymers.
• Stress is proportional to the strain through this relationship.

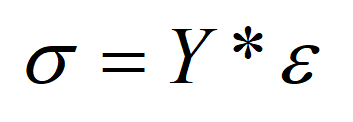
• This is known as Hooke’s Law, where Y the proportionality constant is known as Young’s Modulus or Modulus of Elasticity. It is also denoted by E. It is the slope of the stress-strain curve in the elastic limit. It is one of the most important law in the studies of strength of material.

Modulus of Elasticity Formula

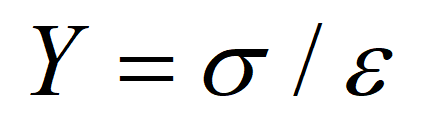
Its value is slightly higher for ceramics than metals and value is slightly lower for polymers than metals. Or most structures are required to have deformation only in the elastic limit; therefore, this region is quite important.

Plastic Deformation

• If the applied force is removed in this region, then the material does not regain its original shape.
• The deformation in the material is permanent.
• In this region, Hooke’s law is not valid.
• This region has ultimate tensile strength of materials and breaking point.

• There are some points on the curve around which type of deformation changes. These points are very important as they tell us about the limitations and ranges of material which are ultimately useful in material’s application.

Proportional Limit

• It is the point in the curve up to which Stress is proportional to the strain.
• When the material is stretched beyond the proportionality limit, stress is not proportional to the strain, but still, it shows elastic behaviour.

Elastic Limit

• It is the point in the curve up to which material shows elastic behaviour.
• After this point, plastic deformation in the material begins.
• Beyond the elastic limit, Stress causes the material to flow or yield.

Yield Point

It is the point where yielding of the material occurs; hence plastic deformation of material begins from this point.

What is Yield Strength?

• Stress corresponding to the yield point is known as yield strength—its resistance to its plastic deformation.
• Many times it is not possible to locate it precisely. The elastic-plastic transition is well-defined and very abruptly, termed as yield point phenomenon.

• Upper Yield Point: It is the point in the graph at which maximum load or Stress required to initiate the plastic deformation of the material.

• Lower Yield Point: It is a point at which minimum Stress or load is required to maintain the material’s plastic behavior.

• The upper yield point is unstable, but lower yield point is stable, so we use a lower yield point while designing the components.

Ultimate Strength Definition | Ultimate Stress Definition

• After yielding, as plastic deformation continues, it reaches a maximum limit known as ultimate Stress or ultimate strength.
• It is also known as Ultimate Tensile Strength (UTS) or tensile strength. It is the maximum stress that can be sustained by material in tension.
• All deformation up to this point is uniform, but at this maximum stress, small narrowing of material begins to form, this phenomenon is termed as ‘necking’.

Rupture Point | Fracture Point | Breaking Point

• Stress necessary to continue plastic deformation starts to decrease after ultimate strength and eventually breaks the material at a point known as rupture point or fracture point.
• The stress of the material at rupture point is known as ‘rupture strength’.

Stress-Strain curve for Brittle material

Stress-Strain Curve for Ductile Material

 Ref. – Stress-Strain

Important Questions and Answer related to Strength of Materials

What is engineering stress?

Instantaneous load or force applied per unit original area of cross-section (Before any application of force) is known as engineering stress.

It is denoted by σ (sigma). SI unit of engineering stress is N/m2 or Pascal (Pa).

What is Engineering Strain?

Change in length at some instant of the material per unit original length (Before any application of force) is known as engineering strain.

It is denoted by ε (Epsilon) or γ (Gamma). It’s a unitless quantity.

What is Tensile Stress?

When the applied force is away from the material, then the Stress produced is known as tensile stress.

What is Compressive Stress?

When the applied force is in towards the object, then the Stress produced is known as compressive stress.

What is Shear Stress?

When the applied force is parallel to the area on which it is applied, the Stress is known as shear stress.

What is Factor of Safety?

There are always some uncertainties in the measured values of stresses; therefore, the stress that we are going to consider for our use known as working Stress (σw) is always less than the experimental value of Stress. In most of the applications, we consider yield strength (σy).

Working Stress is determined by reducing the yield strength by a factor; that factor is known as the factor of safety. So, the factor of safety is a ratio of yield strength to working stress. Its symbol is N. It is a unitless quantity.

What is True Stress-Strain Curve?

It is a stress-strain curve in which true Stress is plotted against true strain. Both Stress and strain are based on instantaneous measurement hence instantaneous area of the cross-section is considered instead of original cross-section and instantaneous length is considered instead of the original length.

What is Breaking Point?

Stress necessary to continue plastic deformation starts to decrease after ultimate strength and eventually breaks the material at a point known as breaking point.

What is Ultimate Tensile Strength?

After yielding, as plastic deformation continues, it reaches a maximum limit known as ultimate Stress or ultimate strength, it is also known as Ultimate Tensile Strength (UTS)

What is Hooke’s Law? | Explain Hooke’s Law

When this type of deformation occurs, the strain in the metal piece is nearly proportional to the stress; therefore, this deformation occurs as a straight line in Stress versus strain plot except for some materials like grey cast iron, concrete and many polymers. Stress is proportional to the strain through this relationship.

This is known as Hooke’s Law, where Y the proportionality constant is known as Young’s Modulus.

It is one of the most important law in the studies of Strength of Materials.

CONCLUSION

In this articles important terminology of strength of materials are explained in detailed such as engineering stress, strain, stress-strain curve for both ductile and brittle materials, young modulus, Poisson’s ratio etc. Strength of materials is also known as mechanics of materials.

To Learn more on mechanical engineering and Strength of Materials click here!