This article discusses about reversible adiabatic expansion in detail. An adiabatic process is a process in which the heat transfer across the walls of system does not take place.
Reversible process are those processes which are ideal. One can trace back the entire path which was followed by the working fluid that means if a process 1-2 takes place then it can go from 2-1 following the same path. This means that there are no losses inside the system.
What is reversible adiabatic expansion?
As discussed above, reversible processes are ideal processes and adiabatic processes are those in which heat transfer does not take place. Reversible processes are infinitesimally slow that is in a piston cylinder arrangement, the piston moves at a very slow speed such that it appears stationary.
Reversible adiabatic expansion is the process in which the volume of the gas expands or increases after the process is completed. The temperature of the working fluid or the system decreases as a result of expansion.
Reversible adiabatic expansion formula
The formula for adiabatic expansion shows the relationship between volume and temperature. The temperature reduces with increase in volume.
The formula is given below-
T2-T1 = (V1/V2)γ-1/γ
Reversible adiabatic expansion temperature
The temperature decreases with increase in volume. Hence, in reversible adiabatic expansion process the temperature decreases.
The temperature in reversible adiabatic expansion process decreases with increase in volume. The relationship between volume and temperature is discussed above sections.
Reversible adiabatic expansion entropy
Entropy is the measure of randomness or degree of disorder. It is a very important quantity in thermodynamics. The efficiency or quality of any thermodynamic cycle depends on entropy.
In reversible adiabatic expansion, the entropy of the system is zero. For any reversible adiabatic process, the entropy of the system remains zero.
Reversible adiabatic expansion of an ideal gas
A gas is considered ideal when it is frictionless and incurs no losses while any thermodynamic process is taking place. While dealing with problems in thermodynamics, the gas is usually considered ideal for easy calculations.
The important formulae relating to ideal gas when it undergoes reversible adiabatic expansion are given below-
T2-T1 = (V1/V2)γ-1/γ
and for pressure-temperature relationship,
T2-T1 = (P2/P1)γ-1/γ
Reversible adiabatic expansion of a real gas
A real gas is non ideal in nature that is they do not obey the ideal gas laws. They show compressible effects, they are not frictionless, they have variable specific heat capacities etc. Hence, the work done by a real gas is always lesser than work done by ideal gas.
Van Der Wall’s equation for a real gas is given below-
(p + an2/V2)(V – nb) = nRT
Clearly the work obtained while doing reversible adiabatic expansion of real gas is much lower than that obtained from ideal gas.
Assumptions made for ideal gas
A gas can never be ideal. All gases are real in some or the other way. Although, some assumptions can be made regarding an ideal gas which helps us get an idea of how ideal a particular gas is. The assumptions made for ideal gas are given below-
- Zero inter particle interactions– The gas atoms don’t collide with each other.
- Frictionless– The gas won’t be affected by friction in its entire course of thermodynamic process.
- Incompressible– The density of the gas remains constant throughout, it does not change with change in surrounding pressure or temperature.
- Tends to fail at lower temperatures and high pressures– This happens because the inter molecular interactions become significant at this stage.
In practical situations, all the gases are ideal in nature and the closest gas to ideal gas is Helium gas due to its inert nature.
Characteristics of a real gas
The characteristics of the real gas are everything that is not ideal in nature. This happens due to inter molecular interactions, friction and other variable. The characteristics of ideal gas are as follows-
- Compressible– The real gases are compressible meaning their density can be changed.
- Variable heat capacity– Their heat capacities are not constant, they can change with change in surroundings.
- Van Der Walls forces– These forces arise due to distance dependent interaction between the molecules. In the for formula for real gas, there is a correction factor for both pressure and volume effects.
- Non equilibrium thermodynamic effects.
Work done in reversible adiabatic process
The heat transfer is zero in reversible adiabatic process. So the work is not transferred in the form of heat but change in volume.
The formula representing the work done in a reversible adiabatic process is given below-
W = nR(T1-T2)/γ-1
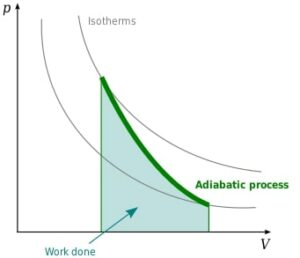
Image credits: User:Stannered, Adiabatic, CC BY-SA 3.0
Reversible adiabatic expansion enthalpy
Enthalpy is a function of heat. It changes with the amount of heat transfer taking place.
Enthalpy depends on the rate of heat transfer taking place. Since, in an adiabatic process, the change in heat content is zero so the enthalpy change is also zero.
Reversible adiabatic expansion final temperature
During an adiabatic expansion process, the final temperature is always lesser than the initial temperature as a result of expansion process.
The final temperature can be calculated from the temperature-volume relationship given below-
T2/T1 = (V1/V2)γ-1/γ
The final temperature can also be calculated from the temperature-pressure relationship given below-
T2/T1 = (p2/p1)γ-1/γ
Reversible adiabatic expansion example
No process is completely reversible or adiabatic, however the closest we can get to reversible adiabatic process is propagation of sound wave in fluids.
In Carnot cycle (again an ideal cycle) uses reversible adiabatic expansion and reversible adiabatic compression for expansion and compression purposes.
Why entropy change for a reversible adiabatic process is zero
The entropy of a system changes if heat content of the system changes. Since, the heat transfer is prohibited by the walls of adiabatic system, the net entropy change is also zero.
Graphically, the properties which form a closed path are zero. That means the starting point and ending points are same. In the case of entropy, since it is following a reversible cycle the entropy gets back to the same path to its original position. Hence, it is zero.
Hi ….I am Abhishek Khambhata, have pursued B. Tech in Mechanical Engineering. Throughout four years of my engineering, I have designed and flown unmanned aerial vehicles. My forte is fluid mechanics and thermal engineering. My fourth-year project was based on the performance enhancement of unmanned aerial vehicles using solar technology. I would like to connect with like-minded people.
Hi Fellow Reader,
We're a small team at Techiescience, working hard among the big players. If you like what you see, please share our content on social media. Your support makes a big difference. Thank you!