Mass flow rate Definition
Mass flow rate Equation | Mass flow rate units | Mass flow rate symbol
It is denoted by ṁ, It is Formulated as,
![]()
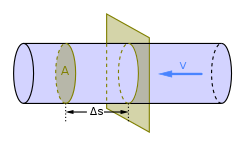
Image credit : MikeRun, Volumetric-flow-rate, CC BY-SA 4.0
In Hydrodynamics
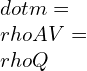
Where,
ρ = Density of the fluid
A = Cross sectional Area
V = Velocity of flow of fluid
Q = Volume flow rate or discharge
It has unit kg/s, lb./min etc.
Mass flow rate conversion
Mass flow rate from volumetric flow rate
In hydrodynamic, the mass-flow rate can be derived from volume flow rate with help of Continuity Equation.
The Continuity equation is given by
Q=AV
Where,
A = Cross sectional Area
V = Velocity of flow of fluid
Multiplying the continuity equation with density of the fluid we get ,
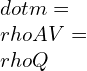
Where,
ρ = Density of the fluid
Mass flow rate to velocity | It’s Relationship with each other
In Hydrodynamics
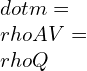
Where,
ρ = Density of the fluid
A = Cross sectional Area
V = Velocity of flow of fluid
Q = Volume flow rate or discharge
For an in-compressible fluid carrying through a fixed cross section, the mass-flow rate is directly proportionate to the velocity of fluid flown.
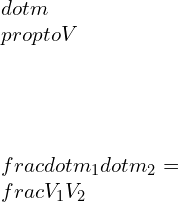
Reynolds number with mass flow rate | Their Generalized relation
The Reynolds number is given by the equation,
![]()
Where,
Lc = Characteristic length
V = Velocity of flow of fluid
ρ = Density of the fluid
μ = dynamic viscosity of the fluid
Multiply numerator and denominator by cross sectional Area A
![]()
But mass-flow rate is
![]()
Thus Reynolds Number becomes
![]()
Mass flow rate problems | Mass flow rate example
Q.1] A Turbine operates on a steady flow of air produces 1 kW of Power by expanding air from 300kPa, 350 K, 0.346 m3/kg to 120 kPa. The inlet and outlet velocity are 30 m/s and 50 m/s respectively. The expansion follows the Law PV1.4 = C. Determine the mass flow rate of air?
Solution:
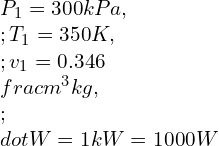
According to Steady Flow energy equation
![]()
Q = 0, Z1 = Z2
![]()
![]()
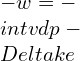
PVn = C
![]()
![]()
![]()
![]()

![]()
We get,
![]()
Mass-Flow rate is
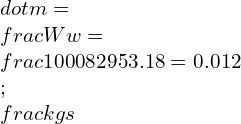
Q.2] Air enters a device at 4 MPa and 300oC with velocity of 150m/s. The inlet area is 10 cm2 and Outlet area is 50 cm2.Determine the mass flux if air exits at 0.4 MPa and 100oC?
Ans: A1 = 10 cm2, P1 = 4 MPa, T1 = 573 K, V1 = 150m/s, A2 = 50 cm2, P2 = 0.4 MPa, T2 = 373 K

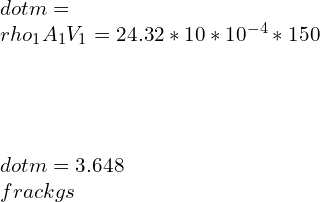
Q.3] A perfect gas having specific heat at constant pressure as 1 kJ/kgK enters and leaves a gas turbine with same velocity. The temperature of the gas at turbine inlet and outlet are 1100, and 400 Kelvin respectively and The power generation is at the rate 4.6 Mega Watt and heat leakages is at the rate of 300 kilo-Joule/seconds through the turbine casing. Compute mass flow rate of the gas through the turbine. (GATE-17-SET-2)
Solution: Cp = 1 kJ/kgK, V1 = V2, T1 = 1100 K, T2 = 400 K, Power = 4600 kW
Heat loss from turbine casing is 300 kJ/s = Q
According to Steady Flow energy equation
![]()
![Rendered by QuickLaTeX.com \\\\\\dot{m}h_1+Q=\\dot{m}h_2+W\\\\ \\\\\\dot{m}[h_1-h_2]=W-Q\\\\ \\\\\\dot{m}C_p[T_1-T_2]=W-Q\\\\ \\\\\\dot{m}=\\frac{W-Q}{C_p[T_1-T_2]}=\\frac{4600+300}{1100-400}=7\\;\\frac{kg}{s}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-ab801810045c79c44023956f8f7184db_l3.png)
FAQ
Why is mass flow rate important?
Ans: Mass-flow rate is important in the wide range of field which include fluid dynamics, pharmacy, petrochemicals etc. It is important to ensure right fluid possessing desired properties is flowing to the required location. It is important for maintaining and controlling the quality of fluid flowing. Its accurate measurements ensure the safety of workers working in a hazardous and dangerous environment. It is also important for good machine performance and efficiency and environment.
Mass flow rate of water
Mass-flow rate is given by the equation
![]()
Density of water is 1000 kg/m3
![]()
Mass flow rate of air
Mass-flow rate is given by the equation
![]()
Density of air is 1 kg/m3
![]()
How to get mass flow rate from enthalpy?
Heat Transfer in fluid and thermodynamics is given by the following equation
![]()
Where Q = heat transfer, m = mass-flow rate, h = change in enthalpy For constant heat supplied or rejected, enthalpy is inversely proportionate to mass flow rate.
How to get mass flow rate from Velocity?
In hydrodynamic, the mass-flow rate can be derived from volume flow rate with help of Continuity Equation.
The Continuity equation is given by
Q = AV
Where,
A = Cross sectional Area
V = Velocity of flow of fluid
Multiplying the continuity equation with density of the fluid we get,
![]()

Image credit : Julius Schröder derivative work: Regi51, Luftmassenmesser2 1, CC BY-SA 3.0
Can mass flow rate be negative
The magnitude of Mass flow rate cannot be negative. If we are provided the mass-flow rate with negative sign it generally indicates the direction of mass flux is reversed than the direction taken into consideration.
Mass flow rate for an ideal compressible gas
Air is assumed to be an Ideal compressible gas with Cp = 1 kJ/kg. K.
Mass flow rate is given by the equation
![]()
Density of air is 1 kg/m3
![]()
How can I find the mass flow of a refrigeration fluid R 134a and its temperatures in a domestic freezer How can I find them?
Assuming the Domestic freezer works on a vapor compression cycle, in order to find out mass-flow rate of the coolant R-134a we are required to find:
- Net refrigeration capacity or effect – generally given for that particular model of freezer.
- Compressor Inlet Pressure and Temperature
- Compressor outlet Pressure and Temperature
- Temperature and pressure at the inlet of evaporator
- Temperature and pressure at the outlet of condenser
- For P-h chart find enthalpy at all the above points.
- Net Refrigeration effect = mass-flow rate * [h1 – h2]
What is the relationship between pressure and mass flow rate Does the mass flow rate increase if there’s a pressure increase and does the mass flow rate decrease if there’s a pressure decrease ?
Let,
L = length of pipe
V = Velocity of flow of fluid
μ = dynamic viscosity of the fluid
d = diameter of pipe
According to Hagen Poiseuille equation
![]()
Multiplying numerator and denominator by ρA
![]()
![]()
![]()
where, ν = kinematic viscosity = μ/ρ
Thus, as pressure difference increases, mass-flow rate increases and vice versa.
For a convergent nozzle if the exit pressure is less than the critical pressure then what will be the mass flow rate?
As per described situation, nozzle’s outlet velocity is
![]()
Mass-flow rate will be
![]()
Where
A1, A2 = Inlet and Outlet Area of nozzle
C1, C2 = Inlet and exit velocity of nozzle
P1, P2 = Inlet and Outlet Pressure
V1, V2 = Volume at Inlet and Outlet of nozzle
r = Pressure ratio =P2/P1
n = Index of expansion
Why is mass flow rate is ρVA but volumetric flow rate is AV?
In hydrodynamic, the mass flux can be derived from volume flow rate with help of Continuity Equation.
The Continuity equation is given by
Q =AV
Where,
A = Cross sectional Area
V = Velocity of flow of fluid
Multiplying the continuity equation with density of the fluid we get the mass flow rate,
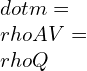
Where,
ρ = Density of the fluid
How is the Coriolis principle used to measure mass flow?
A Coriolis mass flowmeter works on the principle of the Coriolis Effect and this are true mass meter because they measure the mass rate of flow directly rather than measuring the volumetric flow rate and converting it into the mass flow rate.
Coriolis meter operates linearly, In the meantime no adjustments are essential for changing fluid characteristic. It is independent of fluid characteristics.
Operating Principle:
The fluid is allowed to flow through a U-shaped tube. An oscillation-based excitation force is utilized to the tube, causing it to oscillate. The vibration causes the fluid to induce twist or rotation to the pipe because of Coriolis acceleration. Coriolis acceleration is acting opposite to applied excitation force. The generated twist results in a time lag in flow between the entry and exit-side of the tube, and this Lag or phase difference is proportionate to the mass flow rate.
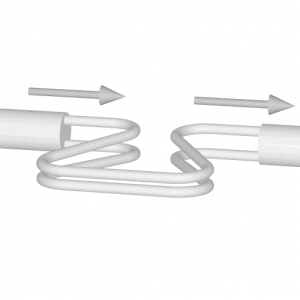
Image credit : Cleontuni at English Wikipedia, Coriolis meter vibrating flow 512×512, CC BY-SA 2.5
What is the relationship between mass flow rate and volume flow rate?
In hydrodynamic, the mass flow rate can be derived from volume flow rate with help of Continuity Equation.
The Continuity equation is given by
Q = AV
Where,
A = Cross sectional Area
V = Velocity of flow of fluid
Multiplying the continuity equation with density of the fluid we get,
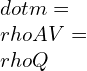
Where,
ρ = Density of the fluid
What is the formula for finding mass flow rate in a water cooled condenser?
Let,
h1 = enthalpy of water at inlet of the condenser
T1 = Temperature of water at inlet of the condenser
h2 = enthalpy of water at exit of the condenser
T2 = Temperature of water at exit of the condenser
Cp = Specific heat of water at constant pressure
Power of the condenser,
![Rendered by QuickLaTeX.com \\\\P=\\dot{m}[h_1-h_2]\\\\ \\\\\\dot{m}=\\frac{P}{h_1-h_2}\\\\ \\\\\\dot{m}=\\frac{P}{C_p[T_1-T_2]}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-f9d3656cc8e9eecbd79512180f849452_l3.png)
How do you find mass flow with temperature and pressure?
Let,
L = length of pipe
V = Velocity of flow of fluid
μ = dynamic viscosity of the fluid
d = diameter of pipe
According to Hagen Poiseuille equation
![]()
Multiplying numerator and denominator by ρA
![]()
![]()
![]()
where, ν = kinematic viscosity = μ/ρ
Thus, as pressure difference increases, m increases.
According to Steady Flow energy equation

Why in choked flow we always control downstream pressure while the maximum mass flow rate is dependent on upstream pressure
It is impossible to regulate Choked mass flows by changing the downstream pressure. When sonic conditions reach the throat, Pressure disturbances caused due to regulated downstream pressure cannot propagate upstream. Thus, you cannot control the maximum flow rate by regulating the downstream backpressure for a choked flow.
What is the average fluid mass flow rate of water in pipes with diameter 10cm, velocity of flow is 20 m/s.
In Hydrodynamics
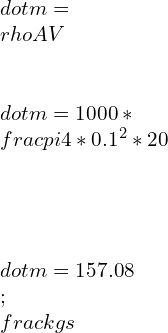
To know about Polytropic Process (click here)and Prandtl Number (Click here)

I am Hakimuddin Bawangaonwala , A Mechanical Design Engineer with Expertise in Mechanical Design and Development. I have Completed M. Tech in Design Engineering and has 2.5 years of Research Experience. Till now Published Two research papers on Hard Turning and Finite Element Analysis of Heat Treatment Fixtures. My Area of Interest is Machine Design, Strength of Material, Heat Transfer, Thermal Engineering etc. Proficient in CATIA and ANSYS Software for CAD and CAE. Apart from Research.