In this article the term “Laminar flow in pipe” and laminar flow in pipe related several facts will be discussed. Streamline flow is another term for the laminar flow.
Laminar flow in pipe or stream line in pipe can be describe as in this way, when a fluid is flow inside a tube or pipe in a motion that time there is no breakdown is present between the layers. In the low velocity the fluid can flow very smoothly without any transverse mixing.
What is laminar flow in pipe?
Laminar flow in pipe can be characterized by highly ordered motion and smooth streamline. The laminar flow in pipe fluid is flow uniformly in both direction and velocity.
The laminar flow in a pipe can be deriving as,
- If the range of the Reynolds number is 2000 and less than 2000 then this flow of fluid is known as laminar flow.
- Mathematical analysis of the laminar flow is not complicated.
- Velocity of the laminar flow is very low for this reason the flow of the fluid is fluid very smoothly without any transverse mixing.
- Regular movement can be observe in the fluids which in laminar flow and flow in a motion.
- Laminar flow in generally rare type of flow of fluid.
- Average motion can observe in which side the fluid is flowing.
- In the laminar flow the velocity profile is very less in the center section of the tube.
- In the laminar flow the velocity profile is high in the wall of the tube.
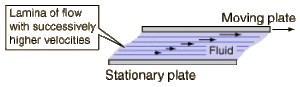
Image Credit – Wikipedia
Laminar flow in pipe formula:
With the help of Poiseuille’s equation we can understand the pressure drop of a flowing fluid is happened for the viscosity. The equation of Hegen Poiseuille’s is applicable for Newtonian fluid and incompressible fluid.
The equation of Hegen Poiseuille’s is not applicable for close entrance of the pipe. The equation of laminar flow is,
Where,
Δp = The amount of difference of pressure which is occur in the two end points of the pipe
μ = The dynamic viscosity of the flowing fluid in the pipe
L = Length of the pipe
Q = Volumetric flow rate
R = Radius of the pipe
A = Cross sectional area of the pipe
The above equation is not appropriate for very short or very long pipe and also for low viscosity fluid. In very short or very long pipe and also for low viscosity fluid turbulent flow is causes, for that that time the equation of Hegen Poiseuille’s is not applicable. In that case we applied more useful equation for calculation such as Darcy – Weisbach equation.
The ratio between from length to radius of a tube is more than the one forty eighth of Reynolds number which is valid for the law of Hegen Poiseuille’s. When the tube is very short that time the law of Hegen Poiseuille’s can be result as high flow rate unphysical.
The flow of the fluid is restricted by the principle of Bernoulli’s under excepting restrictive condition just because of pressure is not can be less than zero in an flow of incompressible.
Δ p = 1/2ρ v-2
Δ p = 1/2ρ(Qmax/π R2}2)
Laminar flow in pipe derivation:
The equation of laminar flow is,
Where in,
The Pressure Gradient (\Delta P):-
The pressure differential between the two ends of the tube, defined by the fact that every fluid will always flow from the high pressure to the low-pressure area.
The flow rate is calculated by the
Δ P = P1 – P2
The radius of the narrow tube:-
The flow of liquid direct changes with the radius to the power four.
Viscosity (η):-
The flow rate of the fluid is inversely proportional to the viscosity of the fluid.
Length of the arrow tube (L):-
The flow rate of the fluid is inversely proportional to the length of the narrow tube.
Resistance(R):-
The resistance is calculated by 8Ln/πr4 and hence the Poiseuille’s law is
Q = (Δ P) R
Heat transfer in pipe flow:
The equation of thermal energy convection-diffusion is given below,
The left-hand side equation is consider convective heat transfer, which transferred by the fluid’s motion. The radial velocity is zero, so the first term equation of the left-hand side can be avoided.
The right-hand side of the equation is representing the thermal diffusion. Since the flow is laminar, we can assume that the dimensionless Eckert number, which represents the ratio between a flow’s kinetic energy and its heat transfer driving force, is small enough to disregard viscous dissipation.
Therefore, the thermal energy equation can be supplemented with the velocity profile defined in the previous section.
A constant heat flux value condition implies that the temperature difference between the wall and the fluid is equal. However we already know that the temperature of the fluid is of non-constant value within the pipe. Therefore, we shall introduce a bulk mean temperature denoted by:
Assuming that the local temperature gradient and the bulk mean temperature gradient in the streamwise direction are equal and of constant value, integration of the aforementioned thermal energy transport equation results in the following formula for radial temperature distribution:
Where, a = k/ρc is the thermal diffusivity coefficient . The mean temperature gradient can be obtained by applying the desired volumetric flow rate Q and heat flux q to the heat conservation equation:
Qρc dTm/dz = πDq
To satisfy the constant wall flux condition, the value of the wall temperature has been coupled with the bulk mean temperature gradient.
Laminar flow in pipe boundary conditions:
Laminar boundary layers are appear when a moving viscous fluid is comes in the touch with a surface which is state in solid and the boundary layer, a layers of rotational fluid forms in response to the action of no slip boundary and viscosity condition of the surface.
Reynolds number for laminar flow in pipe:
The values for the laminar flow for the particular determination of Reynolds number is depend on the pattern of the flow of the fluid through a pipe and geometry of the system through which fluid is flow.
The expression for the Reynolds number for laminar flow in pipe is given below,
Re = ρuDH/μ = u DH/ν = QDH/νA
Where,
Re = Reynolds number
ρ = Fluid density of the pipe and unit is kilogram per cubic meter
u = The mean speed of the flowing fluid in the pipe and unit is meter per second
μ = The dynamic viscosity of the flowing fluid in the pipe and unit is kilogram per meter second
A = Cross sectional area of the pipe and unit is meter square
Q = Volumetric flow rate and unit is cubic meter per second
DH = Hydraulic diameter of the pipe through which fluid is flowing and unit is meter
ν = The kinematic viscosity of the flowing fluid in the pipe and unit is meter square per second
The expression of ν is,
ν = μ/ρ

Image Credit – Wikipedia
Nusselt number for laminar flow in pipe:
When internal laminar flow is fully developed in that case, Nusselt number for laminar flow in pipe can be express as,
Nu = hDh/kf
Where,
Nu = Nusselt number
h = Convective heat transfer coefficient
Dh = Hydraulic diameter of the pipe through which fluid is flowing
kf = Thermal conductivity for flowing fluid in the pipe
Friction factor for laminar flow in pipe:
Friction factor for the laminar flow can be express as,
fD = 64/Re
Where,
fD = Friction factor
Re = Reynolds number
Where,
ν = The kinematic viscosity of the flowing fluid in the pipe and unit is meter square per second
μ = The dynamic viscosity of the flowing fluid in the pipe and unit is kilogram per meter second
ρ= Fluid density of the pipe and unit is kilogram per cubic meter
v = Mean flow velocity and unit is meters per second
D = Diameter of the pipe through which fluid is flowing and unit is meter
ν = μ/ρ
Fully developed laminar flow in pipe:
Fully developed flow is appearing when the viscous effects are happened for the shear stress by the fluid particles and tube wall create a fully developed velocity profile.
For this to appear the fluid must go through a length of a straight tube. The velocity of the fluid for a fully developed flow will be at its fastest at the centre line of the tube (equation 1 laminar flow).
The velocity of the fluid at the walls of the pipe will theoretically be zero.
The fluid velocity can be expressed as an average velocity.
vc = 2Q/πR2……eqn (1)
The viscous effects are caused by the shear stress between the fluid and the pipe wall. In addition, shear stress will always be present regardless of how smooth the pipe wall is. Also, the shear stress between the fluid particles is a product of the wall shear stress and the distance the molecules is from the wall. To calculate shear stress use equation 2.
Due to the shear stress on the fluid particles, a pressure drop will occur. To calculate the pressure drop use equation 3.
P2 = P1 – Δ P…… eqn (3)
Finally, the viscous effects, pressure drop, and pipe length will affect the flow rate. To calculate the average flow rate, we need to use equation 4.
This equation can only applies to laminar flow.
Q = πD4ΔP/128μ L…… eqn (4)
Laminar flow in circular pipe:
In a circular pipe from where fluid is flow in laminar the diameter is express as D_c, for that case the friction factor of the flow is inversely proportional to the Reynolds number by which we can easily published or measured physical parameter.
Taking the help of Darcy – Weisbach equation laminar flow in circular pipe can be express as,
Δp/L = 128/π = μQ/D4c
Where,
Δp = The amount of difference of pressure which is occur in the two end points of the pipe
L = Length of the pipe through which fluid is flow
μ = The dynamic viscosity of the flowing fluid in the pipe
Q = Volumetric flow rate of the flowing fluid in the pipe
Instead of mean velocity the flowing fluid in the pipe volumetric flow rate can be used and its expression is given below,
Dc = Diameter of the pipe through which fluid is flowing
Laminar flow in a cylindrical pipe:
The cylindrical pipe which one contain flowing full, uniform diameter express as D, the loss of pressure for the viscous effects express as \Delta p is directly proportional to the length.
Laminar flow in a cylindrical pipe can be taking the help of Darcy – Weisbach equation is given below,
Where,
Δp = The amount of difference of pressure which is occur in the two end points of the pipe
L = Length of the pipe through which fluid is flow
ρ = Fluid density of the pipe
<v> = Mean flow velocity
DH = Hydraulic diameter of the pipe through which fluid is flowing
Laminar flow in a pipe velocity profile:
Laminar flow is appearing at very low velocities, under a threshold at that point the flow of the fluid is became turbulent.
The pipe velocity profile for laminar flow can be determined using the Reynolds number. The pipe velocity profile for laminar flow is also depending on the density and viscosity of the flowing fluid and dimensions of the channel.
Where,
Re = Reynolds number
ρ = Fluid density of the pipe and unit is kilogram per cubic meter
u = The mean speed of the flowing fluid in the pipe and unit is meter per second
μ = The dynamic viscosity of the flowing fluid in the pipe and unit is kilogram per meter second
A = Cross sectional area of the pipe and unit is meter square
Q = Volumetric flow rate and unit is cubic meter per second
DH = Hydraulic diameter of the pipe through which fluid is flowing and unit is meter
ν = The kinematic viscosity of the flowing fluid in the pipe and unit is meter square per second
The expression of ν is,
ν = μ/ρ
Laminar flow in vertical pipe:
Flowing of fluid in laminar in vertical pipe is given below,
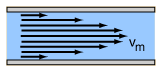
Image Credit – Wikipedia
Laminar flow in rough pipe:
The pressure drop in a fully developed laminar flow through pipe is proportional to mean velocity or average velocity in pipe. In laminar flow, the friction factor is independent of roughness because boundary layer covers the roughness.
Hi..I am Indrani Banerjee. I completed my bachelor’s degree in mechanical engineering. I am an enthusiastic person and I am a person who is positive about every aspect of life. I like to read Books and listen to music.