Isentropic Process: Definition,Types,Application,Limitation

Topic of discussion: Isentropic process

Isentropic Definition

A typical case of an adiabatic process that has no transfer of heat or matter through the process while the entropy of the system remains constant is known as an isentropic process.

The thermodynamic process where the entropy of the gas or fluid remains constant can also be coined as the reversible adiabatic process. This type of process that is both adiabatic in nature and internally reversible while considering that it is frictionless enables the engineering sector to view this as an idealized process and a model for comparing actual processes.

isentrophic — **Isentropic process graph**
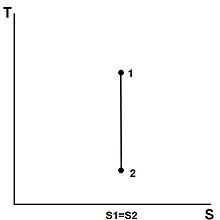
Tyler.neysmith, Isentropic, CC BY-SA 3.0

Ideally, enthalpy of the system is used in the particular isentropic process as the only variables changing are internal energy dU and system volume ΔV while the entropy remains unchanged.

The T-s diagram for an isentropic process is plotted based on the known traits varying from different states such as the pressure and temperature quantity. Since,

ΔS = 0 or s1 = s2

And,

H = U + PV

They are intrinsically related to the first law of thermodynamics in terms of enthalpy measure. Since it both reversible and adiabatic, the equations formed would be as follows:

$Reversible \\rightarrow dS=\\int_{1}^{2}\\left ( \\frac{\\delta Q}{T} \\right )_{rev}$

$Adiabatic\\rightarrow Q=0 \\Rightarrow dS=0$

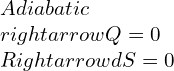
In enthalpy terms,

$dH=dQ+VdP$

Or,

$dH=TdS+VdP$

The water, refrigerants, and ideal gas can be derived using the equations in the molar form to deal with the enthalpy and temperature relation. At the same time, the specific entropy of the system remains unchanged.

From the enthalpy equation abiding by the first law of thermodynamics, VdP is considered a flow process work where a mass flow is involved as work is required to transfer the fluid in or out of the boundaries of the control volume. This flow energy (work) is generally utilized for systems with the difference in pressure dP, like an open flow system found in turbines or pumps. By simplifying the energy transfer description, it is derived that enthalpy change is equivalent to flow energy or process work done on or by the system at constant entropy.

For,

$dQ=0$

$dH=VdP$

$\\rightarrow W=H_{2}-H_{1}$

$\\rightarrow H_{2}-H_{1}=C_{p}\\left ( T_{2}-T_{1} \\right )$

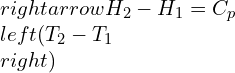
Isentropic process for an ideal gas

Now, for an ideal gas, the isentropic process where entropy changes are involved can be represented as:

$\\Delta S=s_{2}-s_{1}$

$=\\int_{1}^{2}C_{v}\\frac{dT}{T}+Rln\\frac{V_{2}}{V_{1}} \\rightarrow \\left ( 1 \\right )$

$=\\int_{1}^{2}C_{p}\\frac{dT}{T}-Rln\\frac{P_{2}}{P_{1}} \\rightarrow \\left ( 2 \\right )$

$\\Delta S\\rightarrow 0$

$equation \\left ( 1 \\right )\\rightarrow 0$

$=\\int_{1}^{2}C_{v}\\frac{dT}{T}-Rln\\frac{V_{2}}{V_{1}} \\rightarrow \\left ( 2 \\right )$

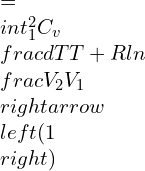
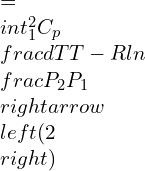
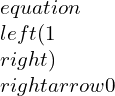
Integrating and rearranging,

$C_{v}ln\\frac{T_{2}}{T_{1}}=-Rln\\frac{V_{2}}{V_{1}}$

(this is by assuming constant specific heats)

$\\frac{T_{2}}{T_{1}}=\\left ( \\frac{V_{2}}{V_{1}} \\right )^{\\frac{R}{C_{v}}}=\\left ( \\frac{V_{2}}{V_{1}} \\right )^{k-1}$

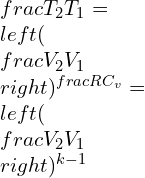
Where k is the specific heat ratio

$k=\\frac{C_{p}}{C_{v}}; R=C_{p}-C_{v}$

Now, setting

$equation \\left ( 2 \\right )\\rightarrow 0$

$\\int_{1}^{2}C_{p}\\frac{dT}{T}=Rln\\frac{P_{2}}{P_{1}}$

$\\Rightarrow C_{p}ln\\frac{T_{2}}{T_{1}}=Rln\\frac{P_{2}}{P_{1}}$

$\\Rightarrow \\frac{T_{2}}{T_{1}}=\\left ( \\frac{P_{2}}{P_{1}} \\right )^{\\frac{R}{C_{p}}}=\\left ( \\frac{P_{2}}{P_{1}} \\right )^{\\frac{k-1}{k}}$

$combining \\left ( 1 \\right ) and \\left ( 2 \\right )relations$

$\\left ( \\frac{P_{2}}{P_{1}} \\right )^{\\frac{k-1}{k}}=\\left ( \\frac{V_{1}}{V_{2}} \\right )^{k}$

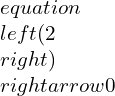
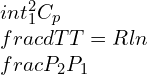
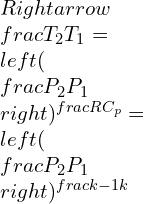
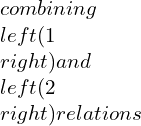
Consolidated expressions of the three relations of the equations in compact form can be projected as:

$TV^{k-1}=constant$

$TP^{\\frac{1-k}{k}}=constant$

$PV^{k}=constant$

If the specific heat constant assumptions are invalid, the entropy change would be:

$\\Delta S=s_{2}-s_{1}$

$s_{2}^{0}-s_{1}^{0}-Rln\\frac{P_{2}}{P_{1}}\\rightarrow \\left ( 1 \\right )$

$equation\\left ( 1 \\right )\\rightarrow 0$

$\\frac{P_{2}}{P_{1}}=\\frac{exp\\left ( \\frac{s_{2}^{0}}{R} \\right )}{exp\\left ( \\frac{s_{1}^{0}}{R} \\right )}$

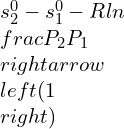
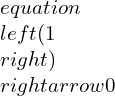
If the numerator of the above equation is construed as the relative pressure, then:

$\\left ( \\frac{P_{2}}{P_{1}} \\right )_{s}=constant=\\frac{P_{r2}}{P_{r1}}$

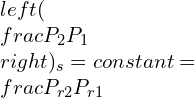
Pressure vs temperature values are tabulated against each other. Hence, the ideal gas relation produces:

$\\frac{V_{2}}{V_{1}}=\\frac{T_{2}P_{1}}{T_{1}P_{2}}$

$Replacing \\rightarrow \\frac{P_{r2}}{P_{r1}}$

$\\left ( \\frac{V_{2}}{V_{1}} \\right )=\\frac{\\left ( \\frac{T_{2}}{P_{r2}} \\right )}{\\left ( \\frac{T_{1}}{P_{r1}} \\right )}$

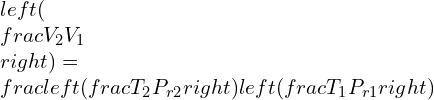
Defining the relative specific volume,

$\\left ( \\frac{V_{2}}{V_{1}} \\right )_{s}=constant=\\frac{V_{r2}}{V_{r1}}$

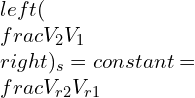
Isentropic process derivation

The total energy change in a system:

$dU=\\delta W+\\delta Q$

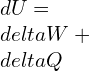
A reversible condition involving work with pressure is,

As established earlier,

$dH=dU+pdV+Vdp$

For isentropic,

$\\delta Q_{rev}=0$

And,

$dS=\\frac{\\delta Q_{rev}}{T}=0$

Now,

$dU=\\delta W+\\delta Q=-pdV+0,$

$dH=\\delta W+\\delta Q+pdV+Vdp=-pdV+0+pdV+Vdp=Vdp$

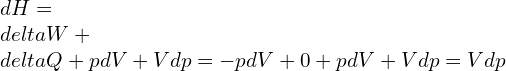
Capacity ratio:

$\\gamma =-\\frac{\\frac{dp}{p}}{\\frac{dV}{V}}$

$cp - cv = R$

$1 - \\frac{1}{\\gamma } = \\frac{R}{C_{p}}$

$\\frac{C_{p}}{R} = \\frac{\\gamma }{\\gamma -1}$

$p = r * R * T$

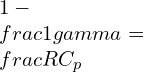
Where, r=density

$ds = \\frac{C_{p}dT}{T} - R \\frac{dp}{p}$

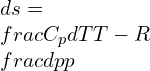
As dS=0,

$\\frac{C_{p}dT}{T} = R \\frac{dp}{p}$

After substitution of PV=rRT equation in the above equation,

$Cp dT = \\frac{dp}{r}$

$\\Rightarrow (\\frac{C_{p}}{r}) d(\\frac{p}{r}) = \\frac{dp}{r}$

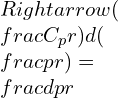
Differentiating,

$(\\frac{C_{p}}{r}) * (\\frac{dp}{r} - \\frac{pdR}{r^{2}}) = \\frac{dP}{r}$

$((\\frac{C_{p}}{r}) - 1) \\frac{dp}{p} = (\\frac{C_{p}}{r}) \\frac{dr}{r}$

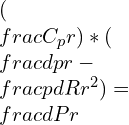
Substituting the gamma equation,

$(\\frac{1}{\\gamma -1}) \\frac{dp}{p} = \\left ( \\frac{\\gamma }{\\gamma -1} \\right )\\frac{dr}{r}$

Simplifying the equation:

$\\frac{dp}{p} = \\gamma \\frac{dr}{r}$

Integrating,

$\\frac{p}{r^{\\gamma }} = constant$

For the flow brought to rest isentropically, the total pressure and density occurring can be evaluated as a constant.

$\\frac{p}{r^{\\gamma }} = \\frac{pt}{rt^{\\gamma }}$

$\\frac{p}{pt} = \\left ( \\frac{r}{rt} \\right )^{\\gamma }$

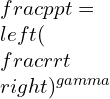
pt being the total pressure and rt being the total density of the system.

$\\frac{rt}{(rt * Tt) } = \\left ( \\frac{r}{rt} \\right )^{\\gamma }$

$\\frac{T}{Tt} = \\left ( \\frac{r}{rt} \\right )^{\\gamma -1}$

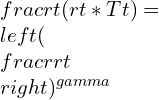
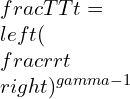
Now, by combining the equations:

$\\frac{p}{pt} = \\left ( \\frac{T}{Tt} \\right )^{\\frac{\\gamma }{\\gamma -1}}$

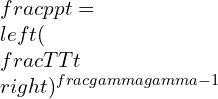
Isentropic work equation

$W=\\int_{1}^{2}PdV=\\int_{1}^{2}\\frac{K}{V^{\\gamma }}dV$

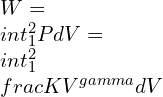
$\\Rightarrow W=\\frac{K}{-\\gamma +1}\\left [ \\frac{V_{2}}{V_{2}^{\\gamma }}-\\frac{V_{1}}{V_{1}^{\\gamma }} \\right ]$

$\\Rightarrow W=\\frac{1}{-\\gamma +1}\\left [ \\left ( \\frac{K}{V_{1}^{\\gamma }} \\right )V_{1}-\\left ( \\frac{K}{V_{2}^{\\gamma }} \\right )V_{2} \\right ]$

$\\Rightarrow W=\\left ( \\frac{1}{\\gamma -1} \\right )\\left [ P_{1}V_{1}-P_{2}V_{2} \\right ]$

$\\Rightarrow W=\\left ( \\frac{1}{\\gamma -1} \\right )\\left [ nRT_{2}-nRT_{1} \\right ]$

$\\therefore W=\\frac{nR\\left ( T_{2}-T_{1} \\right )}{\\gamma -1}$

While satisfying the isentropic equations respectively under enthalpy and entropy values.

Isentropic turbine and isentropic expansion

$\\eta _{T}=\\frac{Actual Turbine work}{Isentropic Turbine work}$

$\\Rightarrow \\frac{W_{real}}{W_{s}}$

$\\Rightarrow \\frac{h_{1}-h_{2r}}{h_{1}-h_{2s}}$

For the purpose of calculations, the adiabatic process for the steady flow devices such as turbines, compressors or pumps is ideally generated as an isentropic process. Specific ratios are evaluated for calculating the efficiency of steady flow machines by including parameters that intrinsically affect the overall system of the process.

Typically, the particular device’s efficiency ranges from 0.7-0.9, which is about 70-90%.

While,

$\\eta _{C}=\\frac{Isentropic Compressor work}{Actual Compressor work}$

$\\Rightarrow \\frac{W_{s}}{W_{real}}$

$\\Rightarrow \\frac{h_{2s}-h_{1}}{h_{2r}-h_{1}}$

Summary and conclusion

The Isentropic process, ideally known as a reversible adiabatic process, is exclusively used in the various thermodynamic cycles such as Carnot, Otto, Diesel, Rankine, Brayton cycle and so on. The numerous mathematical equations and tables plotted utilizing the isentropic process parameters are basically used to determine the efficiency of gases and flows of the systems that are steady in nature such as turbines, compressors, nozzles, etc.

To read more about mechanical related articles click here

lambdageeksScience Core SME

The lambdageeksScience Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.

All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.

Isentropic Process: 5 Important Factors Related To It