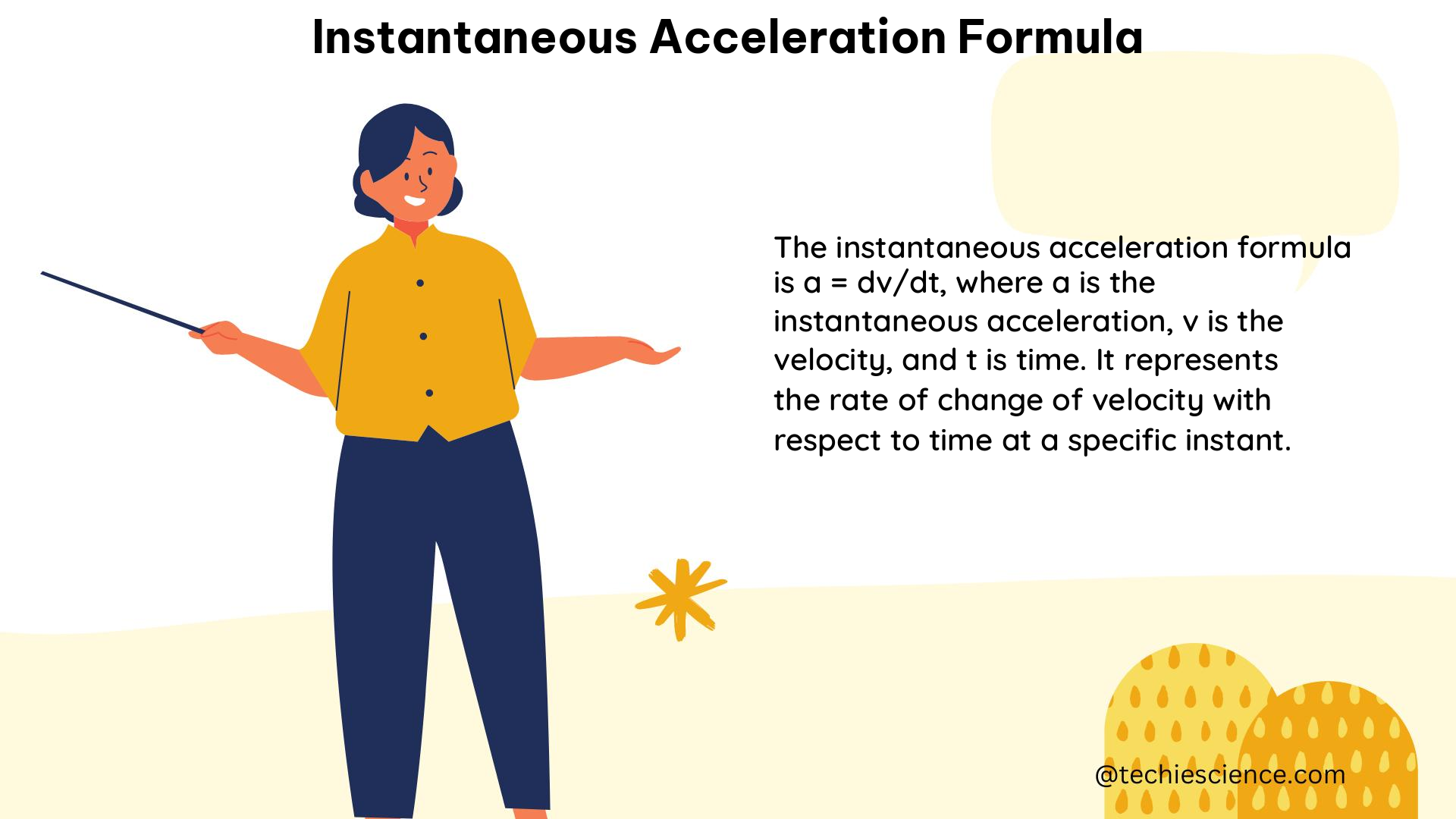
The instantaneous acceleration formula is used to calculate the rate at which an object’s velocity changes at a specific moment in time. It is a measure of how quickly an object’s speed or direction is changing at any given instant. The formula for instantaneous acceleration is given by dividing the change in velocity by the change in time. This can be represented as a = (v2 – v1) / (t2 – t1), where “a” is the acceleration, “v2” and “v1” are the final and initial velocities, and “t2” and “t1” are the final and initial times.
Key Takeaways
| Formula | Description |
|---|---|
| a = (v2 – v1) / (t2 – t1) | Instantaneous acceleration formula |
Understanding Instantaneous Acceleration
Instantaneous acceleration refers to the rate at which an object’s velocity changes at a specific moment in time. It provides insight into how quickly an object’s speed or direction is changing at any given instant. To understand instantaneous acceleration, we need to delve into its definition and explore the difference between instantaneous and average acceleration.
Definition of Instantaneous Acceleration
Instantaneous acceleration is defined as the derivative of an object’s velocity with respect to time. In simpler terms, it is the rate of change of velocity over an infinitesimally small time interval. Mathematically, it can be expressed using the following equation:
a = dv/dt
Where:
– a represents instantaneous acceleration
– dv represents the change in velocity
– dt represents the change in time
To calculate instantaneous acceleration, we need to determine the change in velocity and divide it by the change in time. By doing so, we obtain a value that represents the acceleration at a specific moment.
Difference between Instantaneous and Average Acceleration
While instantaneous acceleration focuses on the rate of change of velocity at a particular instant, average acceleration considers the overall change in velocity over a given time interval. The formula for average acceleration is:
a_avg = Δv/Δt
Where:
– a_avg represents average acceleration
– Δv represents the change in velocity
– Δt represents the change in time
The key distinction between these two concepts lies in the time interval considered. Instantaneous acceleration zooms in on a specific moment, providing a snapshot of the object’s acceleration, while average acceleration provides an average value over a larger time interval.
To better understand the difference, let’s consider an example. Suppose a car is initially at rest and then accelerates uniformly to a velocity of 30 m/s in 5 seconds. At the 2-second mark, the car’s velocity is 12 m/s. To find the instantaneous acceleration at that moment, we can use the acceleration formula:
a = dv/dt = (30 m/s - 0 m/s) / (5 s - 0 s) = 6 m/s²
On the other hand, to find the average acceleration over the entire 5-second interval, we can use the average acceleration formula:
a_avg = Δv/Δt = (30 m/s - 0 m/s) / (5 s - 0 s) = 6 m/s²
In this case, both the instantaneous and average accelerations are equal, as the car’s acceleration is constant. However, in scenarios where acceleration varies, the instantaneous acceleration at a specific moment may differ from the average acceleration over a longer time interval.
Understanding instantaneous acceleration allows us to analyze an object’s motion in more detail. By examining how velocity changes at different moments, we can gain insights into the object’s speed, direction, and the rate at which these properties change. It is a valuable concept in physics that helps us comprehend the dynamic nature of objects in motion.
The Mathematics Behind Instantaneous Acceleration
Instantaneous Acceleration as a Derivative
In the world of physics and calculus, instantaneous acceleration plays a crucial role in understanding the motion of objects. It allows us to determine how an object’s velocity changes over an infinitesimally small period of time. To grasp the concept of instantaneous acceleration, we need to delve into its mathematical foundations.
When we talk about instantaneous acceleration, we are essentially referring to the rate at which an object’s velocity changes at a specific moment in time. In other words, it measures how quickly an object’s velocity is increasing or decreasing at a particular instant. This concept is closely related to the derivative, which is a fundamental concept in calculus.
Instantaneous Acceleration Formula in Physics
In physics, we can calculate instantaneous acceleration using the formula:
[ a = \frac{{\Delta v}}{{\Delta t}} ]
where:
– a represents the instantaneous acceleration
– Δv denotes the change in velocity
– Δt represents the change in time
This formula allows us to determine the rate of change of an object’s velocity over a given time interval. By dividing the change in velocity by the change in time, we obtain the instantaneous acceleration at a specific moment.
Instantaneous Acceleration Formula in Calculus
From a calculus perspective, we can express instantaneous acceleration as the derivative of velocity with respect to time. Mathematically, this can be represented as:
[ a = \frac{{dv}}{{dt}} ]
where:
– a represents the instantaneous acceleration
– v denotes the velocity
– t represents time
By taking the derivative of the velocity function with respect to time, we obtain the instantaneous acceleration function. This allows us to analyze how an object’s velocity changes at any given moment.
Instantaneous Acceleration Formula in Circular Motion
In the context of circular motion, the formula for instantaneous acceleration takes a slightly different form. When an object moves in a circular path, its velocity constantly changes direction. The instantaneous acceleration in circular motion can be calculated using the formula:
[ a = \frac{{v^2}}{{r}} ]
where:
– a represents the instantaneous acceleration
– v denotes the magnitude of the velocity
– r represents the radius of the circular path
This formula tells us that the instantaneous acceleration in circular motion is directly proportional to the square of the velocity and inversely proportional to the radius of the circular path.
Instantaneous Angular Acceleration Formula
In addition to linear acceleration, we also have angular acceleration, which measures how quickly an object’s angular velocity changes. The formula for instantaneous angular acceleration is given by:
[ \alpha = \frac{{d\omega}}{{dt}} ]
where:
– α represents the instantaneous angular acceleration
– ω denotes the angular velocity
– t represents time
Similar to linear acceleration, the instantaneous angular acceleration can be obtained by taking the derivative of the angular velocity function with respect to time.
Understanding the mathematics behind instantaneous acceleration allows us to analyze the motion of objects in a more precise and detailed manner. By utilizing the appropriate formulas and equations, we can calculate and interpret instantaneous acceleration in various scenarios, whether it’s in linear or circular motion.
How to Determine Instantaneous Acceleration
Instantaneous acceleration refers to the rate at which an object’s velocity changes at a specific moment in time. It provides valuable information about an object’s motion, including its direction and magnitude of acceleration. In this guide, we will explore different methods to determine instantaneous acceleration.
How to Calculate Instantaneous Acceleration
To calculate instantaneous acceleration, we need to know the object’s velocity at a particular instant and the time at which it occurs. The formula for instantaneous acceleration is:
a = Δv / Δt
Where:
– a represents instantaneous acceleration
– Δv is the change in velocity
– Δt is the change in time
By dividing the change in velocity by the change in time, we can obtain the instantaneous acceleration of an object.
How to Find Acceleration from Instantaneous Velocity
If we have the instantaneous velocity of an object at a given moment, we can determine its acceleration. The formula for acceleration can be derived from the instantaneous velocity equation:
v = u + at
Where:
– v represents instantaneous velocity
– u is the initial velocity
– a is the acceleration
– t is the time
Rearranging the equation, we can solve for acceleration:
a = (v - u) / t
By substituting the values of instantaneous velocity, initial velocity, and time into the equation, we can find the acceleration.
Using Instantaneous Acceleration Formula Calculator
To simplify the process of calculating instantaneous acceleration, you can use an online calculator. These calculators allow you to input the values of velocity and time, and they will provide you with the instantaneous acceleration.
By utilizing a calculator, you can save time and ensure accurate results. However, it is essential to understand the underlying formulas and concepts to interpret the calculated values correctly.
In conclusion, determining instantaneous acceleration involves calculating the change in velocity over a specific time interval. By understanding the formulas and utilizing calculators, you can easily find the instantaneous acceleration of an object at any given moment.
Practical Applications of Instantaneous Acceleration
Instantaneous Acceleration in Gravity
Instantaneous acceleration plays a crucial role in understanding the behavior of objects in gravity. When an object is in free fall, its velocity changes continuously due to the force of gravity. By calculating the instantaneous acceleration, we can determine the rate at which the object’s velocity is changing at any given moment. This information is particularly useful in fields such as physics, engineering, and astronomy.
To calculate the instantaneous acceleration in gravity, we can use the formula for acceleration:
a = Δv / Δt
Where:
– a represents the instantaneous acceleration
– Δv is the change in velocity
– Δt is the change in time
By measuring the change in velocity and time, we can determine the instantaneous acceleration of an object in free fall. This information can be used to predict the object’s future motion, analyze its behavior, and make informed decisions in various practical scenarios.
When is Instantaneous Acceleration Zero
In certain situations, the instantaneous acceleration of an object may be zero. This occurs when the object reaches its maximum velocity or changes direction. For example, when a car is moving at a constant speed on a straight road, its instantaneous acceleration is zero because there is no change in velocity.
Another instance where instantaneous acceleration is zero is when an object reaches the highest point of its trajectory in projectile motion. At this point, the object momentarily stops moving upward and starts moving downward, resulting in zero acceleration.
Instantaneous Acceleration and Deceleration
Deceleration is essentially negative acceleration, representing a decrease in velocity over time. When an object is slowing down, its instantaneous acceleration is negative. This occurs when the change in velocity is negative, indicating a decrease in speed.
In practical applications, understanding instantaneous acceleration during deceleration is crucial for safety and efficiency. For example, when designing braking systems for vehicles, engineers need to calculate the deceleration required to bring the vehicle to a stop within a certain distance. By analyzing the instantaneous acceleration during deceleration, they can determine the appropriate braking force and optimize the braking system for maximum safety.
In summary, instantaneous acceleration is a valuable concept in various fields and practical applications. By utilizing the acceleration formula and understanding when instantaneous acceleration is zero or negative, we can analyze the behavior of objects, predict their motion, and make informed decisions in real-world scenarios.
Instantaneous Acceleration Formula Examples
Instantaneous Acceleration Formula Example
To understand the concept of instantaneous acceleration, let’s consider an example. Suppose a car is traveling along a straight road. We want to determine the car’s acceleration at a specific moment in time.
To calculate the instantaneous acceleration, we need to know the car’s velocity and the time at which we want to find the acceleration. Let’s say at time t=3 seconds, the car’s velocity is 10 m/s. We can use the following formula to calculate the instantaneous acceleration:
Acceleration = (Change in Velocity) / (Change in Time)
In this case, the change in velocity is 10 m/s (since the car’s velocity is constant), and the change in time is 0 seconds (since we are considering a specific moment). Therefore, the instantaneous acceleration at t=3 seconds is 0 m/s^2.
Instantaneous Acceleration Formula Derivation
The formula for instantaneous acceleration can be derived from the basic principles of calculus. Let’s consider an object moving along a straight line. The object’s velocity at any given time t can be represented by the function v(t).
To find the instantaneous acceleration at a specific time t, we need to find the derivative of the velocity function with respect to time. The derivative represents the rate of change of velocity with respect to time, which is the definition of acceleration.
Mathematically, the instantaneous acceleration a(t) can be calculated as:
a(t) = d(v(t))/dt
where d(v(t))/dt represents the derivative of the velocity function with respect to time.
By finding the derivative of the velocity function, we can determine the instantaneous acceleration at any given time. This formula allows us to analyze the motion of an object and understand how its velocity changes over time.
In summary, the instantaneous acceleration formula allows us to calculate the rate at which an object’s velocity changes at a specific moment in time. By understanding this concept, we can gain insights into the dynamics of objects in motion and analyze their behavior.
Frequently Asked Questions about Instantaneous Acceleration
What is Instantaneous Acceleration Formula?
Instantaneous acceleration refers to the rate at which an object’s velocity changes at a specific moment in time. It is a measure of how quickly an object’s speed or direction is changing at any given instant. The formula for instantaneous acceleration is derived from the concept of calculus and involves taking the derivative of the velocity function with respect to time. Mathematically, the formula for instantaneous acceleration can be expressed as:
Where:
– a represents the instantaneous acceleration
– dv represents the change in velocity
– dt represents the change in time
How to Find Instantaneous Acceleration Formula?
To find the instantaneous acceleration formula, you need to have the velocity function of the object. The velocity function describes how the object’s velocity changes with respect to time. Once you have the velocity function, you can differentiate it with respect to time to obtain the instantaneous acceleration formula. The process involves taking the derivative of the velocity function using calculus.
For example, let’s say we have a velocity function of an object given by:
To find the instantaneous acceleration at a specific time t, we differentiate the velocity function with respect to time:
Simplifying the above expression will give us the instantaneous acceleration formula.
Why are the Units for Average and Instantaneous Rates the Same?
The units for average and instantaneous rates are the same because both quantities measure the change in a physical quantity over a given interval of time. In the case of acceleration, both average and instantaneous acceleration measure how an object’s velocity changes over a specific time interval.
The unit of acceleration is meters per second squared (m/s²), which represents the change in velocity per unit of time. This unit remains the same for both average and instantaneous acceleration because they both measure the rate of change of velocity.
It is important to note that while the units are the same, average and instantaneous acceleration represent different concepts. Average acceleration measures the overall change in velocity over a given time interval, while instantaneous acceleration represents the rate of change of velocity at a specific instant in time.
In summary, the units for average and instantaneous rates, including acceleration, remain the same because they measure the change in a physical quantity over a specific time interval.
Conclusion
In conclusion, the instantaneous acceleration formula is a powerful tool that allows us to calculate the rate at which an object’s velocity is changing at any given moment. By taking the derivative of the velocity function with respect to time, we can determine the exact acceleration of an object at a specific point in time. This formula is particularly useful in physics and engineering, as it helps us understand how objects move and interact with their surroundings. By utilizing the instantaneous acceleration formula, we can make accurate predictions and calculations in various fields of study.
Frequently Asked Questions
1. How to determine instantaneous acceleration?
To determine instantaneous acceleration, you need to find the derivative of velocity with respect to time. This can be done using calculus or by analyzing the slope of a velocity-time graph at a specific point.
2. What is the formula for instantaneous acceleration?
The formula for instantaneous acceleration is the derivative of velocity with respect to time. Mathematically, it can be represented as:
Instantaneous Acceleration = dV/dt
3. How to find acceleration from instantaneous velocity?
To find acceleration from instantaneous velocity, you can differentiate the velocity function with respect to time. The resulting derivative will give you the instantaneous acceleration at a specific point in time.
4. What is the instantaneous acceleration formula?
The instantaneous acceleration formula is the derivative of velocity with respect to time. It can be expressed as:
Instantaneous Acceleration = dV/dt
5. How to find the instantaneous acceleration formula?
The instantaneous acceleration formula can be found by differentiating the velocity function with respect to time. This will give you the rate of change of velocity at a specific point in time.
6. What is instantaneous acceleration?
Instantaneous acceleration refers to the rate at which an object’s velocity changes at a specific instant in time. It is the derivative of velocity with respect to time.
7. What is the formula for instantaneous velocity and acceleration?
The formula for instantaneous velocity and acceleration depends on the specific situation or motion being analyzed. In general, the instantaneous velocity can be found by differentiating the position function with respect to time, while the instantaneous acceleration can be found by differentiating the velocity function with respect to time.
8. What is the instantaneous acceleration formula example?
An example of the instantaneous acceleration formula is:
Instantaneous Acceleration = dV/dt
where dV represents the change in velocity and dt represents the change in time.
9. How to calculate instantaneous acceleration?
To calculate instantaneous acceleration, you need to find the derivative of velocity with respect to time. This can be done using calculus or by analyzing the slope of a velocity-time graph at a specific point.
10. How to find instantaneous acceleration?
To find instantaneous acceleration, you can differentiate the velocity function with respect to time. The resulting derivative will give you the instantaneous acceleration at a specific point in time.
Also Read:
- How to find centripetal acceleration
- How to find tension force with acceleration
- Angular acceleration formula
- How to find acceleration with time and displacement
- Acceleration examples
- How to find acceleration and force
- How to find acceleration with mass and height
- How to find negative acceleration
- How to find acceleration due to gravity with mass
- How to find average acceleration
The TechieScience Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the TechieScience.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.