In the kinematic theory, distance, velocity, acceleration, displacement, and time are the fundamental concepts to derive the equation of motion of in 2-dimensional space.
Generally, the distance traveled by a body per unit time gives the velocity. If the velocity changes with time during the motion, the body possesses the term acceleration. In this post, how velocity, acceleration, and distance are related is discussed in detail, and we get to know how to find velocity with acceleration and distance.
How to find velocity with acceleration and distance?
Suppose the body begins to move with initial velocity zero. The body is moving with acceleration ‘a’ and covers the distance ‘d’ meters; then, we need to find the velocity at which the body is moving. Now arise a question of how to find velocity with acceleration and distance?
Velocity gives how fast an object can move a distance over a given time period.
The expression is given by
v=x/t
But from the considering the equation
v = a*t
t=v/a
Substituting the value of t and rearranging, we get
v=x/(v/a)
v2 = a*x
v=√ax
The equation obtained above is applicable if the body begins to move from zero velocity and then accelerates. The body is moving with constant acceleration to reach a distance d.
Using the general expression, we can find the body’s velocity with acceleration and distance with or without time.
How to find velocity from acceleration and distance without time?
The velocity of the body is always measured with the time taken by the body to travel a certain distance. If the time is not given by then, how to find velocity with acceleration and distance?
We follow two methods to find the velocity with given acceleration and distance. Generally, we consider the time in the very first equation; by eliminating the time factor, we get an equation of velocity without time.
By algebraic method:
To calculate the velocity without time, let us consider the equation of velocity with acceleration and time,
v = a * t
The ratio of distance traveled and time gives the velocity of the body. It is given by the equation,
v=x/t
Where x is the distance covered and t is the time taken to cover the distance d,
x/t=at
Substituting the value of v in the first equation; we get,
x = at2
From the kinematic theory, if the velocity of the body is changing with time, then we take the average of the velocity, therefore;
x= at2/2
But we can say that,t= v/a ,substituting in the above equation,
Solving and rearranging the terms we get,
x=v2/2a
v2 = 2ax
v=√2ax
The above equation answers how to find velocity with acceleration and distance.
By integral calculus method:
The acceleration can be written as,
a=dv/dt
Velocity is nothing but the time derivative of distance covered by the body; it is given by,
dt=dx/v
Substituting the value of dt in the acceleration equation, we get
a=vdv/dx
a dx = v dv Since we have considered that the initial body possesses zero velocity, we integrate the above equation with the limit zero to a maximum value of the velocity and distance.
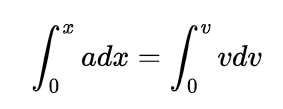
ax=v2/2
v2 = 2 ax
v=√2ax
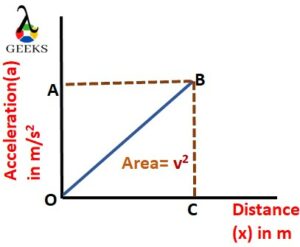
How to find velocity from acceleration and distance graph?
The plot of acceleration vs. distance gives the equation of motion under a specific time period.
The area under the acceleration–distance graph gives the square of the velocity of the moving body. From the definition of acceleration, it is the second-order derivative of the distance, so that the velocity will be two times the area.
For example, the acceleration displacement graph for a body moving with constant acceleration, after a certain time, the body decelerates and covers a certain distance, is given below, the velocity of the body can be calculated using the graph.
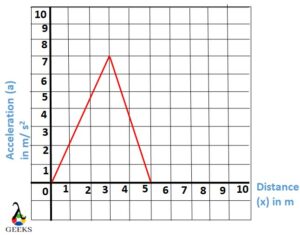
The area covered by the a-d graph is a triangle; therefore, the area of the triangle is given by
A=1/2 hb
A=1/2 5*7
A = 17. 5 units
The velocity can be written as
A=√2*area
A=√35
Because 2A = 35 units.
v = 5.91 m/s.
How to find initial velocity from acceleration and distance?
Initial velocity is the velocity at which the body begins its motion.
In order to calculate the initial velocity, we have to consider the fundamental equation of the velocity; it is given by;
v=x/t
So the distance is given as; x = v*t
Here, the velocity is not constant; hence we can take the average value of the velocity as
v=vi+vf/2
So the equation will be
x=vi+vf/2t
But the equation of motion vf = vi + at, substituting the value of vf, we get
x=vi+(vi+at)/2t
x=2vi+at/2t
x=2vi+at/2
2x = 2vit+at2
On rearranging the above equation,
vi = x/t – 1/2at
The above equation gives the initial velocity with acceleration and distance.
How to find final velocity from acceleration and distance?

The final velocity is the velocity attained by the body before the motion is stopped due to any hindrance.
When the moving body begins to accelerate means the velocity has been changed. This change in velocity is given by the initial and final velocity of the body. Suppose we have provided only initial velocity, then how to find velocity with acceleration and distance at the final point of the motion is answered below.
To derive the equation for final velocity, let us consider the motion of the car. The car is moving with initial velocity vi, and after some time t, the car begins to accelerate. The car attains the acceleration ‘a’ and covers the distance x.
The derivation can be done by three methods
Let us study the above three methods in detail.
By algebraic method:
The distance traveled by the body is given by
x=vi+vf/2t
The velocity is not constant; it changes with the time period, so choose to take the average of the velocities.
From the kinematic equation of motion, we have
vf = vi + at
Let us rearrange the above equation to get the time as
t = vf-vi/2a
Substituting the value in the first equation,
x=vf-vi/2 vf+vi/a
The above equation is similar to (a+b)(a-b)= a2-b2, then the required solution will be
x=vf-vi/2a
vf2– vi2 = 2ax
vf2= vi2 – 2ax
The equation obtained above is the required equation of final velocity. We can further simplify it by taking the square root on both sides; we get
vf2=√(vi2-2ax)
By calculus method:
We know that acceleration is given by the first-order derivative of velocity with respect to time t.
a=dv/dt
And the velocity as
v=dx/dt
Cross multiplying both equations and then integrating by choosing the limit x=0 to x=x and v=vi to v=vf we get;
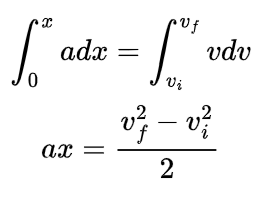
vf2– vi2 = 2ax
Rearranging the terms;
vf2= vi2 – 2ax
By graphical method:
A plot of velocity vs. time can helps to find the final velocity of the body.
Generally the distance travelled by the body can be find finding the area covered by the body. Using these available data, we can calculate the distance traveled so that the equation of final velocity can be calculated.
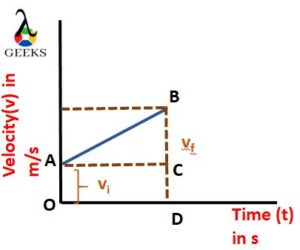
From the above graph, the area of the trapezium OABD gives the distance traveled by the body,
x=OA+BD/2* OD
OA is the initial velocity vi, and BD is the Final velocity vf, and OD is the time, so the equation can be modified as,
x=vf+vi/2* t
But, we know that ]t = vf-vi/a
x=vi+vf/2* vf-vi/a
x=vf2-vi2/2a
vf2– vi2 = 2ax
vf2= vi2 – 2ax
The required equation of final velocity with the graphical method is obtained.
The final velocity equation from acceleration and distance can be rearranged to calculate the initial velocity of the body; it is shown below:
vi2= vf2 – 2ax
How to find average velocity with acceleration and distance?
If the velocity keeps on changing, then we need to find the average velocity to describe the motion.
In order to establish an equation for average velocity, we must know initial and final velocity. But we can find average velocity even if the initial and final velocity is unknown by knowing acceleration and distance. Let us know how to find average velocity.
Let us suppose that a car is moving with initial velocity vi and as it begins to accelerate after covering some distance xi and travels a distance xf at which it has the final velocity vf.
The distance covered by the body is from xi to xf, i.e., at the distance xi, the velocity of the body is vi, and at the point xf, the velocity of the body is vf, then.
A general expression of average velocity is given as,
va=vi+vf/2
Equation of motion for final velocity is vf = vi+ at
Substituting in the general equation, we have
va=vi+vi+at/2
va=2vi+at/2
va=vi+1/2 at
By considering the initial velocity expression, we get
vi = x/t-1/2 at
va= x/t-1/2at+1/2 at
But t=√2x/a
Putting in the above expression, we get
va=x/√2x/a
Squaring on both sides, we get
va2=x2/2x/a
va2=ax2/2x
va2=ax/2
va=√ax/2
The above equation gives the average velocity of the moving body.
Solved Problems On How to find velocity with acceleration and distance
How to find velocity with acceleration and distance is given, if a motor vehicle is moving with a constant acceleration of 12 m/s2 and covers a distance of 87 m, and hence find the time taken by the vehicle to cover the same distance.
Solution:
Given data – The distance covered by the vehicle x = 87 m.
Acceleration of the vehicle a = 12 m/s2.
To find the velocity of the motor vehicle,
v=√ax
v=√12*87
v=√1044
v = 32.31 m/s.
From the relation between velocity, acceleration, distance, and time, we have the equation of velocity.
v= x/t
t= x/v
t= 87/32.31
t = 2.69 s.
In a race, the racer rides the bike with an initial velocity of 9 m/s. After time t, the velocity changes, and the acceleration is 3 m/s2. The racer covers a distance of 10 m. calculate the final velocity of the bike to reach the given distance and hence find the average velocity of the bike.
Solution:
The equation to find the final velocity of the bike is given by;
vf2= vi2 – 2ax
vf2= (9)2 – 2(3 * 10)
vf2= 81 – 60
vf2= 21
vf = 4.58 m/s.
The average velocity is given by
va=vi+vf/2
va=9+4.58/2
va=13.58/2
v = 6.79 m/s.
An athlete runs with an initial velocity of 10 m/s. He covers 10 m with a constant acceleration of 4 m/s2. Find the initial velocity.
Solution:
Data are given for the calculation – the initial velocity vi = 10 m/s.
Acceleration a = 4 m/s2.
Distance x = 10 m
vf2= vi2 – 2ax
vf2= (10)2 – 2( 4 *10)
vf2= 100 – 80
vf2= 100 – 80
vf2= 20
vf = 4.47 m/s.
Calculate the average velocity of particle moving with acceleration of 12 m/s2 and the distance travelled by the particle is 26 meters.
Solution:
The formula gives the average velocity for given acceleration and distance.
va=√ax/2
The data are given – Acceleration of the particle a = 12 m/s2.
Distance traveled by the particle x = 26 m.
Substituting the given values in the equation
√12*26/2
va=√156
va = 12.48 m/s.
A car travels a distance of 56 meters in 4 seconds. The acceleration of the car with the given time is 2 m/s2. Calculate the initial velocity of the car.
Solution:
Given – the distance traveled by the car x = 56 m.
Time is taken by the car to cover the distance x t = 4 s.
Acceleration attain by the car a = 2 m/s2.
The initial velocity of the car is given by the formula
vi = x/t-1/2 at
Substituting the given values in the above equation,
vi = 56/4-1/2*2*4
vi = 14 – 4
vi = 10 m/s.
A graph of acceleration and distance is plotted, then how to find velocity with acceleration and distance is given in the graph.
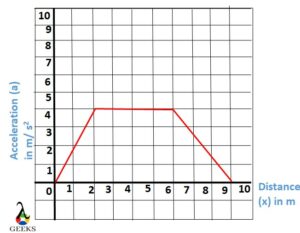
The distance traveled with acceleration given in the graph forms a trapezium, the area of the trapezium is given by
A=a+b/2* h
Where a and b are the adjacent side of the trapezium and h is the height.
From the above graph
a = 4.5 units
b = 9 units
h = 4 units
Substituting in the given equation,
A=(4.5+9/2)4
A = 27 units.
The velocity is given as
v=√2*area
v=√2*27
v=√56
v = 7.34 m/s.
How do you calculate displacement?
A: Displacement can be calculated using the equation s = ut + 1/2at^2, where s is the displacement, u is the initial velocity, a is the acceleration, and t is the time interval.
How do velocity and displacement relate in kinematics?
A: In kinematics, velocity and displacement are closely related. Velocity is the rate of change of displacement with respect to time. In other words, velocity represents the speed and direction of an object’s motion.
What is kinematics?
A: Kinematics is the branch of physics that deals with the motion of objects without considering the forces that cause the motion. It focuses on describing and analyzing the motion of objects using mathematical equations and concepts.
What happens when an object starts from rest?
A: When an object starts from rest, it means that its initial velocity is zero. In this case, the equation to find velocity simplifies to v = at, where v is the final velocity, a is the acceleration, and t is the time interval.
Also Read:
- How to find constant acceleration with velocity and time
- How to find momentum after collision
- How to find amplitude of transverse wave
- How to find acceleration with velocity and distance
- How to find centripetal acceleration
- How to find acceleration with a constant velocity
- How to find final velocity without acceleration
- How to find constant acceleration with distance and time
- How to find angular acceleration of a wheel
- How to find gravitational acceleration
I am Keerthi K Murthy, I have completed post graduation in Physics, with the specialization in the field of solid state physics. I have always consider physics as a fundamental subject which is connected to our daily life. Being a science student I enjoy exploring new things in physics. As a writer my goal is to reach the readers with the simplified manner through my articles.