Mastering the Art of Finding Photon Momentum: A Comprehensive Guide

Photons, the fundamental particles of light, possess a unique property known as momentum. Understanding how to calculate the momentum of a photon is crucial in various fields of physics, from quantum mechanics to astrophysics. In this comprehensive guide, we will delve into the intricacies of finding the momentum of a photon, providing you with a step-by-step approach and a wealth of technical details to help you become a true master in this subject.

The Fundamental Equation: Planck’s Constant and Wavelength

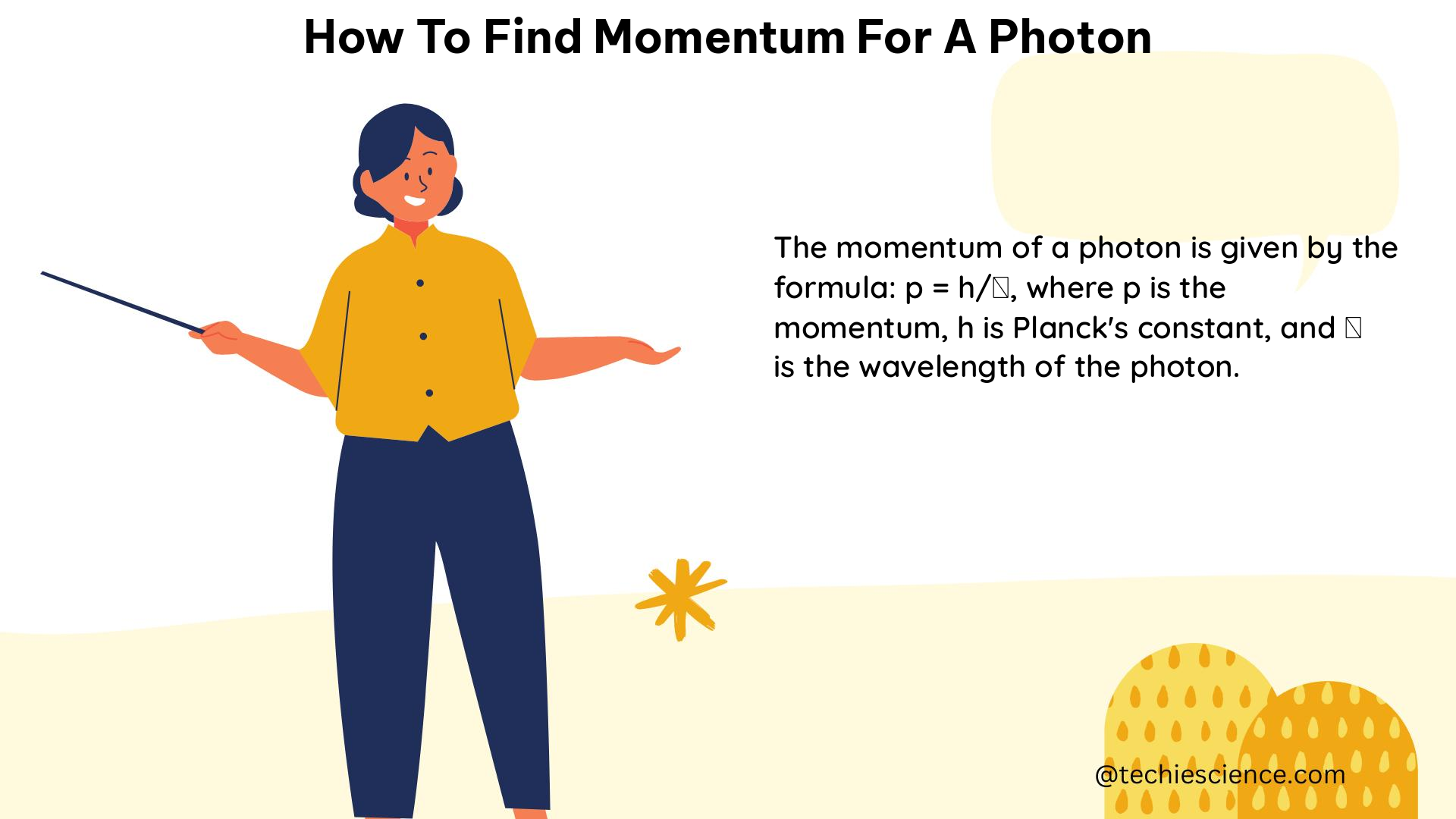
The momentum of a photon is directly related to its wavelength, as described by the equation:

p = h/λ

where:
– p is the momentum of the photon, measured in kg·m/s
– h is Planck’s constant, which has a value of approximately 6.626 × 10^-34 J·s
– λ is the wavelength of the photon, measured in meters (m)

To find the momentum of a photon, you simply need to plug the known wavelength into this equation and perform the calculation. Let’s consider an example:

Suppose we have a photon with a wavelength of 4.5 × 10^-7 meters. To find its momentum, we can substitute the values into the equation:

p = (6.626 × 10^-34 J·s) / (4.5 × 10^-7 m)
p = 1.47 × 10^-27 kg·m/s

Therefore, the momentum of the photon is 1.47 × 10^-27 kg·m/s.

Understanding the Importance of Wavelength Conversion

It’s important to note that the wavelength must be expressed in meters before plugging it into the momentum equation. If the wavelength is given in a different unit, such as nanometers (nm) or angstroms (Å), you must convert it to meters first.

For example, if the wavelength is given as 450 nm, you would need to convert it to meters:

1 nm = 1 × 10^-9 m
450 nm = 450 × 10^-9 m = 4.5 × 10^-7 m

Then, you can use this value in the momentum equation:

p = (6.626 × 10^-34 J·s) / (4.5 × 10^-7 m)
p = 1.47 × 10^-27 kg·m/s

Proper wavelength conversion is crucial to ensure accurate momentum calculations.

Photon Energy and Momentum: The Connection

The momentum of a photon is closely related to its energy. The energy of a photon is given by the equation:

E = hc/λ

where:
– E is the energy of the photon, measured in Joules (J)
– h is Planck’s constant, as before
– c is the speed of light, which is approximately 3 × 10^8 m/s
– λ is the wavelength of the photon, measured in meters (m)

By rearranging this equation, we can express the momentum of the photon in terms of its energy:

p = E/c

This relationship allows us to calculate the momentum of a photon if we know its energy, or vice versa.

Photon Momentum Calculations: Examples and Numerical Problems

Let’s explore some examples and numerical problems to solidify your understanding of finding the momentum of a photon.

Example 1: Photon with a Known Energy

A photon traveling at the speed of light has an energy of 0.00016 Joules. What color does the photon emit, and how much momentum does it carry?

1. Define the variables:
2. E = 0.00016 Joules
3. v = c (speed of light)
4. Use the energy equation E = ρv and solve it for ρ (momentum):
5. ρ = E/v
6. Substitute the values from step 1 to find the photon’s momentum:
7. ρ = (0.00016 Joules) / (2.99 × 10^8 m/s)
8. ρ = 5.35 × 10^-13 J·s/m
9. Use the equation λ = h/ρ to find the wavelength of the photon:
10. λ = (1.602 × 10^-19 J·s) / (5.35 × 10^-13 J·s/m)
11. λ = 2.99 × 10^-7 m

Therefore, the photon emits ultraviolet light with a wavelength of about 300 nm and carries a momentum of 5.35 × 10^-13 J·s/m.

Example 2: Photon with a Different Energy

Repeat Example 1 with a photon of 0.00003 Joules.

1. Define the variables:
2. E = 0.00003 Joules
3. v = c (speed of light)
4. Use the equation ρ = E/v to find the photon’s momentum:
5. ρ = (0.00003 Joules) / (2.99 × 10^8 m/s)
6. ρ = 1.00 × 10^-12 J·s/m
7. Use the equation λ = h/ρ to find the wavelength of the photon:
8. λ = (1.602 × 10^-19 J·s) / (1.00 × 10^-12 J·s/m)
9. λ = 1.60 × 10^-7 m

Therefore, the photon carries a momentum of 1.00 × 10^-12 J·s/m and has a wavelength of 1.60 × 10^-7 m, which is in the infrared region of the spectrum.

Numerical Problem 1

A photon has a wavelength of 5.0 × 10^-7 m. Calculate the momentum of the photon.

Solution:
1. Substitute the given wavelength into the momentum equation:
– p = h/λ
– p = (6.626 × 10^-34 J·s) / (5.0 × 10^-7 m)
– p = 1.325 × 10^-27 kg·m/s

Therefore, the momentum of the photon is 1.325 × 10^-27 kg·m/s.

Numerical Problem 2

A photon has an energy of 2.0 × 10^-19 J. Calculate the momentum of the photon.

Solution:
1. Use the relationship between photon energy and momentum:
– E = hc/λ
– p = E/c
2. Substitute the given energy and the speed of light:
– p = (2.0 × 10^-19 J) / (3.0 × 10^8 m/s)
– p = 6.667 × 10^-28 kg·m/s

Therefore, the momentum of the photon is 6.667 × 10^-28 kg·m/s.

These examples and numerical problems demonstrate the step-by-step process of finding the momentum of a photon, using the fundamental equations and the relationships between photon energy, wavelength, and momentum.

Practical Applications and Implications

The momentum of photons has various practical applications and implications in the field of physics. Some notable examples include:

1. Solar Sails: The momentum of photons can be used to propel spacecraft equipped with large, reflective “solar sails.” As the photons from the Sun strike the sail, they impart a small amount of momentum, which can gradually accelerate the spacecraft over time.

2. Radiation Pressure: The momentum of photons can exert a small but measurable force on objects, known as radiation pressure. This effect is observed in the tails of comets, where the solar radiation pressure pushes the dust and gas away from the comet’s nucleus.

3. Quantum Mechanics: In quantum mechanics, the momentum of photons is a crucial concept, as it is related to the wavelike nature of light and the behavior of particles at the quantum level.

4. Astrophysics and Cosmology: The momentum of photons plays a significant role in various astrophysical and cosmological phenomena, such as the formation and evolution of stars, the dynamics of galaxies, and the study of the early universe.

Understanding the principles of finding the momentum of a photon is not only essential for theoretical physics but also has practical implications in various fields of science and technology.

Conclusion

In this comprehensive guide, we have explored the fundamental equation for finding the momentum of a photon, the importance of wavelength conversion, the connection between photon energy and momentum, and a variety of examples and numerical problems to solidify your understanding. By mastering the techniques presented in this article, you will be well-equipped to tackle any problem related to the momentum of photons, whether in the classroom, the laboratory, or in your own research endeavors.

Remember, the key to success in this field lies in a deep understanding of the underlying principles and a willingness to practice the calculations. With dedication and persistence, you can become a true expert in the art of finding the momentum of a photon.

References

1. Photon Momentum – Khan Academy
2. Energy & Momentum of a Photon | Formula & Calculation – Study.com
3. Photon Momentum Physics – Nagwa
4. Photon Momentum | Physics – Lumen Learning
5. How Do We Measure Photon Momentum? – AZoQuantum