Gravitational acceleration refers to the acceleration experienced by an object due to the force of gravity. It is a fundamental concept in physics and plays a crucial role in understanding the motion of objects in the presence of gravity. In this blog post, we will explore various methods to calculate gravitational acceleration, discuss its applications in different scenarios, and even conduct practical experiments to better comprehend this fascinating phenomenon.
Methods to Calculate Gravitational Acceleration
Using the Slope to Determine Gravitational Acceleration
One method to calculate gravitational acceleration is by using the slope of a position-time graph. By measuring the change in position of an object over time, we can determine its velocity. Then, by analyzing the slope of the velocity-time graph, we can find the object’s acceleration, which in this case is gravitational acceleration. This method is particularly useful for studying free-falling objects or those in projectile motion.
Calculating Gravitational Acceleration Given Mass
Another approach to finding gravitational acceleration involves using Newton’s law of gravitation. According to this law, the force of gravity between two objects is directly proportional to the product of their masses and inversely proportional to the square of the distance between their centers. By rearranging this equation, we can solve for gravitational acceleration. Let’s consider an example:
Suppose we have two objects, Object A and Object B, with masses m_A and m_B respectively, and the distance between their centers is r. The formula for gravitational acceleration (g) can be expressed as:
![]()
where G is the gravitational constant. By plugging in the appropriate values for m_A, m_B, r, and G, we can determine the gravitational acceleration between the two objects.
Measuring Gravitational Acceleration with a Pendulum
A pendulum can also be utilized to measure gravitational acceleration. In this method, we use the formula for the period of a pendulum (T), which is the time it takes for the pendulum to complete one full swing. The formula for the period of a simple pendulum is:
![]()
where L is the length of the pendulum and g is the gravitational acceleration. By rearranging this equation, we can solve for g. By measuring the period and the length of the pendulum, we can calculate the gravitational acceleration.
Finding Gravitational Acceleration without Mass

In some cases, we may need to determine the gravitational acceleration without any knowledge of the masses involved. One way to achieve this is by analyzing the motion of objects in orbit. For example, the gravitational acceleration of a satellite can be determined by examining its orbital period and radius. By using Kepler’s third law of planetary motion, which relates the orbital period (T) and the radius of the orbit (r), we can find the gravitational acceleration (g) using the formula:
![]()
This method enables us to calculate gravitational acceleration without directly considering the masses of the objects involved.
Gravitational Acceleration in Different Scenarios
How to Calculate Gravitational Acceleration on the Moon
The gravitational acceleration on the Moon is significantly less than that on Earth. To calculate the gravitational acceleration on the Moon, we can use the formula we discussed earlier:
![]()
where ![]() and
and ![]() are the masses of the Moon and the Earth, respectively, and
are the masses of the Moon and the Earth, respectively, and 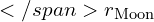 r_{\text{Moon}}” title=”Rendered by QuickLaTeX.com” height=”22″ width=”154″ style=”vertical-align: -6px;”/> is the distance between their centers. By plugging in the appropriate values, we can determine the gravitational acceleration on the Moon.
r_{\text{Moon}}” title=”Rendered by QuickLaTeX.com” height=”22″ width=”154″ style=”vertical-align: -6px;”/> is the distance between their centers. By plugging in the appropriate values, we can determine the gravitational acceleration on the Moon.
Determining Gravitational Acceleration of a Planet
To calculate the gravitational acceleration of a planet, we can use a similar approach as we did for the Moon. By considering the mass of the planet ![]() , the mass of the central body around which it orbits
, the mass of the central body around which it orbits ![]() , and the distance between their centers
, and the distance between their centers ![]() , we can use the formula:
, we can use the formula:
![]()
This formula allows us to determine the gravitational acceleration acting on the planet.
Measuring Gravitational Acceleration of a Satellite

The gravitational acceleration of a satellite can be determined using the formula we discussed earlier:
![]()
By measuring the satellite’s orbital period (T) and the radius of its orbit (r), we can calculate the gravitational acceleration acting on the satellite.
Finding Gravitational Acceleration of an Asteroid
Similar to determining the gravitational acceleration of a planet, we can use the formula:
![]()
where ![]() represents the mass of the asteroid,
represents the mass of the asteroid, ![]() represents the mass of the central body, and
represents the mass of the central body, and ![]() represents the distance between their centers. By plugging in the appropriate values, we can find the gravitational acceleration of an asteroid.
represents the distance between their centers. By plugging in the appropriate values, we can find the gravitational acceleration of an asteroid.
Practical Experiments to Understand Gravitational Acceleration
Simple Experiments to Measure Gravitational Acceleration
There are several simple experiments that can be conducted to measure gravitational acceleration. One such experiment involves dropping objects of different masses from the same height and measuring the time it takes for them to reach the ground. By using the formula for free-fall motion:
![]()
where h represents the height, ![]() represents the gravitational acceleration, and
represents the gravitational acceleration, and ![]() represents the time, we can calculate
represents the time, we can calculate ![]() .
.
Using a Pendulum to Calculate Gravitational Acceleration
As mentioned earlier, a pendulum can be used to measure gravitational acceleration. By measuring the period and length of a pendulum, we can calculate the gravitational acceleration using the equation:
![]()
where L represents the length of the pendulum and T represents the period. By conducting this experiment and plugging in the values, we can determine the gravitational acceleration.
Understanding the Value of Gravitational Acceleration through Experiments
By performing practical experiments, we can gain a deeper understanding of the value of gravitational acceleration. These experiments not only provide us with a hands-on experience but also allow us to apply mathematical formulas and equations to calculate gravitational acceleration accurately. By comparing the results of different experiments, we can verify the consistency of our calculations and further solidify our understanding of this fundamental concept.
By exploring various methods to calculate gravitational acceleration, examining its applications in different scenarios, and conducting practical experiments to comprehend its value, we can develop a comprehensive understanding of this fundamental concept in physics. Gravitational acceleration not only influences the motion of objects on Earth and in space but also plays a crucial role in shaping the structure of the universe itself. So let’s grab our calculators, conduct some experiments, and dive deeper into the captivating world of gravitational acceleration.
How can you calculate gravitational acceleration without mass and why is it important in physics?
The process of calculating gravitational acceleration without mass is crucial in physics as it allows for the determination of the acceleration due to gravity without considering the mass of an object. By understanding this concept, scientists can analyze and predict the motion of objects in gravitational fields without relying on their mass. This becomes particularly significant when studying the general principles of gravity and its effects on various objects or systems. It ultimately provides a more universal understanding of gravitational forces.
Numerical Problems on how to find gravitational acceleration
Problem 1:
A satellite is orbiting the Earth at a height of 800 km above the surface. Calculate the gravitational acceleration experienced by the satellite.
Solution:
Given:
Height above the surface of the Earth, h = 800 km
We know that the gravitational acceleration, g, can be calculated using the formula:
![]()
where G is the gravitational constant, M is the mass of the Earth, R is the radius of the Earth, and h is the height above the surface.
Substituting the given values: ![]()
Simplifying:
![]()
![]()
![]()
Therefore, the gravitational acceleration experienced by the satellite is approximately 9.799 m/s².
Problem 2:
A planet has a mass of 1.2 × 10^24 kg and a radius of 5000 km. Calculate the gravitational acceleration on the surface of the planet.
Solution:
Given:
Mass of the planet, M = 1.2 × 10^24 kg
Radius of the planet, R = 5000 km
We know that the gravitational acceleration, g, can be calculated using the formula:
![]()
where G is the gravitational constant, M is the mass of the planet, and R is the radius of the planet.
Substituting the given values:
![]()
Simplifying:
![]()
![]()
![]()
Therefore, the gravitational acceleration on the surface of the planet is approximately 3.204 m/s².
Problem 3:
A rocket is launched from the surface of a planet with an initial velocity of 200 m/s. If the gravitational acceleration on the planet is 12 m/s², calculate the height reached by the rocket after 10 seconds.
Solution:
Given:
Initial velocity, u = 200 m/s
Gravitational acceleration, g = 12 m/s²
Time, t = 10 s
We can use the formula for height reached in vertical motion:
![]()
Substituting the given values:
![]()
Simplifying:
![]()
![]()
![]()
Therefore, the rocket reaches a height of 1400 meters after 10 seconds.
Also Read:
- Ways to find acceleration
- How to find acceleration with angle and coefficient of kinetic friction
- Constant negative acceleration graph
- Instantaneous acceleration formula
- Constant acceleration formula
- Centripetal acceleration in pendulum
- Is angular acceleration constant
- How to find acceleration with mass and air resistance
- Angular and tangential acceleration
- How to find resultant acceleration

Hello, I’m Manish Naik completed my MSc Physics with Solid-State Electronics as a specialization. I have three years of experience in Article Writing on Physics subject. Writing, which aimed to provide accurate information to all readers, from beginners and experts.
In my leisure time, I love to spend my time in nature or visiting historical places.
Looking forward to connecting you through LinkedIn –