In the world of physics, understanding motion dynamics is crucial. One fundamental aspect of motion is acceleration, which measures how quickly an object’s velocity changes over time. In this blog post, we will explore how to find constant acceleration using velocity and time. We will delve into the mathematical relationship between acceleration, velocity, and time, and provide a step-by-step guide to calculating constant acceleration. Additionally, we will discuss practical applications of these calculations and highlight the importance of accurate measurements in real-world scenarios.
The Mathematical Relationship Between Acceleration, Velocity, and Time
To understand how to find constant acceleration using velocity and time, we must first explore the formula for acceleration. Acceleration ![]() ) is defined as the rate of change of velocity
) is defined as the rate of change of velocity ![]() ) with respect to time
) with respect to time ![]() ). Mathematically, it can be represented as:
). Mathematically, it can be represented as:
![]()
Here, ![]() represents the change in velocity and
represents the change in velocity and ![]() represents the change in time. This formula allows us to quantify how an object’s velocity changes over a given time interval.
represents the change in time. This formula allows us to quantify how an object’s velocity changes over a given time interval.
Velocity ![]() ), on the other hand, measures the rate of change of displacement
), on the other hand, measures the rate of change of displacement ![]() ) with respect to time
) with respect to time ![]() ). The relationship between velocity and time can be expressed as:
). The relationship between velocity and time can be expressed as:
![]()
Where ![]() represents the change in displacement and
represents the change in displacement and ![]() represents the change in time. It is important to note that velocity is a vector quantity, meaning it has both magnitude and direction.
represents the change in time. It is important to note that velocity is a vector quantity, meaning it has both magnitude and direction.
How Velocity and Time Factor into the Equation

When we have information about an object’s velocity and time, we can use these values to calculate acceleration. Let’s consider a scenario where an object starts with an initial velocity ![]() ) and undergoes a uniform acceleration
) and undergoes a uniform acceleration ![]() ) for a specific time interval
) for a specific time interval ![]() ). In this case, we can determine the final velocity
). In this case, we can determine the final velocity ![]() ) using the equation of motion:
) using the equation of motion:
![]()
This equation is derived from the relationship between acceleration, velocity, and time. By rearranging the terms, we can isolate the acceleration and calculate it using the equation:
![]()
Hence, if we have the initial velocity, final velocity, and time interval, we can easily find the constant acceleration.
The Role of Constant Acceleration in the Equation
Constant acceleration plays a significant role in the equation for finding acceleration using velocity and time. When an object experiences constant acceleration, it means that its velocity is changing at a constant rate over the given time interval. This simplifies the calculations and allows us to accurately determine the acceleration using the formulas mentioned earlier.
How to Calculate Constant Acceleration with Velocity and Time
Now that we have a clear understanding of the mathematical relationship between acceleration, velocity, and time, let’s move on to the step-by-step guide for calculating constant acceleration.
Step-by-Step Guide to Calculating Constant Acceleration
- Determine the initial velocity
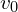 ), final velocity
), final velocity  ), and time interval
), and time interval 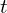 ) for the object in motion.
) for the object in motion. - Subtract the initial velocity from the final velocity to find the change in velocity:
 .
. - Divide the change in velocity by the time interval:
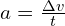 .
. - The result obtained is the constant acceleration of the object.
Worked Out Examples of Calculating Constant Acceleration
Let’s work through a couple of examples to solidify our understanding.
Example 1:
An object starts with an initial velocity of 10 m/s and experiences a constant acceleration for 5 seconds. If the final velocity is 35 m/s, what is the constant acceleration?
![]()
Using the formula ![]() , we can calculate:
, we can calculate:
![]()
Therefore, the constant acceleration is ![]() .
.
Example 2:
A car initially moving at 20 m/s accelerates uniformly for 8 seconds until it reaches a final velocity of 40 m/s. What is the constant acceleration of the car?
![]()
Using the formula ![]() , we can substitute the given values:
, we can substitute the given values:
![]()
Therefore, the constant acceleration of the car is ![]() .
.
Common Mistakes to Avoid When Calculating Constant Acceleration
While calculating constant acceleration, it’s essential to watch out for common mistakes that can lead to inaccurate results. Here are a few errors to avoid:
- Forgetting to subtract the initial velocity from the final velocity when finding the change in velocity
 ).
). - Accidentally swapping the order of the final velocity and initial velocity in the formula
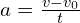 .
. - Failing to convert units consistently throughout the calculation, which can lead to incorrect units in the final answer.
- Rounding off intermediate values too early, as this may introduce rounding errors and affect the accuracy of the final answer.
By being mindful of these potential pitfalls, you can ensure accurate calculations of constant acceleration.
Practical Applications of Calculating Constant Acceleration
Calculating constant acceleration using velocity and time has various practical applications in the real world. Let’s explore a few scenarios where these calculations are employed.
Real-World Scenarios Where Constant Acceleration is Calculated

- Projectile motion: When a projectile, such as a ball thrown into the air, experiences uniform acceleration due to gravity, we can use the formulas discussed to determine its trajectory and various parameters.
- Automotive engineering: Constant acceleration calculations are vital in designing and optimizing cars’ acceleration capabilities, improving fuel efficiency, and ensuring passenger safety.
- Physics experiments: In physics experiments, measuring constant acceleration provides valuable insights into the behavior of objects under different conditions, enabling scientists to develop accurate models and theories.
The Importance of Accurate Calculations in These Scenarios
By understanding how to find constant acceleration using velocity and time, we can contribute to advancements in various fields and enhance our understanding of the physical world.
How can you find constant acceleration with velocity and time, and what role does it play in finding velocity with constant acceleration?
Constant acceleration is an important concept in physics that relates to the change in velocity over time. When we are given the values of velocity and time, we can use these to calculate the constant acceleration experienced by an object. By utilizing the equations and formulas associated with constant acceleration, it is possible to find the change in velocity over a given time period. This information is crucial in determining the effect of acceleration on an object’s velocity. Finding velocity with constant acceleration involves understanding the relationship between time, initial velocity, acceleration, and final velocity. By considering the principles of constant acceleration, we can accurately calculate the final velocity of an object.
Numerical Problems on how to find constant acceleration with velocity and time
Problem 1:
A car starts from rest and accelerates uniformly at a rate of 2 m/s² for 8 seconds. Calculate the final velocity of the car.
Solution:
Given:
Initial velocity, ![]() m/s
m/s
Acceleration, ![]() m/s²
m/s²
Time, ![]() s
s
We can use the equation of motion:
![]()
Substituting the given values:
![]()
Therefore, the final velocity of the car is 16 m/s.
Problem 2:
A train is moving with a velocity of 20 m/s. It accelerates at a constant rate of 3 m/s² for 10 seconds. Find the final velocity of the train.
Solution:
Given:
Initial velocity, ![]() m/s
m/s
Acceleration, ![]() m/s²
m/s²
Time, ![]() s
s
Using the equation of motion:
![]()
Substituting the given values:
![]()
Hence, the final velocity of the train is 50 m/s.
Problem 3:
A rocket is launched vertically upwards with an initial velocity of 50 m/s. The rocket experiences a constant acceleration of 10 m/s². Determine the time taken for the rocket to reach its maximum height.
Solution:
Given:
Initial velocity, ![]() m/s
m/s
Acceleration, ![]() m/s² (negative due to upward direction)
m/s² (negative due to upward direction)
Final velocity, ![]() m/s (at maximum height)
m/s (at maximum height)
We can use the equation of motion:
![]()
Since the rocket reaches its maximum height, the final velocity is 0. Thus, the equation becomes:
![]()
Simplifying the equation:
![]()
![]()
![]() m
m
Now, we can use the equation of motion:
![]()
Substituting the given values:
![]()
![]()
![]() s
s
Therefore, the time taken for the rocket to reach its maximum height is 5 seconds.
Also Read:
- How to find velocity in brane cosmology
- How to find acceleration without final velocity
- Terminal velocity examples
- Escape velocity of sun
- Velocity
- How to find instantaneous velocity from average velocity
- How to find mass with potential energy and velocity
- How to find constant velocity with friction
- Negative velocity and negative acceleration
- How to find magnetic field from velocity
I am Keerthi K Murthy, I have completed post graduation in Physics, with the specialization in the field of solid state physics. I have always consider physics as a fundamental subject which is connected to our daily life. Being a science student I enjoy exploring new things in physics. As a writer my goal is to reach the readers with the simplified manner through my articles.