Explore our comprehensive guide on How To Find Centripetal Acceleration, featuring a variety of use cases and problems, enriched with factual insights to enhance your understanding of this fundamental physics concept.
When an object is moving in a circular motion, a force will tend to pull the object towards the center.
The force that tries to attract the object in a circular motion towards the center is known as the centripetal force, and thus, the centripetal acceleration is the acceleration that acts in it.
As centripetal acceleration consists of both magnitude and direction, it is a vector quantity. In this article, we shall try to find out how to find centripetal acceleration with or without the help of some quantities. The formula for centripetal acceleration is given as: ac = v2/r
Or ![]()
Where,
ac = centripetal acceleration.
v = velocity if the object.
r = radius of the trajectory.
ω = angular velocity.
How To Find Centripetal Acceleration Without Velocity
There are various different ways to find the centripetal acceleration without complete information, depending on what type of information is provided. One such method is finding the centripetal force, though there are a few values that one must have beforehand in order to find any value. The formula for centripetal force is given as: Fc = mv2/r
Where,
Fc= centripetal force.
m = mass of the object.
v = the velocity of the object.
r = radius of the orbit of the object.
As in this section, one needs to find the centripetal acceleration without velocity, assuming that the velocity is not provided in the question. This means that other information like centripetal force, the mass of the object, and radius of the object must be specified in the problem, with the help of which one can find the velocity of the object and then insert it into the formula for centripetal acceleration to obtain the final answer.
Que: What is the centripetal acceleration of a 200 kg vehicle taking a U-turn about a circle whose diameter is 50 m? The force acting on the vehicle is 500 N.
Ans: The radius of the circle can be found by dividing the diameter by 2, as the radius is half of the diameter. Thus, the radius is 25 m. The formula for centripetal force is given as: Fc = mv2/r
Rearrange this formula to obtain the expression for velocity. Therefore,v2 = Fcr/m
Substitute 500 N for Fc, 25 m for r and 200 kg for m into the formula to find the velocity.
The formula for finding centripetal acceleration is given as: ac = v2/r
Substitute 7.91 m/s2 for v and 25 m for r into the formula to calculate centripetal acceleration.
Therefore, the centripetal acceleration of the vehicle is 2.5 m/s2.
How To Find Centripetal Acceleration With Radius And Velocity
The simplest way to calculate the centripetal acceleration is with the help of the velocity of the object traveling in the circular path and the radius of its circular path. Here, the same formula is used as shown earlier, that is, ac = v2/r
Que: An object of mass 3 kg is tied at the end of a rope of length 2 m and revolved around with one end of the rope kept fixed. If it makes 250 rev/min, then find the centripetal acceleration of this object.
ω is given as: ![]()
Where,
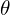 = angular rotation
= angular rotation- t = time
If a body is rotating at ‘N’ number of revolutions per minute, then the formula is given as: ![]()
Where,
T = period for revolution
Here, the period is counted as revolutions per minute. As 1 min = 60 sec, T = 60 s. The S.I. unit for this formula is rad/s. Substitute 250 for N into the formula to calculate the angular velocity.
Now, there are two equations for finding centripetal acceleration: ![]() and
and ![]() . By equating these two equations, we can find the velocity. Therefore,
. By equating these two equations, we can find the velocity. Therefore,
Substitute 2 m for r and 26.16 rad/s for ω into the formula to calculate the velocity.
Now, substitute 52.32 m/s for v and 2 m for r into the formula to calculate centripetal acceleration.
Therefore, the centripetal acceleration of the object is 1368.7 m/s2.
Read about different types of acceleration on Centripetal Acceleration Vs Acceleration
How To Find Centripetal Acceleration Given Time And Radius
One uses the formula involving the angular velocity to find the centripetal acceleration. Using time and radius, the centripetal acceleration, ![]() , is given by
, is given by ![]() .
.
To find the angular velocity, use the formula ![]() .
.
Que: Calculate the centripetal acceleration of a ball tied at the end of a string with a fixed axis 1.5 m away from the center. Spinning 170 rev/min.
Ans: 1 min = 60 sec. Substitute 170 for N and 60 for T into the formula to calculate angular velocity.
Substitute the value of ![]() into the formula for centripetal acceleration involving angular velocity.
into the formula for centripetal acceleration involving angular velocity.
Therefore, the centripetal acceleration of the ball is 474.72 m/s2.
How To Find Centripetal Acceleration Without Mass
There are two main formulae for finding the centripetal acceleration and as observed earlier, any of the centripetal acceleration formula does not involve mass in it, so it is easy to find centripetal acceleration if the rest of the values are given.
Que: Find the centripetal acceleration of a car circling over a cross-road round at the speed of 50 km/hr. The round is about 40 m in length.
Ans: The formula used for this problem will be ac = v2/r
The length of the round means the diameter of the round. As the diameter is 40 m, the radius of the circle will be 20 m. Now, one needs to convert the speed from km/hr into m/s. To convert the speed, one needs to multiply the given speed by 1000 m/3600 sec. Therefore,
Substitute 13.8 m/s for v and 20 m for r into the formula to calculate the centripetal acceleration.
Therefore, the centripetal acceleration of the car is 9.52 m/s2.
How To Find Centripetal Acceleration With Period
The time (T) required for an object to complete one full revolution is known as Period. If the period is mentioned, then one can find the velocity of the object with the help of the period and substitute that value of velocity into the formula for centripetal acceleration. The formula for finding the velocity with the help of period is given as: ![]()
Where,
N = revolutions.
T= time period.
Que: If a propeller of a fighter plane is 2.50 m in diameter and spins at 1100 rev/min, then what is the centripetal acceleration of the propeller tip under these circumstances?
Ans: To find the radius of the propeller, the diameter must be divided by 2. Therefore, the radius of the propeller with the given diameter is 1.25 m. Here, the propeller spins at 1100 revolutions per minute which means that it spins 1100 revolutions per 60 seconds. Therefore, substitute 1100 for N and 60 s for T into the formula to calculate the velocity of the object.
Now, the formula to calculate the centripetal acceleration is given as: ac = v2/r
Substitute 115.13 m/s for v and 1.25 m for r into the formula to calculate the centripetal acceleration of the propeller.
ac = v2/r
= (115.13m/s)2/1.25m
= 10,603.9m/s2
Therefore, the centripetal acceleration of the propeller is 10,603.9 m/s2.
How To Find Centripetal Acceleration From Tangential Acceleration
The magnitude of velocity changing with respect to change in time is known as Tangential acceleration. The formula for tangential acceleration is given as: aT = dv/dt
Where,
aT = tangential acceleration.
dv = change in velocity.
dt = change in time.
The direction of tangential acceleration is denoted by the tangent to the circle, whereas the direction of centripetal acceleration is towards the center of the circle (radially inwards). Therefore, an object in a circular motion with tangential acceleration will experience a total acceleration, which is the sum of tangential acceleration and centripetal acceleration. The formula for total acceleration is given as: a = aT + ac
Where,
a = total acceleration.
aT = tangential acceleration.
ac = centripetal acceleration.

So, if one is provided with total acceleration and tangential acceleration, it is easy to find the centripetal acceleration of any object.
Que: What is the centripetal acceleration of an object that has the net acceleration (total acceleration) of 256.9 m/s2 and tangential acceleration of 101.4 m/s2?
Ans: The given formula for the relation for centripetal acceleration and tangential acceleration is: a = aT + ac
Rearrange the formula to calculate the centripetal acceleration.
ac = a – aT
Substitute 256.9 m/s2 for a and 101.4 m/s2 for aT into the above formula to calculate centripetal acceleration.
Therefore, the centripetal acceleration of the object is 155.5 m/s2.
Another easy way to find centripetal acceleration is by the given formula involving angle, Which is given as: ![]()
Que: Find the centripetal acceleration of an object which makes an angle of 1.6º with respect to the centripetal acceleration vector and has a tangential acceleration of 6.5 m/s2.
Ans: To find the centripetal acceleration, one needs to modify the given equation.
Substitute 6.5 m/s² for ![]() and 1.6° for
and 1.6° for ![]() into the above equation to calculate the centripetal acceleration.
into the above equation to calculate the centripetal acceleration.
Therefore, the centripetal acceleration of the object is 232.7 m/s2.
How To Find Centripetal Acceleration Of A Pendulum
When a pendulum is in motion, centripetal acceleration as well as tangential acceleration act upon it. The net force is accountable for the centripetal acceleration at the bottom of the swing.
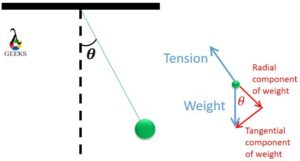
The formula is expressed as: Tension – Weight = m * ac
Where:
- (Tension – Weight) represents the net force.
- m is the mass of the object (such as the bob of a pendulum).
Therefore, this formula can be further written as: ![]()
Where,
T = tension
g = acceleration due to gravity.
One simply needs to rearrange the given equation to find the centripetal acceleration.
![]()
Que: Find the centripetal acceleration of a pendulum of mass 0.250 kg making an angle with the normal of 27°. The tension on the bob is 97 N.
Ans: The value of acceleration due to gravity for the earth is 9.8 m/s2. Substitute 97 N for T, 0.250 kg for m, 27° for ![]() ¸ and 9.8 m/s2 for g into the above formula to calculate the centripetal acceleration.
¸ and 9.8 m/s2 for g into the above formula to calculate the centripetal acceleration.
Therefore, the centripetal acceleration of the pendulum is 379.3 m/s2.
Also Read:
- Negative acceleration example
- How to find acceleration from distance
- How to find acceleration with kinetic friction
- How to find acceleration with coefficient of friction
- Centripetal acceleration vs acceleration
- Instantaneous acceleration vs average acceleration
- How to find acceleration in circular motion
- How to calculate force without acceleration
- Frictional force and centripetal acceleration
- How to find acceleration with mass and air resistance
I am Durva Dave, completed my post-graduation in Physics. Physics fascinates me a lot and I like in knowing the ‘Why’ and ‘How’ of everything that unfolds in our universe. I try to write my blogs in simple yet effective language so that it is easier for the reader to understand as well remember. I hope with my curiosity I am able to provide the readers for what their looking for through my blogs. Let’s connect through LinkedIn.