How to Calculate Terminal Velocity in Fluids: A Comprehensive Guide

Terminal velocity is the maximum speed an object achieves while falling through a fluid, such as air or water. This velocity is reached when the force of gravity acting on the object is balanced by the drag force exerted by the fluid. Calculating the terminal velocity is essential in various fields, including aerodynamics, fluid mechanics, and sports science.

In this comprehensive guide, we will delve into the details of how to calculate terminal velocity in fluids, covering the underlying physics, the formula, and practical examples to help you master this concept.

Understanding the Physics of Terminal Velocity

The terminal velocity of an object falling through a fluid is determined by the balance between the force of gravity and the drag force acting on the object. The force of gravity, which is proportional to the mass of the object, pulls the object downward, while the drag force, which depends on the object’s shape, size, and the fluid’s properties, acts in the opposite direction, slowing the object’s descent.

The formula for terminal velocity is derived from the equation of motion, which states that the net force acting on an object is equal to its mass multiplied by its acceleration. When the object reaches terminal velocity, the net force is zero, and the object’s acceleration is also zero.

The Formula for Terminal Velocity

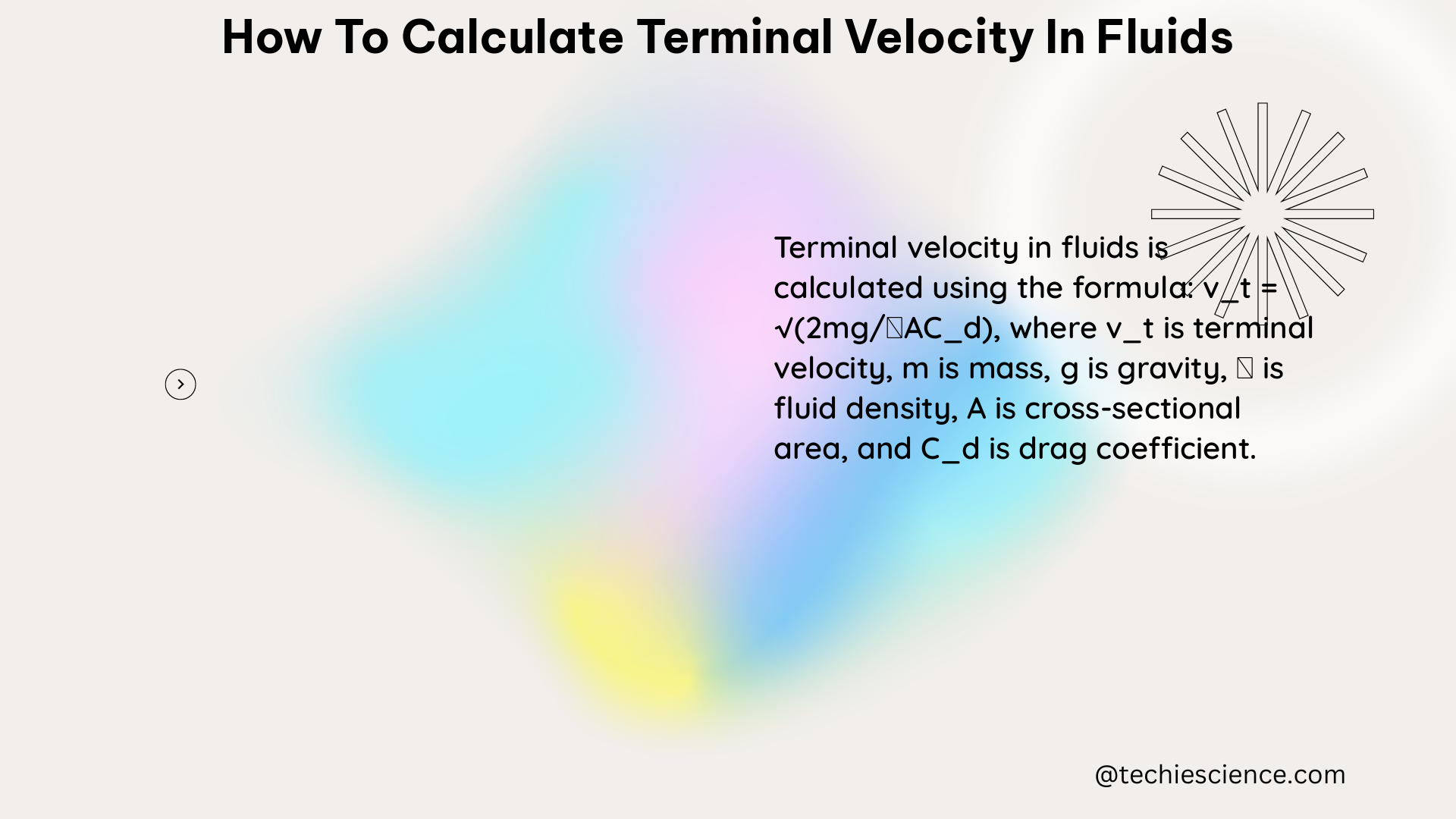
The formula for calculating the terminal velocity of an object falling through a fluid is:

vt = √((2 × m × g) / (ρ × A × Cd))

Where:

• vt is the terminal velocity (in m/s)
• m is the mass of the object (in kg)
• g is the acceleration due to gravity (9.8 m/s²)
• ρ (rho) is the density of the fluid (in kg/m³)
• A is the cross-sectional area of the object (in m²)
• Cd is the drag coefficient of the object (dimensionless)

The drag coefficient, Cd, is a dimensionless quantity that depends on the shape of the object and the flow regime (laminar or turbulent). The drag coefficient can be determined experimentally or obtained from reference tables.

Examples and Calculations

Let’s consider a few examples to illustrate how to calculate the terminal velocity of objects falling through different fluids.

Example 1: Falling Feather in Air

Suppose we have a feather with the following characteristics:
– Mass (m) = 0.001 kg
– Cross-sectional area (A) = 0.0025 m²
– Drag coefficient (Cd) = 0.8 (for a feather)
– Air density (ρ) = 1.225 kg/m³ (at sea level)
– Gravitational acceleration (g) = 9.8 m/s²

Plugging these values into the formula, we get:

vt = √((2 × 0.001 × 9.8) / (1.225 × 0.0025 × 0.8))
   = √((0.0196) / (0.00244))
   = √8.0328
   = 2.83 m/s

Therefore, the terminal velocity of the feather falling through air is approximately 2.83 m/s.

Example 2: Falling Skydiver in Air

Now, let’s consider the case of a skydiver falling through the air:
– Mass (m) = 80 kg (including the skydiver and their equipment)
– Cross-sectional area (A) = 0.8 m² (in a spread-eagle position)
– Drag coefficient (Cd) = 0.7 (for a human in a spread-eagle position)
– Air density (ρ) = 1.225 kg/m³ (at sea level)
– Gravitational acceleration (g) = 9.8 m/s²

Plugging these values into the formula, we get:

vt = √((2 × 80 × 9.8) / (1.225 × 0.8 × 0.7))
   = √((1568) / (0.686))
   = √2286.59
   = 60.8 m/s

Therefore, the terminal velocity of the skydiver falling through air is approximately 60.8 m/s or 137 mph.

Example 3: Falling Sphere in Water

Let’s consider a metal sphere falling through water:
– Mass (m) = 0.5 kg
– Diameter (d) = 0.1 m (cross-sectional area A = π × (d/2)² = 0.00785 m²)
– Drag coefficient (Cd) = 0.47 (for a smooth sphere)
– Water density (ρ) = 1000 kg/m³
– Gravitational acceleration (g) = 9.8 m/s²

Plugging these values into the formula, we get:

vt = √((2 × 0.5 × 9.8) / (1000 × 0.00785 × 0.47))
   = √((9.8) / (3.6795))
   = √2.66
   = 1.63 m/s

Therefore, the terminal velocity of the metal sphere falling through water is approximately 1.63 m/s.

Factors Affecting Terminal Velocity

Several factors can influence the terminal velocity of an object falling through a fluid:

1. Mass: The mass of the object directly affects the force of gravity acting on it. Heavier objects will generally have a higher terminal velocity.

2. Cross-sectional Area: The cross-sectional area of the object determines the drag force acting on it. Larger cross-sectional areas result in higher drag forces and lower terminal velocities.

3. Drag Coefficient: The drag coefficient is a dimensionless quantity that depends on the shape and orientation of the object. Streamlined objects with lower drag coefficients will have higher terminal velocities.

4. Fluid Density: The density of the fluid in which the object is falling affects the drag force. Objects falling through denser fluids, such as water, will have higher terminal velocities compared to those falling through less dense fluids, such as air.

5. Gravitational Acceleration: The acceleration due to gravity, which is approximately 9.8 m/s² on Earth, directly influences the terminal velocity.

Understanding these factors and their interplay is crucial for accurately calculating the terminal velocity of objects in various fluid environments.

Measuring Terminal Velocity

To measure the terminal velocity of an object in a fluid, you can use a simple experimental setup:

1. Obtain a vertical tube or column filled with the fluid of interest (e.g., air or water).
2. Mark two points on the tube at a known distance apart (e.g., 1 meter).
3. Drop the object from the top of the tube and use a stopwatch to measure the time it takes for the object to travel between the two marked points.
4. Calculate the terminal velocity by dividing the distance between the two marks by the measured time.

This method allows you to directly observe and measure the terminal velocity of the object in the fluid environment.

Conclusion

Calculating the terminal velocity of objects falling through fluids is a fundamental concept in physics and engineering. By understanding the underlying physics, the formula, and the various factors that influence terminal velocity, you can accurately determine the maximum speed an object will achieve while falling through a fluid.

This comprehensive guide has provided you with the necessary tools and examples to master the calculation of terminal velocity in fluids. Remember to consider the object’s mass, cross-sectional area, drag coefficient, and the fluid’s density to arrive at the correct terminal velocity.

With this knowledge, you can apply these principles to a wide range of applications, from designing efficient aircraft and parachutes to understanding the motion of falling objects in various environments.

References:

• Fluid Mechanics, 7th Edition, by Frank M. White
• Introduction to Fluid Mechanics, 8th Edition, by Robert W. Fox, Alan T. McDonald, Philip J. Pritchard
• Aerodynamics for Engineers, 6th Edition, by John J. Bertin, Russell M. Cummings