How to Calculate Mass from Gravitational Force
In this blog post, we will explore the concept of calculating mass from gravitational force. We will start by understanding the key terms related to this topic, such as gravitational force, mass in physics, and the concept of gravity. Then, we will dive into the gravity equation and its importance in calculations.
Definition of Key Terms
- Understanding Gravitational Force:
Gravitational force is the attractive force between two objects with mass. It is responsible for keeping planets in orbit around the sun and objects grounded on Earth. The force of gravity can be calculated using the equation:
![]()
where ![]() is the gravitational force,
is the gravitational force, ![]() is the gravitational constant,
is the gravitational constant, ![]() and
and ![]() are the masses of the two objects, and
are the masses of the two objects, and ![]() is the distance between them.
is the distance between them.
- Defining Mass in Physics:
In physics, mass refers to the amount of matter an object contains. It is a fundamental property of an object and is measured in kilograms (kg). Mass can be calculated using the formula:
![]()
where ![]() is the mass,
is the mass, ![]() is the gravitational force acting on the object, and
is the gravitational force acting on the object, and ![]() is the acceleration due to gravity.
is the acceleration due to gravity.
- The Concept of Gravity:
Gravity is the force that attracts two objects with mass towards each other. It is what gives weight to objects and keeps them grounded on the surface of the Earth. The force of gravity is directly proportional to the masses of the objects and inversely proportional to the square of the distance between them.
The Gravity Equation
- Breaking Down the Gravity Formula:
The gravity formula, also known as Newton’s law of gravitation, is given by:
![]()
This equation allows us to calculate the gravitational force between two objects. ![]() represents the gravitational force,
represents the gravitational force, ![]() is the gravitational constant
is the gravitational constant ![]() ),
), ![]() and
and ![]() are the masses of the two objects, and
are the masses of the two objects, and ![]() is the distance between them.
is the distance between them.
- Importance of the Gravity Equation in Calculations:
The gravity equation is crucial in various fields of science and engineering. It helps us understand the behavior of celestial bodies, calculate the gravitational force between objects, determine the motion of satellites, and even explore the universe. Without this equation, our understanding of the cosmos and the physical world would be severely limited.
The Relationship Between Gravitational Force and Mass
A. Does Gravitational Force Depend on Mass?
-
Exploring the Dependency of Gravitational Force on Mass:
Yes, the gravitational force between two objects depends on their masses. According to Newton’s law of gravitation, the force of gravity is directly proportional to the product of the masses of the two objects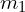 and
and  ). As the masses increase, so does the gravitational force between them.
). As the masses increase, so does the gravitational force between them. -
How Mass Affects Gravitational Force:
Increasing the mass of one or both objects involved in the gravitational interaction will result in a stronger gravitational force. Conversely, decreasing the mass will lead to a weaker force of gravity. This relationship highlights the importance of mass in determining the strength of gravitational interactions.
B. How Gravitational Force is Related to Mass and Distance
-
The Role of Distance in Gravitational Force:
The distance between two objects also plays a crucial role in determining the gravitational force between them. According to the inverse square law, the force of gravity decreases as the distance between the objects increases. In other words, the force is inversely proportional to the square of the distance ).
). -
The Interplay Between Mass, Distance, and Gravitational Force:
The interplay between mass and distance is evident in the gravity equation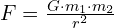 ). As the masses of the objects increase, the force of gravity becomes stronger. Similarly, as the distance between the objects decreases, the force of gravity also increases. These relationships highlight how both mass and distance affect the gravitational force between two objects.
). As the masses of the objects increase, the force of gravity becomes stronger. Similarly, as the distance between the objects decreases, the force of gravity also increases. These relationships highlight how both mass and distance affect the gravitational force between two objects.
Calculating Mass Given Gravitational Force

A. Steps to Calculate Mass Using Gravitational Force
- Detailed Process of Calculating Mass:
To calculate mass from gravitational force, we can rearrange the mass formula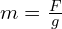 ) to solve for mass
) to solve for mass  ). The steps involved are as follows:
). The steps involved are as follows: - Identify the gravitational force acting on the object
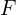 ).
). - Determine the acceleration due to gravity
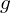 ).
). -
Substitute the values into the formula
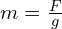 to calculate the mass.
to calculate the mass. -
Worked Out Examples for Better Understanding:
Let’s consider an example to demonstrate the calculation of mass from gravitational force. Suppose an object experiences a gravitational force of 50 N and the acceleration due to gravity is 9.8 m/s^2. Using the formula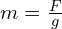 , we can calculate the mass as follows:
, we can calculate the mass as follows:
![]()
Therefore, the mass of the object is 5.10 kg.
B. How to Use the Gravity Formula to Find Mass
-
Practical Application of the Gravity Formula:
The gravity formula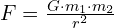 ) can also be used to find the mass of an object. By rearranging the equation, we can solve for mass
) can also be used to find the mass of an object. By rearranging the equation, we can solve for mass 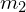 ) when the gravitational force
) when the gravitational force 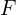 ), the mass of the first object
), the mass of the first object 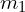 ), and the distance between the objects
), and the distance between the objects  ) are known.
) are known. -
Examples of Using the Gravity Formula to Calculate Mass:
Let’s consider an example where the gravitational force between two objects is 100 N, the distance between them is 2 meters, and the mass of one object is 5 kg. We can use the gravity formula to find the mass of the second object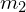 ) as follows:
) as follows:
![]()
Therefore, the mass of the second object is approximately ![]() kg.
kg.
Calculating Gravitational Force Without Mass

A. Understanding the Process of Calculating Gravitational Force
- Steps to Calculate Gravitational Force Without Mass:
The gravitational force between two objects can be calculated even when the masses of the objects are unknown. If we know the acceleration due to gravity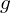 ) and the mass of one of the objects
) and the mass of one of the objects 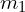 ), we can find the gravitational force
), we can find the gravitational force 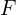 ). The steps involved are as follows:
). The steps involved are as follows: - Identify the acceleration due to gravity
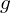 ).
). - Determine the mass of one of the objects involved
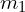 ).
). -
Substitute the values into the formula
 and solve for
and solve for 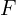 .
. -
Examples to Illustrate the Calculation Process:
Let’s consider an example where the acceleration due to gravity is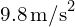 , the mass of one object is
, the mass of one object is 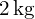 , and the distance between the objects is
, and the distance between the objects is  . Using the formula
. Using the formula  , we can calculate the gravitational force as follows:
, we can calculate the gravitational force as follows:
![]()
Since the mass of the second object ![]() ) is unknown, we cannot calculate the exact value of the gravitational force. However, we can see that the force will be directly proportional to
) is unknown, we cannot calculate the exact value of the gravitational force. However, we can see that the force will be directly proportional to ![]() and inversely proportional to the square of the distance.
and inversely proportional to the square of the distance.
B. How to Calculate Gravitational Force Weight
- The Relationship Between Gravitational Force and Weight:
Weight is the force experienced by an object due to gravity. The weight of an object can be calculated using the formula:
![]()
where ![]() is the weight,
is the weight, ![]() is the mass of the object, and
is the mass of the object, and ![]() is the acceleration due to gravity.
is the acceleration due to gravity.
- Steps and Examples to Calculate Weight from Gravitational Force:
To calculate weight from gravitational force, we can rearrange the weight formula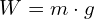 ) to solve for the mass
) to solve for the mass  ). The steps involved are as follows:
). The steps involved are as follows: - Identify the gravitational force acting on the object
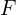 ).
). - Determine the acceleration due to gravity
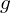 ).
). - Substitute the values into the formula
 to calculate the weight.
to calculate the weight.
Let’s consider an example where the gravitational force acting on an object is ![]() and the acceleration due to gravity is
and the acceleration due to gravity is ![]() . Using the formula
. Using the formula ![]() , we can calculate the weight as follows:
, we can calculate the weight as follows:
![]()
Therefore, the weight of the object is approximately ![]() .
.
Recap of How to Calculate Mass from Gravitational Force
In this blog post, we have discussed how to calculate mass from gravitational force. We started by understanding the key terms, such as gravitational force, mass in physics, and the concept of gravity. Then, we explored the gravity equation and its importance in calculations.
We also delved into the relationship between gravitational force and mass, highlighting how mass affects gravitational force and the interplay between mass, distance, and gravitational force. Additionally, we learned how to calculate mass given gravitational force and how to calculate gravitational force without mass.
Understanding these calculations is crucial in the fields of physics and engineering. They allow us to analyze the behavior of celestial bodies, calculate the motion of satellites, and comprehend the fundamental laws of nature. I encourage you to further explore and learn about these fascinating concepts to deepen your understanding of the universe and our place within it.
How can mass be calculated using both gravitational force and force and distance?
Calculating mass involves various methods depending on the available information. One method is calculating mass using gravitational force. This approach utilizes Newton’s law of universal gravitation to determine the mass of an object based on the gravitational force acting on it. Another method is calculating mass using force and distance. This technique involves applying Newton’s second law of motion and considering the force applied to an object and the distance it moves. By integrating these two methods, we can determine mass using both gravitational force and force and distance. To learn more about calculating mass using force and distance, visit Calculating mass using force and distance.
Numerical Problems on how to calculate mass from gravitational force
Problem 1:
A satellite is in orbit around a planet with a gravitational force of 2.5 N. If the distance between the satellite and the planet is 500 km, calculate the mass of the planet.
Solution:
Given:
Gravitational force (F) = 2.5 N
Distance (r) = 500 km = 500,000 m
Using the formula for gravitational force:
![]()
where:
G is the gravitational constant,
m_1 and m_2 are the masses of the two objects, and
r is the distance between the objects.
To calculate the mass of the planet (m_2), rearrange the formula:
![]()
Substituting the given values:
![]()
The values for G and m_1 are constants, so let’s assume G = 6.67 × 10^-11 N m^2/kg^2 and m_1 = 1 kg for simplicity.
![]()
![]()
Simplifying the expression, we get:
![]()
Therefore, the mass of the planet is approximately 9.375 × 10^12 kg.
Problem 2:
The gravitational force between two objects is 6.7 × 10^-8 N. If the masses of the objects are 2 kg and 4 kg respectively, calculate the distance between them.
Solution:
Given:
Gravitational force (F) = 6.7 × 10^-8 N
Mass of object 1 (m_1) = 2 kg
Mass of object 2 (m_2) = 4 kg
Using the formula for gravitational force:
![]()
Rearranging the formula to solve for the distance (r):
![]()
Substituting the given values:
![]()
Simplifying the expression, we get:
![]()
![]()
![]()
![]()
Therefore, the distance between the two objects is approximately 2.82 × 10^4 meters.
Problem 3:
The gravitational force between two objects is 9.8 N. If the distance between them is 10 meters, calculate the combined mass of the objects.
Solution:
Given:
Gravitational force (F) = 9.8 N
Distance (r) = 10 m
Using the formula for gravitational force:
![]()
Rearranging the formula to solve for the combined mass (m_1 + m_2):
![]()
Substituting the given values and assuming G = 6.67 × 10^-11 N m^2/kg^2:
![]()
Simplifying the expression, we get:
![]()
![]()
Therefore, the combined mass of the objects is approximately 14.79 × 10^9 kg.
Also Read:
- How to find potential energy with height and mass
- How to calculate speed from force and mass
- Magnetic force and mass
- How to calculate mass from weight
- Conservation of mass
- How to find kinetic energy with mass and height
- Biomass energy
- How to find normal force with mass
- How to find binding energy from mass defect
- Disadvantages of biomass

Hello, I’m Manish Naik completed my MSc Physics with Solid-State Electronics as a specialization. I have three years of experience in Article Writing on Physics subject. Writing, which aimed to provide accurate information to all readers, from beginners and experts.
In my leisure time, I love to spend my time in nature or visiting historical places.
Looking forward to connecting you through LinkedIn –