Ankita Biswas

Hi...I am Ankita Biswas. I have done my B.Sc in physics Honours and my M.Sc in Electronics. Currently, I am working as a Physics teacher in a Higher Secondary School. I am very enthusiastic about the high-energy physics field. I love to write complicated physics concepts in understandable and simple words.

Does Aluminum Foil Conduct Electricity?

by
does aluminum foil conduct electricity

Aluminum foil is a highly conductive material that can easily allow the flow of electric charge through it, making it a useful material for a variety of electrical applications. This is due to its high electrical conductivity, which is a measure of how well a material can conduct electricity. Understanding Electrical Conductivity Electrical conductivity is … Read more

The Definitive Guide to Understanding the Direction of Angular Displacement

by
direction of angular displacement

The direction of angular displacement is a crucial concept in rotational kinematics, as it describes the orientation of an object’s rotation around a fixed axis. This guide will provide a comprehensive overview of the topic, covering the fundamental principles, mathematical representations, and practical applications. Understanding Angular Displacement as a Vector Quantity Angular displacement is a … Read more

A Comprehensive Guide to Angular Displacement and Angular Velocity

by
angular displacement and angular velocity

Angular displacement and angular velocity are fundamental concepts in the study of rotational motion, which is a crucial aspect of classical mechanics. These two quantities are closely related and play a vital role in understanding the behavior of rotating objects. In this comprehensive guide, we will delve into the intricacies of angular displacement and angular … Read more

Exploring the Intricacies of Magnetic Field and Time

by
magnetic field and time

The strength of a magnetic field is a crucial parameter in various scientific and technological applications, ranging from geophysical exploration to medical imaging. The measurement and analysis of magnetic fields are often intertwined with the concept of time, as the temporal variations of these fields can provide valuable insights into the underlying physical processes. In this comprehensive blog post, we will delve into the intricate relationship between magnetic field and time, exploring the technical details, practical applications, and the latest advancements in this fascinating field of study.

Understanding Magnetic Field Strength

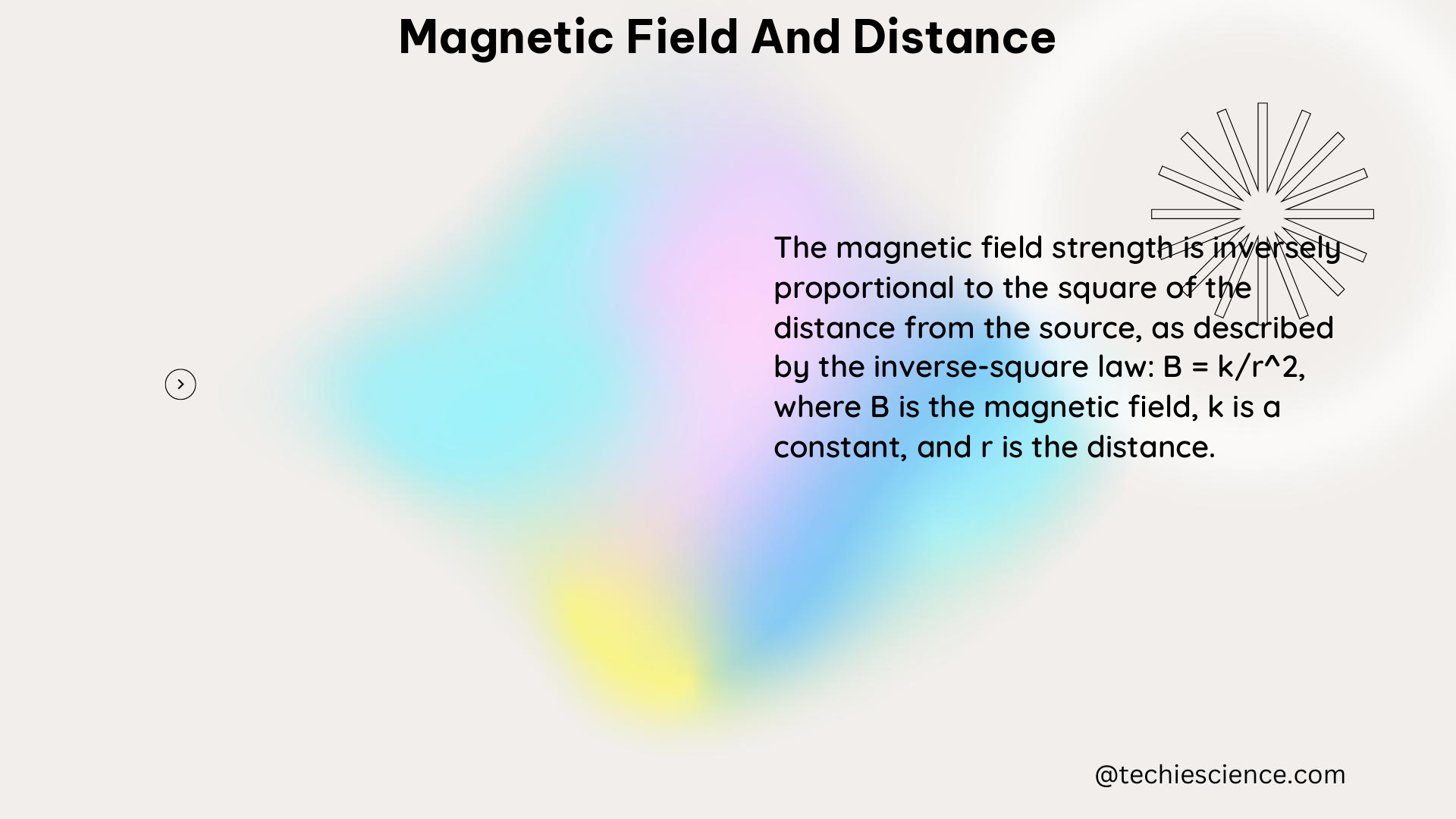
Magnetic field strength is typically measured in units of tesla (T) in the SI system or gauss (G) in the CGS system, where 1 T is equivalent to 10,000 G. The Earth’s magnetic field, for instance, can vary from 20,000 to 80,000 nanotesla (nT), depending on the location. This variation is due to the complex interplay of various factors, including the Earth’s core dynamics, solar activity, and local geological features.

To measure magnetic fields, scientists and engineers employ a range of specialized instruments called magnetometers. These devices come in different types, such as gaussmeters and teslameters, each designed to measure magnetic fields in their respective units. The choice of magnetometer depends on the specific application and the required level of precision.

Magnetic Field Measurement Techniques

  1. Aeromagnetic Surveys: These surveys are conducted from aircraft, providing a comprehensive mapping of the Earth’s magnetic field over large areas. Aeromagnetic data is used to search for mineral deposits, map geological structures, and even locate lost objects.

  2. Borehole Surveys: Magnetometers are lowered into boreholes to measure the magnetic field within the subsurface, which can reveal information about the geological composition and structure of the Earth’s crust.

  3. Ground Surveys: Ground-based magnetic surveys involve the use of portable magnetometers to measure the magnetic field at specific locations on the Earth’s surface. These surveys are often used in archaeological investigations and mineral exploration.

  4. Marine Surveys: Magnetometers mounted on ships or towed behind them are used to map the magnetic field of the seafloor, which can provide insights into the Earth’s geological history and the presence of mineral resources.

  5. Magnetovision Imaging: This technique involves the generation of images based on the spatial distribution of magnetic field parameters, allowing for detailed analysis and data fusion with other geophysical data.

  6. Magnetic Gradiometers: These instruments consist of pairs of magnetometers with their sensors separated by a fixed distance. They measure the difference between the sensed magnetic fields, enabling the detection of magnetic anomalies and their gradients, which can be useful in archaeological and site investigation work.

Temporal Aspects of Magnetic Fields

magnetic field and time

The performance and capabilities of magnetometers are often described through technical specifications, including the sample rate (number of readings per second) and cycle time (seconds per reading). These parameters are particularly important in mobile surveys, where accurate measurements are crucial for mapping the spatial and temporal variations of magnetic fields.

Magnetic Field Variations over Time

  1. Diurnal Variations: The Earth’s magnetic field exhibits daily (diurnal) variations due to the interaction between the Earth’s magnetic field and the ionosphere, which is influenced by solar activity.

  2. Secular Variations: The Earth’s magnetic field also undergoes gradual changes over longer timescales, known as secular variations. These changes are caused by the complex dynamics within the Earth’s core and can be used to study the planet’s geological history.

  3. Magnetic Storms: Sudden and intense disturbances in the Earth’s magnetic field, known as magnetic storms, are often triggered by solar activity, such as coronal mass ejections. These events can have significant impacts on various technological systems, including communication networks and power grids.

  4. Magnetic Pulsations: Rapid fluctuations in the Earth’s magnetic field, known as magnetic pulsations, can occur due to various factors, such as solar wind interactions and magnetospheric processes. These pulsations can provide insights into the dynamics of the Earth’s magnetosphere.

Temporal Sampling and Resolution

The temporal sampling and resolution of magnetic field measurements are crucial for capturing the dynamic nature of these fields. High sample rates and short cycle times are essential for accurately mapping the spatial and temporal variations of magnetic fields, particularly in mobile surveys or when studying rapid magnetic field changes.

For example, in aeromagnetic surveys, the sample rate can range from 10 to 20 readings per second, ensuring that the aircraft’s motion is adequately captured. In contrast, ground-based surveys may have lower sample rates, but the cycle time is still an important factor in ensuring the temporal resolution of the measurements.

Practical Applications and Advancements

The measurement and analysis of magnetic fields over time have numerous practical applications, ranging from geophysical exploration to medical imaging and beyond.

Geophysical Exploration

Magnetic surveys, both in the air and on the ground, are widely used in the search for mineral deposits, such as iron ore, copper, and gold. The temporal variations of magnetic fields can also provide insights into the Earth’s geological history and the presence of hidden structures or anomalies.

Archaeology and Site Investigation

Magnetic gradiometers, which measure the spatial gradients of magnetic fields, have become invaluable tools in archaeological investigations. These instruments can detect subtle magnetic anomalies, which may indicate the presence of buried structures, artifacts, or other features of archaeological significance.

Medical Imaging

Magnetometers play a crucial role in medical imaging techniques, such as magnetoencephalography (MEG) and magnetocardiography (MCG). These methods use the magnetic fields generated by the brain and heart, respectively, to provide non-invasive insights into their function and structure.

Space Weather Monitoring

The monitoring of the Earth’s magnetic field, including its temporal variations, is essential for understanding and predicting space weather events, such as solar storms and their potential impacts on technological systems.

Advancements in Magnetometer Technology

Ongoing research and development in magnetometer technology have led to the creation of more sensitive, accurate, and compact devices. For example, the emergence of quantum magnetometers has significantly improved the precision and stability of magnetic field measurements, opening up new possibilities in various applications.

Conclusion

The interplay between magnetic field and time is a fascinating and multifaceted topic that encompasses a wide range of scientific and technological disciplines. From geophysical exploration to medical imaging, the measurement and analysis of magnetic fields over time have become essential tools for understanding the complex dynamics of our planet and the universe beyond. As research and technological advancements continue to push the boundaries of what is possible, the future of magnetic field and time studies holds the promise of even greater insights and applications.

References:

  1. Blakely, R. J. (1995). Potential Theory in Gravity and Magnetic Applications. Cambridge University Press.
  2. Hinze, W. J., Von Frese, R. R., & Saad, A. H. (2013). Gravity and Magnetic Exploration: Principles, Practices, and Applications. Cambridge University Press.
  3. Merrill, R. T., McElhinny, M. W., & McFadden, P. L. (1996). The Magnetic Field of the Earth: Paleomagnetism, the Core, and the Deep Mantle. Academic Press.
  4. Paperno, E., Lehtinen, J., & Iivanainen, J. (2015). Magnetocardiography. Wiley Encyclopedia of Electrical and Electronics Engineering.
  5. Vrba, J., & Robinson, S. E. (2001). Signal Processing in Magnetoencephalography. Methods, 25(2), 249-271.

Magnetic Flux and Magnetic Induction 2: A Comprehensive Guide

by
magnetic flux and magnetic induction 2

Magnetic flux and magnetic induction are fundamental concepts in electromagnetism, describing the behavior of magnetic fields and the electromotive force induced in coils due to changing magnetic flux. This comprehensive guide delves into the technical details, formulas, and applications of these principles, providing a valuable resource for physics students and enthusiasts.

Understanding Magnetic Flux

Magnetic flux, denoted by the Greek letter Phi or the Phi suffix B (ϕ or ϕB), is the measure of the total magnetic field that penetrates a specified closed surface. It is quantified by counting the magnetic field lines that intersect the surface. The SI unit of magnetic flux is the Weber (Wb).

The formula for calculating the magnetic flux through a coil cross-sectional area is:

ϕB = BA cos θ

Where:
– ϕB is the magnetic flux (in Webers)
– B is the magnetic field strength (in Teslas)
– A is the cross-sectional area of the coil (in square meters)
– θ is the angle between the magnetic field lines and the normal to the coil’s surface (in radians)

This formula demonstrates the relationship between the magnetic field, the area of the coil, and the angle at which the magnetic field lines pass through the coil.

Magnetic Flux Density

Magnetic flux density, also known as magnetic induction, is the physical quantity used as one of the fundamental measures of the intensity of a magnetic field. It is represented by the symbol B and its SI unit is the Tesla (T).

The magnetic flux density is defined as the magnetic flux per unit area, and it can be calculated using the formula:

B = ϕB / A

Where:
– B is the magnetic flux density (in Teslas)
– ϕB is the magnetic flux (in Webers)
– A is the cross-sectional area (in square meters)

Magnetic flux density is an important concept in various applications, such as the design of electrical machines, transformers, and magnetic resonance imaging (MRI) systems.

Faraday’s Law of Induction

magnetic flux and magnetic induction 2

Faraday’s law of induction describes the relationship between the changing magnetic flux and the induced electromotive force (EMF) in a coil. The formula for the induced EMF is:

V = -N (dϕB/dt)

Where:
– V is the induced EMF (in volts)
– N is the number of turns in the coil
– dϕB/dt is the rate of change of the magnetic flux with respect to time (in Webers per second)

The negative sign in the formula indicates that the induced EMF opposes the change in the magnetic flux, as described by Lenz’s law.

Applications of Faraday’s Law

Faraday’s law of induction has numerous applications in various fields, including:

  1. Electrical Generators: The principle of electromagnetic induction, as described by Faraday’s law, is the basis for the operation of electrical generators, where the relative motion between a conductor and a magnetic field induces an EMF.

  2. Transformers: Transformers rely on the principle of electromagnetic induction to transfer electrical energy between two or more circuits through a shared magnetic field.

  3. Eddy Current Brakes: Eddy current brakes use the induced EMF in a conductive material to create a braking force, which is useful in applications such as elevators, cranes, and roller coasters.

  4. Magnetic Induction Accelerometers: The acceleration due to gravity (g) can be measured using magnetic induction, where a falling bar magnet induces a voltage in a series of coils, and the crossing times are used to calculate the value of g.

Measuring Acceleration Due to Gravity (g) Using Magnetic Induction

One practical application of magnetic flux and induction is the measurement of the acceleration due to gravity (g) using a magnetic induction-based approach. This method involves the following steps:

  1. Signal Acquisition: The signal is acquired using an Arduino Uno board, which is a popular open-source microcontroller platform.

  2. Data Analysis: The acquired signal is analyzed using a Python-based graphical user interface (GUI), which allows for the processing and visualization of the data.

  3. Calculation of g: The acceleration due to gravity (g) is calculated by measuring the crossing times of a bar magnet as it falls simultaneously through a number of coils. This method is based on Faraday’s law of induction and the relationship between the magnetic flux and the magnetic field.

The key steps in this process are:

  1. Dropping a bar magnet through a series of coils
  2. Measuring the time it takes for the magnet to pass through each coil
  3. Calculating the acceleration due to gravity (g) using the crossing times and the known distance between the coils

By applying the principles of magnetic flux and induction, this method provides a practical and accessible way to measure the acceleration due to gravity, making it a valuable tool for physics education and research.

Numerical Examples and Problems

To further illustrate the concepts of magnetic flux and induction, let’s consider some numerical examples and problems:

Example 1: Calculating Magnetic Flux

A circular coil with a radius of 5 cm is placed in a uniform magnetic field of 0.8 T, with the magnetic field lines perpendicular to the plane of the coil. Calculate the magnetic flux through the coil.

Given:
– Radius of the coil, r = 5 cm = 0.05 m
– Magnetic field strength, B = 0.8 T
– Angle between the magnetic field and the normal to the coil’s surface, θ = 0° (perpendicular)

Using the formula: ϕB = BA cos θ
ϕB = (0.8 T) × (π × (0.05 m)^2) × cos(0°)
ϕB = 0.0628 Wb

Therefore, the magnetic flux through the circular coil is 0.0628 Wb.

Problem 1: Induced EMF in a Coil

A coil with 100 turns is placed in a uniform magnetic field of 0.5 T. The coil has a cross-sectional area of 0.02 m^2 and is rotated from a position where the magnetic field is perpendicular to the coil’s surface to a position where the magnetic field is parallel to the coil’s surface in 0.1 seconds.

Calculate the induced EMF in the coil.

Given:
– Number of turns in the coil, N = 100
– Magnetic field strength, B = 0.5 T
– Cross-sectional area of the coil, A = 0.02 m^2
– Time taken to rotate the coil, t = 0.1 s
– Initial angle, θ = 90° (perpendicular)
– Final angle, θ = 0° (parallel)

Using the formula: V = -N (dϕB/dt)
dϕB/dt = (ϕB,final – ϕB,initial) / t
dϕB/dt = [(BA cos 0°) – (BA cos 90°)] / 0.1 s
dϕB/dt = (0.5 T × 0.02 m^2 × 1 – 0.5 T × 0.02 m^2 × 0) / 0.1 s
dϕB/dt = 0.1 Wb/s

Substituting in the formula for induced EMF:
V = -N (dϕB/dt)
V = -100 × 0.1 Wb/s
V = -10 V

Therefore, the induced EMF in the coil is -10 V.

These examples and problems demonstrate the application of the formulas and principles related to magnetic flux and induction, providing a deeper understanding of these concepts.

Conclusion

Magnetic flux and magnetic induction are fundamental concepts in electromagnetism that are crucial for understanding the behavior of magnetic fields and the induced electromotive force in coils. This comprehensive guide has explored the technical details, formulas, and applications of these principles, including the measurement of the acceleration due to gravity using magnetic induction.

By delving into the specifics of magnetic flux, flux density, Faraday’s law of induction, and practical applications, this guide aims to serve as a valuable resource for physics students and enthusiasts, providing a solid foundation in these essential electromagnetic phenomena.

References

  1. Geeksforgeeks.org. (n.d.). Magnetic Flux. [online] Available at: https://www.geeksforgeeks.org/magnetic-flux/ [Accessed 1 May 2023].
  2. IOP Publishing. (2023). Measuring the acceleration due to gravity using magnetic induction. [online] Available at: https://iopscience.iop.org/article/10.1088/1361-6552/acb033 [Accessed 1 May 2023].
  3. e-magnetica.pl. (n.d.). Magnetic Flux Density. [online] Available at: https://www.e-magnetica.pl/doku.php/magnetic_flux_density [Accessed 1 May 2023].
  4. Phys.libretexts.org. (n.d.). 22.1: Magnetic Flux, Induction, and Faraday’s Law. [online] Available at: https://phys.libretexts.org/Bookshelves/University_Physics/Physics_%28Boundless%29/22%3A_Induction_AC_Circuits_and_Electrical_Technologies/22.1%3A_Magnetic_Flux_Induction_and_Faradays_Law [Accessed 1 May 2023].

Can Displacement Be Negative?

by
can displacement be negative

Displacement, as a vector quantity, can indeed be negative. This is because displacement is not just about the distance covered by an object, but also about the direction of its motion. In physics, a negative displacement simply means that an object has moved in the opposite direction of the positive direction that has been chosen for the coordinate system.

Theorem and Physics Formula

  1. Displacement as a Vector Quantity: Displacement is a vector quantity, represented by the symbol $\vec{s}$.
  2. Magnitude of Displacement: The magnitude of displacement is given by $|\vec{s}| = \sqrt{x^2 + y^2 + z^2}$, where $x$, $y$, and $z$ are the components of the displacement vector in the $x$, $y$, and $z$ directions, respectively.
  3. Direction of Displacement: The direction of displacement is given by the unit vector $\hat{s} = \vec{s}/|\vec{s}|$.
  4. Average Velocity: The average velocity of an object is given by $\vec{v}_{avg} = \Delta\vec{s}/\Delta t$, where $\Delta\vec{s}$ is the displacement of the object during the time interval $\Delta t$.
  5. Instantaneous Velocity: The instantaneous velocity of an object is given by $\vec{v} = \lim_{\Delta t\to 0} \Delta\vec{s}/\Delta t = ds/dt$, where $s$ is the magnitude of the displacement vector and $t$ is the time.
  6. Acceleration: The acceleration of an object is given by $\vec{a} = d\vec{v}/dt = d^2\vec{s}/dt^2$.

Physics Examples

can displacement be negative

Example 1: A car moves along a straight road for 50 meters in a direction 30 degrees east of north. Calculate the displacement of the car.

Solution:
$\vec{s} = x\hat{i} + y\hat{j}$
$x = 50\ \text{m}\ \cos(30^\circ) = 43.30\ \text{m}$
$y = 50\ \text{m}\ \sin(30^\circ) = 25.00\ \text{m}$
$\vec{s} = 43.30\ \text{m}\ \hat{i} + 25.00\ \text{m}\ \hat{j}$

Example 2: A ball is thrown vertically upward with an initial velocity of 20 m/s. Calculate the displacement of the ball after 2 seconds.

Solution:
$s = v_0t + \frac{1}{2}at^2$
$s = 20\ \text{m/s}\ \times\ 2\ \text{s} – \frac{1}{2}\ \times\ 9.8\ \text{m/s}^2\ \times\ (2\ \text{s})^2 = 30.4\ \text{m}$
The displacement of the ball after 2 seconds is 30.4 meters upward.

Physics Numerical Problems

  1. A car moves along a straight road for 100 meters in a direction 60 degrees east of north. Calculate the displacement of the car.
  2. A ball is thrown vertically downward with an initial velocity of 30 m/s. Calculate the displacement of the ball after 3 seconds.
  3. A runner runs around a circular track with a radius of 50 meters. If the runner starts at the south end of the track and runs clockwise for 200 meters, calculate the displacement of the runner.
  4. A car moves along a straight road for 50 meters in a direction 30 degrees east of north, and then turns around and moves back for 30 meters in a direction 60 degrees west of north. Calculate the displacement of the car.
  5. A ball is thrown vertically upward with an initial velocity of 15 m/s. Calculate the displacement of the ball after 1.5 seconds.

Figures and Data Points

Figure 1: Displacement vector of a car moving along a straight road.

Displacement vector

Data Point 1: Displacement of a car moving along a straight road.

Distance Direction Displacement
100 m 60 degrees east of north 86.6 m, 60 degrees east of north

Data Point 2: Displacement of a ball thrown vertically upward.

Time Displacement
0 s 0 m
1 s 14.7 m
2 s 30.4 m
3 s 46.3 m
4 s 62.2 m

Values and Measurements

The magnitude of the displacement vector can be measured in meters, feet, or any other unit of length. The direction of the displacement vector can be measured in degrees or radians relative to a reference direction.

The initial and final positions of the object can be measured using a ruler or a tape measure. The time can be measured using a stopwatch or a clock.

The acceleration due to gravity can be measured using a accelerometer or by using the formula $g = 9.8\ \text{m/s}^2$.

References

  1. Can displacement be negative? – Socratic
  2. Can displacement be negative? – BYJU’S
  3. Displacement: Unraveling Displacement and Its Impact on Velocity – Faster Capital
  4. Can displacement be negative after calculation? – Physics Stack Exchange
  5. Can Displacement Be Negative? | AcademicHelp.net

Is Distance Always Positive?

by
is distance always positive

Distance is a fundamental concept in physics, and it is often used in conjunction with other variables such as time, velocity, and acceleration to describe the motion of objects. While distance is generally considered a positive quantity, there are certain contexts where negative values may be used to represent reverse travel or displacement in the negative direction.

Understanding Distance as a Scalar Quantity

Distance is a scalar quantity, which means it has only magnitude and no direction. This is in contrast to vector quantities, such as displacement, velocity, and acceleration, which have both magnitude and direction. As a scalar quantity, distance is always positive and cannot be negative.

The formula for calculating distance is:

d = |x2 - x1|

where d represents the distance, x2 is the final position, and x1 is the initial position. The absolute value function |x2 - x1| ensures that the distance is always positive, regardless of the direction of motion.

Negative Values in Displacement and Velocity

is distance always positive

While distance itself is always positive, the related quantities of displacement and velocity can have negative values. Displacement is a vector quantity that represents the change in position of an object, and it can be positive or negative depending on the direction of motion.

The formula for calculating displacement is:

Δx = x2 - x1

where Δx represents the displacement, x2 is the final position, and x1 is the initial position. If the object moves in the positive direction, the displacement will be positive. If the object moves in the negative direction, the displacement will be negative.

Similarly, velocity is a vector quantity that represents the rate of change of an object’s position. Velocity can be positive or negative, depending on the direction of motion. The formula for calculating velocity is:

v = Δx / Δt

where v represents the velocity, Δx is the displacement, and Δt is the change in time.

Negative Values in Physics Experiments and Data Analysis

In physics experiments and data analysis, negative values may be used to represent reverse travel or displacement in the negative direction. This is done to accurately represent the data and maintain consistency in the measurement of position and velocity.

For example, consider an object moving back and forth along a straight line. If the object starts at a position of 0 meters, moves to a position of 5 meters, and then moves back to a position of -3 meters, the displacement would be:

Δx = -3 meters

The negative value of the displacement indicates that the object has moved in the negative direction.

Similarly, if an object is moving in the negative direction with a negative velocity, the velocity would be represented as a negative value. This allows for a more accurate representation of the object’s motion and facilitates the calculation of other quantities, such as acceleration.

Interpreting Negative Values in Physics

When encountering negative values in physics, it is important to understand the context and meaning of these values. Negative values do not necessarily indicate an error or a problem with the measurement; rather, they represent the direction of motion or displacement.

It is crucial to interpret negative values correctly to ensure meaningful analysis and interpretation of the data. For example, a negative displacement may indicate that an object has moved in the opposite direction, while a negative velocity may indicate that the object is moving in the opposite direction.

Examples and Numerical Problems

  1. Example 1: An object starts at a position of 2 meters and moves to a position of -4 meters. Calculate the distance and displacement.

  2. Distance: d = |x2 - x1| = |-4 - 2| = 6 meters

  3. Displacement: Δx = x2 - x1 = -4 - 2 = -6 meters

  4. Example 2: An object starts at a position of 0 meters, moves to a position of 10 meters, and then moves back to a position of -5 meters. Calculate the total distance and displacement.

  5. Total distance: d = |x2 - x1| + |x3 - x2| = |10 - 0| + |-5 - 10| = 10 + 15 = 25 meters

  6. Displacement: Δx = x3 - x1 = -5 - 0 = -5 meters

  7. Numerical Problem 1: An object starts at a position of 3 meters, moves to a position of -7 meters, and then moves to a position of 5 meters. Calculate the total distance and displacement.

  8. Total distance: d = |x2 - x1| + |x3 - x2| = |-7 - 3| + |5 - (-7)| = 10 + 12 = 22 meters

  9. Displacement: Δx = x3 - x1 = 5 - 3 = 2 meters

  10. Numerical Problem 2: An object starts at a position of -2 meters, moves to a position of 8 meters, and then moves to a position of -4 meters. Calculate the total distance and displacement.

  11. Total distance: d = |x2 - x1| + |x3 - x2| = |8 - (-2)| + |-4 - 8| = 10 + 12 = 22 meters

  12. Displacement: Δx = x3 - x1 = -4 - (-2) = -2 meters

These examples and numerical problems demonstrate how distance is always positive, while displacement and other vector quantities can have negative values to represent reverse travel or displacement in the negative direction.

Conclusion

In summary, while distance is a scalar quantity that is always positive, there are situations in physics where negative values may be used to represent reverse travel or displacement in the negative direction. Understanding the context and meaning of these negative values is crucial for accurate analysis and interpretation of data in physics experiments and calculations.

References:

  1. CrossFit’s Measure of Intensity is Power Kinematics
  2. University Physics – Lumen Learning
  3. Very basic question regarding distance : r/AskPhysics – Reddit
  4. Make Measurable: What Galileo Didn’t Say about the Subjectivity of Algorithms
  5. Positive vs. Normative Economics: What’s the Difference?

Magnetic Field and Distance: A Comprehensive Guide for Physics Students

by
magnetic field and distance

Magnetic field and distance are closely related, with the strength of a magnetic field decreasing as the distance from its source increases. This relationship can be quantified through various experiments and calculations, providing a deeper understanding of magnetism and its properties.

Understanding the Inverse Square Law of Magnetic Fields

The relationship between magnetic field strength and distance is governed by the inverse square law, which states that the magnetic field strength is inversely proportional to the square of the distance from the source. This can be expressed mathematically as:

B = k / r^2

Where:
– B is the magnetic field strength
– k is a constant that depends on the source of the magnetic field
– r is the distance from the source of the magnetic field

This means that as the distance from the source of the magnetic field increases, the field strength decreases exponentially. For example, if you double the distance from the source, the magnetic field strength will decrease by a factor of four.

Measuring Magnetic Field Strength with a Gaussmeter

magnetic field and distance

One way to measure the magnetic field strength and its relationship with distance is by building a gaussmeter. This involves creating a circuit that can measure the strength of a magnetic field and observing how the field strength changes with distance.

The basic steps to build a gaussmeter are:

  1. Obtain a Hall effect sensor, which is a device that can measure the strength of a magnetic field.
  2. Create a circuit that can convert the voltage output of the Hall effect sensor into a reading of the magnetic field strength.
  3. Calibrate the gaussmeter by placing it in a known magnetic field and adjusting the circuit to display the correct field strength.
  4. Take voltage readings at different distances from a magnet and use the sensor’s sensitivity and Equation 1 to convert these readings to magnetic field strength.
  5. Plot the magnetic field strength against distance to visualize how the field strength changes with distance.

By following this process, you can create a custom gaussmeter and use it to explore the relationship between magnetic field strength and distance.

Measuring Relative Magnetic Field Strength with a Compass

Another way to measure the relationship between magnetic field strength and distance is by using a compass and a permanent magnet. This method involves:

  1. Placing the permanent magnet at selected distances from the compass.
  2. Measuring the deflection of the compass needle at each distance.
  3. Calculating the relative magnetic field strength using the formula:

Relative Magnetic Field Strength = tan(θ)

Where θ is the angle of deflection of the compass needle.

  1. Plotting the relative magnetic field strength against distance to create a mathematical model of the relationship.

This activity helps students think more deeply about magnetism and the modeling of fields while practicing data collection and analysis.

Investigating the Force Needed to Separate Magnets

Another experiment that can provide insights into the relationship between magnetic field strength and distance is investigating the force needed to separate magnets. This involves:

  1. Placing two magnets in contact with each other.
  2. Measuring the force required to pull the magnets apart at different distances.
  3. Plotting the force against the distance between the magnets.

This can provide a more hands-on approach to understanding the relationship between magnetic field strength and distance, as the force required to separate the magnets is directly related to the magnetic field strength.

Analyzing Precompiled Data on Magnetic Field Strength and Distance

If you don’t have the resources to conduct your own experiments, you can also use precompiled data sets to explore the relationship between magnetic field strength and distance. One such data set is available from Education.ti.com.

This data set includes measurements of magnetic field strength at various distances from a magnet. You can use this data to:

  1. Predict the functional form of the magnetic field strength vs. distance relationship.
  2. Carry out a power regression to determine the relationship’s form.
  3. Use the resulting equation to calculate the magnetic field strength at any distance from the magnet.

By analyzing this precompiled data, you can gain a deeper understanding of the mathematical relationship between magnetic field strength and distance.

Additional Resources and Experiments

Here are some additional resources and experiments that can help you further explore the relationship between magnetic field strength and distance:

  1. Measuring Magnetic Fields with a Smartphone: This video demonstrates how to use a smartphone’s magnetometer to measure magnetic field strength and observe its relationship with distance.
  2. Magnetic Field Mapping: This Science Buddies project guide explains how to create a magnetic field map by measuring the field strength at various points around a magnet.
  3. Magnetic Field Visualization: This activity from TeachEngineering allows students to visualize the magnetic field around a magnet using iron filings or a compass.
  4. Magnetic Force and Distance Experiment: This Physics Forums thread discusses an experiment that investigates the relationship between the force needed to separate magnets and the distance between them.

By exploring these resources and conducting your own experiments, you can gain a deeper understanding of the fascinating relationship between magnetic field strength and distance.

Reference:

  1. https://www.sciencebuddies.org/science-fair-projects/project-ideas/Elec_p030/electricity-electronics/measure-magnetic-fields
  2. https://www.teachengineering.org/activities/view/rice_magnetic_activity1
  3. https://www.physicsforums.com/threads/magnet-experiment-investigating-force-distance.880419/
  4. https://education.ti.com/~/media/BAA91BA517884F8EB03FC0744005BA74
  5. https://www.youtube.com/watch?v=6ZQm081iUBw

7 Interesting System In Equilibrium Examples (Read First)

by

If a system is not continuing its motion state or if its internal energy is not showing any change with respect to time then that system is said to be in an equilibrium. We can represent the walking of a person on a treadmill as an example here. Whenever a person is moving forward on a treadmill the treadmill will move in the opposite direction i.e,the backward direction to balance the person’s motion. It means that the person is in equilibrium with respect to the treadmill.

Now we will discuss 7+ interesting system in equilibrium examples.

1. A book on a table

If we keep a book on a table then that book will be in equilibrium. Why? Because the sum of the net forces that are acting on that book remains zero and the sum of total torques that are acting on that book is also zero. As these two main conditions are satisfied here that is why that book is in equilibrium. In this case the linear acceleration and the angular acceleration both are also zero.

                                                             ∑F=0,∑τ=0

2.A car that is moving with a constant velocity

A truck that is moving with a constant velocity is another important and common system in equilibrium examples. Now the question arises how a moving body can be in equilibrium? The answer is : this type of equilibrium is dynamic equilibrium. It means that in this case also the sum of total forces is zero and the sum of total torque is also zero. ∑F=0,∑τ=0.

In this case the linear acceleration and the angular acceleration are also zero. Hence the net effects of forces and torques are zero. That is why the truck which was moving with a constant velocity keeps moving with the same velocity and there is no change in its velocity. So there is no linear as well as angular acceleration.

3. A man who is standing on a horizontal plane

A man who is standing on a horizontal plane is an example of a system in equilibrium. We all know that when a man is standing upon a horizontal plane then his weight is acting in the downward direction. The weight of the man can be defined as the force acting downwards due to the effect of gravity. This weight or gravitational pull is balanced by the reaction force which is acting in the opposite direction of the gravitational pull.

In this way two forces,weight acting downwards and reaction force acting upwards are balanced by each other. Hence the main conditions of a system in equilibrium are satisfied. The conditions are ∑F=0,∑τ=0. This is the reason why a man on a horizontal plane remains in equilibrium.

4. Dynamic equilibrium and its examples

Let us take a body which is moving at a constant velocity. If the body keeps moving without any kind of external disturbance then that body will be said to be in equilibrium. Why? Because we know that if there is no effect of any external force on a body it will keep moving at the same velocity as earlier. This law is known as Newton’s second law of motion.

So dynamic equilibrium is related to Newton’s second law of motion. Now what are examples of dynamic equilibrium that are observed everyday in our daily life? The examples are a car moving at a constant velocity and a bucket of water that is being raised at a constant velocity by a pulley rope system.

In both of these examples that are mentioned above we have seen that the sum of the net forces on that car as well as the bucket of water is zero,hence both the car and the bucket are moving at a constant velocity. Hence dynamic equilibrium is maintained here.

5.Chemical equilibrium and its examples

There are so many questions related to chemical equilibrium like how it can be achieved,when it can be achieved etc. First of all the reaction should be a reversible reaction. If in a reversible chemical reaction the rate of forward reaction becomes equal to the rate of reverse reaction then a system achieves chemical equilibrium.

Now we have to know what is forward reaction and what is reverse reaction. Basically when products are produced from the reactants in a chemical reaction then this reaction is known as forward reaction. When reactants are formed from products in a reversible chemical reaction then that reaction is known as reverse reaction.         FORWARD REACTION: REACTANTS → PRODUCTS

                                            REVERSE REACTION: PRODUCTS → REACTANTS

To describe chemical equilibrium we will take the help of an example of a bottle of cold drink. In this bottle CO₂ gas is dissolved in the liquid and also it is in gaseous form in the gap between the cap and the surface of the liquid inside the bottle. This gas continuously changes into liquid as well as the liquified gas also turns into gas in the bottle. That means the system is in chemical equilibrium.

CO₂↑ + H₂O⇋H₂CO₃

Another common example is haemoglobin that transports oxygen within our bodies.

6. Thermodynamic equilibrium and its examples

If I connect two containers so that heat can flow from the hot container to the cold one. If there is no flow of heat between two containers,it means that both of them are in thermodynamic equilibrium with each other.

Through a simple example we can describe thermodynamic equilibrium. Say there is a bottle in which a gas is kept and there is a movable piston in it. If the temperature and pressure that are maintained within the container are kept uniform then this container will be in thermodynamic equilibrium.

Another condition is there which must be followed. The external forces acting upon the movable piston should not be able to move it. In this case ΔT=0,ΔP=0. Here ΔT is the change in temperature and ΔP is the change in pressure.

7. Static equilibrium and its examples

A book that is kept on a table,a rigid body kept on a horizontal surface all these are the examples of static equilibrium. in these cases the sum of total force is zero and the sum of total torque is zero.

Some additional points related to the system in equilibrium

In the case of a body which is in equilibrium there will be no existence of linear acceleration and angular acceleration. If there is no effect of any external force present in that system then its state of equilibrium will be continued indefinitely. This will be perturbed only if there is an effect of any external force acting upon the system.

If we take an example of a rigid body then for that body if the sum of all the torques that are acting on it is zero,then only the body is said to be in equilibrium. ∑F=0,

∑τ=0.This happens because in this way the rotational motion remains unchanged. If there is any displacement taking place in a body then if there is any action of a force that can oppose this effect and again turn the body to equilibrium then this type of equilibrium is known as stable equilibrium.

Conclusion

In this article we have discussed different system in equilibrium examples. We have also discussed different types of equilibrium that are dynamic equilibrium,chemicalequilibrium,thermodynamic equilibrium in a brief manner equipped with some of their common examples.

 If a person wants to gain some knowledge regarding a  system in equilibrium he or she can go through this article to have a clear picture of that system in equilibrium.

Also Read:

Magnetic Flux in a Coil: A Comprehensive Guide

by
magnetic flux in a coil

Magnetic flux in a coil is the measure of the total magnetic field passing through the area enclosed by the coil. It is a crucial concept in understanding electromagnetic induction and has numerous applications in various fields of physics and engineering. This comprehensive guide will delve into the intricacies of magnetic flux in a coil, providing you with a thorough understanding of the underlying principles, formulas, and practical applications.

Understanding Magnetic Flux

Magnetic flux, denoted by the symbol Φ, is the measure of the total magnetic field passing through a given area. It is calculated using the formula:

Φ = BAcosθ

Where:
– Φ is the magnetic flux (in webers, Wb)
– B is the magnetic field strength (in teslas, T)
– A is the area of the coil (in square meters, m²)
– θ is the angle between the magnetic field and the normal to the coil’s surface (in radians)

The magnetic flux is a vector quantity, meaning it has both magnitude and direction. The direction of the magnetic flux is determined by the direction of the magnetic field lines passing through the coil.

Magnetic Flux in a Coil

magnetic flux in a coil

When a coil is placed in a magnetic field, the magnetic flux through the coil is the sum of the magnetic flux through each individual turn of the coil. The total magnetic flux through the coil is given by:

Φ = NBAcosθ

Where:
– N is the number of turns in the coil

This formula is particularly useful when analyzing the behavior of a coil in a changing magnetic field, as it allows us to calculate the induced electromotive force (EMF) in the coil.

Faraday’s Law of Electromagnetic Induction

Faraday’s law of electromagnetic induction states that the induced EMF in a coil is proportional to the rate of change of the magnetic flux through the coil. The formula for the induced EMF is:

ε = -N(ΔΦ/Δt)

Where:
– ε is the induced EMF (in volts, V)
– N is the number of turns in the coil
– ΔΦ is the change in magnetic flux (in webers, Wb)
– Δt is the time over which the change in flux occurs (in seconds, s)

The negative sign in the formula indicates that the induced EMF opposes the change in magnetic flux, as per Lenz’s law.

Practical Examples

  1. Permanent Magnet Moving Through a Coil
    Consider a small 10 mm diameter permanent magnet producing a field of 100 mT, moving at a speed of 1 m/s through a 100-turn coil of length 1 mm and diameter just larger than the magnet.

The magnetic flux through the coil can be calculated as:
Φ = NBAcosθ = 100 × 0.1 T × π × (0.005 m)² × 1 = 7.854 × 10^-6 Wb

The induced EMF can be calculated using Faraday’s law:
ε = -N(ΔΦ/Δt) = -100 × (7.854 × 10^-6 Wb) / (0.001 s) = -0.785 V

  1. Solenoid with a Varying Current
    Consider a solenoid with 500 turns and a cross-sectional area of 0.01 m². The solenoid is placed in a uniform magnetic field of 0.5 T, and the current through the solenoid is varied linearly from 0 A to 10 A over a period of 1 second.

The magnetic flux through the solenoid can be calculated as:
Φ = NBAcosθ = 500 × 0.5 T × 0.01 m² × 1 = 2.5 Wb

The change in magnetic flux over the 1-second period is:
ΔΦ = 2.5 Wb (at 10 A) – 0 Wb (at 0 A) = 2.5 Wb

The induced EMF can be calculated using Faraday’s law:
ε = -N(ΔΦ/Δt) = -500 × (2.5 Wb) / (1 s) = -1250 V

These examples demonstrate how the formulas for magnetic flux and induced EMF can be applied to practical situations involving coils and changing magnetic fields.

Factors Affecting Magnetic Flux in a Coil

Several factors can influence the magnetic flux in a coil, including:

  1. Magnetic Field Strength (B): The stronger the magnetic field, the greater the magnetic flux through the coil.
  2. Coil Area (A): The larger the area of the coil, the greater the magnetic flux through the coil.
  3. Angle between Magnetic Field and Coil (θ): The magnetic flux is maximized when the magnetic field is perpendicular to the coil (θ = 0°) and minimized when the magnetic field is parallel to the coil (θ = 90°).
  4. Number of Turns in the Coil (N): Increasing the number of turns in the coil increases the total magnetic flux through the coil.

Understanding these factors is crucial in designing and optimizing coils for various applications, such as transformers, generators, and electromagnetic devices.

Magnetic Flux Density and Magnetic Flux Linkage

In addition to magnetic flux, two other related concepts are important in the study of coils:

  1. Magnetic Flux Density (B): Magnetic flux density, also known as magnetic induction, is the magnetic flux per unit area. It is measured in teslas (T) and is given by the formula:
    B = Φ/A

  2. Magnetic Flux Linkage (Ψ): Magnetic flux linkage is the total magnetic flux linked with a coil or a circuit. It is the product of the number of turns in the coil and the magnetic flux through the coil:
    Ψ = NΦ

These concepts are essential in understanding the behavior of coils in electromagnetic systems and in the analysis of transformers, motors, and generators.

Applications of Magnetic Flux in Coils

Magnetic flux in coils has numerous applications in various fields, including:

  1. Transformers: Transformers rely on the principle of electromagnetic induction, where the changing magnetic flux in the primary coil induces an EMF in the secondary coil, allowing for the transformation of voltage and current levels.
  2. Generators and Motors: Generators and motors convert between mechanical and electrical energy by utilizing the principle of electromagnetic induction and the changing magnetic flux in coils.
  3. Eddy-Current Brakes: Eddy-current brakes use the changing magnetic flux in a conductive material to generate opposing currents, which in turn create a braking force.
  4. Magnetic Resonance Imaging (MRI): MRI machines use strong, uniform magnetic fields and rapidly changing magnetic fields to generate images of the human body.
  5. Induction Heating: Induction heating uses the principle of electromagnetic induction to heat conductive materials, such as metals, by inducing eddy currents within them.

These are just a few examples of the many applications of magnetic flux in coils, highlighting the importance of this concept in various fields of science and engineering.

Conclusion

Magnetic flux in a coil is a fundamental concept in electromagnetism, with far-reaching applications in various fields. By understanding the formulas, factors, and practical examples related to magnetic flux, you can gain a deeper appreciation for the underlying principles and their real-world implications. This comprehensive guide has provided you with the necessary knowledge and tools to explore the fascinating world of magnetic flux in coils and its numerous applications.

References

  1. Khan Academy. (n.d.). What is Faraday’s law? Retrieved from https://www.khanacademy.org/science/physics/magnetic-forces-and-magnetic-fields/magnetic-flux-faradays-law/a/what-is-faradays-law
  2. Search Coil Experiment (measure magnetic field strength) – YouTube. (2020, January 21). Retrieved from https://www.youtube.com/watch?v=vb8KwB0ANrg
  3. WebAssign. (n.d.). Faraday’s Law. Retrieved from https://www.webassign.net/question_assets/ketphysvl1/lab_14/manual.html
  4. Nave, R. (n.d.). Magnetic Flux. Retrieved from http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/magflux.html
  5. Griffiths, D. J. (2013). Introduction to Electrodynamics (4th ed.). Pearson.
  6. Halliday, D., Resnick, R., & Walker, J. (2013). Fundamentals of Physics (10th ed.). Wiley.