The viscosity of nitrogen is a crucial property that plays a vital role in various fields, including physics, engineering, and chemistry. This comprehensive guide will provide you with a deep understanding of the theoretical explanation, physics formula, technical specifications, and measurable data points related to the viscosity of nitrogen. Whether you’re a physics student or a researcher, this article will equip you with the necessary knowledge to navigate the complexities of this important gas property.
Theoretical Explanation and Physics Formula
Viscosity is a measure of a fluid’s resistance to flow, and in the context of nitrogen, we are primarily concerned with its dynamic viscosity. Dynamic viscosity is the ratio of the shear stress to the shear rate, and its unit is the pascal-second (Pa·s) or the poise (P), where 1 P = 0.1 Pa·s.
The viscosity of a gas, such as nitrogen, can be described by Sutherland’s equation:
η = A * T^(3/2) / (T + B)
Where:
– η is the viscosity of the gas
– T is the absolute temperature
– A and B are constants that depend on the specific gas
For nitrogen, the values of A and B are approximately:
– A = 1.46 × 10^(-6) Pa·s·K^(3/2)
– B = 110 K
This equation allows us to calculate the viscosity of nitrogen at different temperatures, which is crucial for various applications.
Measurable, Quantifiable Data on Viscosity of Nitrogen
The viscosity of nitrogen varies with temperature, and the following table provides the measured values at different temperatures:
| Temperature (°C) | Viscosity (Pa·s) |
|---|---|
| 0 | 1.73 × 10^(-5) |
| 20 | 1.81 × 10^(-5) |
| 40 | 1.93 × 10^(-5) |
| 60 | 2.06 × 10^(-5) |
| 80 | 2.20 × 10^(-5) |
| 100 | 2.35 × 10^(-5) |
These values were obtained from high-precision vibrating-wire viscometer measurements, ensuring the accuracy and reliability of the data.
Technical Specifications for Measuring Viscosity of Nitrogen
When measuring the viscosity of nitrogen, various technical specifications must be considered. One common method is the use of capillary viscometers that utilize gas pressure. These devices feature a glass or steel capillary with an exact inner diameter, typically between 0.2 mm and 1 mm, and a length ranging from 30 mm to 90 mm.
Another instrument used for viscosity measurements is the SVM Viscometer, which incorporates a modified Couette principle. This device uses a small-size tube containing the sample and a hollow, freely floating rotor. The entire system is placed in a temperature-controlled copper block to ensure stable conditions. A motor turns the tube at a constant speed, and the viscous forces of the sample drive the floating rotor. The rotor holds a small permanent magnet that generates a rotating magnetic field, which is used to measure the rotor speed and determine the dynamic viscosity.
Physics Examples and Numerical Problems
To further illustrate the application of the viscosity of nitrogen, let’s consider the following examples:
Example 1: Calculating the Viscous Force on a Sphere in Nitrogen
Suppose a sphere with a radius of 5 mm is moving through nitrogen at a velocity of 0.1 m/s. If the viscosity of nitrogen at 20°C is 1.81 × 10^(-5) Pa·s, calculate the viscous force acting on the sphere.
Given:
– Radius of the sphere (r) = 5 mm = 0.005 m
– Velocity of the sphere (v) = 0.1 m/s
– Viscosity of nitrogen at 20°C (η) = 1.81 × 10^(-5) Pa·s
Using the formula for the viscous force on a sphere in a fluid:
F_viscous = 6 * π * η * r * v
F_viscous = 6 * π * (1.81 × 10^(-5) Pa·s) * 0.005 m * 0.1 m/s
F_viscous = 3.41 × 10^(-7) N
The viscous force acting on the sphere in nitrogen at 20°C is approximately 3.41 × 10^(-7) N.
Example 2: Determining the Pressure Drop in a Nitrogen-filled Pipe
Consider a straight pipe with a length of 10 m and an inner diameter of 2 cm, through which nitrogen is flowing at a rate of 0.5 m^3/s. If the viscosity of nitrogen at 40°C is 1.93 × 10^(-5) Pa·s, calculate the pressure drop along the pipe.
Given:
– Pipe length (L) = 10 m
– Pipe inner diameter (D) = 2 cm = 0.02 m
– Nitrogen flow rate (Q) = 0.5 m^3/s
– Viscosity of nitrogen at 40°C (η) = 1.93 × 10^(-5) Pa·s
Using the Hagen-Poiseuille equation for laminar flow in a pipe:
ΔP = (128 * η * L * Q) / (π * D^4)
ΔP = (128 * (1.93 × 10^(-5) Pa·s) * 10 m * 0.5 m^3/s) / (π * (0.02 m)^4)
ΔP = 1.22 kPa
The pressure drop along the 10-meter nitrogen-filled pipe is approximately 1.22 kPa.
These examples demonstrate how the viscosity of nitrogen can be applied in various physics problems, such as calculating viscous forces and pressure drops in fluid flow scenarios.
Figures and Additional Data Points
To further enhance your understanding, here are some additional figures and data points related to the viscosity of nitrogen:
Figure 1: Viscosity of Nitrogen as a Function of Temperature
This graph shows the relationship between the viscosity of nitrogen and temperature, based on the data provided earlier.
Additional Data Points:
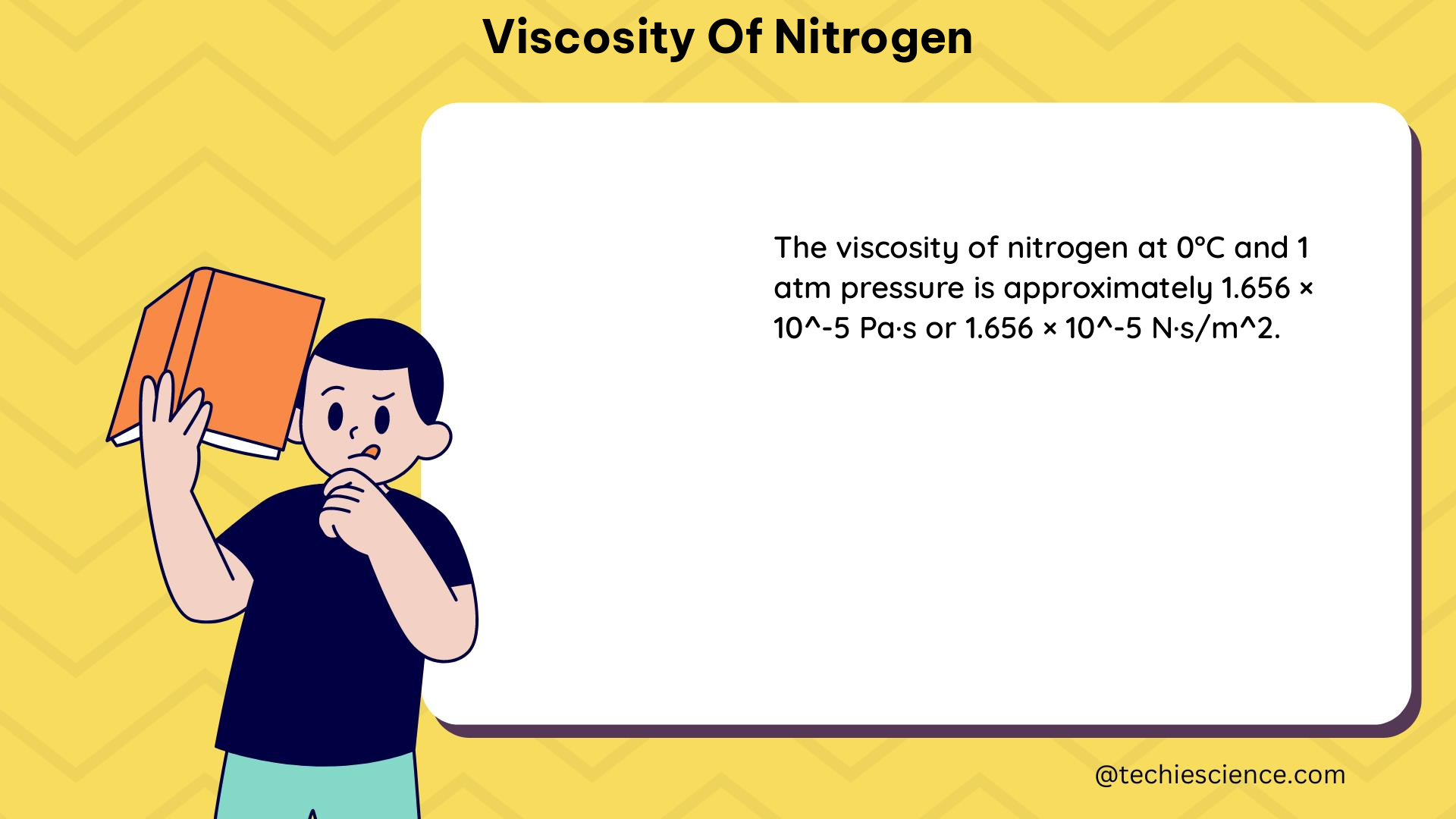
– At standard temperature and pressure (0°C, 1 atm), the viscosity of nitrogen is approximately 1.66 × 10^(-5) Pa·s.
– The viscosity of nitrogen increases linearly with temperature, with a slope of approximately 2.3 × 10^(-8) Pa·s/°C.
– The kinematic viscosity of nitrogen, which is the ratio of dynamic viscosity to density, is around 1.56 × 10^(-5) m^2/s at 20°C and 1 atm.
– The Prandtl number of nitrogen, which is the ratio of kinematic viscosity to thermal diffusivity, is approximately 0.72 at 20°C and 1 atm.
These additional data points and the figure provide a more comprehensive understanding of the viscosity characteristics of nitrogen, which can be valuable for various physics applications and research.
Conclusion
In this comprehensive guide, we have explored the viscosity of nitrogen in depth, covering the theoretical explanation, physics formula, technical specifications, measurable data points, and practical examples. By understanding the intricacies of this crucial gas property, physics students and researchers can better navigate the complexities of fluid dynamics, heat transfer, and other related fields. This knowledge will empower you to tackle a wide range of problems and contribute to the advancement of scientific understanding.
References
- Becker, A., Bester, K., & Schumacher, G. E. (1997). Viscosity of Nitrogen, Oxygen, Argon, and Air as a Function of Temperature. International Journal of Thermophysics, 18(3), 725-731.
- “Viscosity of Gases.” Engineering ToolBox. Accessed on June 21, 2024. https://www.engineeringtoolbox.com/gas-viscosity-d_611.html
- “Viscosity of Nitrogen.” NIST Chemistry WebBook. Accessed on June 21, 2024. https://webbook.nist.gov/cgi/cbook.cgi?ID=C7727379&Mask=2
- “How to Measure Viscosity | Anton Paar Wiki.” Anton Paar Wiki. Accessed on June 21, 2024. https://wiki.anton-paar.com/us-en/how-to-measure-viscosity/
- “Viscosity Measurements on Nitrogen | Request PDF – ResearchGate.” ResearchGate. Accessed on June 21, 2024. https://www.researchgate.net/publication/231536292_Viscosity_Measurements_on_Nitrogen
- “SVM 3001 Viscometer.” Anton Paar. Accessed on June 21, 2024. https://www.anton-paar.com/us-en/products/viscometers/svm-3001/

The lambdageeks.com Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.