The Viscosity of Hydrogen: A Comprehensive Guide

The viscosity of hydrogen is a critical property that has been extensively studied and measured under various conditions. This comprehensive guide delves into the intricate details of hydrogen viscosity, providing a wealth of technical information and quantifiable data to aid physics students and researchers in their understanding of this fundamental fluid property.

Viscosity at Different Temperatures and Pressures

Low Temperatures

• At 15 K, the viscosity of para-hydrogen is approximately 6.45 micropascal seconds (μPa·s), while for normal hydrogen, it is around 6.55 μPa·s.
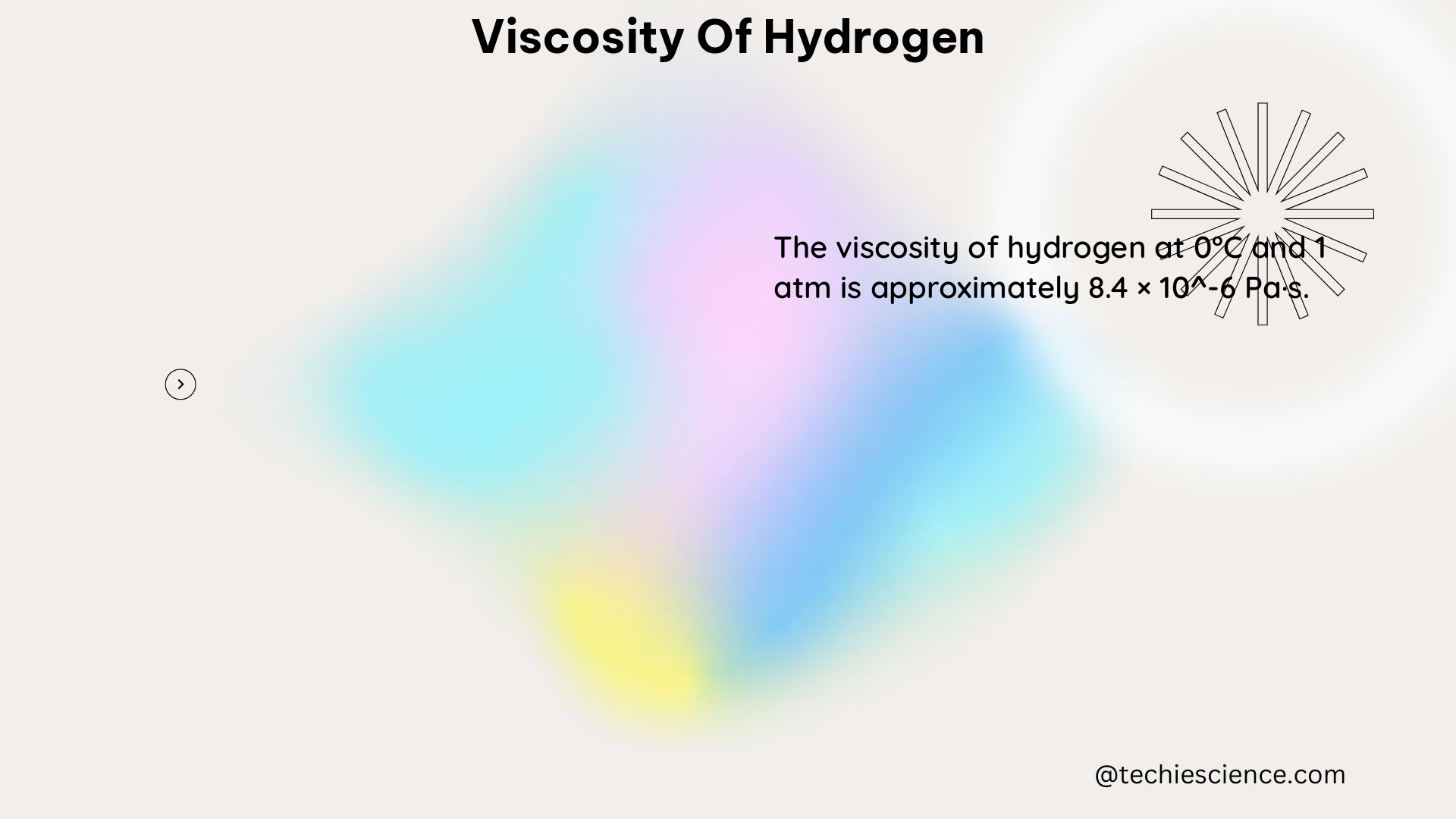
• At 300 K, the viscosity of hydrogen is about 8.37 μPa·s.

High Temperatures

• At 1000 K, the viscosity of hydrogen is around 17.45 μPa·s.
• At 5000 K, the viscosity of hydrogen is approximately 34.15 μPa·s.

High Pressure

• At 100 bar and 300 K, the viscosity of hydrogen is about 10.35 μPa·s.
• At 1000 bar and 300 K, the viscosity of hydrogen is around 24.15 μPa·s.

Viscosity of Hydrogen Mixtures

Hydrogen-Oxygen Mixtures

The viscosity of a hydrogen-oxygen mixture can be calculated using the equation of Herning and Zipperer, which involves the partial viscosities of each component weighted by the square root of their molecular weights:

μ_mixture = Σ(x_i * μ_i * √(M_i)) / Σ(x_i * √(M_i))

Where:
– μ_mixture is the viscosity of the mixture
– x_i is the mole fraction of component i
– μ_i is the viscosity of component i
– M_i is the molecular weight of component i

Hydrogen-Argon Mixtures

The viscosity of a hydrogen-argon mixture can be calculated using Brokaw’s method, which requires effective collision diameters and dipole moments for the components:

μ_mixture = Σ(x_i * μ_i * Φ_ij) / Σ(x_i * Φ_ij)

Where:
– Φ_ij is the interaction parameter between components i and j, which depends on the effective collision diameters and dipole moments.

Viscosity of Hydrogen Peroxide Vapor

At 170°C, the viscosity of hydrogen peroxide vapor is estimated to be around 150 micropoises (μP).

Viscosity of Hydrogen at High Pressure and High Temperature

Various correlation methods have been developed to estimate the viscosity of hydrogen at high pressure and high temperature, including the use of intermolecular potential functions and kinetic theory. These methods often involve complex equations and the evaluation of various parameters, such as effective collision diameters and dipole moments.

Viscosity of Hydrogen in Different States

Dilute Gaseous Hydrogen

The viscosity of dilute gaseous hydrogen has been critically evaluated and correlated using kinetic theory, with numerical results presented from 15 to 5000 K. The viscosity of dilute gaseous hydrogen can be calculated using the Chapman and Cowling equation, which involves a complicated form with an eight-term polynomial divided by a six-term polynomial, requiring the evaluation of integrals involving the force law for molecular interactions.

Liquid Hydrogen

The viscosity of liquid hydrogen can be obtained by analyzing the density dependence of viscosity in the dense gas and liquid state. This approach often involves the use of empirical correlations or theoretical models to estimate the viscosity of liquid hydrogen under various conditions.

Theoretical Models and Equations

Chapman and Cowling Equation

The Chapman and Cowling equation for the viscosity of a gas is given by:

μ = (5/16) * (√(π*m*k*T) / (π*σ^2)) * Ω^(2,2)

Where:
– m is the molecular mass
– k is the Boltzmann constant
– T is the absolute temperature
– σ is the collision diameter
– Ω^(2,2) is the collision integral, which requires the evaluation of integrals involving the force law for molecular interactions.

Brokaw’s Equation

Brokaw’s equation for the viscosity of a gas mixture is given by:

μ_mixture = Σ(x_i * μ_i * Φ_ij) / Σ(x_i * Φ_ij)

Where:
– Φ_ij is the interaction parameter between components i and j, which depends on the effective collision diameters and dipole moments.

This equation has been reported to have an average deviation of 0.7% and a maximum deviation of 3.7% for 25 gas pairs and 280 mixtures.

Herning and Zipperer Equation

The Herning and Zipperer equation for the viscosity of a gas mixture is given by:

μ_mixture = Σ(x_i * μ_i * √(M_i)) / Σ(x_i * √(M_i))

Where:
– x_i is the mole fraction of component i
– μ_i is the viscosity of component i
– M_i is the molecular weight of component i

This equation calculates partial viscosities without the evaluation of Wilke’s coefficients and has been reported to have an average deviation of 1.5% and a maximum deviation of 5% for hydrocarbon mixtures.

Experimental Methods

Measurement Techniques

Various experimental techniques have been used to measure the viscosity of hydrogen, including the use of capillary flow and oscillating disk methods. These techniques often involve the measurement of the time required for a fixed volume of gas to flow through a capillary or the damping of an oscillating disk in the presence of the gas.

Data Analysis

Experimental data have been analyzed using quantum mechanical 12–6 functions to obtain reliable viscosity values. This approach involves the use of advanced computational methods and the incorporation of intermolecular potential functions to accurately model the behavior of hydrogen under various conditions.

References

1. A Simple and Accurate Method for Calculating Viscosity of Gaseous Mixtures: Link
2. The Viscosity of Vapor Mixtures of Hydrogen Peroxide: Link
3. Prediction of Hydrogen Gas Viscosity at High Pressure and High Temperature: Link
4. Hydrogen Viscosity at Different Temperatures and Pressures: Link
5. The Viscosity and Thermal Conductivity of Dilute Gaseous Hydrogen: Link