Velocity vs Speed: A Comprehensive Guide for Physics Students

Summary

Velocity and speed are two fundamental concepts in physics that describe the motion of objects. While both measure the rate of change in an object’s position, they differ in their definitions and applications. This comprehensive guide will delve into the technical details of velocity and speed, providing physics students with a thorough understanding of these concepts, including their mathematical formulas, examples, and numerical problems.

Understanding Speed

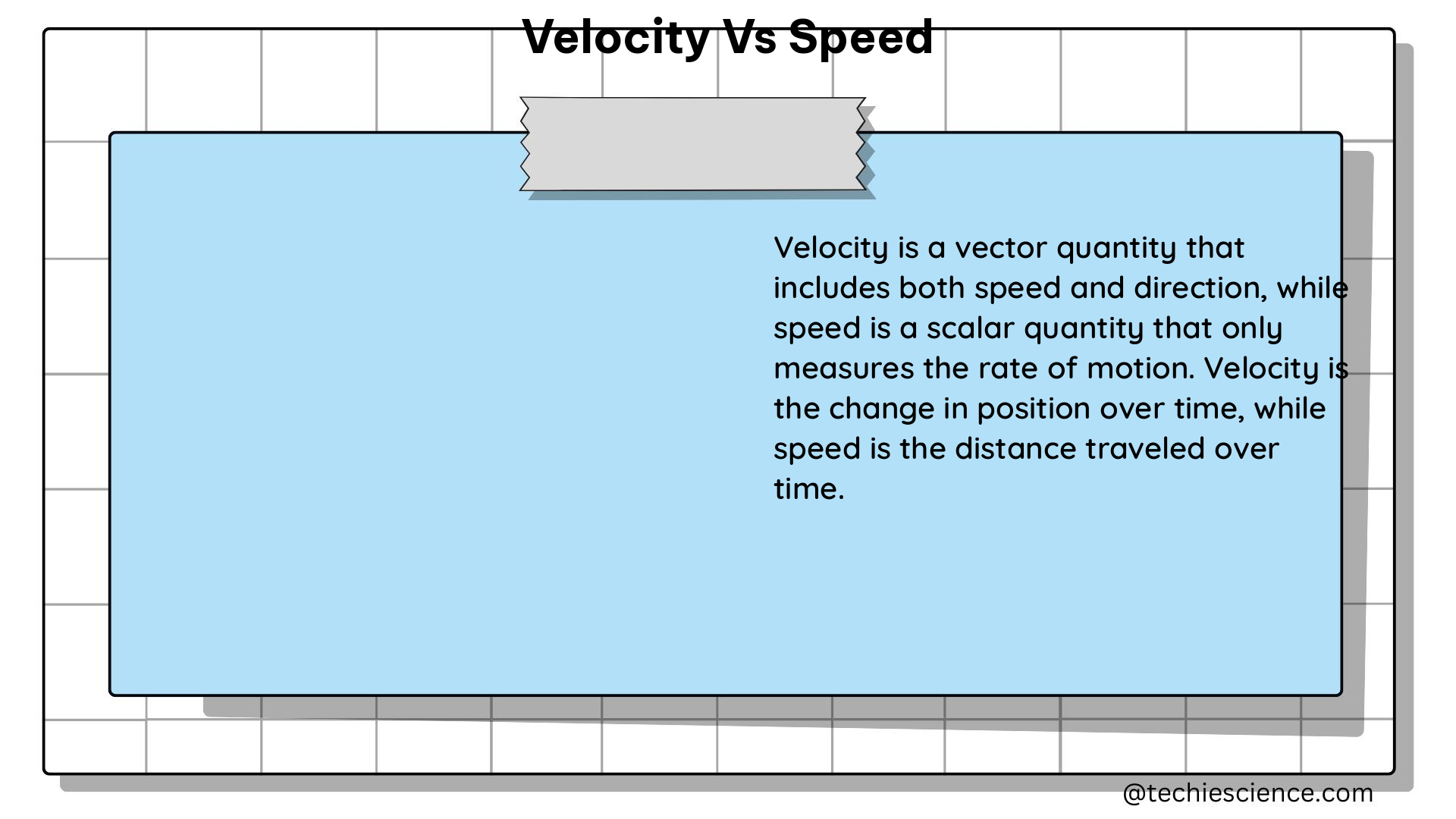
Speed is a scalar quantity that measures the rate of change in an object’s position, without considering the direction of motion. It is calculated as the total distance traveled divided by the time taken. The formula for speed is:

Speed = Distance / Time

For example, if a car travels 100 miles in 2 hours, its average speed is 50 miles per hour.

Types of Speed

There are two main types of speed:

1. Average Speed: The total distance traveled divided by the total time taken.
2. Instantaneous Speed: The speed of an object at a specific moment in time.

Average speed is calculated using the formula above, while instantaneous speed is the derivative of the object’s position with respect to time.

Numerical Examples

1. A car travels 120 miles in 3 hours. Calculate the average speed.

2. Average Speed = 120 miles / 3 hours = 40 miles per hour

3. A runner runs at a constant speed of 8 meters per second. Calculate the distance traveled in 30 seconds.

4. Distance = Speed × Time
5. Distance = 8 meters/second × 30 seconds = 240 meters

Understanding Velocity

Velocity is a vector quantity that measures the rate of change in an object’s position, considering both the magnitude (speed) and the direction of motion. It is calculated as the displacement (change in position) divided by the time taken. The formula for velocity is:

Velocity = Displacement / Time

For example, if a car travels 100 miles east in 2 hours, its average velocity is 50 miles per hour, east.

Components of Velocity

Velocity can be broken down into two components:

1. Magnitude: The speed of the object.
2. Direction: The direction of the object’s motion.

Velocity is often represented using a vector, where the length of the vector represents the magnitude (speed), and the direction of the vector represents the direction of motion.

Numerical Examples

1. A car travels 80 meters north in 4 seconds. Calculate the average velocity.
2. Displacement = 80 meters
3. Time = 4 seconds
4. Velocity = Displacement / Time

5. Velocity = 80 meters / 4 seconds = 20 meters per second, north

6. A ball is thrown at an angle of 30 degrees above the horizontal with an initial speed of 20 meters per second. Calculate the initial velocity of the ball.

7. Initial speed = 20 meters per second
8. Angle = 30 degrees above the horizontal
9. Velocity = Speed × cos(angle)
10. Velocity = 20 meters/second × cos(30 degrees) = 17.32 meters per second, 30 degrees above the horizontal

Differences between Speed and Velocity

The main differences between speed and velocity are:

Characteristic	Speed	Velocity
Definition	Scalar quantity that measures the rate of change in an object’s position	Vector quantity that measures the rate of change in an object’s position, considering both magnitude and direction
Formula	Speed = Distance / Time	Velocity = Displacement / Time
Representation	Magnitude only (e.g., 50 miles per hour)	Magnitude and direction (e.g., 50 miles per hour, east)
Calculation	Total distance traveled divided by total time taken	Displacement (change in position) divided by time taken
Applications	Useful for describing the overall motion of an object	Useful for analyzing the motion of an object in a specific direction

Practical Applications of Speed and Velocity

Speed and velocity have numerous practical applications in various fields, including:

1. Transportation: Measuring the speed and velocity of vehicles to ensure safe and efficient travel.
2. Sports: Analyzing the speed and velocity of athletes to improve their performance and technique.
3. Astronomy: Calculating the speed and velocity of celestial bodies to understand their motion and predict future positions.
4. Engineering: Designing and optimizing the performance of moving systems, such as machinery and robots, based on their speed and velocity.
5. Scrum Methodology: Measuring the velocity of a team to improve their productivity and project management.

Conclusion

In conclusion, understanding the concepts of speed and velocity is crucial for physics students to accurately describe and analyze the motion of objects. This comprehensive guide has provided detailed information on the definitions, formulas, examples, and practical applications of these fundamental concepts. By mastering the differences between speed and velocity, students will be better equipped to tackle complex physics problems and apply these principles in real-world scenarios.

References

1. The Physics Classroom. (n.d.). Speed versus Velocity. Retrieved from https://www.physicsclassroom.com/class/1DKin/Lesson-1/Speed-and-Velocity
2. Quizlet. (n.d.). Chapter 11 review Flashcards. Retrieved from https://quizlet.com/279143386/chapter-11-review-flash-cards/
3. Atlassian. (n.d.). Velocity in Scrum: How to Measure and Improve Performance. Retrieved from https://www.atlassian.com/agile/project-management/velocity-scrum
4. Khan Academy. (n.d.). Velocity and speed. Retrieved from https://www.khanacademy.org/science/physics/one-dimensional-motion/displacement-velocity-time/a/what-is-velocity
5. University of Colorado Boulder. (n.d.). Velocity vs. Speed. Retrieved from https://phet.colorado.edu/en/simulation/legacy/velocity-vs-speed