The Velocity After Collision Formula: A Comprehensive Guide for Physics Students

Summary

The velocity after collision formula is a fundamental concept in physics that allows us to calculate the final velocities of objects involved in both inelastic and elastic collisions. This formula is derived from the principle of conservation of momentum, which states that the total momentum of a closed system remains constant before and after a collision. By understanding and applying this formula, physics students can solve a wide range of problems related to collisions and their effects on the motion of objects.

Understanding Inelastic Collisions

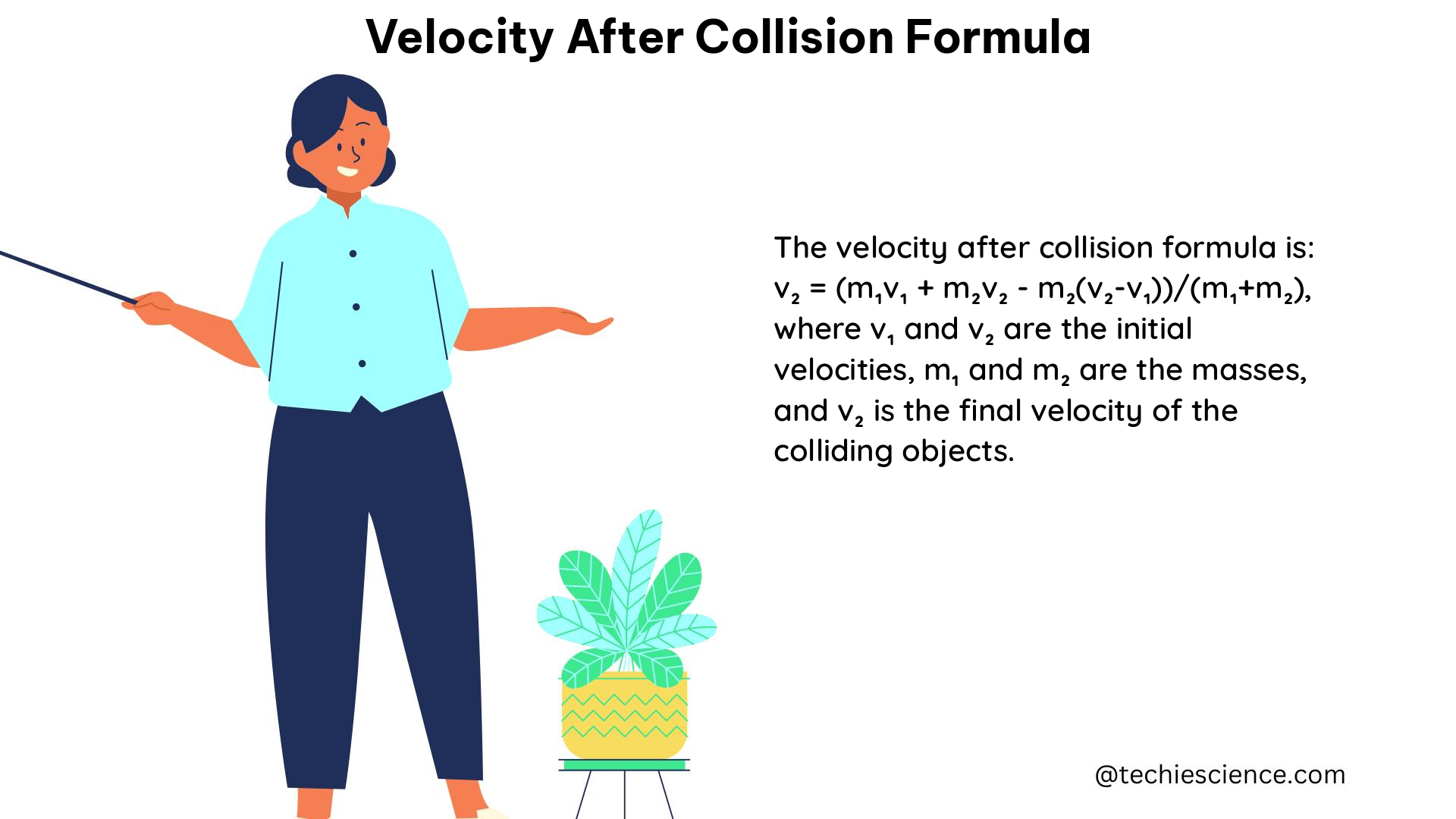
In an inelastic collision, the colliding objects stick together after the collision, and their final velocity is a weighted average of their initial velocities. The formula for the final velocity in an inelastic collision is:

v' = (m1v1 + m2v2) / (m1 + m2)

Where:
– v' is the final velocity of the objects after the collision
– m1 and m2 are the masses of the two objects
– v1 and v2 are the initial velocities of the two objects

Let’s consider an example:

Suppose two objects with masses of 0.6 kg and 0.8 kg are moving with initial velocities of 4 m/s and 2 m/s, respectively, and they undergo an inelastic collision. To find the final velocity, we can plug the values into the formula:

v' = (0.6 kg × 4 m/s + 0.8 kg × 2 m/s) / (0.6 kg + 0.8 kg) = 3.2 m/s

This means that after the collision, both objects will be moving with a common velocity of 3.2 m/s in the same direction.

Understanding Elastic Collisions

In an elastic collision, the colliding objects do not stick together, and their final velocities depend on the angle of collision. The formula for the final velocities in an elastic collision is more complex and involves the principle of conservation of momentum and the coefficient of restitution.

The principle of conservation of momentum states that the total momentum of the system before the collision is equal to the total momentum of the system after the collision. This can be expressed mathematically as:

m1v1 + m2v2 = m1v'1 + m2v'2

Where:
– m1 and m2 are the masses of the two objects
– v1 and v2 are the initial velocities of the two objects
– v'1 and v'2 are the final velocities of the two objects

The coefficient of restitution, denoted as e, is a measure of the elasticity of the collision. It ranges from 0 (completely inelastic collision) to 1 (perfectly elastic collision). The coefficient of restitution can be used to relate the final velocities to the initial velocities and the angle of collision.

Let’s consider an example:

Suppose two objects with masses of 0.25 kg and 0.4 kg are moving with initial velocities of 2 m/s and 0 m/s, respectively, and they undergo an elastic collision. To find the final velocities, we can use the principle of conservation of momentum:

0.25 kg × 2 m/s + 0.4 kg × 0 m/s = 0.25 kg × v'1 + 0.4 kg × v'2
0.5 kg m/s = 0.25 kg × v'1 + 0.4 kg × v'2

Solving for v'1 and v'2, we get:
v'1 = 1.2 m/s
v'2 = 0.6 m/s

This means that after the collision, the first object will be moving with a velocity of 1.2 m/s, and the second object will be moving with a velocity of 0.6 m/s, in opposite directions.

Theorems and Principles

1. Conservation of Momentum: The total momentum of a closed system remains constant before and after a collision.
2. Coefficient of Restitution: The coefficient of restitution, denoted as e, is a measure of the elasticity of a collision. It ranges from 0 (completely inelastic collision) to 1 (perfectly elastic collision).
3. Inelastic Collision: In an inelastic collision, the colliding objects stick together after the collision, and their final velocity is a weighted average of their initial velocities.
4. Elastic Collision: In an elastic collision, the colliding objects do not stick together, and their final velocities depend on the angle of collision, the masses of the objects, and the coefficient of restitution.

Formulas and Equations

1. Velocity After Inelastic Collision:
   v' = (m1v1 + m2v2) / (m1 + m2)

2. Conservation of Momentum in Elastic Collision:
   m1v1 + m2v2 = m1v'1 + m2v'2

3. Coefficient of Restitution:
   e = (v'2 - v'1) / (v2 - v1)

Examples and Numerical Problems

1. Inelastic Collision Example:
2. Two objects with masses of 0.6 kg and 0.8 kg are moving with initial velocities of 4 m/s and 2 m/s, respectively.
3. Calculate the final velocity after the inelastic collision.

4. Solution: v' = (0.6 kg × 4 m/s + 0.8 kg × 2 m/s) / (0.6 kg + 0.8 kg) = 3.2 m/s

5. Elastic Collision Example:

6. Two objects with masses of 0.25 kg and 0.4 kg are moving with initial velocities of 2 m/s and 0 m/s, respectively.
7. Calculate the final velocities after the elastic collision.

8. Solution: m1v1 + m2v2 = m1v'1 + m2v'2
0.25 kg × 2 m/s + 0.4 kg × 0 m/s = 0.25 kg × v'1 + 0.4 kg × v'2
0.5 kg m/s = 0.25 kg × v'1 + 0.4 kg × v'2
   Solving for v'1 and v'2, we get:
   v'1 = 1.2 m/s
v'2 = 0.6 m/s

9. Numerical Problem:

10. Two objects with masses of 1.5 kg and 2.0 kg are moving with initial velocities of 3 m/s and -2 m/s, respectively.
11. Calculate the final velocity after an inelastic collision.

12. Solution: v' = (1.5 kg × 3 m/s + 2.0 kg × (-2 m/s)) / (1.5 kg + 2.0 kg) = 0.6 m/s

13. Numerical Problem:

14. Two objects with masses of 0.8 kg and 1.2 kg are moving with initial velocities of 4 m/s and -3 m/s, respectively.
15. Calculate the final velocities after an elastic collision, given that the coefficient of restitution is 0.8.
16. Solution: m1v1 + m2v2 = m1v'1 + m2v'2
0.8 kg × 4 m/s + 1.2 kg × (-3 m/s) = 0.8 kg × v'1 + 1.2 kg × v'2
3.2 kg m/s - 3.6 kg m/s = 0.8 kg × v'1 + 1.2 kg × v'2
v'1 = 1.6 m/s
v'2 = -2 m/s

Figures and Data Points

1. Collision Angle Diagram:
   
   This diagram illustrates the angle of collision in an elastic collision, which is a crucial factor in determining the final velocities of the objects.

2. Coefficient of Restitution Data:
   | Material Combination | Coefficient of Restitution (e) |
   | ——————– | —————————— |
   | Steel-Steel | 0.8 – 0.9 |
   | Steel-Wood | 0.5 – 0.6 |
   | Rubber-Concrete | 0.3 – 0.4 |
   | Glass-Glass | 0.9 – 0.95 |

The coefficient of restitution values provided in this table can be used to estimate the elasticity of a collision between different materials.

Conclusion

The velocity after collision formula is a fundamental concept in physics that allows us to calculate the final velocities of objects involved in both inelastic and elastic collisions. By understanding the principles of conservation of momentum and the coefficient of restitution, physics students can solve a wide range of problems related to collisions and their effects on the motion of objects. The examples and numerical problems provided in this guide should help students develop a deeper understanding of this topic and apply the concepts in real-world scenarios.

References

1. How to Find the Velocity of Two Objects after Collision – Dummies.com
2. Final Velocity in Inelastic Collision | Formula & Examples – Study.com
3. Collisions – Physics LibreTexts
4. Coefficient of Restitution | Formula & Equation – Lesson – Study.com
5. Experiment 5: Conservation of Momentum – Ole Miss Physics