The Terminal Velocity Formula: A Comprehensive Guide for Physics Students

Summary

The terminal velocity formula is a fundamental equation in physics that describes the maximum velocity an object can reach while falling through a medium, such as air or a fluid. This formula is derived from Newton’s second law of motion and takes into account the force of gravity, the mass of the object, and the drag force exerted by the medium. By understanding and applying this formula, physics students can gain valuable insights into the behavior of falling objects and their interactions with the surrounding environment.

Understanding the Terminal Velocity Formula

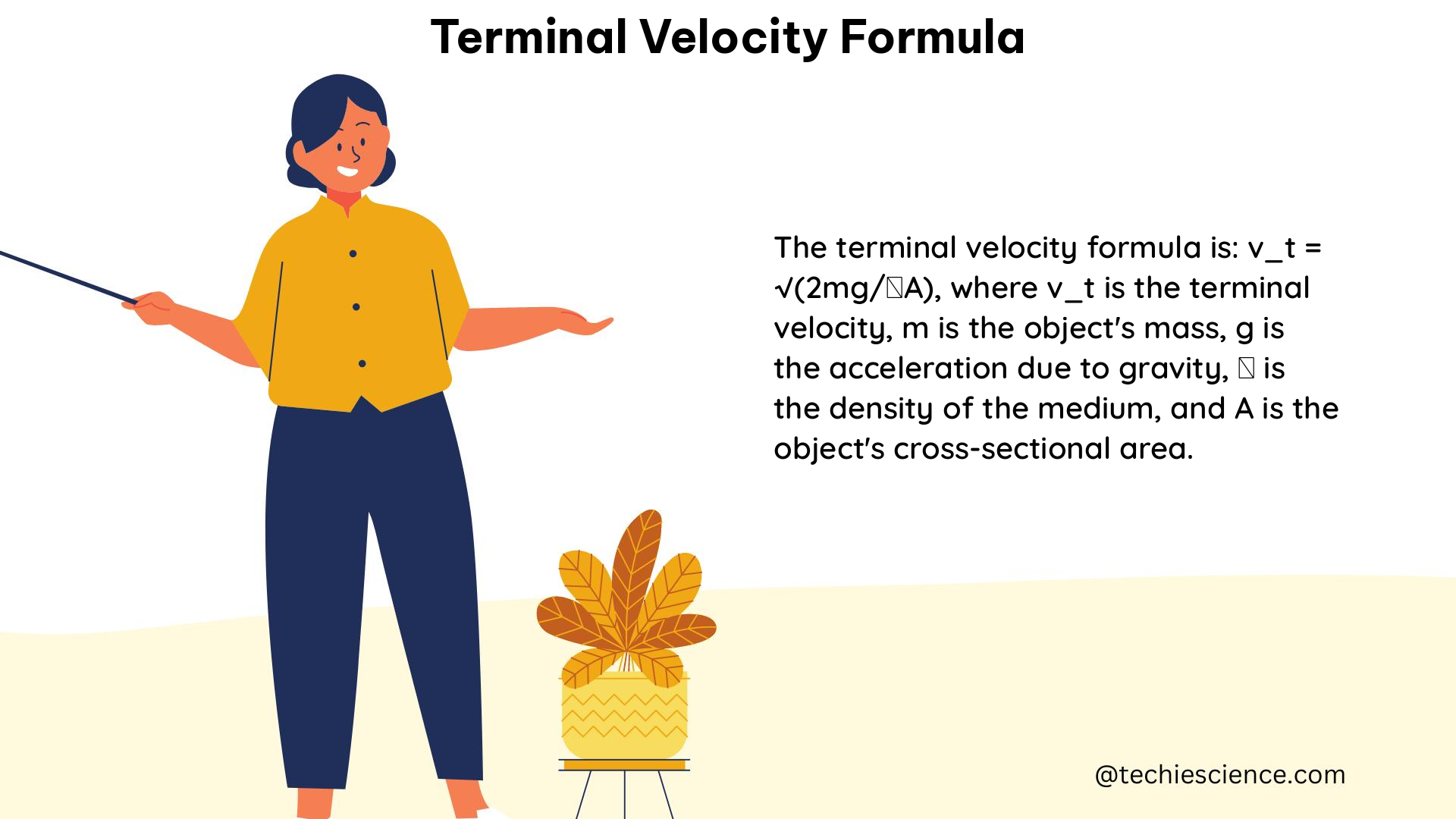
The terminal velocity formula is given by:

vT = sqrt((2mg)/(CdAρ))

where:

• vT is the terminal velocity
• m is the mass of the object
• g is the acceleration due to gravity
• Cd is the drag coefficient, which depends on the shape and orientation of the object
• A is the cross-sectional area of the object
• ρ is the density of the medium

This formula shows that the terminal velocity of an object is influenced by several key factors, including its mass, the acceleration due to gravity, the drag coefficient, the cross-sectional area, and the density of the medium.

Theorem and Derivation

The terminal velocity formula is derived from Newton’s second law of motion, which states that the net force acting on an object is equal to the product of its mass and acceleration. In the case of a falling object, the net force is the difference between the force of gravity and the drag force.

The force of gravity is given by mg, where m is the mass of the object and g is the acceleration due to gravity. The drag force, on the other hand, is proportional to the square of the velocity and the cross-sectional area of the object, and is given by (1/2)CdAρv^2, where Cd is the drag coefficient, A is the cross-sectional area, ρ is the density of the medium, and v is the velocity of the object.

At terminal velocity, the net force on the object is zero, meaning that the force of gravity is balanced by the drag force. Equating these two forces and solving for the velocity, we arrive at the terminal velocity formula.

Physics Examples

1. Skydiving: Consider a skydiver jumping out of an airplane. The mass of the skydiver, the acceleration due to gravity, and the density of air are all known quantities. By measuring the cross-sectional area of the skydiver’s body and the drag coefficient, it is possible to calculate the terminal velocity using the formula.

2. Falling Feather: Imagine a feather falling through the air. The mass of the feather is relatively small, but its cross-sectional area is large compared to its mass. As a result, the feather will have a low terminal velocity, allowing it to float gently to the ground.

3. Falling Sphere: A sphere falling through a fluid, such as water or air, will have a terminal velocity that depends on its mass, diameter, and the fluid’s density and viscosity. The terminal velocity formula can be used to predict the behavior of such objects.

Physics Numerical Problems

1. Calculating Terminal Velocity of a Skydiver
2. Given:
   • Mass of the skydiver: 80 kg
   • Acceleration due to gravity: 9.8 m/s^2
   • Drag coefficient: 0.7
   • Cross-sectional area: 0.5 m^2
   • Air density: 1.225 kg/m^3

3. Calculate the terminal velocity of the skydiver.

4. Determining the Mass of a Falling Object

5. Given:
   • Terminal velocity: 50 m/s
   • Drag coefficient: 0.3
   • Cross-sectional area: 0.2 m^2
   • Air density: 1.225 kg/m^3
   • Acceleration due to gravity: 9.8 m/s^2

6. Calculate the mass of the falling object.

7. Estimating the Drag Coefficient of a Falling Object

8. Given:
   • Terminal velocity: 30 m/s
   • Mass of the object: 2 kg
   • Cross-sectional area: 0.1 m^2
   • Air density: 1.225 kg/m^3
   • Acceleration due to gravity: 9.8 m/s^2
9. Calculate the drag coefficient of the falling object.

These numerical problems demonstrate how the terminal velocity formula can be applied to solve real-world physics problems and gain a deeper understanding of the factors that influence the motion of falling objects.

Figures and Data Points

To further illustrate the concepts related to the terminal velocity formula, consider the following figures and data points:

Figure 1: Relationship between Terminal Velocity and Object Mass
This graph shows the relationship between the terminal velocity of an object and its mass, assuming constant values for the other parameters (drag coefficient, cross-sectional area, and medium density).

Table 1: Typical Drag Coefficients for Various Shapes
This table provides the typical drag coefficients for different object shapes, which is an important parameter in the terminal velocity formula.

Shape	Drag Coefficient (Cd)
Sphere	0.47
Cube	0.80
Cylinder (side on)	0.82
Cylinder (end on)	0.50
Flat Plate	1.28

Data Point 1: Terminal Velocity of a Skydiver
A skydiver with a mass of 80 kg, a cross-sectional area of 0.5 m^2, and a drag coefficient of 0.7 has a terminal velocity of approximately 60 m/s in air with a density of 1.225 kg/m^3.

Data Point 2: Terminal Velocity of a Falling Feather
A feather with a mass of 0.1 g and a cross-sectional area of 0.01 m^2, falling through air with a density of 1.225 kg/m^3 and a drag coefficient of 0.8, has a terminal velocity of approximately 0.5 m/s.

These figures and data points provide a more comprehensive understanding of the terminal velocity formula and its practical applications.

Conclusion

The terminal velocity formula is a fundamental equation in physics that allows us to predict the maximum velocity an object can reach while falling through a medium. By understanding the factors that influence terminal velocity, such as mass, drag coefficient, cross-sectional area, and medium density, physics students can gain valuable insights into the behavior of falling objects and their interactions with the surrounding environment.

Through the examples, numerical problems, and data points presented in this guide, you should now have a solid understanding of the terminal velocity formula and its practical applications. Remember to continue exploring and experimenting with this formula to deepen your knowledge and apply it to real-world scenarios.

References

1. StudySmarter. (2021). Terminal Velocity: Definition, Formula, Speed, Calculate. Retrieved from https://www.studysmarter.co.uk/explanations/physics/mechanics-and-materials/terminal-velocity/
2. Study.com. (2022). How to Calculate the Terminal Velocity of a Moving Object Subject to a Given Resistive Force. Retrieved from https://study.com/skill/learn/how-to-calculate-the-terminal-velocity-of-a-moving-object-subject-to-a-given-resistive-force-explanation.html
3. LibreTexts. (2024). Unit 8 Lab Extension- Modeling Terminal Velocity and Extracting Drag Coefficient. Retrieved from https://phys.libretexts.org/Bookshelves/Conceptual_Physics/Body_Physics_-_Motion_to_Metabolism_%28Davis%29/14:_Lab_Extension_Activities/14.06:_Unit_8_Lab_Extension-_Modeling_Terminal_Velocity_and_Extracting_Drag_Coefficient%2A
4. GeeksforGeeks. (2024). Terminal Velocity Formula. Retrieved from https://www.geeksforgeeks.org/terminal-velocity-formula/