The Telescope Diffraction Limit Formula: A Comprehensive Guide

The telescope diffraction limit formula is a fundamental concept in optics that determines the maximum resolution achievable by a telescope. This formula, derived from the principles of wave optics, is crucial for understanding the performance and capabilities of telescopes in various astronomical applications. In this comprehensive guide, we will delve into the intricacies of the telescope diffraction limit formula, providing a detailed exploration of its theoretical foundations, practical applications, and the factors that influence its implementation.

Understanding the Telescope Diffraction Limit Formula

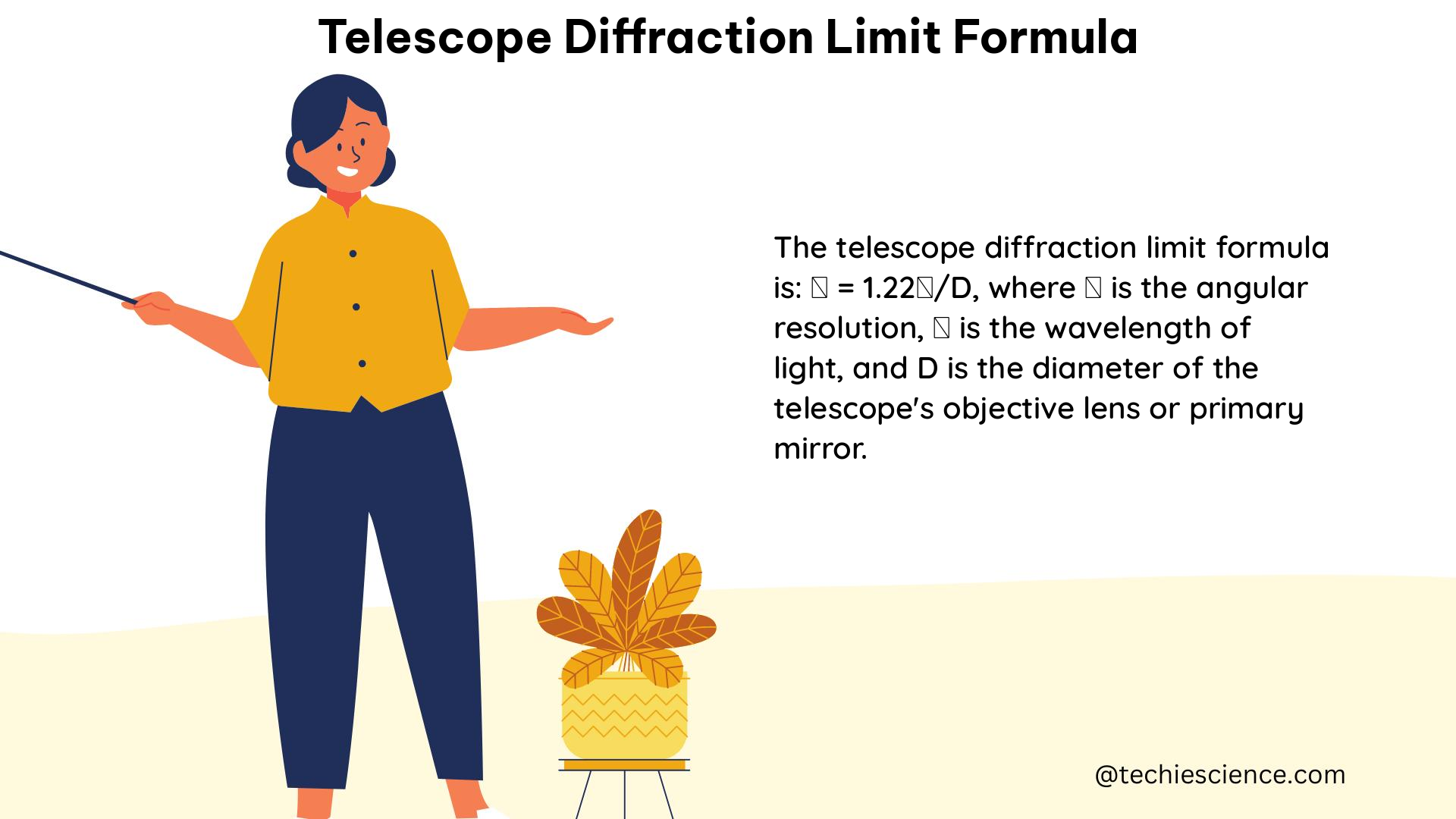
The telescope diffraction limit formula is expressed as:

θ = 1.22 λ / D

Where:
– θ is the angular resolution of the telescope, measured in radians
– λ is the wavelength of the observed light, measured in meters
– D is the diameter of the telescope’s objective (primary mirror or lens), measured in meters

This formula, known as the Rayleigh criterion, states that the minimum angular separation between two distinct objects that can be resolved by a telescope is determined by the wavelength of the observed light and the diameter of the telescope’s objective.

Theoretical Foundations

The telescope diffraction limit formula is derived from the principles of wave optics, specifically the phenomenon of diffraction. When light passes through an aperture, such as the objective of a telescope, it undergoes diffraction, resulting in a characteristic diffraction pattern. The Rayleigh criterion, which forms the basis of the telescope diffraction limit formula, states that two point sources are considered just resolvable when the center of the diffraction pattern of one source coincides with the first minimum of the diffraction pattern of the other.

The mathematical derivation of the telescope diffraction limit formula involves the analysis of the Airy disk, which is the diffraction pattern produced by a circular aperture. The diameter of the Airy disk is inversely proportional to the diameter of the telescope’s objective, and the angular size of the Airy disk is given by the telescope diffraction limit formula.

Practical Applications

The telescope diffraction limit formula has numerous practical applications in the field of astronomy and astrophysics. It is used to determine the maximum achievable resolution of a telescope, which is crucial for observing and studying celestial objects with high precision.

For example, the Hubble Space Telescope, with a primary mirror diameter of 2.4 meters, has an angular resolution of approximately 2.8 × 10^-7 radians for light with a wavelength of 550 nanometers. This corresponds to a spatial resolution of 0.56 light-years for objects at a distance of 2 million light-years.

The telescope diffraction limit formula is also used to design and optimize the optical systems of telescopes, ensuring that the instrument’s resolution matches the scientific objectives of the observation. It is particularly important in the development of large ground-based telescopes, where the effects of atmospheric turbulence can significantly degrade the image quality.

Factors Influencing the Telescope Diffraction Limit

While the telescope diffraction limit formula provides a theoretical limit to the resolution of a telescope, there are several factors that can influence the actual achievable resolution in practice:

1. Atmospheric Turbulence: The Earth’s atmosphere can cause distortions and blurring of the observed images, limiting the telescope’s resolution. Adaptive optics systems are often employed to correct for these atmospheric effects.

2. Exposure Time: Longer exposure times can improve the signal-to-noise ratio and enhance the resolution of the observed images, but they are also susceptible to the effects of atmospheric turbulence.

3. Image Processing Techniques: Advanced image processing techniques, such as image stacking and deconvolution, can be used to enhance the resolution of the observed images, often surpassing the theoretical diffraction limit.

4. Observing Wavelength: The telescope diffraction limit formula is wavelength-dependent, so the choice of observing wavelength can impact the achievable resolution. Shorter wavelengths, such as those in the visible or ultraviolet range, generally provide higher resolution compared to longer wavelengths, such as those in the infrared range.

5. Telescope Design: The specific design and construction of the telescope, including the shape and quality of the primary mirror or lens, can also influence the achievable resolution.

Numerical Examples and Calculations

To illustrate the application of the telescope diffraction limit formula, let’s consider a few numerical examples:

1. Example 1: Suppose you have a telescope with a primary mirror diameter of 4 meters and you are observing at a wavelength of 500 nanometers. What is the theoretical angular resolution of the telescope?

Using the telescope diffraction limit formula:
θ = 1.22 λ / D
θ = 1.22 × (500 × 10^-9 m) / (4 m)
θ = 3.05 × 10^-7 radians

1. Example 2: The Hubble Space Telescope has a primary mirror diameter of 2.4 meters and observes at a wavelength of 550 nanometers. What is the theoretical angular resolution of the Hubble Space Telescope?

Using the telescope diffraction limit formula:
θ = 1.22 λ / D
θ = 1.22 × (550 × 10^-9 m) / (2.4 m)
θ = 2.80 × 10^-7 radians

1. Example 3: Suppose you have a ground-based telescope with a primary mirror diameter of 8 meters and you are observing at a wavelength of 650 nanometers. What is the theoretical angular resolution of the telescope, and how does it compare to the Rayleigh limit and the Dawes limit?

Using the telescope diffraction limit formula:
θ = 1.22 λ / D
θ = 1.22 × (650 × 10^-9 m) / (8 m)
θ = 9.88 × 10^-8 radians

The Rayleigh limit is given by θ_Rayleigh = 1.22 λ / D, which in this case is 9.88 × 10^-8 radians.
The Dawes limit is given by θ_Dawes = 116 / D (in arcseconds), which in this case is 0.0145 arcseconds.

Comparing the calculated angular resolution to the Rayleigh and Dawes limits:
– Angular resolution: 9.88 × 10^-8 radians
– Rayleigh limit: 9.88 × 10^-8 radians
– Dawes limit: 0.0145 arcseconds (6.98 × 10^-7 radians)

In this case, the calculated angular resolution matches the Rayleigh limit, indicating that the telescope is operating at the theoretical diffraction limit.

These examples demonstrate the practical application of the telescope diffraction limit formula and how it can be used to assess the performance and capabilities of different telescopes.

Conclusion

The telescope diffraction limit formula is a fundamental concept in optics that plays a crucial role in understanding the resolution and performance of telescopes. By exploring the theoretical foundations, practical applications, and the factors that influence the telescope diffraction limit, this comprehensive guide provides a deep understanding of this important topic. The numerical examples and calculations presented further illustrate the practical implementation of the telescope diffraction limit formula, equipping readers with the knowledge to analyze and optimize the resolution of telescopes in various astronomical applications.

References

1. Limits of Resolution: The Rayleigh Criterion | Physics, https://courses.lumenlearning.com/suny-physics/chapter/27-6-limits-of-resolution-the-rayleigh-criterion/
2. Telescope Equations: Resolving Power – RocketMime, https://www.rocketmime.com/astronomy/Telescope/ResolvingPower.html
3. Diffraction-limited system – Wikipedia, https://en.wikipedia.org/wiki/Diffraction-limited_system
4. Telescope Resolving Power and the Rayleigh Criterion – Astronomy Notes, https://www.astronomynotes.com/telescop/s3.htm
5. Telescope Diffraction Limit – Hyperphysics, http://hyperphysics.phy-astr.gsu.edu/hbase/geoopt/telres.html