Telescope Chromatic Aberration Formula: A Comprehensive Guide

Summary

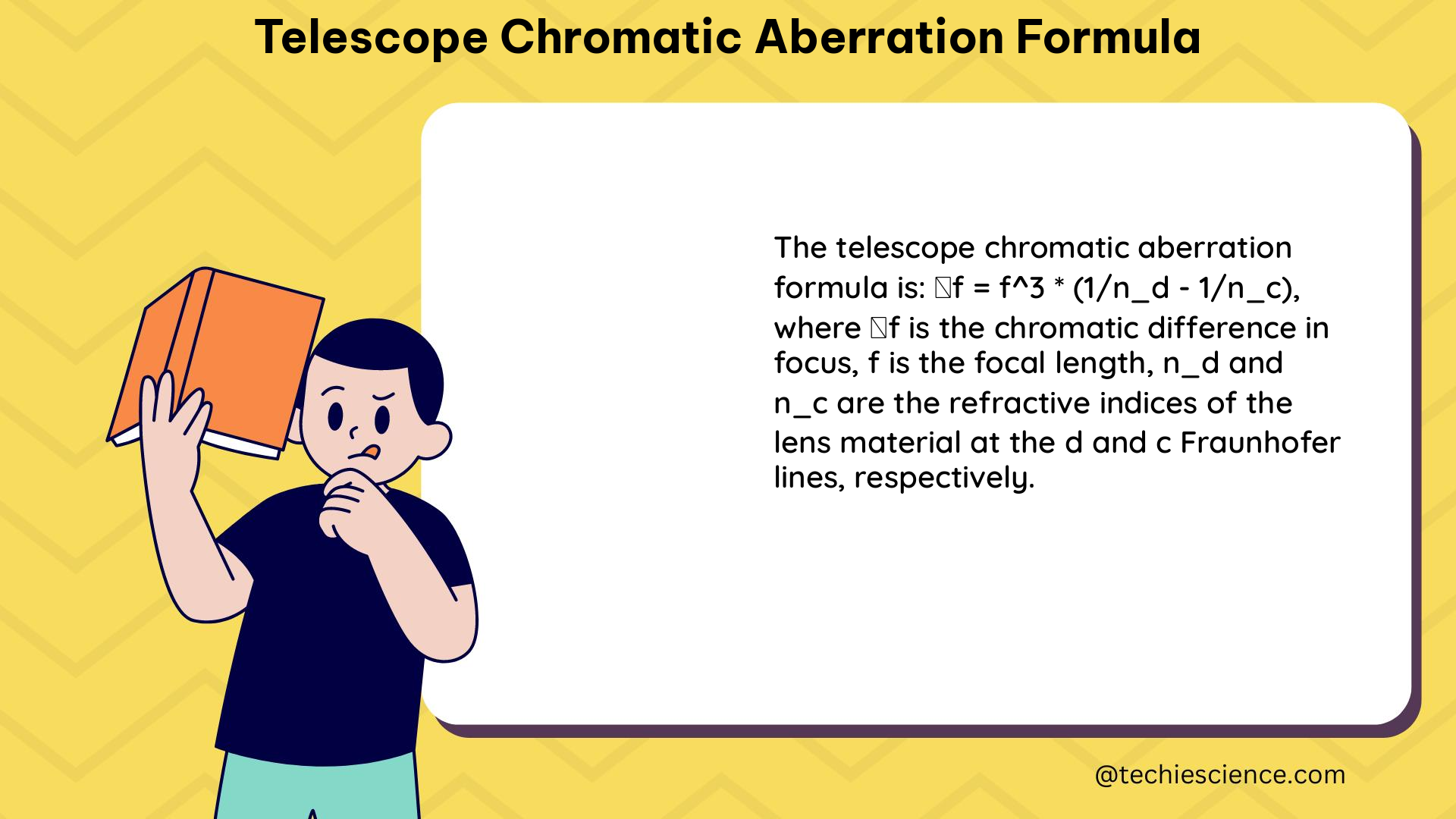
The formula for chromatic aberration (CA) in telescopes is given by Cc = Dc / M / λ / d ΔV / V, which is derived from the lensmaker’s equation. CA is a measure of the difference in focal length between the red, green, and blue color channels, and is expressed in units of distance in pixels. The visibility of the CA is proportional to the area between the edges with the highest amplitude (red) and the lowest (blue), which can be calculated using the integral formula CA (area) = ∫ [Edgemax(x) – Edgemin(x)] dx. This guide provides a detailed explanation of the telescope chromatic aberration formula, including its derivation, the factors that influence it, and how to measure and minimize CA in telescopes.

Understanding Chromatic Aberration in Telescopes

Chromatic aberration (CA) is a common problem in telescopes that can degrade the image quality. It occurs due to the fact that different wavelengths of light (colors) have different refractive indices when passing through a lens, resulting in a difference in the focal length for each color. This leads to a blurring or fringing effect around the edges of the image, particularly for high-contrast objects.

The formula for chromatic aberration, Cc = Dc / M / λ / d ΔV / V, is derived from the lensmaker’s equation, which describes the relationship between the focal length of a lens and its curvature, refractive index, and the wavelength of light. Let’s break down the components of this formula:

• Cc: Chromatic aberration, expressed in units of distance (typically pixels)
• Dc: Diameter of the lens or objective
• M: Magnification of the telescope
• λ: Wavelength of light
• d: Distance from the lens to the image plane
• ΔV: Difference in refractive index between the red and blue wavelengths
• V: Average refractive index of the lens material

Factors Influencing Chromatic Aberration

The chromatic aberration formula reveals several key factors that contribute to the severity of CA in a telescope:

1. Lens Diameter (Dc): Larger lenses tend to have more pronounced chromatic aberration, as the difference in focal length between different wavelengths becomes more significant.

2. Magnification (M): Higher magnification telescopes amplify the effects of chromatic aberration, making it more visible in the final image.

3. Wavelength (λ): The difference in refractive index between red and blue wavelengths (ΔV) is the primary driver of chromatic aberration. Telescopes designed for specific wavelength ranges (e.g., visible light, infrared, or ultraviolet) may have reduced CA.

4. Lens-to-Image Distance (d): As the distance between the lens and the image plane increases, the chromatic aberration becomes more pronounced.

5. Refractive Index Difference (ΔV): The difference in refractive index between the red and blue wavelengths (ΔV) is a material property of the lens. Lenses made from different types of glass can have varying degrees of ΔV, which affects the severity of CA.

6. Average Refractive Index (V): The average refractive index of the lens material (V) also plays a role in the chromatic aberration formula. Lenses with higher average refractive indices tend to have more pronounced CA.

Measuring Chromatic Aberration

There are two primary methods for measuring the chromatic aberration in a telescope:

1. Integral Formula: The integral formula for chromatic aberration is CA (area) = ∫ [Edgemax(x) – Edgemin(x)] dx. This formula calculates the area between the edges with the highest amplitude (red) and the lowest amplitude (blue), which is proportional to the visibility of the chromatic aberration.

2. Lonely Speck Aberration Test: The Lonely Speck Aberration Test measures the width of the chromatic aberration at its longest point (in pixels) and compares it as a percentage of the height of the image. For example, an aberration of 62 pixels wide in a 4000-pixel image would result in an aberration level of approximately 1.5%.

By using these measurement techniques, you can quantify the level of chromatic aberration in your telescope and compare it to the theoretical predictions based on the chromatic aberration formula.

Minimizing Chromatic Aberration

There are several strategies for minimizing chromatic aberration in telescopes:

1. Lens Design: Combining glass elements with different dispersion properties (different ΔV values) can help to cancel out the chromatic aberration. This is the principle behind achromatic and apochromatic lens designs.

2. Lens Coatings: Applying specialized coatings to the lens surfaces can help to reduce the reflections and improve the transmission of specific wavelengths, reducing the overall chromatic aberration.

3. Digital Correction: In many digital camera and telescope designs, chromatic aberration can be corrected in the digital domain if the correction is applied before the demosaicing process.

4. Wavelength Selection: Choosing a telescope design that is optimized for a specific wavelength range (e.g., visible light, infrared, or ultraviolet) can help to minimize the effects of chromatic aberration.

5. Aperture Stops: Reducing the effective aperture of the telescope can also help to mitigate chromatic aberration, as it reduces the overall light path through the lens.

By understanding the telescope chromatic aberration formula and the factors that influence it, you can make informed decisions about the design and use of your telescope to achieve the best possible image quality.

Examples and Numerical Problems

1. Example 1: Calculating Chromatic Aberration for a Telescope
2. Lens Diameter (Dc) = 100 mm
3. Magnification (M) = 50x
4. Wavelength (λ) = 550 nm (green light)
5. Lens-to-Image Distance (d) = 1000 mm
6. Refractive Index Difference (ΔV) = 0.02
7. Average Refractive Index (V) = 1.5

Using the chromatic aberration formula, Cc = Dc / M / λ / d ΔV / V, we can calculate the chromatic aberration:
Cc = 100 mm / 50 / 550 nm / 1000 mm * 0.02 / 1.5 = 0.073 mm or 73 μm

1. Numerical Problem 1: Calculating the Aberration Level using the Lonely Speck Aberration Test
2. Aberration Width = 62 pixels
3. Image Height = 4000 pixels

Aberration Level = (Aberration Width / Image Height) * 100%
Aberration Level = (62 / 4000) * 100% = 1.55%

1. Example 2: Comparing Chromatic Aberration in Different Telescope Designs
2. Telescope A: Dc = 150 mm, M = 75x, λ = 600 nm, d = 1500 mm, ΔV = 0.018, V = 1.52
3. Telescope B: Dc = 80 mm, M = 40x, λ = 500 nm, d = 800 mm, ΔV = 0.022, V = 1.48

Using the chromatic aberration formula, we can calculate the CA for each telescope:
Telescope A: Cc = 150 / 75 / 600e-9 / 1500 * 0.018 / 1.52 = 0.089 mm or 89 μm
Telescope B: Cc = 80 / 40 / 500e-9 / 800 * 0.022 / 1.48 = 0.088 mm or 88 μm

In this case, Telescope A has a slightly higher chromatic aberration due to its larger lens diameter and higher magnification, despite the slightly lower refractive index difference.

Conclusion

The telescope chromatic aberration formula, Cc = Dc / M / λ / d ΔV / V, provides a comprehensive understanding of the factors that contribute to chromatic aberration in telescopes. By understanding this formula and the associated principles, you can design, optimize, and use your telescope to minimize the effects of chromatic aberration and achieve the best possible image quality.

References

1. Chromatic Aberration Measurement and Correction
2. Chromatic Aberration in Computer Science
3. A Practical Guide to Lens Aberrations and the Lonely Speck Aberration Test
4. Chromatic Aberration in Optical Systems
5. Achromatic and Apochromatic Lens Design