Velocity

Relative velocity is a fundamental concept in physics, particularly in the field of kinematics, which deals with the motion of objects without considering the forces or masses involved. It refers to the velocity of an object as measured with respect to a given observer, which can differ from the measurement obtained by another observer. In the context of relative velocity in opposite directions, this concept becomes particularly important, as it allows us to understand the interactions between objects moving in opposite directions.

Understanding Relative Velocity Formulas

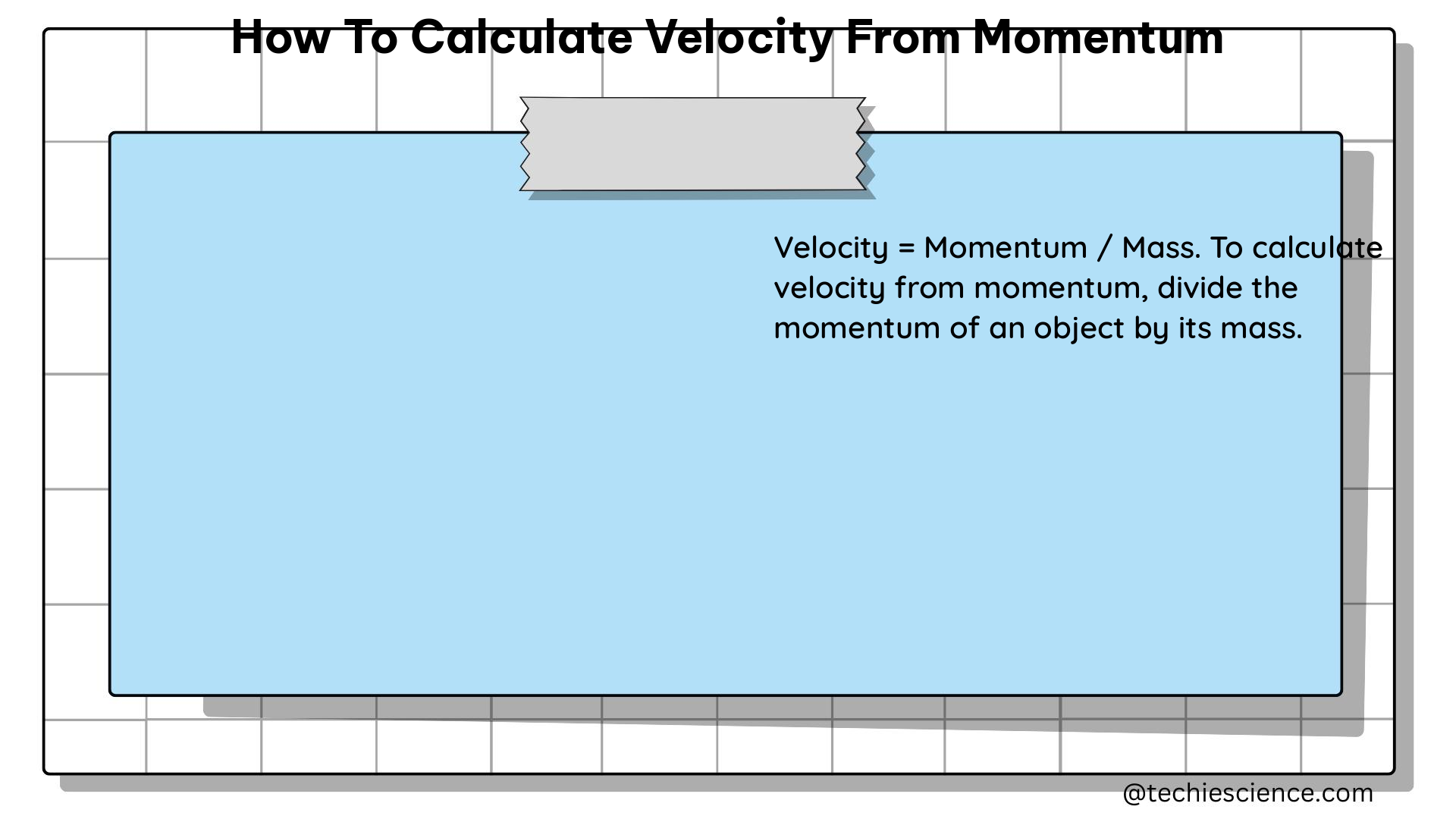
The formula for calculating the relative velocity of an object A with respect to an observer B is:

Vab = Va - Vb

Where:
– Vab is the relative velocity of object A with respect to observer B
– Va is the velocity of object A measured by a third observer
– Vb is the velocity of observer B measured by the same third observer

This formula is crucial in understanding the relative motion of objects, especially when they are moving in opposite directions.

Relative Velocity in Opposite Direction: Examples

Example 1: Two Cars Moving Towards Each Other

Consider two cars moving towards each other on a straight road. If one car is moving at a velocity of 60 mph northbound, and the other car is moving at the same speed of 60 mph southbound, the relative velocity between them is:

Vab = Va - Vb
Vab = 60 mph - (-60 mph)
Vab = 120 mph

This indicates that the two cars are approaching each other at a combined speed of 120 mph.

Example 2: Man Running on a Highway

Imagine a man running on a highway to the west at a velocity of 10 mph, while observing a car coming in the opposite direction at a speed of 80 mph. The relative velocity of the car with respect to the man is:

Vab = Va - Vb
Vab = 80 mph - (-10 mph)
Vab = -90 mph

The negative sign indicates that the car is moving eastward at a speed of 90 mph relative to the man.

Relative Velocity in Fluid Dynamics

In the context of fluid dynamics, relative velocity plays a significant role in understanding the flow of fluids and gases. For instance, when an airplane encounters a tailwind, the wind’s velocity contributes to the plane’s resulting velocity, increasing its speed relative to an observer on the ground. Conversely, a headwind would decrease the plane’s resulting velocity, while a crosswind would change its direction.

The relative velocity between the airplane and the wind can be calculated using the same formula:

Vab = Va - Vb

Where:
– Vab is the relative velocity of the airplane with respect to the wind
– Va is the velocity of the airplane measured by an observer on the ground
– Vb is the velocity of the wind measured by the same observer on the ground

Relative Velocity in Special Relativity

In the context of special relativity, the relative velocity of two objects moving at the speed of light in opposite directions is 2c, where c is the speed of light. However, it is important to note that this does not imply that the objects are moving faster than the speed of light; rather, it reflects the change in separation distance/time for the two different objects moving relative to a reference frame.

The formula for calculating the relative velocity in special relativity is:

Vab = (Va - Vb) / (1 - (Va * Vb) / c^2)

Where:
– Vab is the relative velocity of object A with respect to object B
– Va is the velocity of object A
– Vb is the velocity of object B
– c is the speed of light

Numerical Problems on Relative Velocity in Opposite Direction

1. Two cars are moving towards each other on a straight road. Car A is moving at a velocity of 80 km/h northbound, and Car B is moving at a velocity of 60 km/h southbound. Calculate the relative velocity between the two cars.

2. A person is running on a track at a velocity of 8 m/s westbound, while observing a car moving in the opposite direction at a velocity of 20 m/s. Calculate the relative velocity of the car with respect to the person.

3. An airplane is flying at a velocity of 500 km/h eastbound, while encountering a headwind of 80 km/h. Calculate the relative velocity of the airplane with respect to an observer on the ground.

4. Two spaceships are moving at the speed of light in opposite directions. Calculate the relative velocity between the two spaceships.

Conclusion

Relative velocity is a crucial concept in physics, with applications in various fields such as kinematics, fluid dynamics, and special relativity. It involves the measurement of an object’s velocity with respect to a given observer, taking into account the direction and magnitude of the velocities involved. The formula for calculating relative velocity varies depending on whether the objects are moving in the same or opposite directions, and understanding this concept is essential for understanding the motion of objects in various contexts.

References:
– Understanding Relative Velocity in Kinematics
– Relative Velocity and River Boat Problems
– Is the Maximum Possible Measurable Velocity 2c?

Centripetal acceleration is a fundamental concept in physics that describes the change in the direction of an object’s velocity as it moves in a circular path. This acceleration is directed towards the center of the circular motion and is essential in understanding various phenomena, from the motion of planets to the dynamics of amusement park rides. In this comprehensive guide, we will delve into the intricacies of centripetal acceleration and velocity, exploring the underlying principles, mathematical formulations, and practical applications.

Understanding Centripetal Acceleration

Centripetal acceleration, denoted as a_c, is the rate of change in the direction of an object’s velocity as it moves in a circular path. It is calculated by dividing the square of the object’s speed (v) by the radius (r) of the circular trajectory:

a_c = v^2 / r

This formula is derived from the vector nature of velocity, where the direction of the velocity vector is constantly changing in circular motion. The centripetal acceleration is the component of the acceleration that is directed towards the center of the circular path.

Numerical Example

Consider an object moving in a circular path with a radius of 5 meters and a speed of 4 meters per second. The centripetal acceleration can be calculated as follows:

a_c = (4 m/s)^2 / 5 m = 16 m^2/s^2 / 5 m = 3.2 m/s^2

This means that the direction of the object’s velocity is changing at a rate of 3.2 meters per second squared.

Centripetal Force and Circular Motion

Centripetal acceleration is closely related to the concept of centripetal force, which is the force responsible for causing the change in the direction of an object’s velocity. The relationship between centripetal acceleration and centripetal force is given by the formula:

F_c = m * a_c

where F_c is the centripetal force, and m is the mass of the object.

In the previous example, if the mass of the object is 2 kilograms, the centripetal force can be calculated as:

F_c = 2 kg * 3.2 m/s^2 = 6.4 N

This means that a force of 6.4 Newtons is required to keep the object moving in a circular path with a radius of 5 meters and a speed of 4 meters per second.

Uniform Circular Motion

When an object moves in a circular path with a constant speed, it is said to be in uniform circular motion. In this case, the centripetal acceleration is constant, and the centripetal force is also constant. The velocity of the object is perpendicular to the centripetal force, and the object’s path is a perfect circle.

Non-uniform Circular Motion

In non-uniform circular motion, the speed of the object is not constant, and the centripetal acceleration and centripetal force can vary over time. In this case, the object’s path may not be a perfect circle, and the velocity vector may not be perpendicular to the centripetal force.

Numerical Problems and Applications

Centripetal acceleration and velocity can be applied to a wide range of problems in physics, from the motion of planets to the dynamics of amusement park rides. Here are some examples of numerical problems and applications:

Problem 1: Calculating Velocity from Centripetal Acceleration and Radius

A particle’s centripetal acceleration is a_c = 4.0 m/s^2 at t = 0 s. It is executing uniform circular motion about an axis at a distance of r = 5.0 m. What is its velocity at t = 10 s?

To solve this problem, we can use the formula for centripetal acceleration:

a_c = v^2 / r

Rearranging the formula, we can solve for the velocity:

v = sqrt(a_c * r)
v = sqrt(4.0 m/s^2 * 5.0 m)
v = 4.0 m/s

Therefore, the velocity of the particle at t = 10 s is 4.0 meters per second.

Problem 2: Calculating Centripetal Force from Mass, Radius, and Velocity

An object with a mass of 2.5 kg is moving in a circular path with a radius of 3.0 m and a speed of 6.0 m/s. Calculate the centripetal force acting on the object.

To solve this problem, we can use the formula for centripetal force:

F_c = m * v^2 / r
F_c = 2.5 kg * (6.0 m/s)^2 / 3.0 m
F_c = 2.5 kg * 36 m^2/s^2 / 3.0 m
F_c = 30 N

Therefore, the centripetal force acting on the object is 30 Newtons.

Application: Circular Motion in Amusement Park Rides

Centripetal acceleration and velocity are crucial in the design and operation of amusement park rides that involve circular motion, such as Ferris wheels, loop-the-loops, and spinning rides. Engineers must carefully calculate the centripetal acceleration and centripetal force to ensure the safety and comfort of the riders, as well as the structural integrity of the ride.

For example, in a loop-the-loop ride, the centripetal acceleration experienced by the riders must be carefully controlled to prevent them from experiencing excessive forces or becoming disoriented. The radius of the loop and the speed of the ride are key factors in determining the centripetal acceleration, which must be within a safe range for the riders.

Conclusion

Centripetal acceleration and velocity are fundamental concepts in the study of circular motion and rotational dynamics. By understanding the underlying principles, mathematical formulations, and practical applications of these concepts, students and professionals in the field of physics can gain a deeper understanding of the behavior of objects moving in circular paths. This knowledge is essential for a wide range of applications, from the motion of celestial bodies to the design of amusement park rides.

References:

1. Centripetal Acceleration | Physics – Lumen Learning
2. Problem 87 A particle’s centripetal acceleration is (a_mC = 4.0 m/s^2) at (t=0 s). It is executing uniform circular motion about an axis at a distance of (5.0 m). What is its velocity at (t=10 s)?
3. Proof of centripetal acceleration formula (a_c = v^2/r) for non-uniform circular motion
4. Centripetal Acceleration | Definition, Equation & Formula – Lesson
5. Centripetal acceleration (Item No.: P6000560)