What is Relative Motion?
The relative motion is the motion between two interacting bodies defined as
“The motion of the body is moving with respect to another body which is either moving or at rest, known as relative motion between both interacting bodies.”
Relative Motion Examples
Let’s take one of the examples that explain the meaning of relative motion between two bodies.
Suppose you are travelling with other passengers in a train that is moving at 40 km/hr. Have you noticed that you appear stationary to the other passengers? But if someone sees you from the outside of the train, your motion appears similar to the train velocity to them.
The theory of relative motion will help us understand why these motions appear different for different observers.

All motions have various appearances or perspectives as we view them from different frames. Hence, whenever two bodies interact, those different frames describe the motion between interacting bodies as a ‘relative motion’.
What is the Relative Motion Theory?
Motion is Relative mean?
When two moving bodies interact with each other, we can say their motion is relative. But the next question is ‘relative to whom?‘,
The motion of two moving bodies might look different for different observers based on which frames they observed. Therefore, their motion is relative to the observer’s frames.
Why is Motion Relative?
The relative motion is the estimation of the body’s motion concerning other moving or stationary objects. That means the motion is not determined with reference to the earth but with the other moving body on the earth.
To understand the motion of the moving body, we need to observe it from specific frames – to say the body’s motion is relative to that frame.
Let’s make it clear that while defining the ‘relative motion’, the three things we should consider:
- two interacting bodies
- motions of the bodies
- the frame of the observer
Absolute Motion Vs Relative Motion
| Absolute Motion | Relative Motion |
| When the motion of the body is observed from a fixed point, it is said to be absolute motion. | When there is no fixed point for observing the motion of the body, it is said to be relative motion. |
| The body’s position does not vary with time. | The body’s position does vary with time. |
Explain what is Absolute and Relative Motion with Examples?
Moving Vehicles:
Suppose you are standing at the side of the road and watching all other vehicles passing by. Here, you are the observer, and your position is not changing.
Hence, the motion of the vehicles is absolute as the observer’s position is not changing with time.
But when you travel in a car, you watch other vehicles passing by from the car window. You may have noticed the vehicles are moving slowly past you; even though you know their actual velocities, those are very high.
This occurs because of their relative motion between your car and other vehicles as the observer’s position changes with time.
Rest and Motion are relative terms explain with Examples
When the body’s position does not vary with time, we say the body is at ‘rest’. Whereas the body’s position does vary with time, we say the body is at ‘motion’.
The change in the body’s position depends on its surroundings. Or, to be more specific, the rest of the body and the state of motion is determined concerning its surroundings.
The Book on the Table:
The book’s position lying on the table is not changing with respect to the table itself. So here, we can say the book is at rest or stationary with respect to the table.
But when someone picked up the book and moved away from the table, the book’s position changed. So now we can say the book is in motion or moves with respect to the table.
Rest and motion are relative as the body at rest with respect to another body that might be in motion with respect to other body.
Person in a Hot Air Balloon:
To get more certainty on this statement, let’s take the case that you are standing on the ground and observing another person traveling in a hot air balloon.
Here, the person travelling in the balloon appears in motion with respect to you or the ground. But the same person is at rest with respect to the moving balloon.
How Rest and Motion relative?
An object’s position relates to its state of rest and motion as follows:
- If an observer is at rest or stationary to their reference frame, that observer must be in motion to another observer’s reference frame.
- If both observers are not at rest or stationary with respect to each other, they get different results.
- Based on the observer’s frame of reference or perspective, both rest and motion are related.
Relative Motion and Frame of Reference
The concept of the frame of reference was introduced to discuss the relative motion between two bodies. While defining moving body’s specific velocity, we refer to the velocity with respect to a particular frame or perspective as the frame of reference.
In the example of passengers on a train, we say that passengers are travelling in the train are moving relative to the earth. Hence, the frame of reference is the earth.
But when we consider the outer universe where the earth is spinning around the sun in its orbit, the frame of reference is the solar system.
What is Meaning of Frame of Reference?
The frame of reference or reference frames can be defined as
“A set of coordinate systems that illustrate the relative motion between two interacting bodies by measuring their position, velocity, and acceleration.”
- A set of three coordinates (x,y,z) specify the motion of a body in space.
- A set of three coordinates (x,y,z,t) specify the motion of a body in any event.

Depending on the motion of an object, the frame of references possess two groups as:
- Inertial Frame of Reference
- Non-Inertial Frames of Reference
Inertial Frame of Reference Vs Non-Inertial Frame of Reference
| Inertial Frame of Reference | Non-Inertial Frame of Reference |
| The frame of reference where the body remains rests or moves linearly with constant motion unless external forces act on it. | A frame of reference that moves in rotating or linear motion with other inertial frames of reference. |
| Newton’s laws of motion on an object are valid. | Newton’s laws of motion on an object are not valid. |
| The acceleration of the frame is zero. | The acceleration of the frame is non-zero. |
Relative Motion in 1D and 2D
Let’s take the relative motion examples that explains the concepts of relative motion in both one and two dimensions.
Direction of moving Car:
While travelling in the car, when you look out of the window, you see other vehicles running in the same direction and at equal motion alongside your bus. Since the relative motion between you concerning those vehicles is zero, you think that those vehicles are not moving. But when you see a motionless tree or light poles with respect to the ground, you perceive it is moving toward you.
These perceptions result from relative motion between two bodies problems, and you are the observer in this case.
Explain what is Relative Motion in One Dimension
Since the motion is one-dimensional, two bodies are moving straight in the same or opposite direction. We first introduce the relative motion between two interacting bodies in one dimension.
Relative Velocity in One Dimensional Motion
Let’s take the case of a man travelling on a train towards the West. We choose West as the position direction and the Earth as the frame of reference. So we address the moving train’s velocity with respect to the Earth as VTE, where Subscript TW points to the ‘Train to Earth’.
The man walks towards the East inside the train, which shows the man’s velocity as VMT relative to the moving train’s frame of reference. Note that the value of velocity VMT is negative as the man moves in the train’s opposite direction.
What is Relative Motion in One Dimension Formulas?
The relative motion formulas in one dimension can be obtained by adding the two velocity vectors. Therefore, the velocity of the man relative to the earth VME is given by,
 ……………..(1)
……………..(1)
What is Relative Motion Formula?
Mathematically, the relative motion formula between two interacting bodies is the vector difference between their velocities.
If V1 is the velocity of body 1 and V2 is the velocity of another body 2.
Relative velocity of Body 1 moving with respect to 2 is
V12 = V1 – V2 ………………….(A)
Similarly, Relative velocity of Body 2 moving with respect to 1 is
V21= V2 – V1 ……………….(B)
The relative velocity between interacting bodies 1 and 2 is the velocity of body 1 appearing to an observer on body 2 and vice versa.
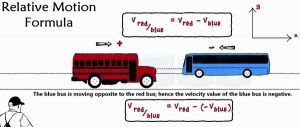
Explain what is Relative Motion in Two Dimensions
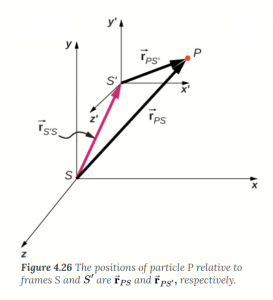
Let’s apply the concept of describing the motion of two interacting bodies in two dimensions. Consider a point P as a moving particle and S and S’ as two frames of references
What is Relative Motion Triangle?
The relative motion triangle is a triangular figure – which illustrates the relative motion between the bodies in two dimensions.
(Image Credit: Lumenlearning)
Derive the equation of Relative Motion for Two Body Problem
As per the relative motion triangle figure, the measured position of S’ frame with respect to S frame is ![]() , whereas the position of particle P with respect to S’ frame is
, whereas the position of particle P with respect to S’ frame is ![]() and with respect to S frame is
and with respect to S frame is ![]()
From the relative motion triangle figure, we get
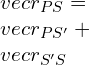
The velocities of the particle and reference frames are time derivatives of its position vectors, Therefore,

The above equation says that
The relative velocity between particle P and frame S is equal to the sum of the relative velocities between particle P and frame S’, and both frames S’ and S.
Let’s see how the accelerations of the particle P to two reference frames, S’ and S:

Here, we see that if the relative velocity between frame S’ and S is constant, ![]() . Therefore,
. Therefore,
![]()
Relative Motion Analysis
- The relative motion comprises all aspects of motions such as velocity, speed, or acceleration.
- To describe the body’s motion, the frames of reference need to be specified in terms of the body’s position, velocity, and acceleration.
- The relative motion of the body is observed from a particular frame of reference and varies with respect to the choice of frame of reference.
- When both frames of reference, S and S’, are moving relatively at a constant velocity, the accelerations of the bodies observed from both frames of reference are equal.
How are Position Time and Motion related?
The position-time graph shows the relation between motion and position of the body by illustrating how much it moves from one position to another in a given time.
The slope of the position-time graph then calculates the body’s motion.
Position-Time Graph
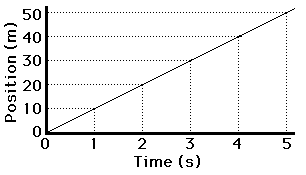
The average velocity of the moving body is equal to the change in its position per corresponding change in time.
Here, a change in the position is represented by Δs, and Δt represents a change in time.
Therefore,
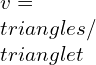 …………………(2)
…………………(2)
From the average velocity formula, we can derive the various equations of motion.
First Equation of Motion Derivation gives Relation between
Let’s we derive the first equation of motion which gives the relation between velocity and time.
The acceleration on the moving body is equal to the change in its velocity per corresponding change in time.
 …………………(3)
…………………(3)
Let’s expand Δv to v-v0 and condense Δt to t.
Where v0 is the body’s initial velocity, and v is the body’s final velocity.
![]() ……….. (4)
……….. (4)
Let’s solve the above equation for v as a function of t.
![]() ………..(5)
………..(5)
The equation (5) is known as the first equation of motion in terms of velocity-time relation.
Second Equation of Motion Derivation gives Relation between
Let’s we derive the second equation of motion which gives the relation between position and time.
Expand Δs to s-s0 and condense Δt to t.
Hence, the equation (2) becomes,
![]() ………..(6)
………..(6)
Solving above equation in terms of position, we get
![]() ………..(7)
………..(7)
As per the Merton Rule,
“When the rate of change of any physical quantity is constant, the average value of that physical quantity is half of its initial and final values.”
![]() ………..(8)
………..(8)
Substitute the equation of motion (5) into the above equation (8) and simplify by eliminating v, we get
![]() which gives,
which gives,
![]() ………..(9)
………..(9)
Substituting equation (9) into equation (7) to eliminate v,
![]()
Finally,
![]() ……… (10)
……… (10)
Write Equation of Motion in terms of Position Time Relation
The change in the position (s-s0) is called the displacement Δs.
Hence, the equation (10) becomes,
![]() ……….. (11)
……….. (11)
The equation (11) is known as the second equation of motion in terms of position-time relation.
How are Linear Motion and Angular Motion related?
Linear Motion Vs Angular Motion
| Linear Motion | Angular Motion |
| It is a translational motion of the body in a straight path from one position to another. | It is a rotational motion of the body about an axis of the centre in a circular direction. |
| Unit is meters per second. | Unit is radian per second. |
| Linear displacement denoted as ‘s’ | Angular displacement denoted as ‘θ’ |
| Linear Velocity is denoted as “v” | Angular Velocity denoted as “w” |
| Linear Acceleration denoted as “a” | Angular Acceleration denoted as “α” |
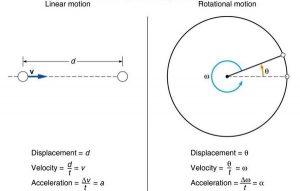
(Image Credit: Mc graw hill)
Relationship between Linear and Angular Motion
We can substitute the angular quantities into linear motion formulas to obtain angular motion formulas.
The equation (2) can be rewritten as,
 …………….(12)
…………….(12)
Multiplying both sides by radius r we get,
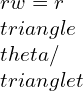
The term rΔθ represents the total distance (Δs= s-s0) travelled by the body moving in a circle of radius r.
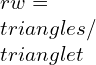
Notice the right-hand side equation is the formula of linear velocity (v).
Hence, the relation between linear velocity and angular velocity can be written as,
![]()
How Uniform Circular Motion and Simple Harmonic Motion are related?
Uniform Circular Motion Meaning
- When the body revolves or spins at a constant motion along a circular path, it is said that the body is in ‘Uniform Circular Motion (UCM)’.
- When the body defines a circular motion, its direction is continually changing, and the total distance covered by the body from the centre of the axis remains fixed at all times.
Simple Harmonic Motion Meaning
- It is a special kind of periodic motion of the body in which it moves to and fro repeatedly with varying motions about mean position.
- A restoring force acting on the body responsible for its to and fro periodic motion.
Relation between Simple Harmonic Motion(SHM) and Uniform Circular Motion (UCM)
Let’s demonstrate a simple method which relates Uniform Circular Motion to Simple Harmonic Motion.

The figure shows that a ball is attached to a vertical turntable rotating in a circular direction with angular velocity w. Since the light source illuminates from the top, the shadows of the ball are projected onto the floor.
When the ball moves in the upper part of a turntable, its projections start moving towards the left. When the ball moves in the lower part of a turntable, its projections start moving towards the right.
Hence, the ball is swinging with velocity v from left to right and again from right to left from position x shows its to-and-fro motion, which is called simple harmonic motion.
The position of the ball when it performs simple harmonic motion:
![]() ………………(*)
………………(*)
Where A is amplitude and θ is the Angular displacement of the ball.
As per equation (12), ![]()
The above equation becomes,
![]()
In Simple Harmonic motion, angular velocity w is 2π radians per unit one revolution time.
That is, ![]()
Substituting value of w, we get
![]() ………………(13)
………………(13)
How are Frequency and Period related in Simple Harmonic Motion?
The frequency and period related in the simple harmonic motions as follows:
As the simple harmonic motion is a repeating oscillation,
- The total amount of time required for performing one oscillation is called the period T of SHM.
- This number of oscillations in unit time is the simple harmonic motion’s frequency (f).
![]()
Therefore, the equation (13) becomes,
![]()
The above equation is the same as the equation of SHM.
The simple harmonic motion(SHM) is the projection of UCM into one direction.
How is Inertia related to Motion?
Inertia is the object’s natural tendency to resist any changes in their velocity.
How is Inertia related to Newton’s First Law of Motion?
Even though Newton’s laws of motion fit inertial frames of reference, Newton never clearly defined the theories of inertial frames. But the inertial reference frames are a natural consequence of Newton’s first law of motion due to net external force.
Newton First Law of Motion
“An object remains at rest or moves at a constant motion unless there is a net force acting on it.”
Mathematically, F = ma
The inertia of an object depends on its mass; it must be overcome by a net external force (mg) acting on the object to change its velocity to accelerate the object. The larger the object’s mass, the more significant net external force is required to move that object.
The concept of inertia leads to the idea of the inertial frame of reference in terms of relative motion between two moving objects.
Newton’s first law of motion that explains the relation of an object’s inertia to its motion; also known as the ‘Law of Inertia’.

How Newton’s Second Law of Motion relates Force and Acceleration?
Newton’s Second Law of Motion
“A net force acting on any object to change its momentum over time”.
Derive the Mathematical Relation of Newton’s Second Law of Motion
Mathematically newton’s second law of motion can be written as,
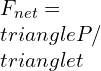 ………(14)
………(14)
Where, ΔP is change in momentum of the body = P-P0
P0 is initial momentum at initial time t0 and P is final momentum at final time t of the body
Formula of momentum is P= mv
Solving the equation (14),
![]()
Substituting formula of momentum,
![]()
![]()
![Rendered by QuickLaTeX.com F_{net} = m[\\triangle v/ \\triangle t]](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-822f93588a27fdde7fbd8be7c2f5f116_l3.png)
Where ![]() is acceleration ’a’ of the body
is acceleration ’a’ of the body
Therefore, ![]() can be written in terms of acceleration as,
can be written in terms of acceleration as,
![]() …….(15)
…….(15)
Newton’s Second Law of Motion relates Object’s Acceleration to
Equation (15) also Newton’s second law of motion which relates force with acceleration as follow:
“The acceleration of an object is directly proportional to its net force applied to the object and inversely proportional to its mass.”
Newton’s second law of motion say that when the net external force acts on the object, it causes a change in its velocity. This velocity change with time is known as the object is accelerated. Acceleration refers to slowing down or speeding up the object and changing the direction of motion.
To accelerate the object from rest to some velocity, you need a net external force. The net external force is a sum of all forces acting on the body in each particular direction.
However, suppose the object is already in motion. In that case, if we observed such a situation from a moving inertial frame of reference, the object changes its motions or directions based on the directions of net applied force, and the directions of that object and frame of reference are moves relative to each other.
Therefore, Newton’s second law of motion is also known as the “Law of Force”.

Relation Between First and Second Law of Motion
Newton’s first law of motion, also recognized as the Law of Inertia, discovers that any object possesses a particular mass to oppose or resist the change in its motion.
Hence, any object with large inertia makes it hard to move, or once they are moving, they are difficult to stop. So the object’s inertia is a significant factor in determining the force that can accelerate that object at a given rate.
Newton’s second law of motion in term of mass is,
“The mass of an object is directly proportional to its applied force, and inversely proportional to its acceleration”.
Hence, the more massive the moving object is, the more net force it requires to move and the smaller acceleration produced on the massive object.
Motion of Cricket Ball and Football:
Let’s take the case of cricket ball and football. The cricketing ball has a larger mass inside compared to the football. Hence, when you kick both a football and cricket ball, the football will move more than the cricketing ball.
That’s how Newton’s second law of motion also called ‘Law of Force’ directly relates to the Law of Inertia, Newton’s first law of motion.
Discovery of Gravity
One of the famous events in history that leads to discovering the first force known as “gravity”.
A young newton was resting at the apple tree base. One apple fell on his head, and he realized what would be a mysterious thing is responsible for the apple to fall into the ground.

From observing the circular motion of the moon around the earth, Newton figured out that some natural force is responsible for making an object falling towards the ground. That’s leading to the discovery of the gravity force, which changes the way we understand the universe. The analysis of the force of gravity was rooted to understand the relationship between motion and force. Then, he also discovered various types of forces existing in the universe that cause an object’s motion. Therefore, the measuring unit of force is also called ‘Newton‘.
Read more about the Units of Forces
Relate Force and Motion
- A force is defined as “either a push or pull to the object which causes the change in its motion.”
- A motion is defined as “the change in the position of the object in a given time when a force is applied.”
From both definitions, it is clear that force affects the state of motion of any object.
Explain how Forces related to Motion
Sir Isaac Newton gave us the best description of links between force and motion through its laws of motion. It gives you a clear picture of what happens when any force is applied to the object having mass.
Combining statements of both Newton’s first and second law of motion, we understand that,
“An unbalanced force requires accelerating the object by changing its motion, and this amount of acceleration of the object is directly proportional to an unbalanced force and inversely proportional to the object’s mass.”
How are Force and Motion Related?
From newton’s laws of motion, the following conclusions show the force and motion are related to each other:
- When a net force applies to the stationary object in a similar direction, it accelerates the object.
- When a net force applies to the moving object in the opposite direction, it deaccelerates the object.
- When a net force applies to the moving object at a different angle with respect to the direction of its motion, it changes the direction of an object.

Read more about the Types of Forces
Explain How Balanced and Unbalanced Forces are related to Motion
If two forces are acting on one object, one pushes the object to the left and the other is to the right. The object will only move in such a case when one of the forces is stronger than the other.
- If both forces have different strengths, then they are said to be ‘unbalanced forces‘ that cause the change in the object’s motion.
- If both forces have the same strength, they are said to be the ‘balanced forces‘ that does not cause the change in the object’s motion.
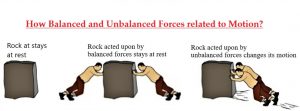
How do Forces and Motion relate to our lives?
When we recall any motion, we often think of kids running, vehicles moving, airplanes flying, etc. But actually, the motion is much more than this. Since the various types of natural forces are always acting on each object in the universe, they are all the time in motion.
The force and motion impact many things that we do by making things move and stay still. The primary example is kicking the ball, which is force and that causes the ball to fly in the air, which is motion. That is why, to do any activities, force and motion are essential things that we need daily.
How are Rockets related to Newton’s Law of Motion?
Like all other moving bodies, the motion of the rocket is directed by newton’s laws of motion.
How are Rockets related to Newton’s 1st Law of Motion?
Newton’s 1st law of motion explains how the body remains stationary or moves with constant motion except there is no force acting upon it.
Similarly, the rockets stay stationary until any external forces are applied to eject them. Then, once it is projected into space, it moves with its constant velocity until a more force like thrust is applied.
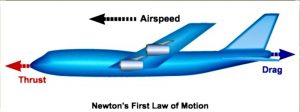
How are Rockets related to Newton’s 2nd Law of Motion?
Newton’s 2nd law of motion is used to understand that the larger mass any object has, the more significant force is required to accelerate the object.
Thus, implying newton’s 2nd law of motion rule into rocket working, the giant rocket will have required more vital force to expedite the rockets. Usually, the rocket needed fuel of around seven pounds for each payload they carry.
Rocket Scientists use newton’s 1st and 2nd laws of motion to calculate the thrust(force), which required accelerating the rocket into a planned trajectory.
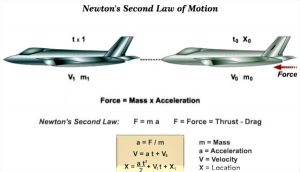
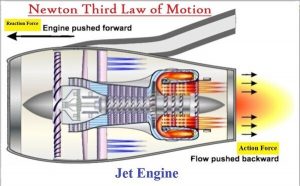
How are Rockets related to Newton’s 3rd Law of Motion?
Newton’s Third Law of Motion
“To every action on an object, there is an equal and opposite reaction.”
A pair of forces will act on two interacting objects if one exerts a force on another, and in return, another exerts an equal but opposite force first. These equal and opposite pair forces on objects mean that both forces have magnitude but are in opposite directions.
In a rocket engine, the principle of action or reaction is important to projection:
- The burning of fuel at high temperature and high pressure produced a hot exhaust gas which is supposed to be the first force to the rocket. This hot gas flows through the rocket and finally accelerates the rocket.
- In reaction, a thrust is produced in the engine, which is supposed to be the second force that accelerates the rocket as per newton’s 2nd law of motion.
- As per newton’s third law states, the action is hot exhaust gas, and the reaction is thrust required to accelerate the rocket.
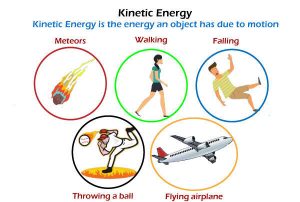
How are Energy and Motion Related?
There are various energies that exist in different forms in the universe, as the motion of an object is the energy stored in that moving object.
One form of energy is kinetic energy – correlated with an object’s motion; and another is potential energy – correlated with an object’s position.
Kinetic Energy relation with Motion
- If work is done on any object by applying a net external force, it transfers energy which causes the increase in its motion and finally, it gains more kinetic energy.
- The motion of the molecules depends on how they interact less or more strongly with each other. This process leads to the foundation of the object’s kinetic energy.
- Kinetic energy is stored in an object for all motions such as linear, rotation, vibration, translation, or any combination of motions.
Kinetic Energy Formula
The kinetic energy of the object depends on its motion as well as its mass.
The formula for kinetic energy is given by,
![]()
This formula is valid only for low to relatively high velocities. When the object’s velocity approaches the velocity of light c = 3 x 108 m/s, the theory of relativity comes into the picture.
The velocity has both positive or negative values, but the squared velocity is always positive. Hence, the kinetic energy is always either zero or positive.
How is Temperature related to the Motions of Molecules?
To learn about the nature of temperature associated with the motion of molecules, first, it is important to acknowledge that matter consists of various tiny particles that can be atoms or molecules, or both.
When the random motion between particles is slow, the particles form solids. When a force applies to solids, the particles move faster and then slide over one another, forming a liquid. When both atoms and molecules move fast due to another force, they disconnected from each other and formed a gas. Hence, the state of matter, such as solid, liquid, and gas, depends on the motion of the particles.

Here, the temperature is an external force that changes the motion to change the state of matter. Therefore, the more temperature is provided to the particles, the matter gets warmer, and then its particles move faster. That is how the temperature is related to the random motions of the particles at a molecular level.
How are Temperature and Molecular Motion related?
The energy of the particles within the matter does not have the same energy since it is constantly changing due to change in motion as the particles undergo the transition from different states of matter.
In gas, the motion of the molecules is along a straight path called molecular motion. Whereas in solids and liquids, the particle’s motion is more restrained, and they only have potential energy, which leads to complications in energy measurements.
Hence, the temperature is closely related to the average kinetic energy of molecules that shows molecular motion. So, for example, the warmth we feel when we touch any hot surface is the kinetic energy transferred by gas molecules on the solid or liquid material we touch.
The kinetic energy of all moving molecules is proportional to their molecular motion.
Therefore, as the motion of colliding molecules increases, the total kinetic energy is also increased. Since it is difficult to measure the molecular motion of each gas molecule instead, the temperature can measure the average kinetic energy of all gas molecules.
The Mathematical Relation between Temperature and Average Kinetic Energy

T is the gas temperature, R is the universal gas constant, NA is Avogadro’s number.
Since, the term \frac{R}{N_{A}} is also known as Boltzmann Constant KB.
![]()
The average kinetic energy resulting from the molecular motion of molecules is directly proportional to their temperature.
How are Temperature Thermal Energy and Particle Motion related?
The total energy of the substance is the total energy of all particles like atoms and molecules, and it depends on the number of particles, temperature and physical state.
Though the temperature only measures the average kinetic energy of molecules, the thermal energy of the substance measures the total energy of the particles within the substance. Thus, thermal energy includes both potential energy and kinetic energy. For greater the motion of the particles, the greater the temperature of the substance, so it’s thermal energy. Therefore, the thermal energy is higher in gas because of molecular motion, followed by liquid, then solid.
How is Heat Energy related to the Motion of Particles?
The concept of temperature related to motion is now familiar to you, but you may confuse temperature word with heat. Note that the temperature measures how hot or cold any object is concerning another object. In contrast, heat transports the energy from one body to another as both bodies have different temperatures.
The first law of Thermodynamics says that “the loss or gain of thermal energy is proportional to the amount of heat transferred when the heat is flowing in or out of substances”. Hence, the measurement of the temperature changes of one object in contact with another; used to determine the amount of heat energy transferred between them.

Relative Motion Problems
If two bodies M and N, are moving with the same velocity of 50 km/hr in the opposite directions, find the relative velocity of body M with respect to body N and relative velocity of body N with respect to body M.
Solution:
Given:
VM is the velocity of body M = 50 km/hr
VN is velocity of another body N that moving in the opposite direction = -50 km/hr
Formula:
Relative velocity of Body 1 moving with respect to 2 is
V12 = V1 – V2
Relative velocity of Body 2 moving with respect to 1 is
V21= V2 – V1
Calculation:
Relative velocity of Body M moving with respect to N is
VMN = VM – VN = 50 – (-50) = 100 km/hr
Relative velocity of Body N moving with respect to M is
VNM = VM – VN = (-50) – 50 = -100 km/hr
If two objects move at the same motion in opposite directions, then their relative motion is equal in magnitude but opposite sign due to opposite direction.
If two bodies M and N, are moving with the same velocity of 50 km/hr in the same directions, find the relative velocity of body M with respect to body N and relative velocity of body N with respect to body M.
Solution:
Given:
VM is the velocity of body M = 50 km/hr
VN is velocity of another body N that moving in the same direction = 50 km/hr
Formula:
Relative velocity of Body 1 moving with respect to 2 is
V12 = V1 – V2
Relative velocity of Body 2 moving with respect to 1 is
V21= V2 – V1
Calculation:
Relative velocity of Body M moving with respect to N is
VMN = VM – VN = 50 – 50 = 0
Relative velocity of Body N moving with respect to M is
VNM = VM – VN = 50 – 50 = 0
If two objects move at the same motion in exact directions, the relative motion between them is zero.
The passengers are travelling in the airplane flying at 250 m/s to the west with respect to air. The velocity of air is 35 m/s flowing to the south with respect to the ground. What is the velocity of the airplane and its angle with respect to the ground?
Solution:
Given:
The airplane’s velocity relative to the air VPA = 250 m/s
The air velocity relative to the ground VAG = 35 m/s
Formula:
The airplane’s velocity relative to the ground VPG is equal to the sum of the airplane’s velocity relative to the air VPA and air velocity relative to the ground VAG.
Mathematically it is written as,
VPG = VPA + VAG
Calculation:
Since the airplane is moving to the west and air is flowing to the south, airplane and air directions are perpendicular.
In such a case, let’s draw a relative motion triangle diagram to solve this problem.

As per the relative motion triangle diagram, the magnitude of the velocity of the airplane with respect to the ground can be obtained using the Pythagorean Theorem:
![]()
![]()
![]()
![]()
To find angle of airplane with respect to ground, we use basic trigonometric functions,
From the relative motion triangle,
tan θ = triangle opposite side / triangle adjacent side
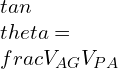
![Rendered by QuickLaTeX.com \\theta = tan^{-1} [\\frac{V_{AG}}{V_{PA}}]](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-2aa2b7b8ad4cc662e340fa55c0d26ae8_l3.png)
![Rendered by QuickLaTeX.com \\theta = tan^{-1} [\\frac{35}{250}]](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-fe2837c999ea2ec7b8fa14787049f9a5_l3.png)
![]()
The airplane is flying at an angle of 80 with a velocity of 252 m/s concerning the ground.
Relative Motion Exercises
A bike running on the highway at a velocity of 80 km/h passes a truck travelling at a speed of 60 km/h. What is the velocity of the bike with respect to the truck driver’s point of view?
Ans: 30 km/h
A bus travels at 50 m/s to the east and a passenger in the bus walks towards the west at 5 m/s. What is the velocity of a passenger concerned to the ground?
Ans: -45 km/h
A car ‘M’ is running with a velocity of 40 m/s to the north. Also, Car ‘N’ runs to the south with a velocity of 60 m/s beside car ‘M’.
1) If the car ‘N’ is running in the opposite direction to the car ‘M’, calculate relative velocity to the car ‘M’ with respect to car ‘N’.
2) Suppose both cars were running to the north. i.e. in the same direction, then calculate relative velocity to the car ‘M’ with respect to car ‘N’.
Ans: 100 m/s and -20 m/s
FREQUENTLY ASKED QUESTIONS
How can we say that body is relative motion or not?
Ans: The relative motion is the estimation of the body’s motion concerning other moving or stationary objects.
Therefore, when the body is moving with respect to another body that is moving or at rest, the body’s motion is said to be relation motion with respect to another body.
Two objects are in relative motion. Is it possible or not for one of them to have real motion?
Ans: All the motion is relative. When both objects are in relative motion, that means they are moving relative to each other.
Hence, there is no such motion called ‘real motion’.
What is the difference between relative motion and absolute motion?
Ans: The difference between relative motion and absolute motion is,
The position of any object does not change with time in absolute motion, and it changes with time in the relative motion.
What is the relative motion of the galaxy in the universe?
Ans: As per Hubble’s law, the galaxies in the universe are flying away from each other at speeds proportional to the distance between them.
Hence, the greater the distance between two flying galaxies, the greater the relative motion between them.
What two measurements will two observers in relative motion always agree on?
Ans: Both observers always agree on the following two measurements:
- The space-time interval: It is the straight line’s length between two situations in space and time.
- The speed of light: Itis the maximum velocity at which all matter and its energy in the universe can move.
Also Read:
