Let us discuss some detailed facts about a super elastic collision, how and where does it occurs, some examples, and detailed facts.
Super elastic collisions are those in which the colliding particle does not lose its kinetic energy, instead gains some kinetic energy from the particle it is colliding with and accelerates at a faster rate after the collision.
What is a super elastic collision
The collision is said to be elastic when the momentum and the kinetic energy of the object after the collision are conserved. There may be loss or gain of energy during the collision of the objects.
A collision in which there is no loss of energy instead the object gains an additional amount of energy then the collision is said to be a super elastic collision. This auxiliary supply of kinetic energy may be the result of the conversion of the potential energy of the object into kinetic energy.
Where does super elastic collision occur
Most of the collisions in nature are inelastic collisions where the kinetic energy of the colliding object is converted into some other form of energy.
Well, a super elastic collision occurs mostly in explosive reactions like nuclear fissions, reactors, supernovas, explosions, etc that create critical impact. This is a result due to a gain of the additional amount of kinetic energy without any loss of energy. On collision, subsequently, an object receives the energy from the object it is colliding with, which excels the kinetic energy of the object.
Super elastic collision formula
Consider two molecules of mass m1 and m2. A molecule of mass m1 is approaching from infinity with velocity u1 and collides with mass m2 moving at velocity u2. After a collision, both the masses diverts away from each other making an angle with a plane with velocities v1 and v2.
In an elastic collision, the momentum of the particles before and after a collision is conserved, hence given by the relation
m1u1+m2u2=m1v1+m2v2
Where m1, m2 are masses of particle 1 & 2 respectively
u1, u2 are initial velocities of both the particle before colliding, and
v1, v2 are final velocities of the particles after collision.
The momentum of the colliding molecule after a collision will be greater than the momentum of the molecule before the collision.
m1u1<m1v1
Which implies that u1<v1
And the kinetic energy of the particle in the collision is
1/2 m1u12+1/2 m2u22=1/2 m1v12+1/2 m2v22
Since u1<v1, the kinetic energy of the colliding molecule after colliding will be increased.
1/2 m1u12<1/2 m1v12
This means the energy associated with molecule 2 will be reduced as it will transfer its potential energy to molecule 1 that will convert into kinetic energy.
Super elastic collision example
Let us discuss some examples of super elastic collision to understand the term better.
Nuclear fission
Fission is the process of splitting a reactant into two or more products. A nucleus of the atom splits into two or more nuclei when a highly energetic photon collides with the nuclei.
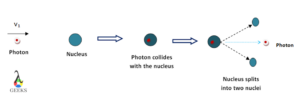
A photon approaching from infinity carries kinetic energy with it, on bombarding with the nucleus it releases its energy to the nucleus due to which the nucleus becomes unstable. This results in a splitting of the nucleus into two daughter nuclei releasing out the photon.
The mass of the nucleus reduces to half and the potential energy of the nucleus is converted into kinetic energy and hence the final kinetic energy given out in a process after the collision is high. This technique is used in nuclear weapons, in nuclear reactors to produce huge energy.
Shape memory alloys
The shape memory alloys are super elastic materials manufactured at a specific temperature. The alloy is molded into a particular shape while heating, maintaining a certain temperature, and quickly cooling it down. This shape is memorized by the alloy.
An object changes its shape when an external load is imposed on it but regains its shape once the load is removed and exposed to the same temperature at which it was formed. This superelasticity is a reversible process.
Mostly, Copper-Aluminium-Nickel and Nickel-Titanium alloys are used as a shape memory alloy. Nickel-titanium is one such shape memory alloy used in manufacturing orthodontic wires.
Uranium bomb
Uranium-235 is a highly radioactive atom and gives out a large amount of energy during its fission, that is why it is mostly used in reactors and explosives.
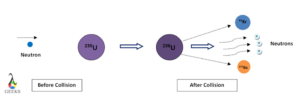
This is similar to nuclear fission, the neutron when collides with the uranium-235 atom, the kinetic energy of the neutron is transferred onto a uranium atom and becomes unstable due to an extra neutron availability. This neutron recoils along with the atom.
The highly unstable atom splits up into two daughter nuclei shown in the above diagram, releasing three free nuclei which then react with another atom of uranium for fission. This reaction gives out an enormous amount of energy and heat in the surrounding, thus it is an exothermic reaction.
Spring
The spring when compressed, stores the potential energy in it. On releasing the pressure from the string, it gives out a large amount of potential energy in the form of kinetic energy.
Read more on spring potential energy.
Comet approaching sun
The sun has the highest gravitational attraction force in a solar nebula and hence most of the comets approaching from the far nebula reach around the Sun. They gain enough potential energy through radiations emitted by the Sun and deflect in a parabolic pathway. The kinetic energy of the comet after deflection is far greater than its kinetic energy while approaching the Sun.
Is impulse conserved in an elastic collision
Impulse is defined as a force stimulated on the object in a definite time interval and given by the formula
I=FΔ t
Where I is the impulse
F is a force
Δ t is a change in time.
Impulse is also equal to the change in the momentum of the object.
I=ΔP
Hence, ΔP=F Δ t
In an elastic collision, the change in momentum of the object is equal to the difference between the momentum of the object before and after the collision.
ΔP=m[Vf-Vi]
Where m is a mass of the colliding object.
Vf is the final velocity of the object
Vi is the initial velocity of the object
Therefore,
F Δ t= m[Vf-Vi]
The impulse on the object in a collision can be found out by finding the difference between the velocities of the object before and after colliding.
It is obvious that there is an impulse on the collision on both the objects, but due to the opposite force of reaction the impulse is reduced and canceled out. In most cases, there is a slight change in the momentum of the object.
How do you solve a perfectly elastic collision
In a perfectly elastic collision, there is no loss of the kinetic energy of the object after the collision. The momentum and the kinetic energy of the object in a perfectly elastic collision are conserved.
Consider a particle of mass m1 accelerating at a velocity u1 strikes the particle of mass m2 moving with velocity u2, then the momentum of particle 1 is m1 u1 and that of particle 2 is m2u2. Particle 1 approaches particle 2 and collides with it creating a net impact zero and both particles 1 & 2 gains velocity v1 and v2 respectively and divert in two different directions.
Since the momentum of the particles is conserved before and after collision
m1u1+m2u2= m1v1+ m2v2
There is no loss of kinetic energies of the particles, hence the kinetic energy before and after collision remains unchanged.
1/2 m1u1+1/2 m2u2=1/2 m1v1+1/2 m2v2
m1(u1-v1)=m2(v2-u2)
m1/m2=v2-u2/u1-v1
Read more on 8+ Perfectly Elastic Collision Examples: Detailed Facts And FAQs.
Frequently Asked Questions
Q1. An object A of mass 5 kg collides with object B at rest at a speed of 3m/s. After colliding both the objects move at a speed of 0.8m/s. What is the mass of object B? What is the impulse on the object due to collision?
Given:m1=5kg
m2=?
u1=3m/s
u2=0
v1=v2=0.8m/s
Since, the momentum is conserved in the collision
m1u1+m2u2=m1v1+m2v2
5* 3+m2*0=5*0.8+m2*0.8
15+0=4+m2*0.8
11=m2*0.8
m2=11/0.8=13.75kg
The mass of object 2 is 13.75 kg.
The total momentum of the object before the collision is
Pinitial=m1u1+m2u2=5*3+13.75*0=15
Pfinal=m1v1+m2v2 = 5*0.8 + 13.75 * 0.8 = 4+11 = 15
The impulse on the object due to collision is
I = ΔP = Pfinal – Pinitial = 15-15 = 0
Hence, there is no impulse conserved in the collision.
What is the impulse due to collision?
An impulse is the duration of the force applied on the particles while colliding.
It is also defined as the change in momentum of the objects before and after a collision and is equal to the force imposed by the object for finite time duration.
How does the impulse defer in a perfectly elastic collision and super elastic collision?
The momentum of the object is conserved hence the impulse becomes zero in a perfectly elastic collision.
In super elastic collision, the momentum of the object increases after colliding as the kinetic energy excels, therefore the impulse is positive.
Also Read:
Hi, I’m Akshita Mapari. I have done M.Sc. in Physics. I have worked on projects like Numerical modeling of winds and waves during cyclone, Physics of toys and mechanized thrill machines in amusement park based on Classical Mechanics. I have pursued a course on Arduino and have accomplished some mini projects on Arduino UNO. I always like to explore new zones in the field of science. I personally believe that learning is more enthusiastic when learnt with creativity. Apart from this, I like to read, travel, strumming on guitar, identifying rocks and strata, photography and playing chess.