The Definitive Guide to Steel Density: A Comprehensive Exploration

Steel is a ubiquitous material in modern construction, engineering, and manufacturing, and its density is a critical property that plays a crucial role in various applications. In this comprehensive guide, we will delve into the intricacies of steel density, exploring its measurement, factors that influence it, and its practical implications.

Understanding Steel Density

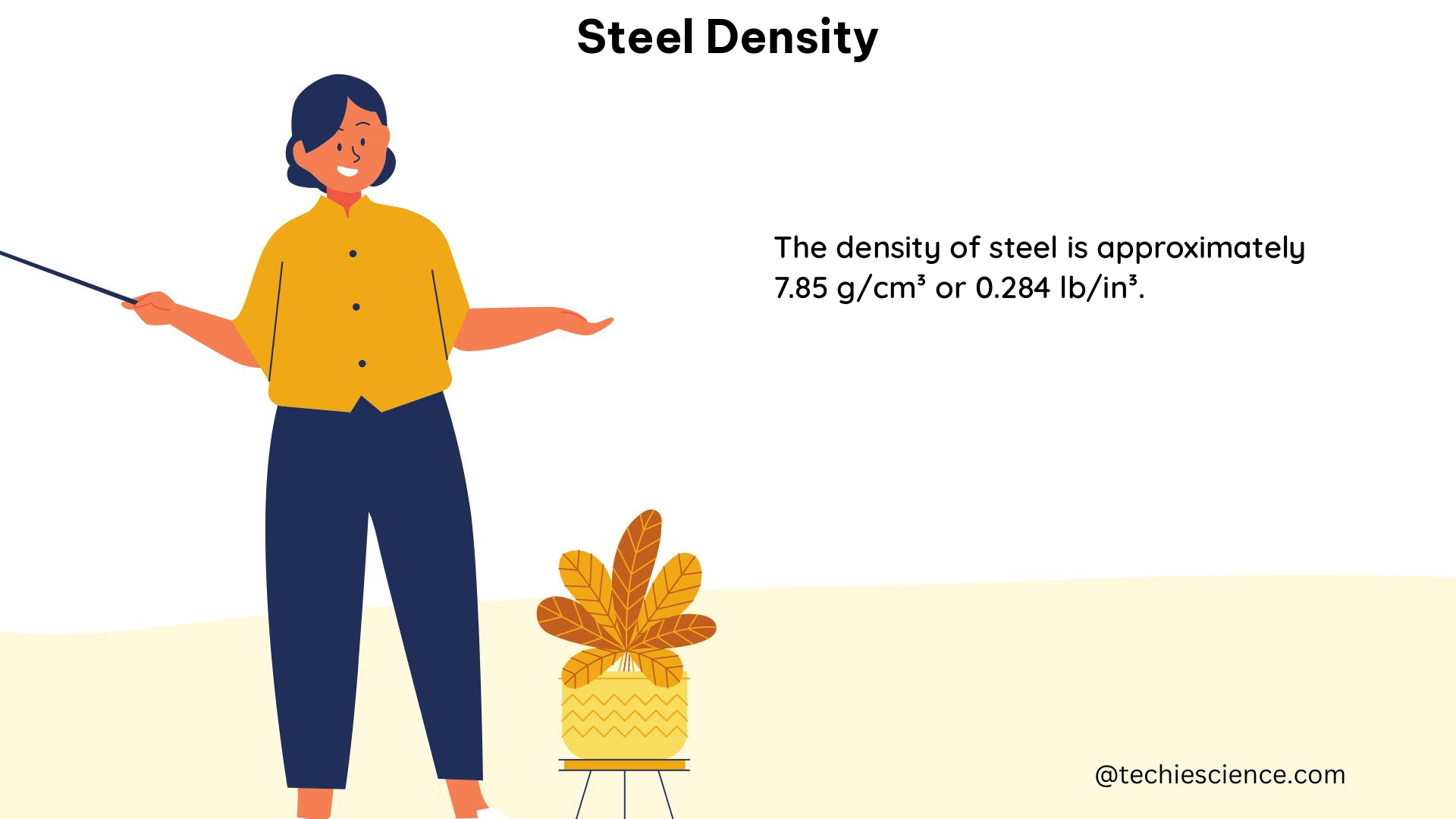
Steel density, typically around 490 lb/ft³ (pounds per cubic foot) or 7.85 g/cm³ (grams per cubic centimeter), is a measure of the mass of steel per unit volume. This makes steel one of the most dense common metals, surpassing the density of other widely used materials like aluminum (2.70 g/cm³) and copper (8.96 g/cm³).

The density of steel is an intensive property, meaning that it does not change regardless of the amount of material present. This property is crucial in various applications, as it allows for accurate calculations and predictions of the weight and strength of steel structures, components, and products.

Factors Influencing Steel Density

The density of steel can be influenced by several factors, including:

1. Alloy Composition: The addition of alloying elements, such as carbon, chromium, and nickel, can alter the density of steel. For example, the density of carbon steel (0.1-2.1% carbon) ranges from 7.85 to 7.87 g/cm³, while the density of stainless steel (containing chromium and other alloying elements) can range from 7.75 to 8.06 g/cm³.

2. Heat Treatment: The heat treatment process, which involves controlled heating and cooling of steel, can also affect its density. Different heat treatment methods, such as quenching and tempering, can result in slight variations in steel density.

3. Impurities and Defects: The presence of impurities or defects in the steel, such as voids or inclusions, can slightly alter its density. Careful manufacturing processes and quality control measures are essential to maintain consistent steel density.

Measuring Steel Density

Accurate measurement of steel density is crucial for various applications, and several methods have been developed to achieve this:

1. Hydrostatic Weighing: This method involves submerging a sample of steel in a liquid, typically water, and measuring its buoyant force. By applying the principles of Archimedes’ principle, the density of the steel can be calculated using the formula:

ρ = m / V = m / (m_air - m_water / ρ_water)
where ρ is the density of the steel, m is the mass of the steel, V is the volume of the steel, m_air is the mass of the steel in air, m_water is the mass of the steel in water, and ρ_water is the density of the water.

1. Pycnometry: This method involves measuring the volume of a known mass of steel using a pycnometer, a device designed to accurately measure the volume of small samples. The density is then calculated by dividing the mass by the measured volume.

2. Displacement Method: In this method, the volume of a steel sample is determined by measuring the amount of liquid it displaces when submerged. The density is then calculated by dividing the mass of the sample by the measured volume.

3. Analytical Calculations: In some cases, the density of steel can be estimated based on its chemical composition and the densities of its constituent elements. This approach is particularly useful when physical measurements are not feasible or practical.

Practical Applications of Steel Density

The density of steel is a critical property that has numerous practical applications across various industries:

1. Structural Design: In construction and civil engineering, the density of steel is used to calculate the weight of structural components, such as beams, columns, and trusses, which is essential for designing safe and efficient buildings and infrastructure.

2. Transportation and Logistics: The density of steel is crucial in the transportation and logistics industry, as it determines the weight and load-bearing capacity of steel components used in vehicles, ships, and other modes of transportation.

3. Manufacturing and Machining: The density of steel affects the performance of machining processes, such as cutting and drilling, as well as the design of tools and equipment used in manufacturing.

4. Material Selection: The density of steel, along with other properties like strength and corrosion resistance, is a key factor in material selection for various applications, such as the production of consumer goods, industrial equipment, and aerospace components.

5. Quality Control and Certification: Accurate measurement of steel density is essential for quality control and certification processes, ensuring that the material meets industry standards and specifications.

Advanced Concepts in Steel Density

To further enhance your understanding of steel density, let’s explore some advanced concepts and their practical implications.

Density Variations in Steel Grades

Different grades of steel, characterized by their chemical composition and manufacturing processes, can exhibit slight variations in density. For example, the density of low-carbon steel (0.05-0.25% carbon) ranges from 7.85 to 7.86 g/cm³, while the density of high-carbon steel (0.60-1.50% carbon) can be slightly higher, ranging from 7.86 to 7.87 g/cm³.

These density variations are important considerations in applications where precise weight calculations are required, such as in the design of load-bearing structures or the optimization of transportation and logistics.

Density Measurement Techniques

In addition to the methods mentioned earlier, more advanced techniques have been developed to measure the density of steel with high accuracy and precision:

1. X-ray Densitometry: This technique uses X-ray absorption measurements to determine the density of steel samples. It is particularly useful for analyzing the density of thin or coated steel products, where traditional methods may be less effective.

2. Gamma-ray Densitometry: Similar to X-ray densitometry, this method uses gamma-ray absorption to measure the density of steel. It is often employed in industrial settings, such as steel mills, to monitor the density of steel products in real-time.

3. Ultrasonic Densitometry: This technique utilizes the propagation of ultrasonic waves through a steel sample to determine its density. It is a non-destructive method that can be used to measure the density of steel components without the need for physical sampling.

These advanced measurement techniques are particularly useful in specialized applications, such as the production of high-performance steel alloys or the quality control of critical steel components in the aerospace and defense industries.

Density-Related Calculations and Formulas

To fully understand the implications of steel density, it is essential to be familiar with the relevant calculations and formulas:

1. Mass-Volume Relationship: The fundamental formula for density is:

ρ = m / V
where ρ is the density, m is the mass, and V is the volume of the steel sample.

1. Weight Calculations: The weight of a steel component can be calculated using the formula:

W = ρ × V
where W is the weight, ρ is the density, and V is the volume of the steel component.

1. Buoyancy and Archimedes’ Principle: As mentioned earlier, the buoyant force acting on a submerged steel sample can be used to determine its density using Archimedes’ principle:

F_b = ρ_fluid × V × g
where F_b is the buoyant force, ρ_fluid is the density of the fluid, V is the volume of the steel sample, and g is the acceleration due to gravity.

1. Density-Related Material Properties: The density of steel is closely related to other material properties, such as its strength, stiffness, and thermal conductivity. Understanding these relationships can aid in the selection and application of steel in various engineering and design contexts.

By mastering these advanced concepts and formulas, you can develop a deeper understanding of steel density and its practical implications, enabling you to make informed decisions and optimize the performance of steel-based systems and structures.

Numerical Examples and Problem-Solving

To further solidify your understanding of steel density, let’s explore some numerical examples and problem-solving scenarios:

Example 1: Calculating the Weight of a Steel Beam

Suppose you have a steel beam with the following dimensions:
– Length: 5 meters
– Width: 0.2 meters
– Height: 0.3 meters

Given that the density of the steel is 7.85 g/cm³, calculate the weight of the beam.

Solution:
1. Calculate the volume of the beam:
Volume = Length × Width × Height
Volume = 5 m × 0.2 m × 0.3 m = 0.3 m³
2. Convert the volume to cubic centimeters:
Volume = 0.3 m³ × (100 cm/m)³ = 300,000 cm³
3. Calculate the weight of the beam:
Weight = Density × Volume
Weight = 7.85 g/cm³ × 300,000 cm³ = 2,355,000 g = 2,355 kg

Therefore, the weight of the steel beam is approximately 2,355 kg.

Example 2: Determining the Density of a Steel Alloy

A sample of a steel alloy has a mass of 50 grams and occupies a volume of 6.35 cm³. Calculate the density of the steel alloy.

Solution:
1. Apply the formula for density:
Density = Mass / Volume
Density = 50 g / 6.35 cm³ = 7.87 g/cm³

Therefore, the density of the steel alloy is approximately 7.87 g/cm³.

Example 3: Buoyancy and Archimedes’ Principle

A steel block with a mass of 10 kg is submerged in water. The buoyant force acting on the block is 98 N. Calculate the volume of the steel block.

Solution:
1. Apply Archimedes’ principle:
Buoyant force = Density of fluid × Volume of object × Acceleration due to gravity
98 N = 1 g/cm³ × Volume × 9.8 m/s²
2. Rearrange the equation to solve for the volume:
Volume = Buoyant force / (Density of fluid × Acceleration due to gravity)
Volume = 98 N / (1 g/cm³ × 9.8 m/s²)
Volume = 10 L = 10,000 cm³

Therefore, the volume of the steel block is 10,000 cm³.

These examples demonstrate the practical application of steel density calculations in various engineering and design scenarios. By working through these problems, you can develop a deeper understanding of the concepts and their real-world implications.

Conclusion

In this comprehensive guide, we have explored the intricacies of steel density, its measurement, factors that influence it, and its practical applications across various industries. From understanding the fundamental principles to delving into advanced concepts and problem-solving, this guide provides a thorough exploration of steel density, equipping you with the knowledge and tools necessary to make informed decisions and optimize the performance of steel-based systems and structures.

References

1. What is the Density of Steel? – Service Steel Warehouse. (2024-03-28). Retrieved from https://www.servicesteel.org/resources/density-of-steel
2. Accurate density measurement of stainless steel weights by hydrostatic weighing system. (2019-01-01). Retrieved from https://www.sciencedirect.com/science/article/abs/pii/S0263224118307723
3. Mechanical properties of structural steel – GovInfo. (n.d.). Retrieved from https://www.govinfo.gov/content/pkg/GOVPUB-C13-8620f9e60cbfd1c3ac9e0bf55ba3770c/pdf/GOVPUB-C13-8620f9e60cbfd1c3ac9e0bf55ba3770c.pdf
4. Density of Metals and Alloys. (n.d.). Retrieved from https://www.engineeringtoolbox.com/metal-alloys-densities-d_50.html
5. Archimedes’ Principle and Buoyancy. (n.d.). Retrieved from https://www.physicsclassroom.com/class/fluid/Lesson-1/Archimedes-Principle-and-Buoyancy