Steam turbines convert kinetic energy/pressure energy to mechanical energy; these are used for electricity production by coupling the turbine with a generator.
The practical steam turbine efficiency varies with the turbine’s size, type, and frictional losses. Although the maximum value reaches 50% for a 1200MW turbine, small turbines have less efficiency. The efficiency of the steam turbine is maximised by expanding the steam in different stages instead of a single stage.
Impulse and reaction turbines are two types of steam turbine; the efficiency of these turbines varies. The upcoming section explains the equation of efficiencies.
Steam turbine efficiency formula
Many parameters control steam turbine efficiency. The steam turbine is equipped with a nozzle/stator and rotor. Hence, the efficiency of each component affects turbine efficiency.

The basic formula for the calculation of turbine efficiency is
Efficiency = Work done on turbine/input kinetic energy of steam
First, let’s define some of the efficiencies.
Blade efficiency
The blade efficiency is defined as, The ratio of work done on the blades divided by the input kinetic energy.
Nozzle efficiency
Each stage of the impulse turbine is equipped with a nozzle and blades. Hence, overall efficiency is affected by the nozzle efficiency,
The nozzle efficiency is defined as; the ratio of output kinetic energy from the nozzle to the difference in the inlet and exit enthalpies of the steam.
Stage efficiency
The overall efficiency of the combination of nozzle and blade stage is known as stage efficiency.
The stage efficiency is obtained by multiplying the blade efficiency with nozzle efficiency.
Isentropic efficiency
The isentropic efficiency is thermodynamic efficiency. This is also known as the 2nd law efficiency of the turbine.
The isentropic efficiency is the ratio of actual work produced in the turbine to the maximum possible work produced if the ideal isentropic process has occurred.
Efficiency of impulse turbine
The impulse turbine utilises the kinetic energy of the steam and converts it to mechanical energy. The steam pressure energy is converted to kinetic energy with the help of a nozzle before entering the rotor blades in impulse turbine.
The final efficiency of one stage, i.e. one nozzle and blade set of impulse steam turbine, is given as,
(1) 
(2) 
Where blade efficiency is,
(3) 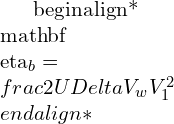
Where, U is the blade speed, V1 is the velocity of inlet steam from nozzle and ΔVw is the difference between whirl component of inlet and exit velocity
And Nozzle efficiency is,
(4) 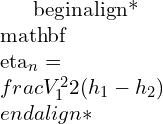
Where, h1 and h2 is inlet and exit enthalpy of the steam respectively.
Let’s do the detailed analysis of stage efficiency,
The velocity triangle of impulse turbine is given below.

In the figure, the steam enters from the top and leaves through the bottom.
Vr is the relative velocity of steam
V is the absolute velocity of steam
Vw is the whirl component of steam velocity and Vf is the flow component of steam velocity.
U is the blade velocity
Α is the guide vane angle and β is the blade angle
The suffix 1 and 2 represents inlet and exit, respectively.
The whirl component is helping to rotate the blade and the flow component helps the flow of steam over the turbine. Hence, a momentum is created in the direction of blade rotation due to the difference in whirl component. Applying the law of moment of momentum gives
(5) ![]()
The r1=r2=r for an impulse turbine.
Hence,
(6) 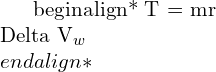
Now,
(7) 
(8) 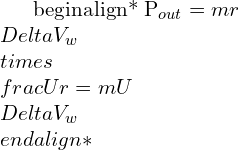
(9) 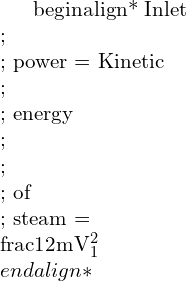
Hence the final blade efficiency is
(10) 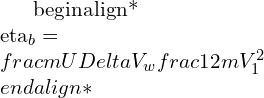
(11) 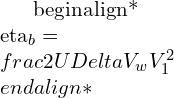
Substituting blade efficiency and nozzle efficiency in stage efficiency equation,
(12) 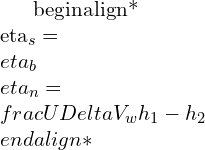
Now let’s find out the ΔVw,
(13) 
(14) 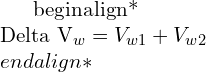
From velocity triangle,
(15) 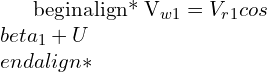
(16) 
Substituting these give,
(17) 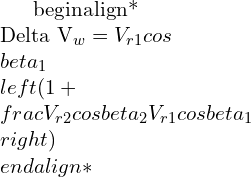
(18) 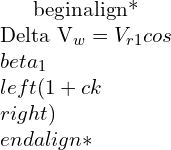
Where,
(19) 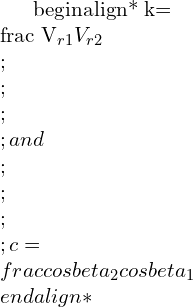
Applying ΔVw on blade efficiency equation,
(20) 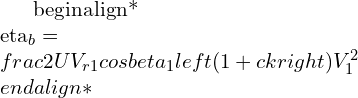
From velocity triangle,
(21) 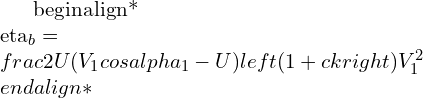
(22) 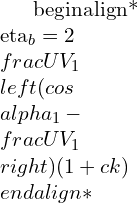
k is the ratio of relative velocities, for perfect smooth blades, k = 1 and otherwise, k is less than 1.
Differentiating the efficiency equation with respect to U/V1 and equating to zero gives the criteria for maximum turbine efficiency. U/V1 is known as blade speed ratio.
Efficiency of Reaction turbine
Let’s analyse the efficiency of reaction turbine by analysing the most commonly used Parson’s reaction turbine.The degree of reaction of parson turbine is 50%. The rotor and stator are symmetrical and velocity triangles are similar.
The final blade efficiency equation of Parson’s Turbine is given below,
(23) 
The reaction turbine uses the reaction force to generate the power. The steam flow over the stator, the stator itself acts as convergent nozzle. The flow to rotor is controlled by fixed vanes known as stator. In impulse turbine the pressure remains constant while the steam flows over the rotor, however, in the reaction turbine the pressure drops while steam flows over the rotor.
Let’s derive the efficiency equation.
Figure shows the velocity triangle of Parson’s reaction turbine.
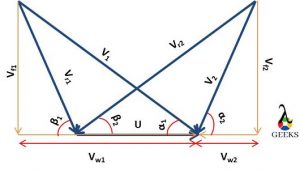
In the reaction turbine, the primary objective is to find out the total energy supplied by the steam.
In the case of reaction turbine, the energy is supplied in the form of pressure energy also, additional to the kinetic energy. Therefore, the equation of input energy includes the term for kinetic energy and pressure energy. The pressure energy term can be represented with the change in total relative velocity.
Finally, the total input energy
In the reaction turbine, the primary objective is to find out the total energy supplied by the steam.
In the case of reaction turbine, the energy is supplied in the form of pressure energy also, additional to the kinetic energy. Therefore, the equation of input energy includes the term for kinetic energy and pressure energy. The pressure energy term can be represented with the change in total relative velocity.
Finally, the total input energy
(24) 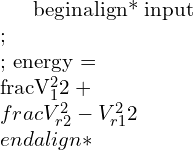
For parson’s turbine, V1 = Vr2, V2 = Vr1, α1=β2 and α2=β1
Applying these conditions,
(25) 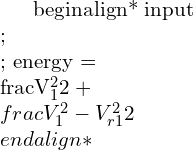
(26) 
From input velocity triangle, applying Cosine rule,
(27) 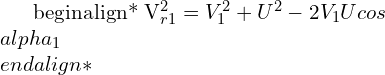
Hence, the input energy equation becomes,
(28) 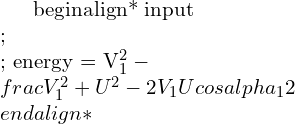
(29) 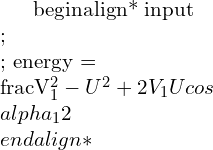
The work done is similar to impulse turbine,
(30) 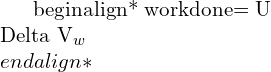
(31) 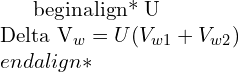
(32) 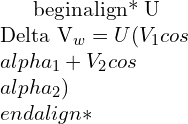
(33) 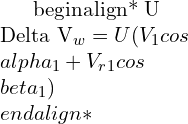
Where,
(34) 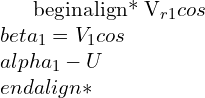
Hence,
(35) 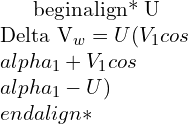
Finally, ,
(36) 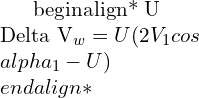
Hence the equation efficiency,
(37) 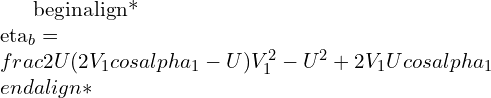
Condition for maximum efficiency of steam turbine
It is always better to operate the turbine in maximum efficiency.
By analysing the efficiency equation explained above, the variable that we can change is U/V1 , hence by differentiating the equation with respect to U/V1 and equating it to zero yields the condition for maximum efficiency.
Condition for maximum efficiency of impulse turbine
The equation for maximum efficiency of impulse turbine is,
(38) 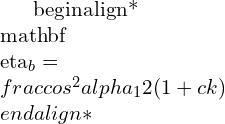
Now, let’s derive the equation for maximum efficiency.
The blade efficiency equation of impulse turbine is,
(39) 
Differentiating it with respect to , For simplification let’s take ρ = U/V1
Hence,
(40) ![Rendered by QuickLaTeX.com \\begin{align*} \\frac{d \\eta_b}{d \\rho}=2(1+ck)\\left[\\left(cos \\alpha_1-\\frac{U}{V_1} \\right )-\\frac{U}{V_1} \\right ]\\end{align*}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-5693bc70259d43e2071d4a33bb56b8b1_l3.png)
Equation it to zero gives,
(41) ![Rendered by QuickLaTeX.com \\begin{align*} 2(1+ck)\\left[\\left(cos \\alpha_1-\\frac{U}{V_1} \\right )-\\frac{U}{V_1} \\right ] = 0\\end{align*}](https://lambdageeks.com/wp-content/ql-cache/quicklatex.com-ecaacc4c250263d730dde482b6037ed1_l3.png)
(42) 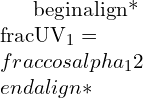
This is the condition for maximum efficiency.
Applying this condition to efficiency equation yields the maximum blade efficiency.
(43) 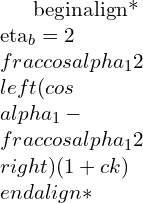
(44) 
If blades are equiangular, β1=β2, hence c = 1, and for smooth blades k=1.
Finally, the maximum efficiency of impulse turbine with equiangular smooth blades is,
(45) 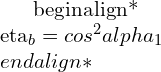
Condition for maximum efficiency of reaction turbine
The equation for maximum efficiency of parson’s reaction turbine is,
(46) 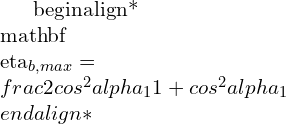
Now, let’s derive the equation.
The efficiency equation of Parson’s reaction turbine is,
(47) 
Let’s take ρ =U/V1
Then,
(48) 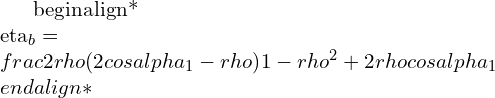
Differentiating this with respect to ρ
(49) 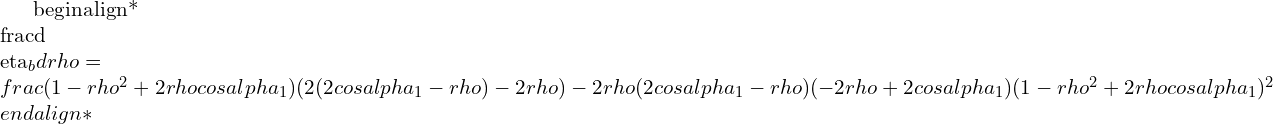
Equating the above equation to zero yields,
(50) 
Applying this on efficiency equation yields the maximum efficiency,
(51) 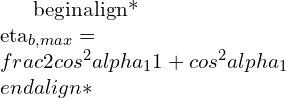
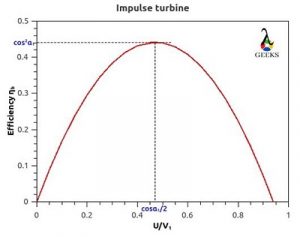
Steam turbine efficiency curve
The curve between ρ and is efficiency curve.
The efficiency curve for equiangular smooth impulse turbine for α=20o is shown below,
The efficiency curve of parson’s reaction turbine for α=20o is shown below,

Factors affecting steam turbine efficiency
Now, we can easily the take out the factors affecting the steam turbine by looking into the efficiency equation.
The factors affecting steam turbine,
- The blade angle (α1)
- Inlet steam velocity (V1)
- The smoothness of turbine blade (k)
- Blade angle on the rotor.
- The blade velocity (U)
Thermal efficiency of steam turbine
The steam power plants are based on Rankine cycle. Hence, the efficiency of the plant is calculated based on the Rankine cycle
The thermal efficiency of steam turbine power plant is defined as,
(52) 
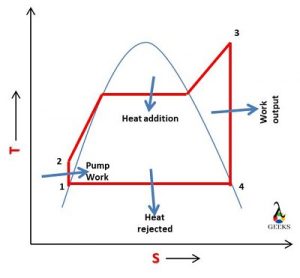
The figure shows the ideal Rankine cycle, from the figure the thermal efficiency can be calculated as,
(53) 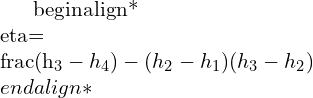
How to calculate steam turbine efficiency?
The efficiency is the ratio of obtained work to given work.
The efficiency of steam turbine can be calculated by measuring the amount of work produced by the turbine to the amount of energy supplied. The supplied energy depends on the steam input, and output power depends on the turbine.
The equation to calculate the turbine efficiencies are explained in previous sections.
In a steam power plant, we calculate the efficiency by calculating the ratio amount of electricity produced to the energy equivalent of fuel burned. The steam plant efficiency depends each component, which include steam turbine, boiler, pump, electricity generator etc.
How to improve steam turbine efficiency?
The methods to improve steam turbine efficiency are,
- Improve the design of turbine blades.
- Minimise the friction loses.
- Increase steam velocity, achieved by optimising the temperature and pressure of steam.
- Minimise the leakage of steam in turbine

The lambdageeksScience Core SME Team is a group of experienced subject matter experts from diverse scientific and technical fields including Physics, Chemistry, Technology,Electronics & Electrical Engineering, Automotive, Mechanical Engineering. Our team collaborates to create high-quality, well-researched articles on a wide range of science and technology topics for the lambdageeks.com website.
All Our Senior SME are having more than 7 Years of experience in the respective fields . They are either Working Industry Professionals or assocaited With different Universities. Refer Our Authors Page to get to know About our Core SMEs.