The Definitive Guide to Quartz Density: A Comprehensive Exploration

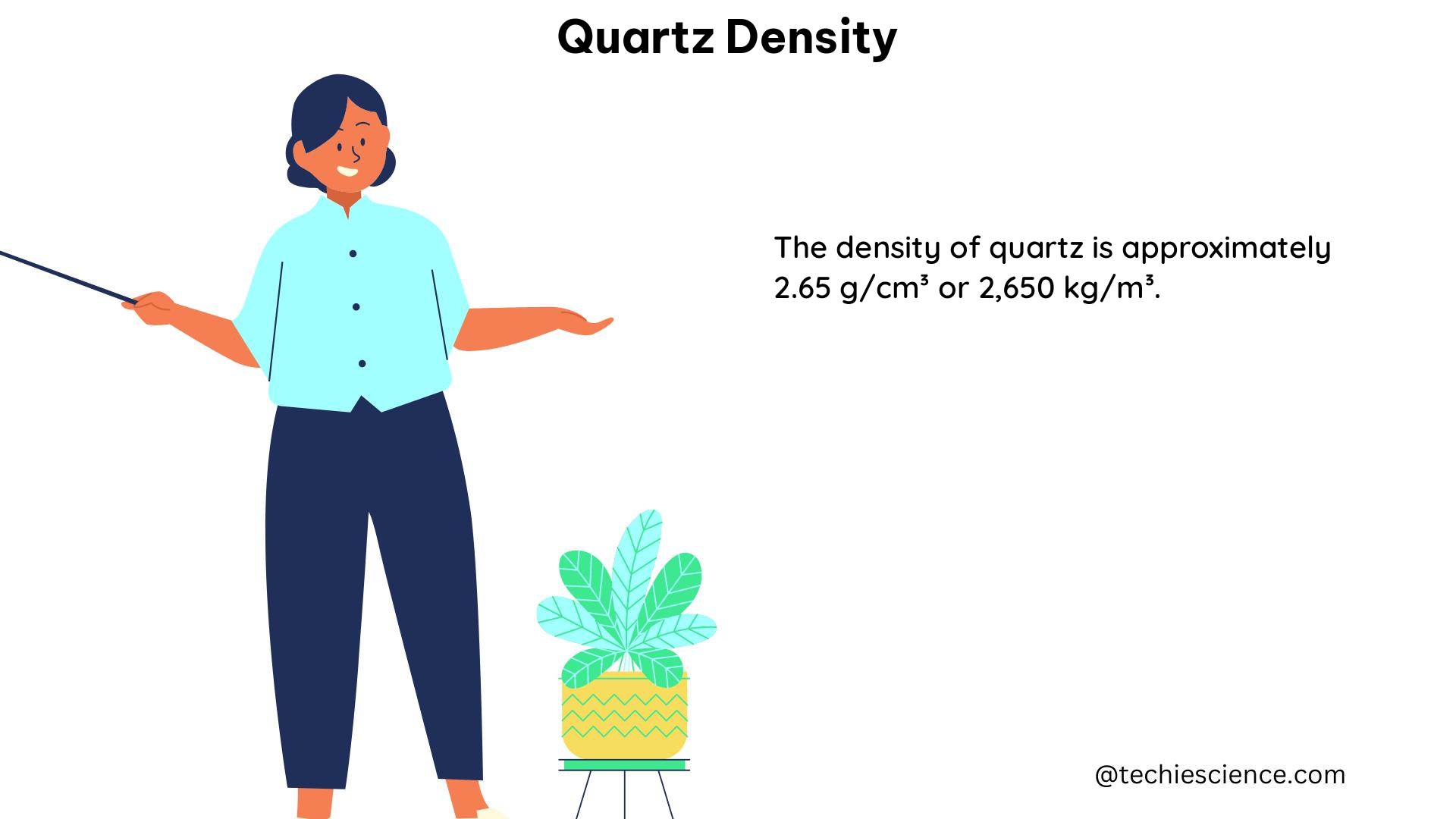
Quartz, a ubiquitous mineral found in the Earth’s crust, is known for its remarkable density of approximately 2.65 g/mL. This value, which is the accepted density for quartz, serves as a standard for comparison in various scientific experiments and applications. In this comprehensive guide, we will delve into the intricacies of quartz density, exploring its measurement, factors affecting it, and its practical implications in the world of physics and beyond.

Understanding Quartz Density

Quartz, a crystalline form of silicon dioxide (SiO2), is one of the most abundant minerals on Earth. Its density, a measure of the mass per unit volume, is a crucial property that has far-reaching implications in various fields, from geology and materials science to precision metrology.

The accepted density value of quartz, 2.65 g/mL, is the result of extensive research and experimentation. This value is widely used as a reference point for comparison in experiments and calculations involving quartz samples.

Factors Affecting Quartz Density

The density of quartz can be influenced by several factors, including:

1. Purity: The presence of impurities or inclusions within the quartz crystal can affect its density. Highly pure quartz crystals will have a density closer to the accepted value of 2.65 g/mL.

2. Temperature: The density of quartz can vary slightly with changes in temperature. As the temperature increases, the volume of the quartz crystal expands, leading to a slight decrease in density.

3. Pressure: Increased pressure can cause the quartz crystal to compress, resulting in a higher density. This relationship is described by the Clausius-Clapeyron equation.

4. Crystalline Structure: Different polymorphs of quartz, such as α-quartz and β-quartz, can have slightly different densities due to variations in their atomic arrangements.

Measuring Quartz Density

Accurately measuring the density of quartz samples is crucial for various applications. Several methods are commonly used to determine the density of quartz, including:

1. Hydrostatic Weighing: This method involves submerging a weighed quartz sample in a liquid, typically water, and measuring the volume displacement. The density is then calculated using the formula: density = mass / volume.

2. Pycnometry: In this technique, a pycnometer, a calibrated glass vessel with a known volume, is used to measure the volume of a quartz sample. The density is then calculated by dividing the mass of the sample by its volume.

3. Helium Pycnometry: This method utilizes the displacement of helium gas to determine the volume of a quartz sample, allowing for the calculation of its density.

4. X-ray Diffraction (XRD): By analyzing the diffraction patterns of X-rays interacting with the quartz crystal structure, the atomic spacing and unit cell parameters can be determined, enabling the calculation of the material’s density.

Precision and Accuracy in Quartz Density Measurements

Achieving high precision and accuracy in quartz density measurements is crucial, as small variations can have significant implications in various applications. The example provided in the initial question illustrates the importance of consistent and reliable measurements.

In the first example, the two students’ measurements showed significant variations, with values ranging from 2.10 g/mL to 3.20 g/mL, which are not consistently close to the accepted value of 2.65 g/mL. This suggests that the measurements were not precise or accurate, and the errors were likely random rather than systematic.

In the second example, the density of the quartz sample was determined to be 2.65 g/mL, which is the accepted value. This demonstrates the importance of using well-established experimental methods and adhering to standard protocols to ensure reliable and accurate results.

Quartz Density in Precision Mass Metrology

The density of quartz plays a crucial role in precision mass metrology, where the accurate measurement and monitoring of mass artifacts are of paramount importance.

Challenges in Mass Artifact Stability

Over time, the mass of artifacts can vary due to various physical and chemical processes, such as sorption effects. This challenge is particularly pronounced when mass artifacts are transported to different locations, as the varying environmental conditions, including temperature, pressure, and humidity, can lead to unpredictable shifts in the calibrated mass values.

The Quartz Crystal Microbalance (QCM)

One solution to address the issue of mass artifact stability is the use of a well-characterized resonance-based sensor, such as the quartz crystal microbalance (QCM). The QCM is a device that utilizes the piezoelectric properties of quartz crystals to measure minute changes in mass.

The QCM works on the principle that the resonance frequency of a quartz crystal is inversely proportional to the mass of the crystal. By monitoring the changes in the resonance frequency of the quartz crystal, the QCM can detect and quantify even the slightest variations in the mass of the artifact, including those caused by changes in the density of the quartz or other materials.

Applications of QCM in Mass Metrology

The QCM has found widespread applications in precision mass metrology, particularly in the following areas:

1. Mass Artifact Monitoring: The QCM can be used to travel with mass artifacts, providing real-time data on any changes in the mass of the artifact during transportation or storage. This information can help identify potential issues and take corrective actions to maintain the integrity of the mass standard.

2. Surface Adsorption Studies: The QCM’s sensitivity to mass changes can be leveraged to study the adsorption of molecules or particles on the surface of quartz crystals. This information is valuable in fields such as materials science, catalysis, and environmental monitoring.

3. Thin Film Deposition Monitoring: The QCM is commonly used to monitor the deposition of thin films in various manufacturing processes, such as semiconductor fabrication and optical coatings, where precise control of film thickness is crucial.

4. Biosensing Applications: The QCM’s ability to detect minute mass changes has made it a valuable tool in the field of biosensing, where it can be used to study biomolecular interactions, detect pathogens, and monitor cellular processes.

Numerical Examples and Data Points

To further illustrate the concepts of quartz density, let’s consider the following numerical examples and data points:

1. Quartz Density Variation with Temperature:
2. At 0°C, the density of quartz is approximately 2.66 g/mL.
3. At 20°C, the density of quartz is approximately 2.65 g/mL (the accepted value).

4. At 100°C, the density of quartz is approximately 2.63 g/mL.

5. Quartz Density Variation with Pressure:

6. At 1 atm (standard atmospheric pressure), the density of quartz is 2.65 g/mL.

7. At 1000 atm (high pressure), the density of quartz increases to approximately 2.67 g/mL.

8. Quartz Density Measurement Precision:

9. In a well-controlled laboratory setting, the density of quartz can be measured with a precision of ±0.01 g/mL.

10. In field or industrial settings, the precision may be lower, with a typical range of ±0.05 g/mL.

11. Quartz Density in Different Polymorphs:

12. α-quartz (the most common polymorph) has a density of 2.65 g/mL.
13. β-quartz has a slightly lower density of 2.53 g/mL.

14. Amorphous silica (non-crystalline quartz) has a density of approximately 2.2 g/mL.

15. Quartz Density in Natural Samples:

16. Pure, well-crystallized quartz samples can have a density very close to the accepted value of 2.65 g/mL.
17. Quartz samples with inclusions or impurities may have slightly lower densities, typically in the range of 2.60-2.64 g/mL.
18. Highly fractured or porous quartz samples may have even lower densities, down to around 2.55 g/mL.

These numerical examples and data points provide a more comprehensive understanding of the factors that can influence the density of quartz, as well as the typical ranges and precision levels encountered in various measurement scenarios.

Conclusion

Quartz, with its well-defined density of approximately 2.65 g/mL, is a fundamental material in the world of physics and beyond. Understanding the intricacies of quartz density, its measurement, and the factors that can affect it, is crucial for a wide range of applications, from geological studies to precision mass metrology.

The use of the quartz crystal microbalance (QCM) as a resonance-based sensor has emerged as a valuable tool in addressing the challenges of mass artifact stability, particularly during transportation and storage. By monitoring the changes in the mass of artifacts, the QCM can provide valuable insights and help maintain the integrity of mass standards.

As we continue to explore the fascinating world of quartz and its properties, the knowledge gained from this comprehensive guide will undoubtedly prove invaluable for physics students, researchers, and professionals working in various fields where the precise understanding of quartz density is paramount.

References:

1. Quartz Density and Its Measurement
2. Factors Affecting Quartz Density
3. Quartz Crystal Microbalance in Mass Metrology
4. Polymorphs of Quartz and Their Densities