The Comprehensive Guide to Projectile Motion Formula

Projectile motion is a form of motion where an object is thrown or projected into the air, and it moves under the influence of gravity alone. The motion can be broken down into horizontal and vertical components, and the kinematic equations for constant acceleration can be applied separately to each component. The acceleration due to gravity is typically given as -9.8 m/s^2.

Understanding the Projectile Motion Formulas

The range, time of flight, and maximum height of a projectile can be calculated using the following formulas:

1. Range (R):
2. Formula: R = v_0^2 * sin(2θ) / g

3. Where:

   • v_0 is the initial velocity
   • θ is the angle of projection
   • g is the acceleration due to gravity

4. Time of Flight (T):

5. Formula: T = 2 * v_0 * sin(θ) / g

6. Where:

   • v_0 is the initial velocity
   • θ is the angle of projection
   • g is the acceleration due to gravity

7. Maximum Height (H):

8. Formula: H = v_0^2 * sin^2(θ) / (2 * g)
9. Where:
   • v_0 is the initial velocity
   • θ is the angle of projection
   • g is the acceleration due to gravity

When a projectile lands at a different height from that of launch, the time of flight and impact velocity can be calculated using the following formulas:

1. Time of Flight (T) with Different Launch and Landing Heights:
2. Formula: T = (v_0 * sin(θ) + sqrt((v_0 * sin(θ))^2 + 2 * g * Δy)) / g

3. Where:

   • v_0 is the initial velocity
   • θ is the angle of projection
   • g is the acceleration due to gravity
   • Δy is the difference in height between the launch and landing points

4. Impact Velocity (v_i) with Different Launch and Landing Heights:

5. Formula: v_i = sqrt(v_0^2 * cos^2(θ) + 2 * g * Δy)
6. Where:
   • v_0 is the initial velocity
   • θ is the angle of projection
   • g is the acceleration due to gravity
   • Δy is the difference in height between the launch and landing points

The trajectory of a projectile can be calculated using the following equations:

1. Horizontal Motion (x):
2. Formula: x = v_0 * cos(θ) * t

3. Where:

   • v_0 is the initial velocity
   • θ is the angle of projection
   • t is the time

4. Vertical Motion (y):

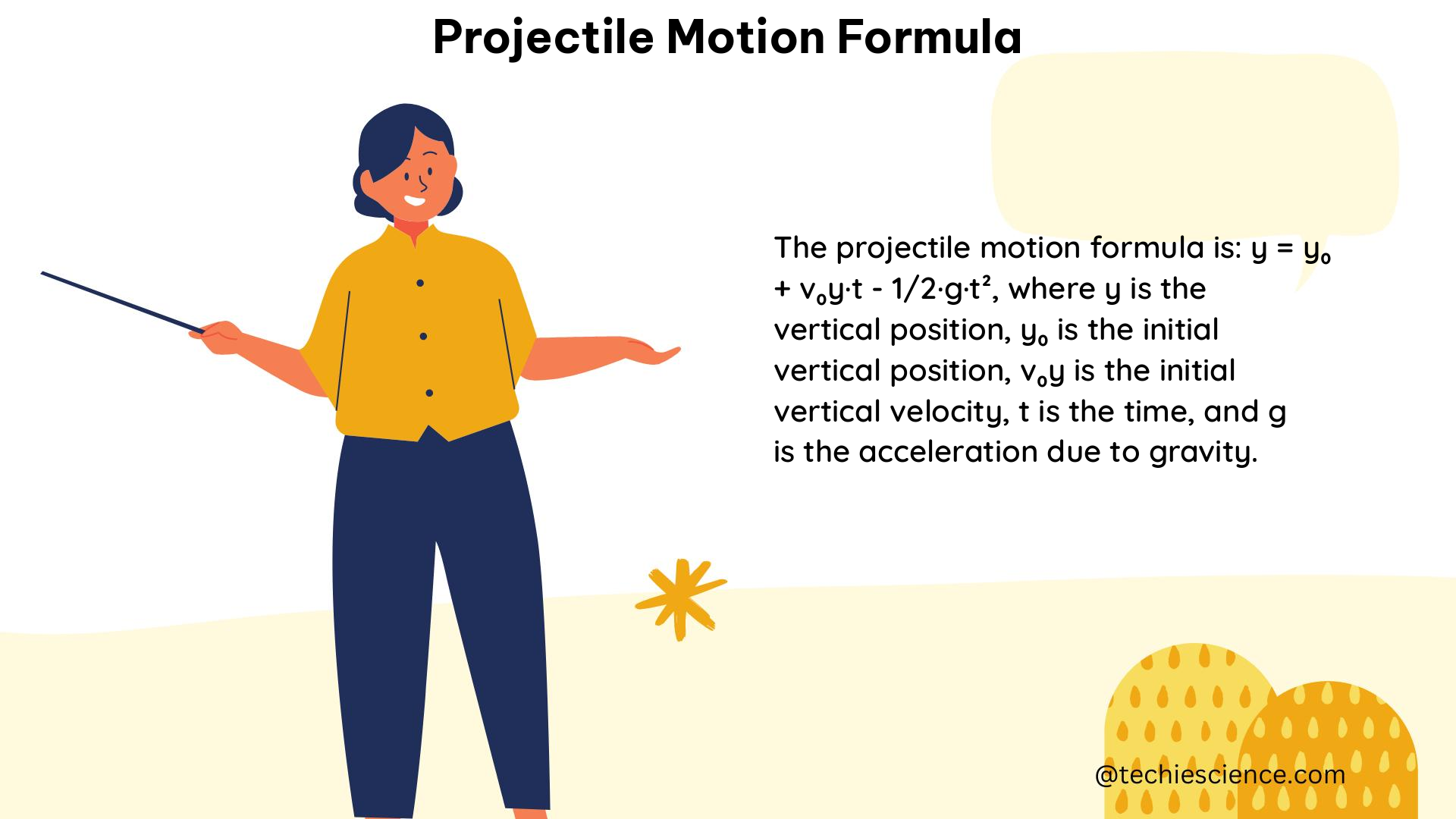
5. Formula: y = v_0 * sin(θ) * t - 0.5 * g * t^2
6. Where:
   • v_0 is the initial velocity
   • θ is the angle of projection
   • g is the acceleration due to gravity
   • t is the time

Projectile Motion Examples and Numerical Problems

Let’s consider an example of a cannonball launched at 120 m/s at an angle of 35° above the horizontal.

1. Initial Vertical Launch Velocity (v_0y):
2. Formula: v_0y = v_0 * sin(θ)

3. Calculation: v_0y = 120 m/s * sin(35°) = 64.24 m/s

4. Initial Horizontal Launch Velocity (v_0x):

5. Formula: v_0x = v_0 * cos(θ)

6. Calculation: v_0x = 120 m/s * cos(35°) = 99.14 m/s

7. Maximum Vertical Displacement (H):

8. Formula: H = v_0y^2 / (2 * g)

9. Calculation: H = (64.24 m/s)^2 / (2 * 9.8 m/s^2) = 211.85 m

10. Time to Reach Maximum Height (t):

11. Formula: t = v_0y / g

12. Calculation: t = 64.24 m/s / 9.8 m/s^2 = 6.56 s

13. Horizontal Displacement when Returning to Launch Height (R):

14. Formula: R = v_0x * t
15. Calculation: R = 99.14 m/s * 6.56 s = 649.91 m

These calculations demonstrate how the projectile motion formulas can be applied to determine the various parameters of a projectile’s trajectory.

Additional Considerations and Factors

1. Air Resistance: In real-world scenarios, air resistance can have a significant impact on the projectile’s motion. The formulas presented here assume negligible air resistance, but in more advanced analyses, air resistance should be taken into account.

2. Angle of Projection: The angle of projection, θ, is a crucial parameter in determining the projectile’s range, time of flight, and maximum height. Varying the angle of projection can significantly affect the projectile’s behavior.

3. Initial Velocity: The initial velocity, v_0, is another critical factor in projectile motion. Increasing the initial velocity generally results in a longer range, higher maximum height, and longer time of flight.

4. Acceleration due to Gravity: The acceleration due to gravity, g, is typically assumed to be -9.8 m/s^2 on Earth’s surface. However, this value can vary slightly depending on the location and altitude.

5. Coordinate Systems: The formulas presented here use a Cartesian coordinate system, with the x-axis representing the horizontal direction and the y-axis representing the vertical direction. In some cases, it may be more convenient to use other coordinate systems, such as polar coordinates.

6. Projectile Motion in Three Dimensions: The formulas discussed so far assume a two-dimensional projectile motion, where the motion is confined to a single plane. In some cases, the projectile may have a three-dimensional trajectory, which requires additional considerations and equations.

Conclusion

Projectile motion is a fundamental concept in physics, and understanding the associated formulas is crucial for solving a wide range of problems. By mastering the equations for range, time of flight, maximum height, and trajectory, you can accurately analyze and predict the behavior of projectiles in various scenarios. Remember to consider the factors that can influence projectile motion, such as air resistance, angle of projection, initial velocity, and acceleration due to gravity, to obtain more accurate results.

References

1. Projectile Motion – Foundations of Physics – BC Open Textbooks. Retrieved from https://opentextbc.ca/foundationsofphysics/chapter/projectile-motion/
2. 4.3 Projectile Motion – University Physics Volume 1 | OpenStax. Retrieved from https://openstax.org/books/university-physics-volume-1/pages/4-3-projectile-motion
3. Projectile Motion – University Physics Volume 1. Retrieved from https://pressbooks.bccampus.ca/universityphysicssandbox/chapter/projectile-motion/